1. Introduction
The usual graph theory notions not herein, refer to [1] . The neighborhood of vertex u is denoted by 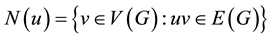 and the close neighborhood of vertex u is denoted by
and the close neighborhood of vertex u is denoted by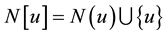 . Let
. Let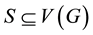 , the neighborhood and closed neighborhood of S are defined as
, the neighborhood and closed neighborhood of S are defined as  and
and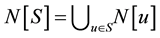 . If
. If , then
, then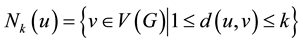 . If
. If 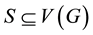 and
and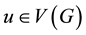 ,
,
then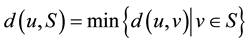 . The diameter of G denoted by
. The diameter of G denoted by 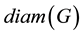 is defined as
is defined as 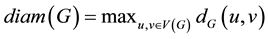 . A set
. A set 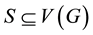 is a dominating set of G if every vertex of
is a dominating set of G if every vertex of 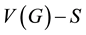 is adjacent to at least one vertex of S. The cardinality of the smallest dominating set of G, denoted by
is adjacent to at least one vertex of S. The cardinality of the smallest dominating set of G, denoted by![]() , is called the domination number of G. A dominating set of cardinality
, is called the domination number of G. A dominating set of cardinality ![]() is called a g-set of G [2] . A dominating set S is a minimal dominating set if no proper subset
is called a g-set of G [2] . A dominating set S is a minimal dominating set if no proper subset ![]() is a dominating set. Given any graph G, its square graph
is a dominating set. Given any graph G, its square graph ![]() is a graph with vertex set
is a graph with vertex set ![]() and two vertices are adjacent whenever they are at distance 1 or 2 in G. For example
and two vertices are adjacent whenever they are at distance 1 or 2 in G. For example![]() . A set
. A set ![]() is a 2-distance dominating set of G if
is a 2-distance dominating set of G if ![]() or 2 for every vertex of
or 2 for every vertex of![]() . The cardinality of the smallest 2-distance dominating set of G, denoted by
. The cardinality of the smallest 2-distance dominating set of G, denoted by![]() , is called 2-distance domination number of G. Every 2-distance dominating set of G is a
, is called 2-distance domination number of G. Every 2-distance dominating set of G is a
dominating set of![]() , so
, so![]() . The Cartesian product of Graphs G and H denoted by
. The Cartesian product of Graphs G and H denoted by ![]() is a
is a
graph with vertex set ![]() and the edge set
and the edge set
![]() . The graph
. The graph ![]() is obtained by
is obtained by
locating copies ![]() of grpah H instead of vertices of G and connecting the corresponding vertices of
of grpah H instead of vertices of G and connecting the corresponding vertices of ![]() to
to ![]() if vertex
if vertex ![]() is adjacent to
is adjacent to ![]() in G.
in G. ![]() is isomorphic to
is isomorphic to![]() . We denote a cycle with n vertices by
. We denote a cycle with n vertices by ![]() and a path with n vertices by
and a path with n vertices by![]() . The bipartite geraph
. The bipartite geraph ![]() is named claw.
is named claw.
2. Preliminaries Results
Theorem 1. Let G be a graph. Then
a) If![]() , then
, then![]() .
.
b) If![]() , then
, then![]() .
.
Proof. a) Every dominating set of ![]() is a dominating set of G so
is a dominating set of G so![]() .
.
b) Every dominating set of G is a dominating set of ![]() so
so![]() .
.
Theorem 2. [3] A dominating set S is a minimal dominating set if and only if for each vertex![]() , one of the following conditions holds:
, one of the following conditions holds:
a) u is an isolated vertex of S.
b) there exist a vertex ![]() for which
for which![]() .
.
Theorem 3. [3] If G is a graph with no isolated vertices and S is a minimal dominating set of G, then ![]() is a dominating set of G.
is a dominating set of G.
Proof. Let S be a g-set of G. S is a minimal dominating set of G. By Theorem 3, ![]() is a dominating set of G too, so
is a dominating set of G too, so![]() , so
, so![]() .
.
Theorem 4. [4] If G is a connected claw free graph, then![]() .
.
Theorem 5. [5] Let G be a graph. Then![]() .
.
Since![]() , by Theorems 4 and 5 we have the following corollary.
, by Theorems 4 and 5 we have the following corollary.
Corollary 6.![]() .
.
Vizing conjecture
Let G and H be two graphs. Then ![]() [6] .
[6] .
3. Domination Number of Square of Graphs
Theorem 7. Let S be a dominating set of![]() . Then S is a minimal dominating set of
. Then S is a minimal dominating set of ![]() if and only if each vertex
if and only if each vertex ![]() satisfies at least one of the following conditions:
satisfies at least one of the following conditions:
a) There exists a vertex ![]() for which
for which![]() .
.
b) ![]() for every vertex
for every vertex![]() .
.
Proof. If ![]() and u does’t satisfy conditions a) and b), then the set
and u does’t satisfy conditions a) and b), then the set ![]() is a dominating set of
is a dominating set of ![]() that is contradiction. Conversely, let S be a dominating set of
that is contradiction. Conversely, let S be a dominating set of ![]() but not minimal. Then there exists a vertex
but not minimal. Then there exists a vertex ![]() such that
such that ![]() is a dominating set of
is a dominating set of![]() , too. So
, too. So ![]() or 2 for every
or 2 for every![]() ; therefore S doesn’t satisfy in condition a). In addition
; therefore S doesn’t satisfy in condition a). In addition ![]() or 2, so S doesn’t satisfy in condition b).
or 2, so S doesn’t satisfy in condition b).
Theorem 8. If![]() , then
, then![]() .
.
Proof. Let![]() . Since
. Since![]() , the set
, the set ![]() is a dominating set of
is a dominating set of![]() . Therefore
. Therefore![]() .
.
Theorem 9. If![]() , then
, then![]() , for every
, for every![]() .
.
Proof. Let u be an arbitrary vertex of G. Let![]() . Since
. Since![]() ,
, ![]() , for every
, for every![]() . Therefore
. Therefore ![]() is a dominating set of
is a dominating set of![]() .
. ![]() and
and![]() , Hence
, Hence![]() .
.
Theorem 10. Let G be a graph. Then![]() .
.
Proof. Let ![]() and
and ![]() be the copies of
be the copies of ![]() in
in ![]() corresponding to the vertices
corresponding to the vertices![]() . Let
. Let ![]() be a g-set of G. Then the set
be a g-set of G. Then the set ![]() that contains a vertex of each copies
that contains a vertex of each copies ![]() is a g-set of
is a g-set of![]() . Since
. Since![]() , the result holds.
, the result holds.
Theorem 11. For every![]() ,
,![]() .
.
Proof. The graphs ![]() and
and ![]() are complete graphs, therefore
are complete graphs, therefore![]() . So the result holds for
. So the result holds for ![]() and
and![]() . Let
. Let![]() ,
,![]() . Since
. Since![]() , by the Theorem 5 we have
, by the Theorem 5 we have![]() . On the other hand by Figure 1 the set
. On the other hand by Figure 1 the set ![]() is a dominating set of size
is a dominating set of size ![]() for
for![]() . So
. So![]() , therefore
, therefore![]() .
.
Theorem 12. For every![]() ,
,![]() .
.
Proof.![]() , and the result holds for these graphs. Let
, and the result holds for these graphs. Let![]() ,
,![]() . Since
. Since![]() , by Theorem 5 we have
, by Theorem 5 we have![]() . By Figure 2 the set:
. By Figure 2 the set:
![]()
is a dominating set of size ![]() for
for![]() , so
, so![]() ; therefore
; therefore![]() .
.
Theorem 13. For every![]() ,
, ![]() , and for every
, and for every![]() ,
,![]() .
.
Proof. The graphs ![]() and
and ![]() have mn vertices and every vertex u dominates at least 13 vertices in
have mn vertices and every vertex u dominates at least 13 vertices in ![]() and
and ![]() (Figure 3), so the result holds.
(Figure 3), so the result holds.
By Theorem 13, ![]() or
or ![]() equals the minimum number of diamonds like Figure 4. we can cover all the vertices of
equals the minimum number of diamonds like Figure 4. we can cover all the vertices of ![]() or
or![]() .
.
![]()
Figure 3. Dominated vertices by u in ![]() and
and![]() .
.
![]()
Figure 4. Dominated vertices by one vertex in ![]() and
and![]() .
.
In this paper we use short display or s.d to show the graphs ![]() and
and ![]() for simplicity; it means that we don’t draw the edges of these graphs and draw only their vertices.
for simplicity; it means that we don’t draw the edges of these graphs and draw only their vertices.
Theorem 14. For every![]() ,
,![]() .
.
Proof. By Theorem 13 we have![]() . In Figure 5 that is s.d of
. In Figure 5 that is s.d of![]() . It is determined by a g-set of size 13 for
. It is determined by a g-set of size 13 for![]() . Therefore
. Therefore![]() ; hence
; hence![]() .
.
We can obtain s.d of ![]() with dominating set of size 13kt for
with dominating set of size 13kt for ![]() by locating kt copies of Figure 5 in k rows and t columns. Hence
by locating kt copies of Figure 5 in k rows and t columns. Hence![]() . By Theorem 13 we have
. By Theorem 13 we have
![]()
Figure 5. A dominating set of size 13 for![]() .
.
![]() , so
, so
Theorem 15.![]() , for every
, for every![]() .
.
Proof. Since![]() , by Theorem 10 and Corollary 6 we have
, by Theorem 10 and Corollary 6 we have
![]()
Theorem 16.![]() ,
, ![]() , and
, and
![]()
Proof. By Theorem 13 we have![]() . In Figure 6 it is determined by a dominating set of size
. In Figure 6 it is determined by a dominating set of size ![]() for
for![]() ,
, ![]() , so for these graphs we have
, so for these graphs we have![]() .
.
In Figure 6, the seventh column of s.d of ![]() (from left to right) is similar to the first column of s.d of
(from left to right) is similar to the first column of s.d of![]() ,
, ![]() and
and![]() ,
,![]() . By setting s.d of k graphs
. By setting s.d of k graphs ![]() and one s.d of
and one s.d of ![]() or
or ![]() or
or ![]() or
or ![]() consecutively from left to right such that the first column of every s.d of graph locates on the last column of s.d of the previous graph, we can obtain a s.d of
consecutively from left to right such that the first column of every s.d of graph locates on the last column of s.d of the previous graph, we can obtain a s.d of ![]() with a dominating set of size
with a dominating set of size ![]() for
for![]() ,
,![]() . By the same setting for s.d of k graphs
. By the same setting for s.d of k graphs ![]() we can obtain a s.d of
we can obtain a s.d of ![]() with a dominating set of size
with a dominating set of size ![]() for
for![]() . Also by the same setting for s.d of
. Also by the same setting for s.d of ![]() graphs
graphs ![]() and one s.d of
and one s.d of ![]() we can obtain a s.d of
we can obtain a s.d of ![]() with a dominating set of size 2k for
with a dominating set of size 2k for![]() .
.
Theorem 17.![]() ,
, ![]() , and
, and
![]()
Proof. By Theorem 13 we have![]() . In Figure 7 it is determined by a dominating set for
. In Figure 7 it is determined by a dominating set for
![]() , and,.
, and,.
By Figure 7 we have![]() ,
,![]() . So for these graphs equality holds.
. So for these graphs equality holds.
In Figure 7, the seventh column of s.d of ![]() (from left to right) is similar to the first column of s.d of
(from left to right) is similar to the first column of s.d of![]() ,
, ![]() and
and![]() ,
,![]() . By setting s.d of k graphs
. By setting s.d of k graphs ![]() and one s.d of
and one s.d of ![]() or
or ![]() or
or ![]() consecutively from left to right such that the first column of every s.d of graph locates on the last column of the previous s.d of graph, we can obtain a s.d of
consecutively from left to right such that the first column of every s.d of graph locates on the last column of the previous s.d of graph, we can obtain a s.d of ![]() with a dominating set of size
with a dominating set of size ![]() for
for![]() ,
,![]() . By the same setting for s.d of k graphs
. By the same setting for s.d of k graphs ![]() we can obtain a s.d of
we can obtain a s.d of ![]() with a dominating set of size
with a dominating set of size ![]() for
for ![]() and by the same setting for s.d of k graphs
and by the same setting for s.d of k graphs ![]() and one s.d of
and one s.d of ![]() we can obtain a s.d of
we can obtain a s.d of ![]() with a dominating set of size
with a dominating set of size ![]() for
for![]() . Also by the same setting for s.d of
. Also by the same setting for s.d of ![]() graphs
graphs ![]() and one s.d of
and one s.d of ![]() we can obtain a s.d of
we can obtain a s.d of ![]() with a dominating set of size 3k for
with a dominating set of size 3k for![]() .
.