1. Introduction
The optimality conditions for systems consisting of only one equation and for n × n systems governed by different types of partial differential equations defined on spaces of functions of infinitely many variables have been discussed for example in [1-11].
In addition, optimal control problems for systems involving operators with an infinite number of variables for non-standard functional and time delay have been introduced in [12,13].
Furthermore, time-optimal control of systems with an infinite number of variables has been studied in [14-18].
Some applications of optimal control problem for systems involving Schrodinger operators are introduced for example in [19-21].
Making use of the theory of Lions [22] and Berezanskiĭ [23], we consider the optimal control problem of distributed type for 2l order (n × n) cooperative systems governed by Dirichlet and Neumann problems involving hyperbolic operators with an infinite number of variables and with variable coefficients. We first prove the existence and uniqueness of the state for these systems, then we find the set of equations and inequalities that characterize the optimal control of these systems. Finally, we impose some constraints on the control. Necessary and sufficient conditions for optimality with control constraints are derived.
This paper is organized as follows. In Section 1, we introduce spaces of functions of an infinite number of variables. In Section 2, we discuss the distributed control problem for these systems with Dirichlet conditions. In Section 3, we consider the problem with Neumann conditions.
2. Sobolev Spaces with an Infinite Number of Variables
This section covers the basic notations, definitions, and properties, which are necessary to present this work [24]. Let 
be a sequence of continuous positive probability weights such that
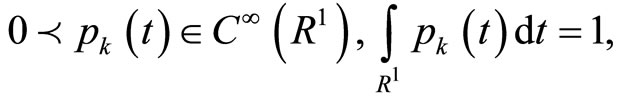
with respect to it we introduce on the region 
, the measure dr(x) by:
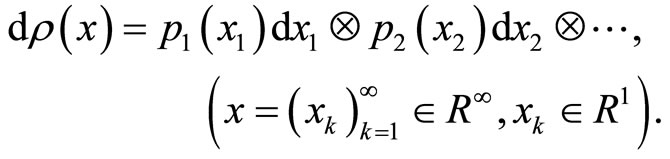
On 
we construct the space 
with respect to this measure such that 
is the space of all square integrable functions on 
i.e.
.
We shall set
.
is a Hilbert space for the scalar product
associated to the above norm.
We consider a Sobolev space in the case of an unbounded region. For functions which are continuously differentiable l times up to the boundary G of 
and which vanish in a neighborhood of ¥, we introduce the scalar product
where 
is defined by
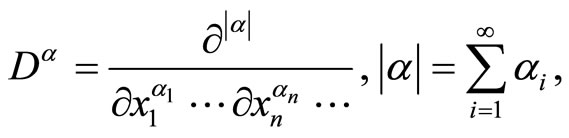
and the differentiation is taken in the sense of generalized function on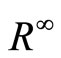
, and after the completion, we obtain the Sobolev space
, which is a Hilbert space and dense in
. The space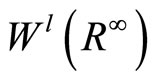 
forms a positive space. We can construct the negative space 
with respect to the zero space 
and then we have the following imbedding
,
.
Analogous to the above chain we have a chain of the form
,
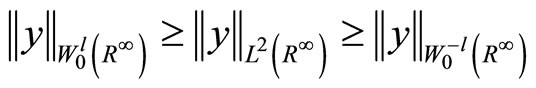where
with the scalar product
and 
is its dual.
denotes the space of measurable function t ® f(t) on open interval (0,T) for the Lebesgue measure dt and such that
endowed with the scalar product
which is a Hilbert space.
Analogously, we can define the spaces
and
then we have a chain in the form
where 
with boundary
.
By the Cartesian product, it is easy to construct the following Sobolev spaces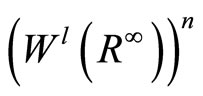 
with the norm defined by
where 
is a vector function and
, also we can construct the Cartesian product for the above Hilbert spaces. Finally we have the following chain:
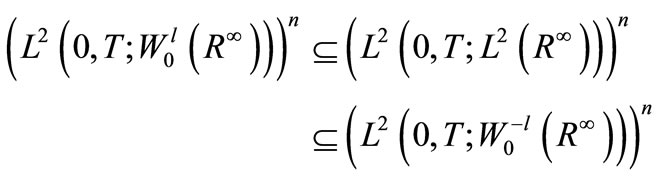
where
and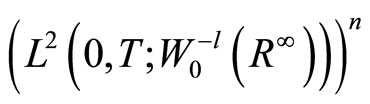 
are the dual spaces of 
and 
resp.
3. Dirichlet Problem for 2l Order (n × n) Cooperative Hyperbolic System with an Infinite Number of Variables and with Variable Coefficients
In this section, we study the existence and uniqueness of solutions for 2l order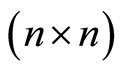 
cooperative systems governed by Dirichlet problems involving hyperbolic operators with an infinite number of variables and with variable coefficients, then we find the necessary and sufficient conditions of the optimal control of distributed type.
For
, we have the following system:
(1)
where
, 
is a given function, and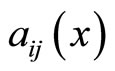 
are bounded functions such that
(2)
(3)
System (1) is called cooperative if (2) holds.
The operator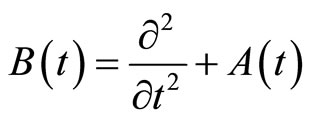 
in system (1) is 2l order hyperbolic operator with an infinite number of variables with
[23] is given by:
(4)
since q(x,t) is a real valued function in x which is bounded and measurable on
, such that
(5)
Definition 1:
For each t Î (0,T), we define a bilinear form
by
where
Then,
,
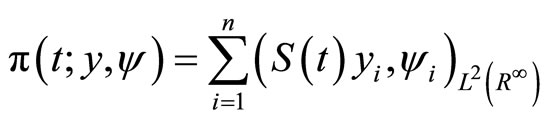(6)
3.1. The Existence and Uniqueness of Solution
Lemma 1:
The bilinear form (6) is coercive on
, that is, there exists c, c1 Î R, such that:
(7)
Proof:
We have,
thus,
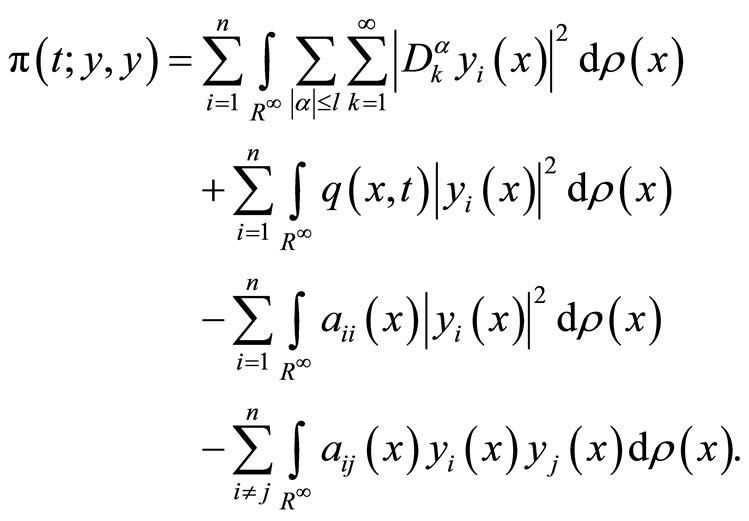
From (2), (3), and (5), we deduce
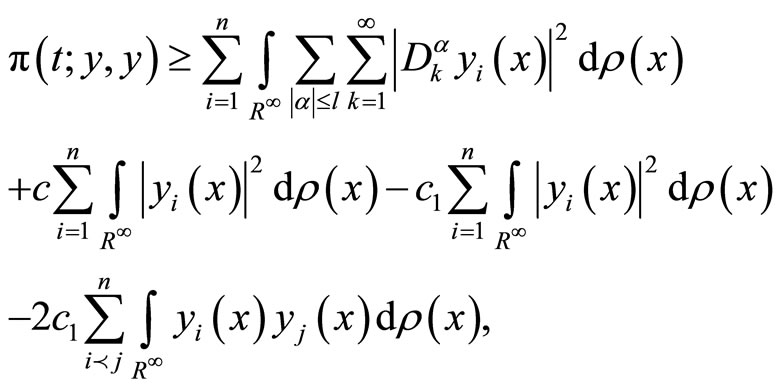
then,
then,
since 0 < c £ 1, we have,
which proves the coerciveness condition on
.
Under all the a bove consideration, theorems of Lions [22] and using the Lax-Milgram lemma we have proved the following theorem.
Theorem 1:
Under the hypotheses (2), (3) and (7), if
, 
and 
are given in
, 
and 
resp., then there exists a unique solution
for system (1).
Proof:
Let 
be a continuous linear form defined on 
by
then by Lax-Milgram lemma, there exists a unique element 
such that
(9)
Now, let us multiply both sides of first equation of system (1) by
, then integration over Q, we have:
by applying Green’s formula
by entering the summation on the both sides, we have
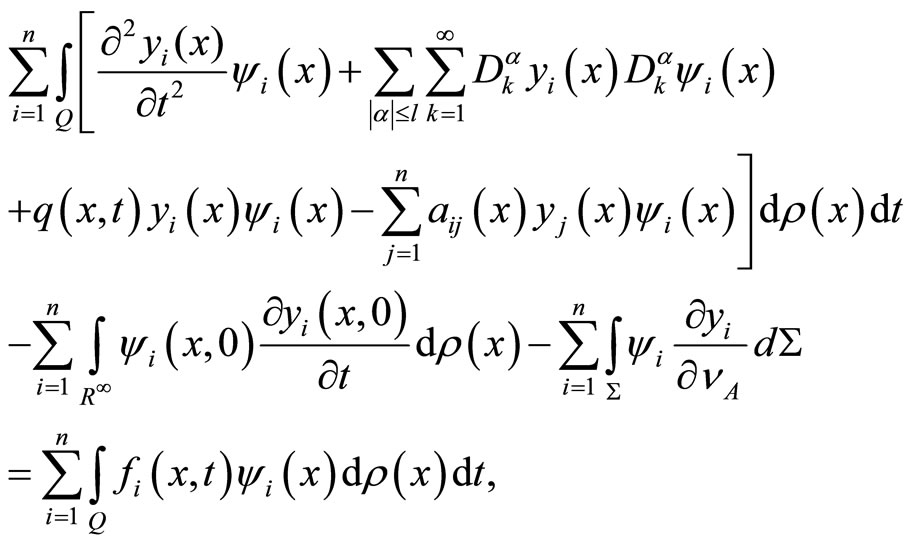
by comparing the summation with (6), (8) and (9) we obtain:
then we deduce that:
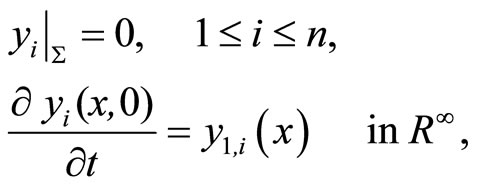
which completes the proof.
3.2. Formulation of Dirichlet Problem
The space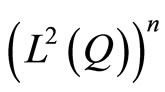 
being the space of controls. For a control
, the state
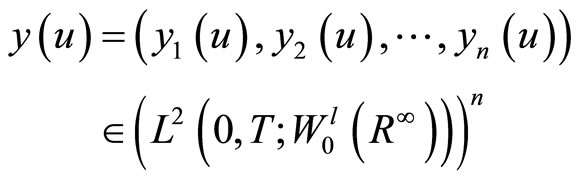
of system (1) is given by the solution of
(10)
.
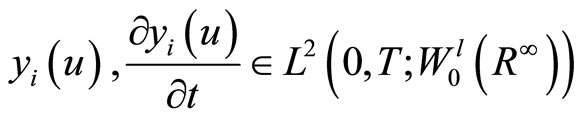The observation equation is given by
.
N is given as
such that,
.
For a given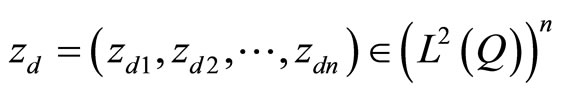
, the cost function is given by
. (11)
4. Control Constraints
The set of admissible controls Uad is a closed convex subset of
, Then the control problem is to find inf J(v) over Uad.
Then using the general theory of Lions [22], there exists a unique optimal control u Î Uad such that J(u) = inf J(v) for all v Î Uad. Moreover, we have the following theorem which gives the necessary and sufficient conditions of optimality.
Theorem 2:
Assume that (7) holds and the cost function is given by (11). The necessary and sufficient conditions for
to be an optimal control are the following equations and inequalities:
(12)
with
,
,
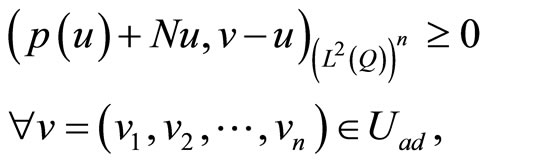(13)
together with (10), where
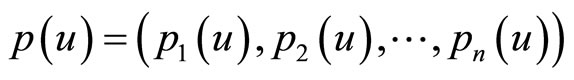
is the adjoint state.
Proof:
The optimal control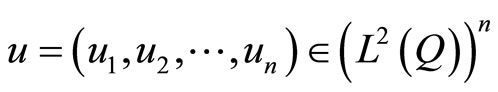 
is characterized by [23]:
that is
this inequality can be written as
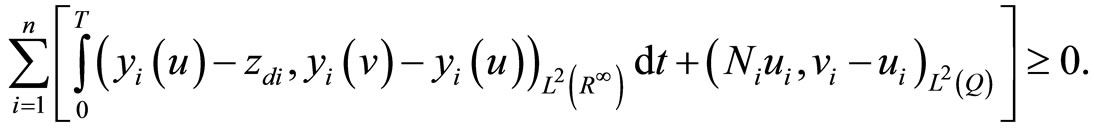(14)
Now, since
by using Green’s formula, (3) and (10), we have
Then
and
(15)
Since the adjoint equation for hyperbolic systems in Lions [22] takes the following form:
then, from (15) we obtain the first equation in (12), and from theorem1, system (12) admits a unique solution which satisfies
.
Now, we transform (14) by using (12) as follows:
using Green’s formula, (10) and (12), we obtain
using (10), we have
which is equivalent to
.
Thus the proof is complete.
5. Neumann Problem for 2l Order (n × n) Cooperative Hyperbolic System with an Infinite Number of Variables and with Variable Coefficients
In this section, we discuss the optimal control for 2l order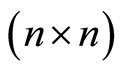 
cooperative non-homogenous Neumann systems involving hyperbolic operators with an infinite number of variables and with variable coefficients.
(16)
where
, for all 1 £ i £ n,
is a given function in
and the operator 
in system (16) is 2l order hyperbolic operator with an infinite number of variables with
is given by:
since q(x,t) is defined as in (5).
For each t Î (0,T), we define a bilinear form
as in (6).
5.1. The Existence and Uniqueness of Solution
Lemma 2:
The bilinear form 
is also coercive on
, that is, there exists c, c1Î R, such that:
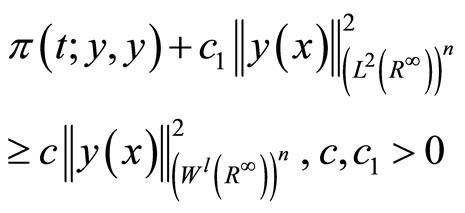(17)
Proof:
Since 
is everywhere dense in 
with topological inclusion, then we have
. (18)
By using (18) in (7), we obtain
which proves the coerciveness condition on
.
By the Lax-Milgram lemma, we shall introduce the following theorem which gives the existence and uniqueness of the state for system (16).
Theorem 3:
Under the hypotheses (2), (3) and (17), if
, 
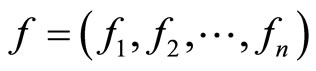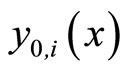
and 
are given in
,
and 
resp., then there exists a unique solution
for system (16).
Proof:
Let 
be a continuous linear form defined on 
by
(19)
then by Lax-Milgram lemma, there exists a unique element
such that (9) is satisfied.
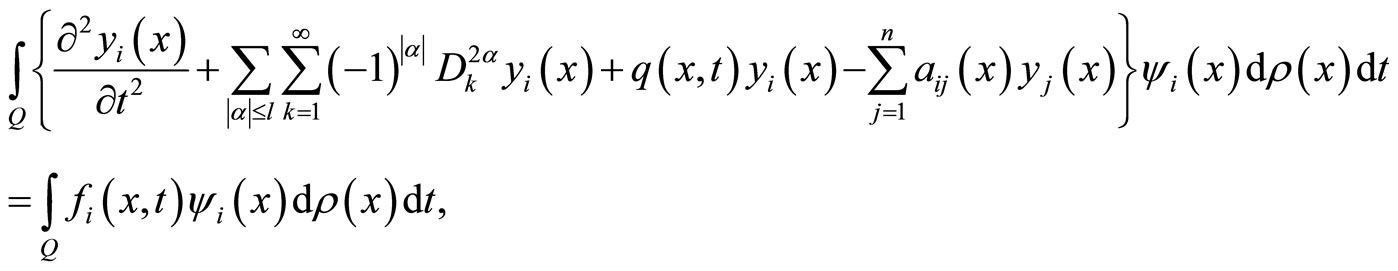
by applying Green’s formula
by entering the summation on the both sides, we have
by comparing the summation with (6), (8) and (9) we obtain:
then we deduce that:
which completes the proof.
5.2. Formulation of Neumann Problem
The space 
is the space of controls. The state
of system (16) is given by the solution of
(20)
.
The observation equation is given by
.
For a given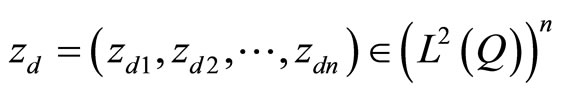
, the cost function is given by
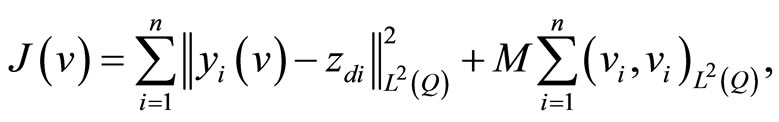(21)
where M is a positive constant.
The control problem then is to find inf J(v) over Uad with the same control constraints in Section II.
Then as in Section II, there exists a unique optimal control uÎ Uad such that
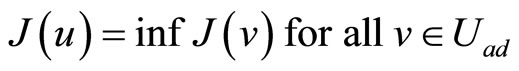. (22)
Under the given considerations, we may apply theorems of Lions [22] as in Section II to obtain the following theorem:
Theorem 4:
The necessary and sufficient conditions for optimality of the control problem (20), (21) and (22) are given by the following equations and inequalities:
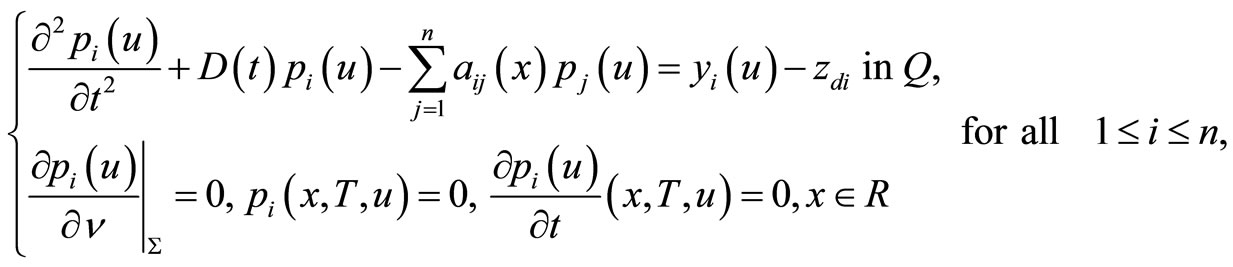(23)
with
,
,
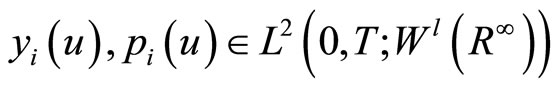together with (16).
The case of no constraints on the control:
In the case of no constraints on the control, i.e.
, the condition (13) reduces to
hence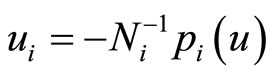
.
Example 1:
If we take n = 2 in Dirichlet problem (1) with the same conditions of coefficients (2) and (3), then the space of controls is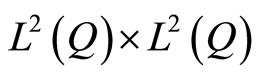
. For a control
the state
of the system is given by the solution of
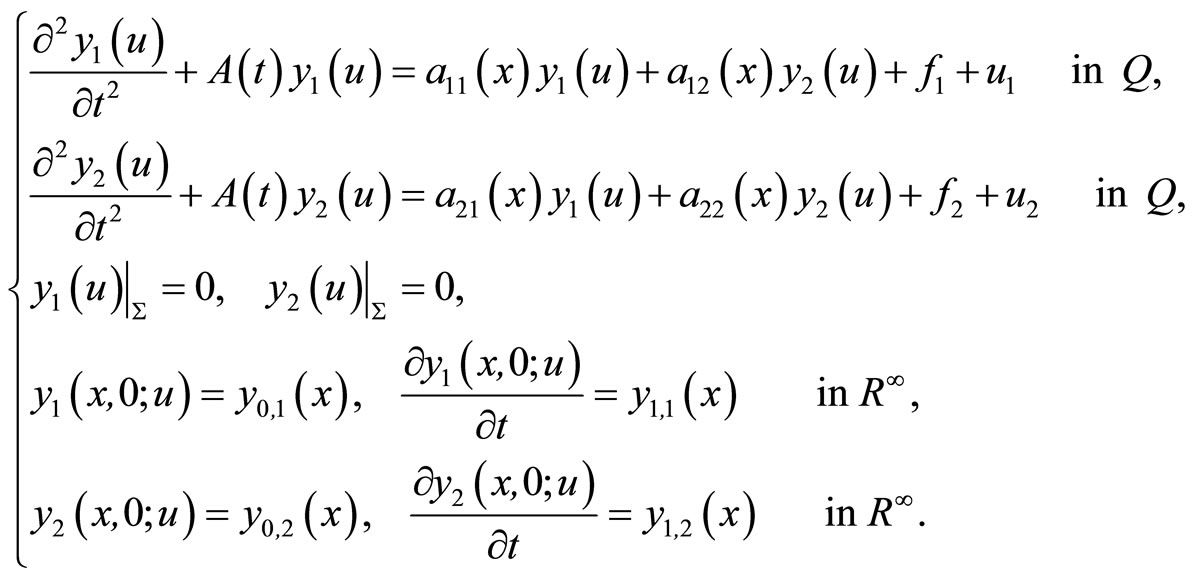(24)
.
The necessary and sufficient conditions for the optimality are the following equations and inequalities:
(25)
,
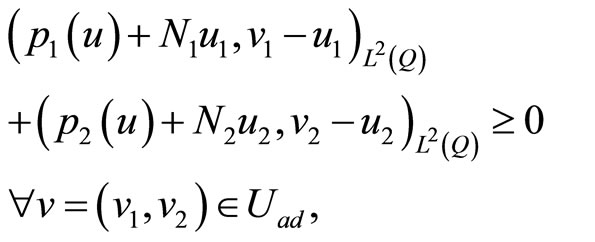(26)
together with (24), where 
is the adjoint state.
Example 2:
If we take
. (27)
Thus there are no constraints on 
then the inequality (26) is equivalent to
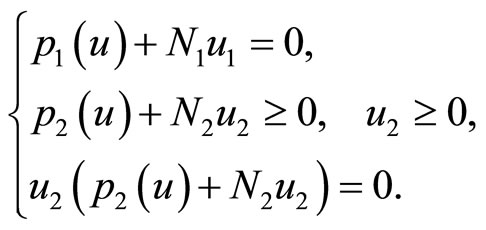(28)
Thus the optimal control is given by the solution of the following set of equations and inequalities
(29)
Further
(30)
6. Conclusions
The main result of this paper finds the necessary and sufficient conditions of optimality of distributed control for 2l order (n ´ n) cooperative systems governed by Dirichlet and Neumann problems involving hyperbolic operators with an infinite number of variables and with variable coefficients that give the characterization of optimal control (Theorem 2, 4).
Also it is evident that by modifying:
• the boundary conditions (Dirichlet, Neumann, mixed)
• the nature of the control (distributed, boundary)
• the nature of the observation (distributed, boundary)
• the initial differential system
• the number of variables
• the type of equation (elliptic, parabolic and hyperbolic)
• the type of coefficients (constant, variable)
• the type of system (non-cooperative, cooperative)
• the order of equationmany of variations on the above problems are possible to study with the help of Lions formalism.