A Model of Spatial Spread of an Infection with Applications to HIV/AIDS in Mali ()
1. Introduction
Let  be an open bounded lipschitzian domain of
be an open bounded lipschitzian domain of  satisfying the cone property in which it will be assumed that the population is fixed. To describe the disease transmission, a traditional
satisfying the cone property in which it will be assumed that the population is fixed. To describe the disease transmission, a traditional  model is introduced. Each member of the population is supposed to belong to one of the these two classes: Susceptible individuals (denoted by
model is introduced. Each member of the population is supposed to belong to one of the these two classes: Susceptible individuals (denoted by ) or Infected individuals (denoted by
) or Infected individuals (denoted by ). Each individual which begins in the class
). Each individual which begins in the class , moves to the class
, moves to the class , having had a contact with an infected person. Infected individuals eventually recover from the disease due to a medical treatment. The disease is assumed to be transmitted from infected to susceptible individuals with a probability
, having had a contact with an infected person. Infected individuals eventually recover from the disease due to a medical treatment. The disease is assumed to be transmitted from infected to susceptible individuals with a probability , by a “mass action” contact term and spreads spatially with the coefficient
, by a “mass action” contact term and spreads spatially with the coefficient . The infected individuals are assumed to recover at a per capita rate of
. The infected individuals are assumed to recover at a per capita rate of . Demographic changes are neglected under the assumption that the duration of the epidemic is short in comparison with the average life span of an individual. Assuming these assumptions to be relevant, we suppose that the following holds: there are positive constants
. Demographic changes are neglected under the assumption that the duration of the epidemic is short in comparison with the average life span of an individual. Assuming these assumptions to be relevant, we suppose that the following holds: there are positive constants
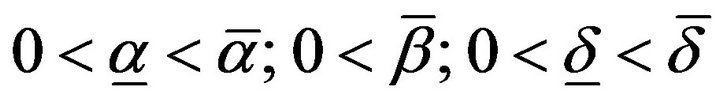
such that functions  and satisfy:
and satisfy: 
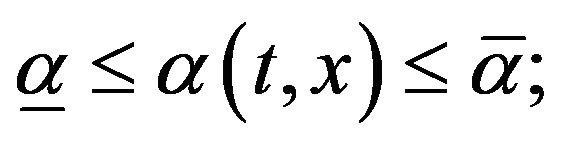

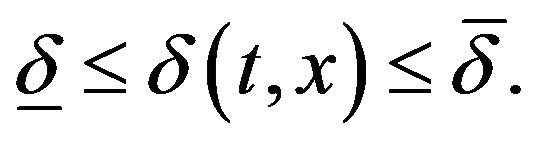
At an initial time , we have two nonnegative, regular
, we have two nonnegative, regular  functions satisfying:
functions satisfying:
 ;
;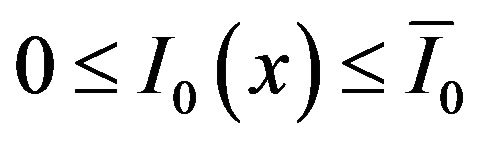 .
.
The no flux boundary conditions mean that the system is isolated.
The propagation of the disease for a fixed  is governed by the following simple model:
is governed by the following simple model:
 (1)
(1)
where  denotes the outward normal vector to
denotes the outward normal vector to .
.
System (1) has also been used to modelling chemistry reactions (with a different sign in reaction term) [1] or combustion phenomenon.
Even if the dynamics of the system (1) is quite simple, the question we address in this work is: are there parameters that allow to control the system in a finite time in case where the spacial diffusion is directed? In [2] a model structured by spatial position in a bounded onedimensional environment is proposed and analyzed. The spatial mobility is assumed to be governed by random diffusion with coefficients  and
and  for the susceptible and infected individuals, respectively.
for the susceptible and infected individuals, respectively.
In the present paper, the susceptible population doesn’t move away, so that its diffusion coefficient is equal to zero. Many other models of epidemics with spatial diffusion are studied, see for example [3,4].
The paper is organized as follows:
In Section 2 some a priori estimates are derived for the solution  of the system (1). In Section 3 the existence and uniqueness of solutions are studied. In Section 4, the existence of coefficients
of the system (1). In Section 3 the existence and uniqueness of solutions are studied. In Section 4, the existence of coefficients  and
and  allowing to control the system (1) in a finite time is derived. This section is ended with some numerical results which take into account the data of the spread of VIH/AIDS in Mali.
allowing to control the system (1) in a finite time is derived. This section is ended with some numerical results which take into account the data of the spread of VIH/AIDS in Mali.
2. A Priori Estimates
We denote by  (respectively,
(respectively, ) the classical Sobolev space of order 1 (respectively, Sobolev space of order 2) [5]. Let
) the classical Sobolev space of order 1 (respectively, Sobolev space of order 2) [5]. Let  be fixed. By integrating the first equation of the system (1) we obtain
be fixed. By integrating the first equation of the system (1) we obtain
 (2)
(2)
Definition 2.1. A pair of functions  defined on
defined on 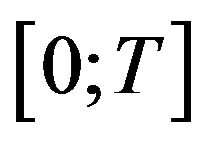 is said to be a solution to the system (1) whether
is said to be a solution to the system (1) whether
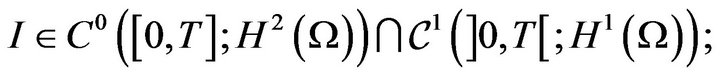
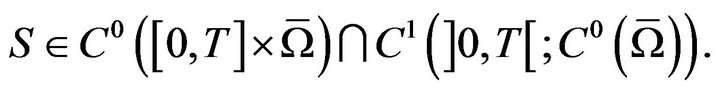
Lemma 2.2. Let  be a solution of the system (1), then the following holds:
be a solution of the system (1), then the following holds:

 (3)
(3)
Proof. Define the function  for
for . A very easy computation provides the following equation, for the function
. A very easy computation provides the following equation, for the function ,
,
 (4)
(4)
where  The weak maximum principle applies [5] and thus
The weak maximum principle applies [5] and thus
 (5)
(5)
3. Existence of a Solution
Existence of solution to (1) will be obtained using some classical arguments. Define the unbounded linear operator
 (6)
(6)
with homogeneous Neumann boundary conditions and where .
.
It is well known that  is strongly elliptic and invertible [6,7]. Define the function
is strongly elliptic and invertible [6,7]. Define the function
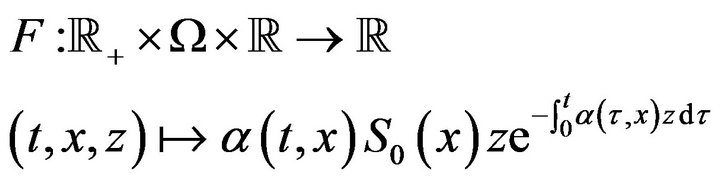
Lemma 3.1. Let
 be given. The operator
be given. The operator
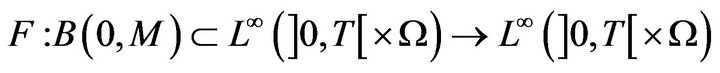
which associates  to
to  is Lipschitzian with a Lipschitz constant
is Lipschitzian with a Lipschitz constant .
.
Proof. Since the function 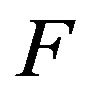 is continuously differentiable, its derivative is bounded on
is continuously differentiable, its derivative is bounded on . We then obtain the estimate by using the fundamental theorem of calculus.
. We then obtain the estimate by using the fundamental theorem of calculus.
Theorem 3.2. Assume that the assumptions on the functions ;
; ;
;  and
and  hold. Then for all
hold. Then for all , the problem (1) has a unique solution
, the problem (1) has a unique solution .
.
Proof. Problem (1) is rewritten in the following way:
 (7)
(7)
The operator  generates an analytical semigroup. According to Lemma 2.2 we consider Problem (7) on a bounded subset of
generates an analytical semigroup. According to Lemma 2.2 we consider Problem (7) on a bounded subset of . From Lemma 3.1 we know that
. From Lemma 3.1 we know that  is Lipschitzian. Therefore, one obtains the existence and uniqueness of a solution by using Theorem 3.1 and 3.3 in [7].
is Lipschitzian. Therefore, one obtains the existence and uniqueness of a solution by using Theorem 3.1 and 3.3 in [7].
4. Controllability of Problem (1) with the Functions α and β
Problem (1) is expressed in  as:
as:
 (8)
(8)
Theorem 4.1. Assume that the assumptions on the functions ;
; ;
;  and
and 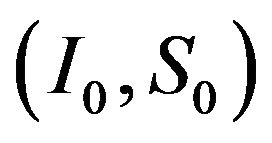 hold and that
hold and that  has
has 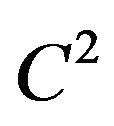 regularity. Let
regularity. Let  be given and let
be given and let  be an open subset and let
be an open subset and let  be fixed. For
be fixed. For  the solution to Problem (1) there is a real
the solution to Problem (1) there is a real  such that, if the functions
such that, if the functions ,
,  satisfy the following condition:
satisfy the following condition:
 (9)
(9)
then .
.
Proof. The solution to Problem (1) is a classical solution. Since the boundary of the domain  is regular, from the theory of analytical semigroup, we know that
is regular, from the theory of analytical semigroup, we know that
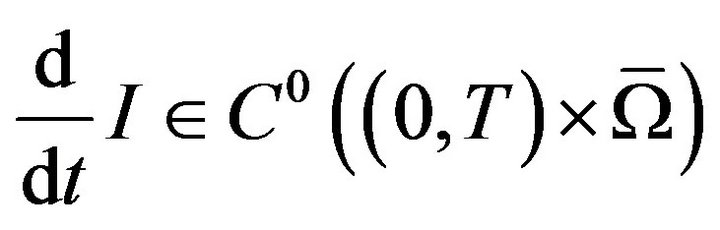
because the time derivative of 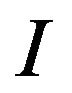 is bounded in the graph norm of a fractional power of the generator
is bounded in the graph norm of a fractional power of the generator  ([7] Chapter 2 Section 2.6 and Theorem 8.4.3). The strong maximum principle applies. Assume the maximum
([7] Chapter 2 Section 2.6 and Theorem 8.4.3). The strong maximum principle applies. Assume the maximum  of the function
of the function  is reached at the point
is reached at the point , from Equation (8) we deduce that
, from Equation (8) we deduce that
 (10)
(10)
Since  is uniformly continuous, there is
is uniformly continuous, there is  independent of
independent of  such that
such that
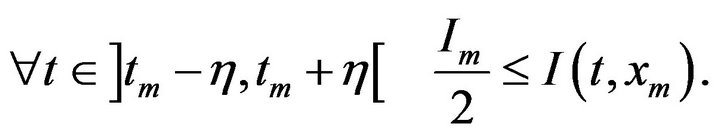
We have:
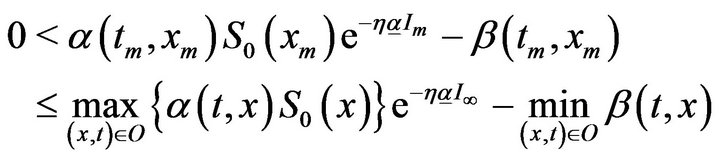
and we get a contradiction.
5. Numerical Applications and Discussions
In the following figures we give the isovalues of the infection in a two dimensional environment.
Figure 1 corresponds to the case in which the diffusion is isotropic.
Figure 2 corresponds to the unisotropic case. We can see in the two cases the spread of the infection.
Now we give some numerical results in order to show the effect of the medical care effort on the intensity of the epidemic in two different areas: site 1 and site 2, representing two cities with two different incidence rates.
In Figure 3 we suppose that no effort for medical care is made.
In Figure 4 we consider that the rates of medical care effort are  in the site 1 and
in the site 1 and  in the site 2. Then we note a decrease of the intensity of the infection in all the two sites.
in the site 2. Then we note a decrease of the intensity of the infection in all the two sites.
In Figure 5 we consider a medical care effort rate  in site 1 and
in site 1 and  in the site 2. Then we note a decrease of the intensity of the infection in all the two sites. These results mean that all medical care effort in one of the regions contributes to the decrease of the epidemic in the other one.
in the site 2. Then we note a decrease of the intensity of the infection in all the two sites. These results mean that all medical care effort in one of the regions contributes to the decrease of the epidemic in the other one.
In Figure 6 we use simultaneously the same medical care effort rate in the two sites: , then we can see that the intensity of the infection decreases more.
, then we can see that the intensity of the infection decreases more.
These results show that we can control the spread of the epidemic if we augment the medical care effort. A best result can be obtained, in the two sites, if efficient actions are done simultaneously in the two sites.
Therefore, by a policy of education we can operate on
 (a)
(a) (b)
(b)
Figure 1. Isotropic case: isovalues of the spread in the domain at (a) t = 100; (b) t = 500.
 (a)
(a) (b)
(b)
Figure 2. Unisotropic case: isovalues of the spread along the road at (a) t = 10; (b) t = 1000.
 (a)
(a) (b)
(b)
Figure 3. Unisotropic diffusion without medical care effort (β1 = β2 = 0) in the two areas at t = 300 and t = 350.
 (a)
(a) (b)
(b)
Figure 4. Unisotropic diffusion with t = 300, (a) β1 = 0.15, β2 = 0; (b) β1 = 0, β2 = 0.15.
 (a)
(a) (b)
(b)
Figure 5. Unisotropic diffusion, t = 300. (a) β1 = 0, β2 = 0.30; (b) β1 = 0.15, β2 = 0.15.
 (a)
(a) (b)
(b)
Figure 6. Unisotropic diffusion, t = 300. (a) β1 = 0.2, β2 = 0.2; (b) α1 = α2 = 0.013, β1 = 0.15, β2 = 0.15.
the incidence rate in the two sites and well control the spread of the infection (Figure 6(b) where  ).
).
In this way, it will be important that the leaders in the countries of the same area define together their policies in the fight against HIV spread.
In conclusion, we can say that, in addition to the medical treatment, if in the two sites, we reduce the incidence rate by more sensitization, then we can expect that the epidemic is controllable. That must be an operational aim for the deciders to fight against the spread of VIH/AIDS.