1. Introduction
Recently the dual-phase-lag (DPL) heat conduction model has stimulated considerable interest in the heat transfer community, by offering alternative interpretations and new perspectives to a large body of non-Fourier thermal behaviors in energy transportation process under special considerations, such as heat conduction in biological materials, heat transport in amorphous media, layered-film heating in superconductors, fins and reactor walls. and many commonly used devices, such as personal computers or cellular phones. Needless to say, numerous efforts have been invested to the development of an explicit mathematical solution to the heat conduction equation under the DPL model. Most of these analytical solutions to the DPL heat conduction problems in the literature were formulated ad hoc, only applicable to specific formulations of initial-boundary conditions. Other than the notoriously annoying fictitious numerical oscillations frequently encountered in solving hyperbolic partial differential equations (HPDE), the intrinsic complexity of the DPL heat conduction equation alone (highorder mixed derivative with respect to space and time which dramatically alter the fundamental characteristics of the solution) poses a tremendous hindering obstacle against a general solution [5]. In the present work highorder mixed derivative with respect to space and time is treated in special manner in the dual-phase-lag heat equation in order to construct a general solution applicable to wide range of dual-phase-lag heat transfer problems of general initial-boundary conditions using Green’s function solution method.
The definition of Green’s functions for a wave-type conduction equation and a general form of the Green’s function solution method for finite bodies is introduced by Haji-Sheikh and Beck [6]. Loureiro et al. [7] studied the hyperbolic bioheat conduction equation using the explicit Green’s approach method. The dual-phase-lag heat equation was used to generalize macroscopic model in treating the transient heat conduction in finite slabs irradiated by short pulse laser using Green’s function method by [8,9]. For powerful reviewing of construction of several Green’s functions for different boundary and initial condition of various physical equations, the reader is referred to [10].
The present work is devoted to define a generalized Green’s function solution for the dual-phase-lag model in homogeneous materials. Also, the Green’s function for a finite medium subjected to arbitrary heat source and arbitrary initial and boundary conditions is constructed. To examine the applicability of the present method, calculations are performed on four different previously solved researches [1-4]. The obtained results show good agreement with these researches.
2. The Dual-Phase-Lag Heat Equation
Let 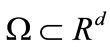 be an open bounded domain with smooth boundary
be an open bounded domain with smooth boundary 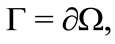 where d is the number of space dimensions and let
where d is the number of space dimensions and let  be the time domain with
be the time domain with  the dual-phase lag model (DPL), given by Tzou [11], which allows either the temperature gradient (cause) to precede the heat flux vector (effect) or the heat flux vector (cause) to precede the temperature gradient (effect) in the transient process, can be represented, mathematically, by
the dual-phase lag model (DPL), given by Tzou [11], which allows either the temperature gradient (cause) to precede the heat flux vector (effect) or the heat flux vector (cause) to precede the temperature gradient (effect) in the transient process, can be represented, mathematically, by
 (1)
(1)
where 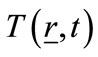 and
and  are the temperature and heat flux distributions at position
are the temperature and heat flux distributions at position 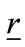 at time
at time 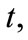 respectively.
respectively. 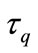 is the phase lag (relaxation time) of the heat flux vector,
is the phase lag (relaxation time) of the heat flux vector, 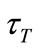 is the phase lag (relaxation time) of the temperature gradient,
is the phase lag (relaxation time) of the temperature gradient, 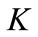 is the thermal conductivity. Combining Equation (1) with the energy conservation law,
is the thermal conductivity. Combining Equation (1) with the energy conservation law,
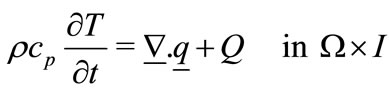 (2)
(2)
leads to the energy transport equation (the dual-phase-lag heat equation) in the form
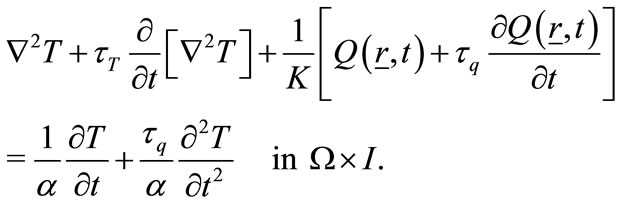 (3)
(3)
where  is specific heat at constant pressure,
is specific heat at constant pressure,  is the density,
is the density,  is the heat generation per unit volume and
is the heat generation per unit volume and 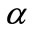 is the thermal diffusivity. The highorder mixed derivative with respect to space and time is dramatically alter the fundamental characteristics of the solution and completely destroys the wave structure resulting from the wavy term, the second-order derivative term with respect to time, and the energy equation is parabolic in nature. It predicts a higher temperature level in the heat-penetration zone than diffusion but does not have a sharp wavefront in heat propagation.
is the thermal diffusivity. The highorder mixed derivative with respect to space and time is dramatically alter the fundamental characteristics of the solution and completely destroys the wave structure resulting from the wavy term, the second-order derivative term with respect to time, and the energy equation is parabolic in nature. It predicts a higher temperature level in the heat-penetration zone than diffusion but does not have a sharp wavefront in heat propagation.
The smooth boundary 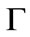 can be imposed on either prescribed temperature or prescribed heat flux. In addition to the prescribed boundary values, the initial condition on temperature may be also specified as below
can be imposed on either prescribed temperature or prescribed heat flux. In addition to the prescribed boundary values, the initial condition on temperature may be also specified as below
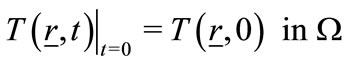 (4)
(4)
while according to the conservation law (2), with the consideration that the initial value of the heat flux  the initial value of the time derivative of the temperature distribution may takes the form
the initial value of the time derivative of the temperature distribution may takes the form
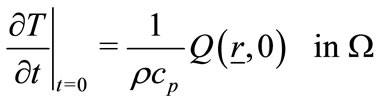 (5)
(5)
3. Solution with Green’s Function
The Green’s functions are an important tool in solving partial differential equations since the solution of the problem subjected to any kind of initial conditions, boundary conditions and internal heat generation can be obtained through integral equations once the Green’s function is known. The Green’s function 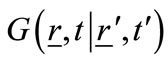 for finite or semi-infinite medium of constant physical properties with arbitrary initial and boundary conditions which correspond to the dual-phase-lag heat conduction Equation (3) is defined as the solution of
for finite or semi-infinite medium of constant physical properties with arbitrary initial and boundary conditions which correspond to the dual-phase-lag heat conduction Equation (3) is defined as the solution of
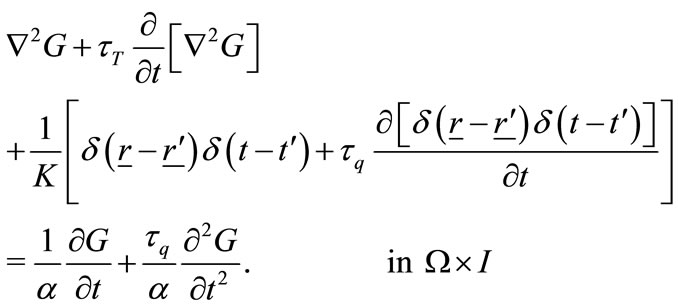 (6)
(6)
For convenience of subsequent analysis, the following dimensionless variables are defined
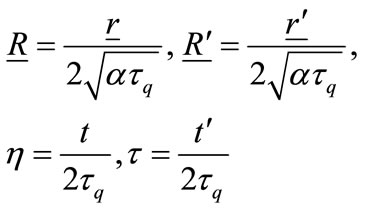 (7a)
(7a)
 (7b)
(7b)
 (7c)
(7c)
Using the above dimensionless variables, Equations (3) and (6) are expressed as
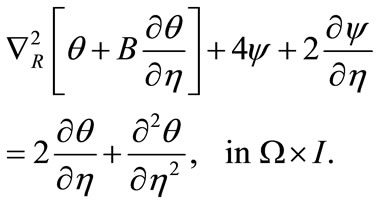 (8)
(8)
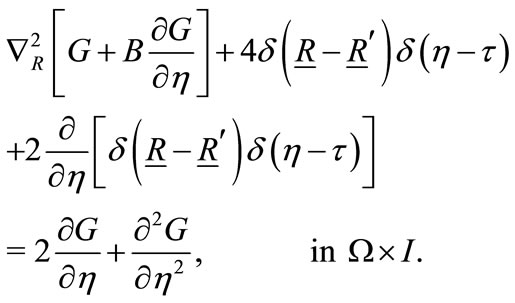 (9)
(9)
where  is Dirac delta function. For convenience of algebra, Equation (9) can be reduced to a simpler form. To accomplish this task, one can define a Green’s function
is Dirac delta function. For convenience of algebra, Equation (9) can be reduced to a simpler form. To accomplish this task, one can define a Green’s function 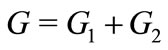 so that
so that
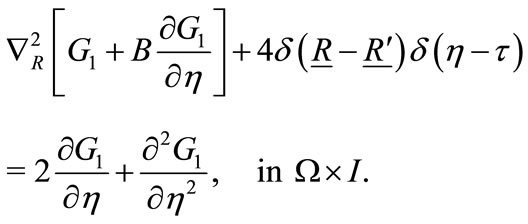 (10a)
(10a)
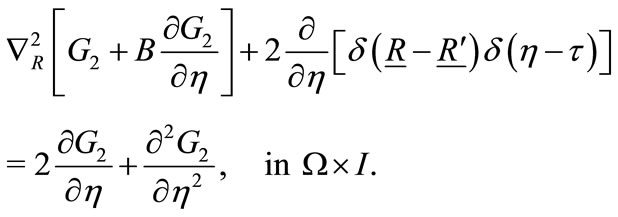 (10b)
(10b)
Examining the above two equations, one can hypothesize that 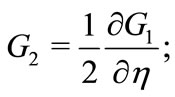 this acceptable since both
this acceptable since both 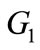 and
and 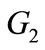 have homogeneous boundary conditions and their initial conditions, including all time derivatives, are zero. To show this relation between
have homogeneous boundary conditions and their initial conditions, including all time derivatives, are zero. To show this relation between 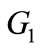 and
and , simply substitute for
, simply substitute for  in Equation (10b) and get
in Equation (10b) and get
 (11)
(11)
that reduces to the equation,
 (12)
(12)
Notice that any function  that satisfies Equation (10a) also satisfy Equation (10b). Therefore, instead of solving for
that satisfies Equation (10a) also satisfy Equation (10b). Therefore, instead of solving for  from Equation (9), it is sufficient to solve a simpler Equation (10a), and then utilize the relation
from Equation (9), it is sufficient to solve a simpler Equation (10a), and then utilize the relation
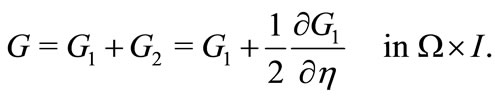 (13)
(13)
Changing the spatial variables in Equation (10a) to “prime” space and time from  to
to  yields
yields
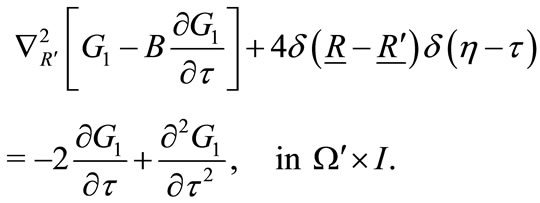 (14)
(14)
Moreover, the dual-phase-lag heat equation in 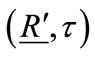 space is
space is
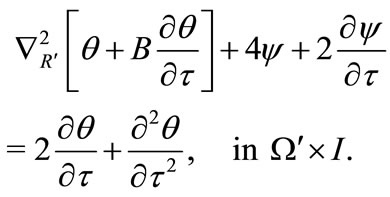 (15)
(15)
Multiplying Equation (15) by  and Equation (14) by
and Equation (14) by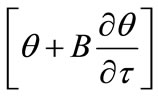 , then subtracting the results to produce equation
, then subtracting the results to produce equation
 (16)
(16)
Both sides of Equation (16) are integrated, 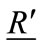 over volume
over volume 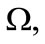 and
and 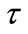 from 0 to
from 0 to , where
, where 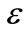 is a small positive number. Then, following the application of the Green’s theorem and after letting
is a small positive number. Then, following the application of the Green’s theorem and after letting  to go to zero, one gets
to go to zero, one gets
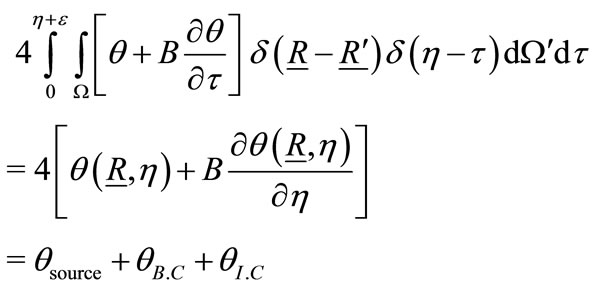 (17)
(17)
where the source contribution to the temperature distribution is given by
 (18)
(18)
while the boundary conditions contribution to temperature distribution is
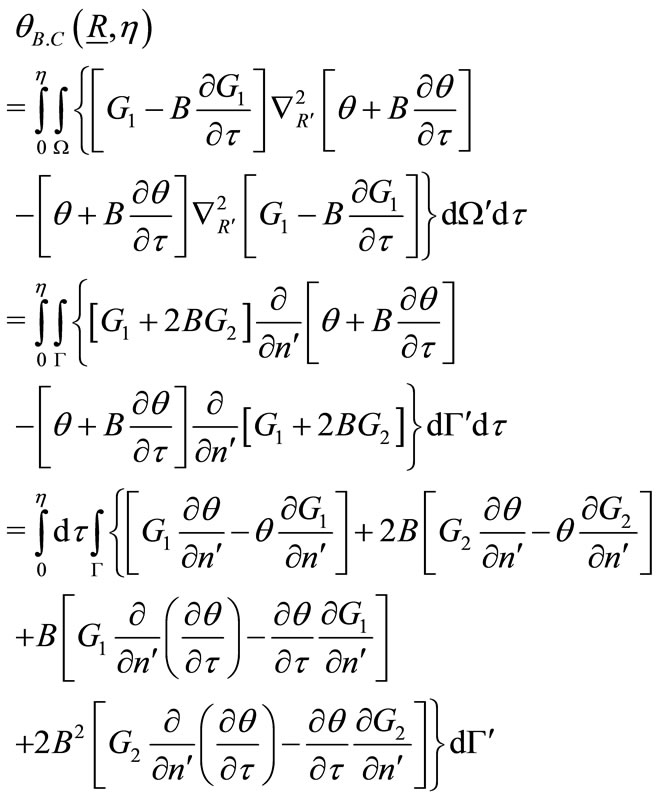 (19)
(19)
where  is the boundary of the volume
is the boundary of the volume  and
and  is the unit vector outward normal to the boundary
is the unit vector outward normal to the boundary . Notice that due to the causality principle one has
. Notice that due to the causality principle one has 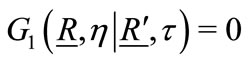 and
and 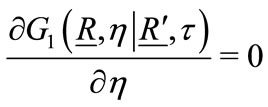 for
for  and consequently, the initial conditions contribution to the temperature distribution is
and consequently, the initial conditions contribution to the temperature distribution is
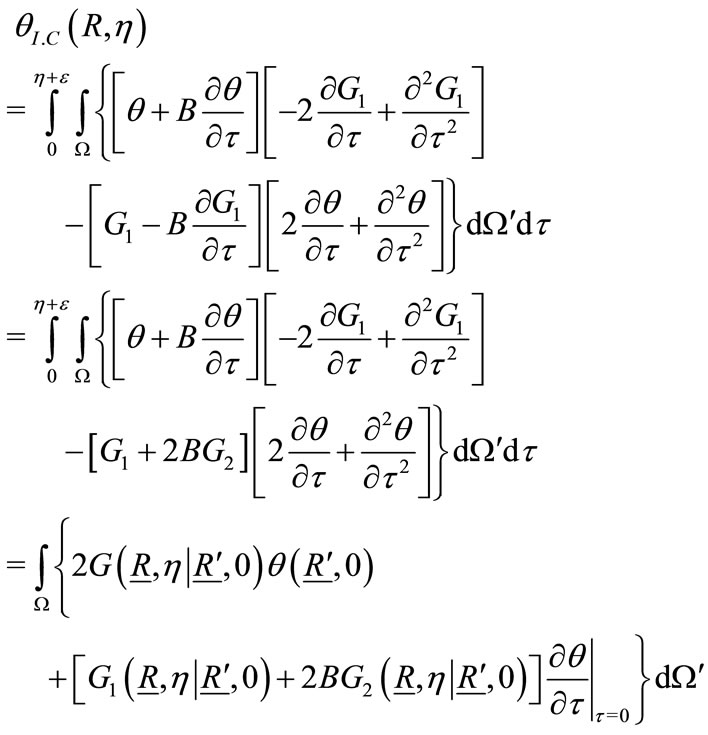 (20)
(20)
Thus, the temperature distribution can be expressed as
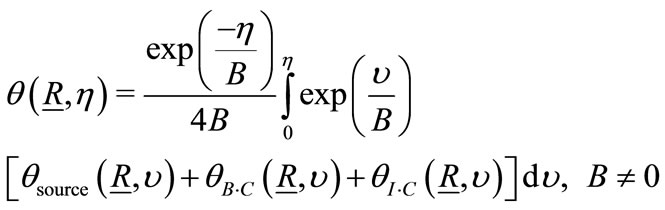 (21)
(21)
For the hyperbolic model, i.e.  the temperature distribution can be expressed as
the temperature distribution can be expressed as
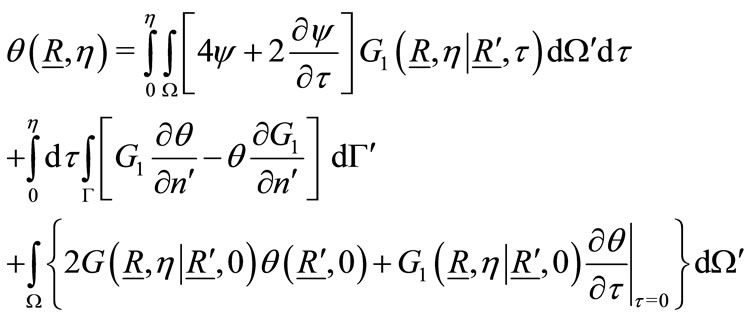 (22)
(22)
which cionside with that given by [6], with the consideration that the present formulae is dimensionless one.
4. Construction of Green’s Function for Finite Medium
Green’s function for finite medium can be derived by solving Equation (10a) for 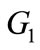 with homogeneous initial and boundary conditions. Applying a suitable finite transform to Equation (10a) using homogeneous boundary conditions either of Nueman or Dirrichlet kind or even radiation boundary conditions, yields to
with homogeneous initial and boundary conditions. Applying a suitable finite transform to Equation (10a) using homogeneous boundary conditions either of Nueman or Dirrichlet kind or even radiation boundary conditions, yields to
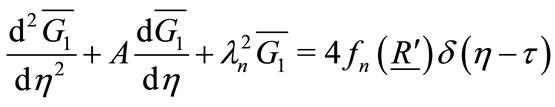 (23)
(23)
where 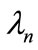 are the eigen values corresponding to the eigen functions
are the eigen values corresponding to the eigen functions 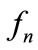 and
and
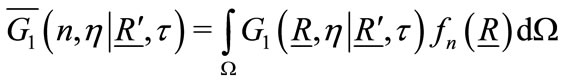 (24a)
(24a)
 (24b)
(24b)
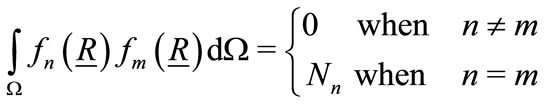 (24c)
(24c)
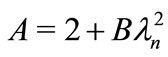 (24d)
(24d)
where 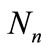 is the orthogonality constant.
is the orthogonality constant.
Now, solving Equation (23) with homogeneous initial conditions and then using the inversion Formula (24b), the first component of the Green’s function can be expressed as
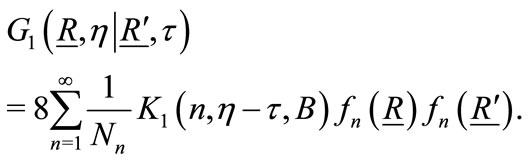 (25a)
(25a)
where
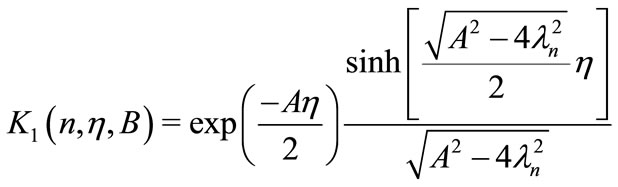 (25b)
(25b)
Then using equation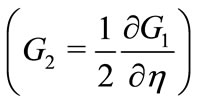 , the second component
, the second component 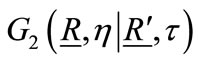 of the Green’s function can be expressed as
of the Green’s function can be expressed as
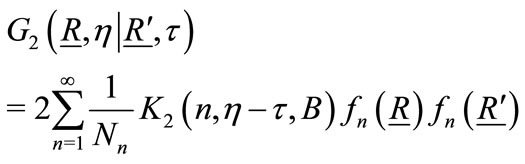 (26a)
(26a)
where
 (26b)
(26b)
Thus the Green’s function  can be written in the form
can be written in the form
 (27a)
(27a)
where

(27b)
Note that
 (27c)
(27c)
Note that the above postulated Green’s function can be modified to a semi-infinite medium by extending  to a semi-open domain and consequently the integral transform (24a) and its inversion (24b) should be modified.
to a semi-open domain and consequently the integral transform (24a) and its inversion (24b) should be modified.
5. Discussion
Since the lagging behavior is a special response to time, the consideration of one-dimensional problems in space is sufficient to illustrate its fundamental characteristics. In addition, from a mathematical point of view, the lagging behavior introduces the highest order differentials in the energy equation, reflected by the mixed-derivative and the wave term. These terms characterize the fundamental solutions of the energy equation employing the dual-phase-lag model. Consideration of multidimensional problem will not alter the qualitative behavior depicted by the one-dimensional problem.
With the objective of showing the applicability and generality of the given Green’s function method to deal with any heat generation and any kind of initial and boundary conditions, three one-dimensional examples and one two dimensional example are discussed.
5.1. Example 1
In this example the overshooting phenomenon was investigated by M. Xu et al. [1]. The overshooting phenomenon is studied based on the one-dimensional dualphase-lagg heat conduction model. The thermal wave interference is found to trigger the overshooting of temperature field. A condition for the occurrence of overshooting phenomenon is established for the one-dimensional dual-phase-lagging heat conduction in a finite medium. According to this condition, the overshooting phenomenon may occur in heat conduction across gold films with the thickness ranging from 4.8555 nm to 19.581 mm.
The purpose of this example is to show the method of determining the temperature distribution 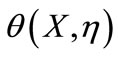 in a slab using the one dimensional dual-phase-lag Green’s function when its faces are imposed to constant boundary conditions by solving the system
in a slab using the one dimensional dual-phase-lag Green’s function when its faces are imposed to constant boundary conditions by solving the system
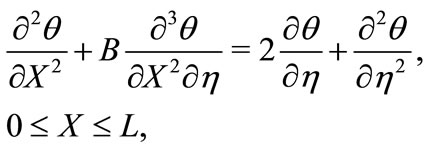 (28a)
(28a)
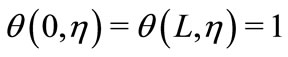 (28b)
(28b)
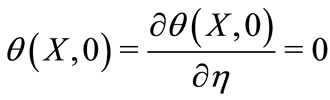 (28c)
(28c)
Accordingly, the temperature distribution in terms of Green’s function is given in the form
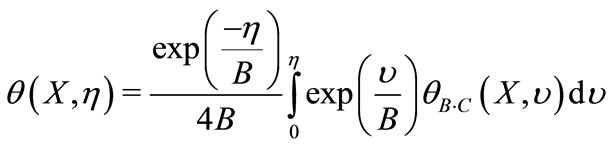 (29a)
(29a)
where
 (29b)
(29b)
Using Green’s functions from Equations (25a) and (26a) with recognizing that the eigen functions of this example are 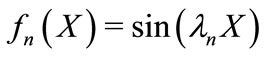 with eigen values
with eigen values 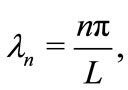 and the normalization constant
and the normalization constant 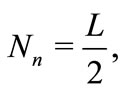 the temperature distribution (29a) can be written as
the temperature distribution (29a) can be written as
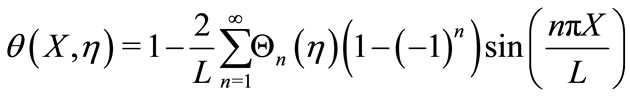 (30a)
(30a)
where
 (30b)
(30b)
Equation (30b) is plotted using Mathematica program ver. 5. Figure 1 shows the temperature distribution for a thin gold film where the averaging values of the two lag times over nominal range of temperature are 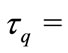

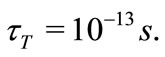 thus
thus  with dimensionless thickness
with dimensionless thickness 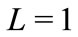 at dimensionless times
at dimensionless times .
.
The obtained results show good agreement with those depicted by [1] who solved this problem using separation of variables method.
5.2. Example 2
The objective of this example is to test the proposed Green’s method using heat source and prescribed initial conditions with insulated boundaries. In this example the one dimensional dual-phase-lag heat equation in a thin film subjected to symmetrical time dependent laser heating is investigated by Alkhairy [2] by solving the system
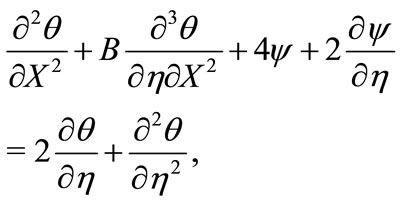 (31a)
(31a)
 (31b)
(31b)
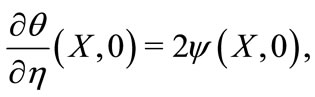 (31c)
(31c)
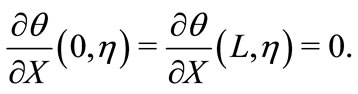 (31d)
(31d)
where
 (32a)
(32a)
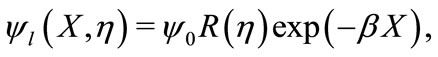 (32b)
(32b)
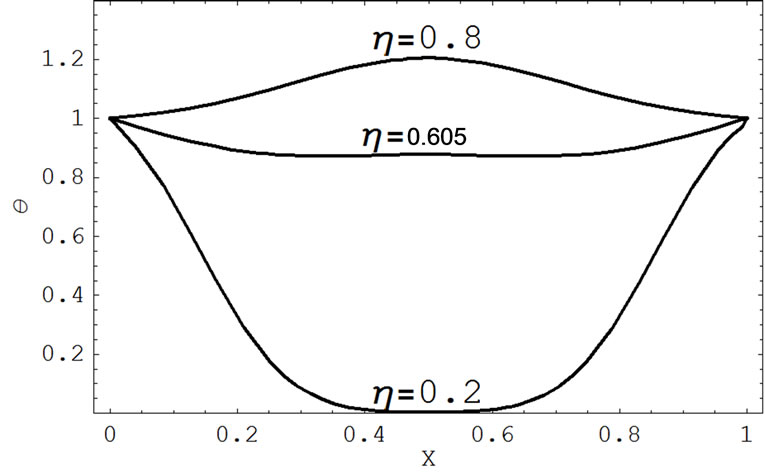
Figure 1. Variation of dimensionless temperature θ for a thin gold film of B = 4:7222E−2; with dimensionless thickness L = 1 at dimensionless times η = 0.2, 0.605, 0.8.
 (32c)
(32c)
where 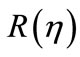 is the characteristic of the laser beam intensity,
is the characteristic of the laser beam intensity, 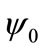 is the dimensionless capacity of internal heat source,
is the dimensionless capacity of internal heat source,  is the dimensionless absorption coefficient and the subscripts
is the dimensionless absorption coefficient and the subscripts  refer to the left and right edges of the film, respectively. In our example, a light heat pulse is adopted, i.e.,
refer to the left and right edges of the film, respectively. In our example, a light heat pulse is adopted, i.e.,
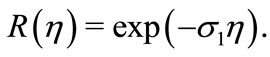 (33)
(33)
where  and
and  is the laser heating duration.
is the laser heating duration.
Accordingly, the temperature distribution in terms of Green’s function is given in the form
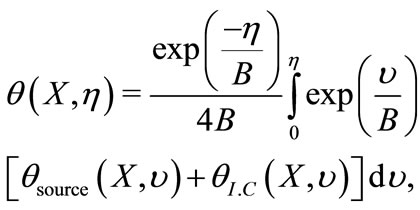 (34a)
(34a)
where
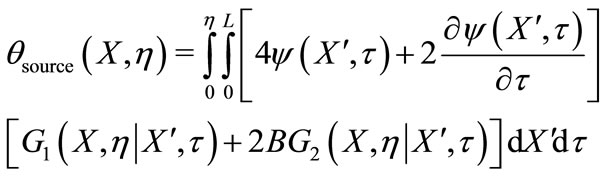 (34b)
(34b)
 (34c)
(34c)
Using Green’s functions from Equations (25a) and (26a) with recognizing that the eigen functions of this example are 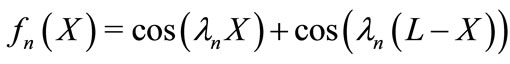 with eigen values
with eigen values 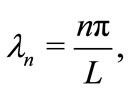 and the normalization constant
and the normalization constant  the temperature distribution (34a) can be written as
the temperature distribution (34a) can be written as
 (35a)
(35a)
where
 (35b)
(35b)
where
 (35c)
(35c)
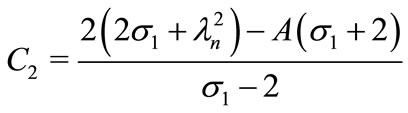 (35d)
(35d)
Figure 2 depict the results of calculations of Equation (35a) in a film of thickness  for light heat source of dimensionless laser heating duration
for light heat source of dimensionless laser heating duration  and dimensionless absorption coefficient
and dimensionless absorption coefficient 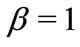 at dimensionless time
at dimensionless time , for various dimensionless controlling coefficients
, for various dimensionless controlling coefficients 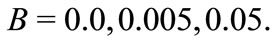
With increasing 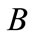 from zero, it is clear that the sharp wave fronts are smoothed and the portions of the disturbance are dissipated. The behavior of temperature response for
from zero, it is clear that the sharp wave fronts are smoothed and the portions of the disturbance are dissipated. The behavior of temperature response for  is called wavelike behavior. Figure 2 manifests that the wavelike behavior has smaller amplitude of temperature rise than the wavy one
is called wavelike behavior. Figure 2 manifests that the wavelike behavior has smaller amplitude of temperature rise than the wavy one 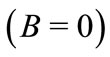 and the increase of
and the increase of  results attenuation of the amplitude but not any change in the wide of the portion of the thermal disturbance.
results attenuation of the amplitude but not any change in the wide of the portion of the thermal disturbance.
The obtained results show good agreement with those depicted by [2] who solved this problem using the integral transforms and the variation of parameters method.
5.3. Example 3
Example 2 was also investigated by [3], but for hyper-

Figure 2. Variation of dimensionless temperature θ for a film of L = 1; η = 0.4; β = 1 for light heat source R(η) = exp of dimensionless laser heating duration σ = 0.01.
of dimensionless laser heating duration σ = 0.01.
bolic heat model i.e., . For purpose of comparison, the present Green’s method is applied to the corresponding hyperbolic system of Example 2 for instantaneous heat source whose time characteristic of the laser beam intensity
. For purpose of comparison, the present Green’s method is applied to the corresponding hyperbolic system of Example 2 for instantaneous heat source whose time characteristic of the laser beam intensity  is given as
is given as
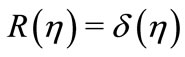 (36)
(36)
According, the temperature distribution in terms of Green’s function is given in the form
 (37)
(37)
Using integration by parts and the causality principle Equation (37) can be written as
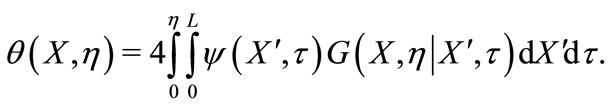 (38)
(38)
Using Green’s functions from Equation (37) with recognizing that the eigen functions of this example are 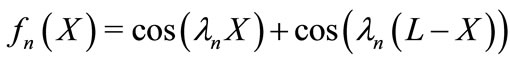 with eigen values
with eigen values  and the normalization constant
and the normalization constant 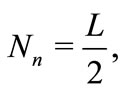 the temperature distribution (38) can be written as
the temperature distribution (38) can be written as
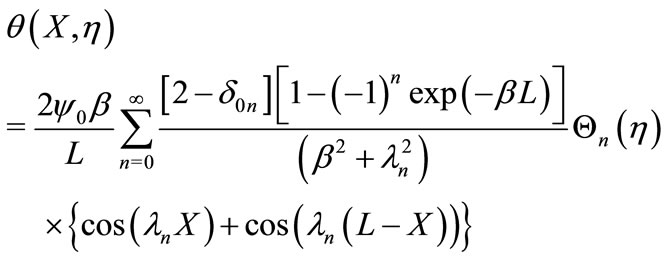 (39)
(39)
where
 (40)
(40)
Figure 3 depict the results of calculations of Equation
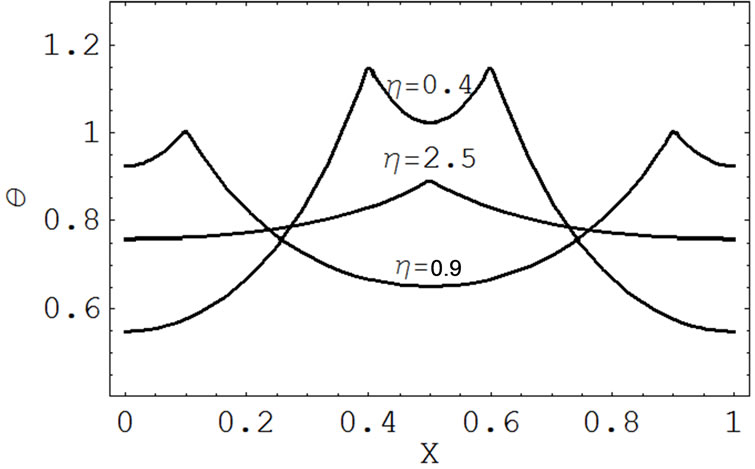
Figure 3. Variation of dimensionless temperature θ for a film of L = 1; β = 5 for instantanous heat source R(η) = δ(η).
(39) for a film of thickness  with instantaneous heat source of dimensionless absorption coefficient
with instantaneous heat source of dimensionless absorption coefficient  at various dimensionless times
at various dimensionless times 
The obtained results show good agreement with those depicted by [3] who solved this problem using Laplace transforms method.
5.4. Example 4
In this example the two-dimensional dual-phase-lag (DPL) model of heat conduction was investigated numerically by [4] for treating the transient heat conduction problems in finite rigid medium under short-pulse-laser heating with Gaussian distributions in both temporal and spatial profiles by solving the system
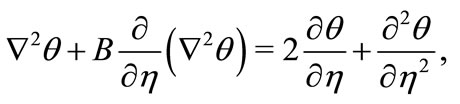 (41)
(41)
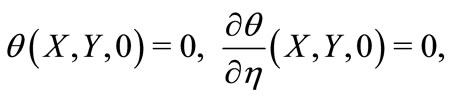 (42a)
(42a)
 (42b)
(42b)
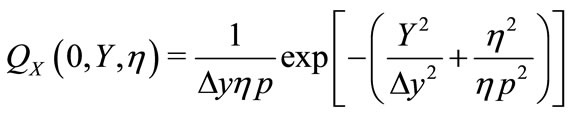 (42c)
(42c)
where 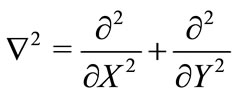 and
and  is the heat flux at the boundary
is the heat flux at the boundary 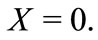 Accordingly, the temperature distribution in terms of Green’s function is given in the form
Accordingly, the temperature distribution in terms of Green’s function is given in the form
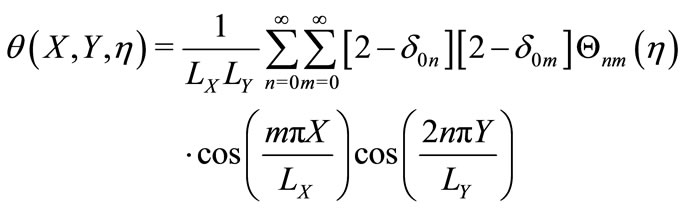 (43)
(43)

Figure 4. Variation of dimensionless temperature θ for a finite meduim of dimensions LX = 1; LY = 2 irradiated by laser pulse with characteristic time ηp = 0.1 and characteristic length Δy = 0.1 with controlling coecient B = 0.0.
where
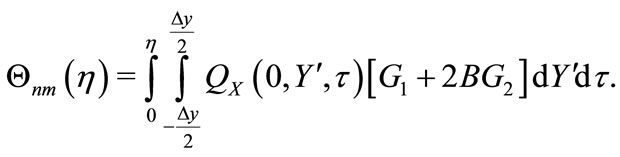 (44)
(44)
Figure 4 depict the results of calculations of Equation (43) for a rectangular medium of dimensions 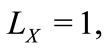
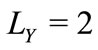 irradiated by laser pulse with characteristic time
irradiated by laser pulse with characteristic time  and characteristic length
and characteristic length 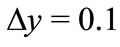 with controlling coefficient
with controlling coefficient 
The obtained result show good agreement with that depicted by [4] who solved this problem numerically using finite-difference method method.
6. Conclusion
Hence, the purpose of the present paper is to describe the analytical solution of the dual-phase-lag heat equation in a unified manner by Green’s function method. The highorder mixed derivative with respect to space and time which reflect the lagging behavior is treated in special manner in the dual-phase-lag heat equation in order to construct a general solution applicable to wide range of dual-phase-lag heat transfer problems of general initialboundary conditions using Green’s function solution method. Also, the Green’s function for a finite medium subjected to arbitrary heat source and arbitrary initial and boundary conditions is constructed. Since the lagging behavior is a special response to time, the consideration of one-dimensional problems in space is sufficient to illustrate its fundamental characteristics. Therefore, three one dimensional examples and one two dimensional example of different physical situations are analyzed in order to illustrate the accuracy and potentialities of the proposed unified method. The obtained results show good agreement with works of [1-4].
7. Acknowledgements
The author would like to thank the scientific deanship of University of Dammam for its generous support of this work through the project No. 2011082.