1. Introduction
Throughout this paper, G denotes a graph with order p and size q. By a graph we mean a finite undirected graph without loops or multiple edges. For graph theoretic terms we refer Harary [1] and in particular, for terminology related to domination theory we refer Haynes et al. [2].
1.1. Definition
Let G = (V, E) be a graph. A subset D of E is said to be an edge dominating set if every edge in E-D is adjacent to at least one edge in D. An edge dominating set D is said to be a minimal edge dominating set if no proper subset of D is an edge dominating set of G. The edge domination number γ'(G) of a graph G equals the minimum cardinality of an edge dominating set of G. An edge dominating set of G with cardinality γ'(G) is called a γ'(G)-set or γ'-set.
Acharya [3] introduced the concept of domsaturation number ds(G) of a graph. For any graph G of order p, and for any integer r such that γ(G) ≤ r ≤ p, we call the set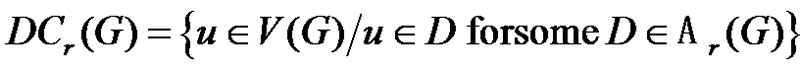 
the r-level domination core of G. We say that G is r-level domination-saturated (or in short, “r-domsaturated”) if DCr(G) – V(G). The domsaturation number ds(G) is then defined by
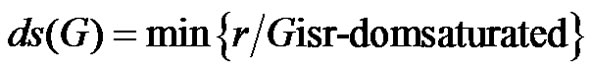. Arumugam and Kala [4] observed that for any graph G, 
or 
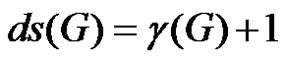and obtained several results on ds(G). We now extend the concept of domsaturation number of a graph to edges.
1.2. Definition
The least positive integer k such that every edge of G lies in an edge dominating set of cardinality k is called the edge-domsaturation number of G and is denoted by ds'(G).
If G is a graph with edge set E and D is a γ'-set of G, then for any edge e Î E-D, 
is also an edge dominating set and hence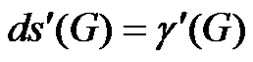 
or
.
Thus we have the following definition.
1.3. Definition
A graph G is said to be of class 1 or class 2 according as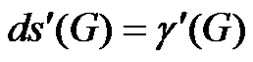 
or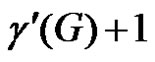
.
1.4. Definition
An edge e of G is
1) γ'-critical if
;
2) γ'+-critical if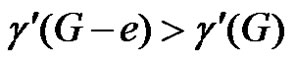
;
3) γ'–-critical if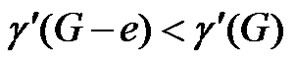
;
4) γ'-fixed if every γ'-set contains e;
5) γ'-free if there exists γ'-sets containing e and also γ'-sets not containing e;
6) γ'-totally free if there is no γ'-set containing e.
We use the following theorem.
1.5. Theorem [5]
For any connected unicyclic graph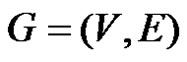 
with cycle C, 
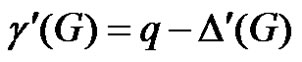if and only if one of the following holds.
1)
;
2)
, 
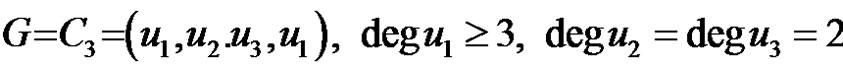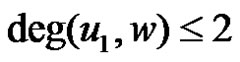for all vertices w not on C and 
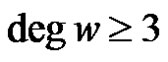for at most one vertex w not on C;
3)
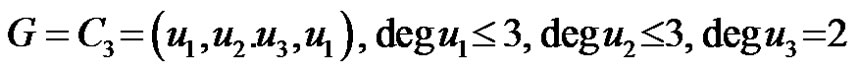all the vertices not on C adjacent to u1 have degree at most 2 and all vertices whose distance from u1 is 2 are pendent vertices;
4) 
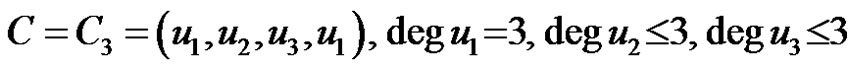and all vertices not on C are pendent vertices;
5)
;
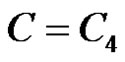6)
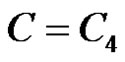, either exactly one vertex of C has degree at least 3 and all vertices not on C are pendent vertices.
2. Main Results
2.1. Lemma
An edge e of G is γ'–-critical if and only if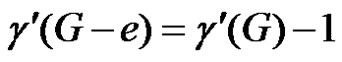 
Proof
For any edge e, we observe that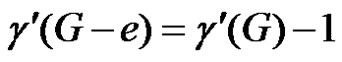 
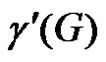or 
or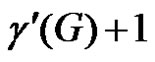
. Now, suppose e is γ'–-critical. Then
. Hence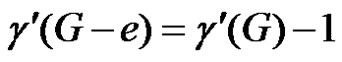
. The converse is obvious.
2.2. Theorem
An edge e is γ'–-critical if and only if
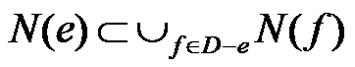(1)
for some γ'-set D containing e.
Proof
If e is γ'–-critical, 
by lemma 2.1. Let S be a γ'-set of G – e. If S contains an edge of N(e), then S will be an edge dominating set of G and hence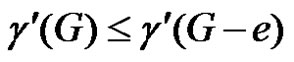
, a contradiction. Thus S does not contain any edge of N(e). Since
, 
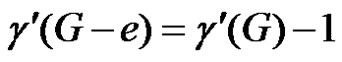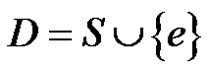is a γ'-set of G and so Equation (1) holds. Conversely, suppose e is an edge such that (1) is true. Then G – e is an edge dominating set of G – e and hence
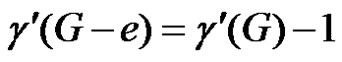. Thus e is γ'–-critical.
2.3. Theorem
Let G be a graph without isolated edges. An edge e in G is γ'–-critical if and only if 1) e is γ'-free, and 2) no γ'-set of G – e contains any edge of N(e).
Proof
If e is γ'–-critical, then 
by Lemma 2.1. As in theorem 2.2, if S is any γ'-set of G – e, then S will not contain any edge of N(e) and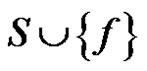 
is a γ'- set of G for every
. This implies that e is γ'-free. Conversely, suppose (1) and (2) are true. Let S be a γ'- set of G – e. By (2) S does not contain any edge of N[e]. Hence S cannot be an edge dominating set of G. But, for any edge
, 
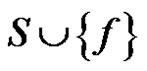is an edge dominating set of G. Since S is a minimum edge dominating set for G – e, 
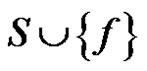is also a minimum edge dominating set for G and hence
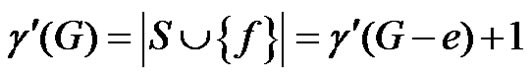. Thus e is γ'–-critical.
2.4. Theorem
Let G be a graph and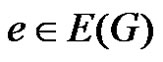
. Then
1) e is γ'-fixed if and only if there exists no edge dominating set of G – e with γ'(G) edges which is also an edge dominating set of G.
2) e is γ'-totally free if and only if every γ'-set of G is a γ'-set of G – e.
Proof
1) Assume that e is γ'-fixed. Suppose there exists an edge dominating set S of G – e with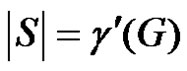 
which is also an edge dominating set of G. Then S is a γ'-set not containing e which is impossible as e is γ'-fixed. The converse is obvious.
2) Let e be γ'-totally free. Then e does not belong to any γ'-set of G and so every γ'-set D of G is an edge dominating set of G – e. Thus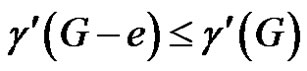
. If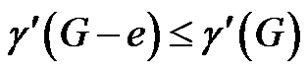
, then by theorem 2.3, e is γ'-free and so
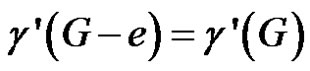, D is a γ'-set of G – e. The converse is obvious.
2.5. Theorem
Let G be a connected graph. If a cut edge e of G is γ'- fixed, then e is γ'+-critical
Proof
Let S be a γ'-set of G. Let e be a cut edge that is γ'- fixed. Then e belongs to every γ'-set. Since e is a cut edge, G – e is a disconnected graph with at least two components G' and
. Let e' and e" be the neighbors of e in G' and G" respectively. Therefore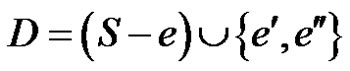 
is a minimum edge dominating set of G – e so that
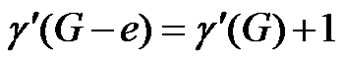. Hence e is γ'+-critical.
2.6. Theorem
An edge e in a graph G is γ'+-critical if and only if 1) e is not isolated edge 2) e is γ'-fixed and 3) There is no edge dominating set for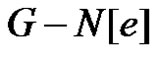 
having γ'(G) edges which also dominates N[e].
Proof
If e is γ'+-critical, then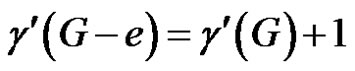
, by lemma 2.1. Clearly e is not an isolated edge. If S is a γ'-set of 
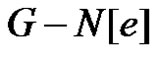having γ'(G) edges which also dominates N(e) then
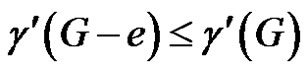, a contradiction. Thus no edge dominating set of 
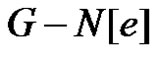having γ'(G) edges can dominate N(e). By Theorem 2.4, e is γ'-fixed. The converse is obvious.
We now investigate relationships between, γ'-free edges, γ'-totally free edges and graphs which are class 1 and class 2.
2.7. Theorem
If G is a graph without isolated edges, then G is of class 2 if and only if G has γ'-totally free edges.
Proof
Suppose G has a γ'-totally free edge e. By Theorem 2.4 (2), G is of class 2. Conversely, suppose G is of class 2. Then there exists an edge e which is not in any γ'-set. Hence every γ'-set of G is also a γ'-set of G – e so that e is γ'-totally free.
2.8. Theorem
Proof
Let G be a connected graph. If G has a γ'-fixed edge, then it has a γ'-totally free edge.
Suppose G has a γ'-fixed edge e. Then e belongs to every γ'-set.
Claim: No neighbor of e belongs to any γ'-set of G. Suppose at least one of its neighbor say e' belongs to a γ'- set D. Let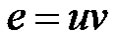 
and e' be incident with u. Then
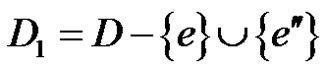, where e" is any edge incident with v is an edge dominating set of G – e with γ'-edges which is also an edge dominating set of G. But by Theorem 2.6, this is a contradiction, since e is a γ'-fixed edge. Therefore no neighbor of e belongs to any γ'-set of G. Thus neighbors of e are all γ'-totally free in G.
We now investigate the class of graphs which are ds'+, ds'–-critical.
2.9. Lemma
Let e Î E(G). If e is γ'-totally free and G – e is of class 1, then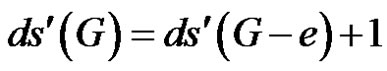
.
Proof
Since e is γ'-totally free, by Theorem 2.4,
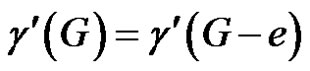(1)
Since e is γ'-totally free, by Theorem 2.3, G is of class 2 and so
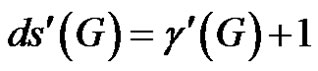(2)
Since G – e is of class 1, we have
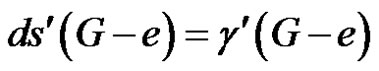(3)
From Equations (1), (2) and (3), we have
.
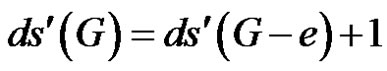2.10. Lemma
Let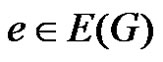
. If e is γ'-totally free and G – e is of class 2, then
.
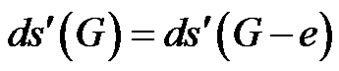Proof
If e is γ'-totally free, then by Theorem 2.4,
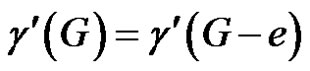(1)
Since G and G – e are of class 2, we have
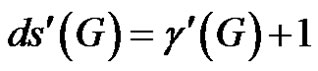(2)
and
(3)
From equations (1), (2) and (3), we have
.
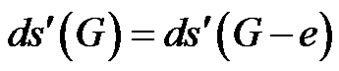2.11. Lemma
Let e be an edge of G. If e is γ'-free and G – e is of class 1, then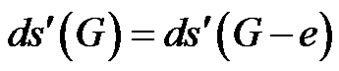 
or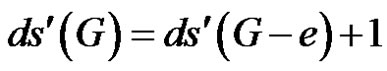
.
Proof
Suppose e is a γ'-free edge. In any case G is either of class 1 or class 2.
Case (1). G is of class 1.
Let S be a γ'-set of G – e. If S does not contain any neighbor of e, then every neighbor of e is γ'-totally free in G – e. This implies that G – e is of class 2. But this is a contradiction and so S must contain a neighbor of e. Then by theorem 2.4,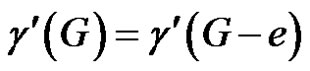
. Since G and G – e are of class 1, we have
.
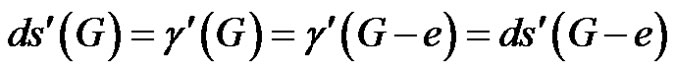Case (2). G is of class 2.
Since G – e is of class 1, then by a similar argument, S must contain a neighbor of e. Since G is of class 2, we have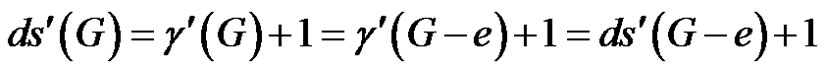
.
2.12. Lemma
Let e be an edge of G. If e is γ'-free and G – e is of class 2, then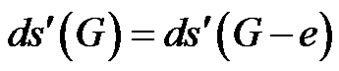
, 
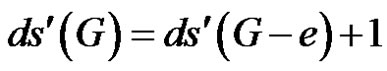or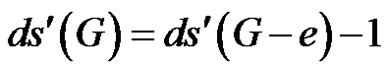
.
Proof
Case (1). G is of class 1.
Let S be a γ'-set of G – e. We have the following cases:
Subcase (1). S contains a neighbor of e.
Now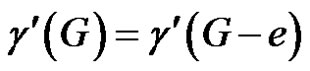
. Since G is of class 1 and G – e is of class 2, we have
.
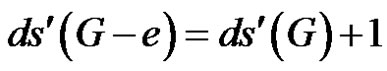Subcase (2). S does not contain a neighbor of e.
Now
. Since G – e is of class 2 and G is of class 1, we have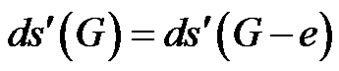
.
Case (2). G is of class 2.
By an argument similar to that in case (1), we have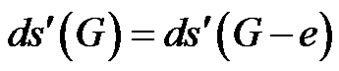 
or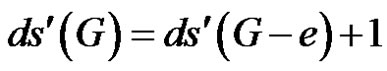
.
2.13. Lemma
Let e be an edge of G. If e is γ'-fixed and G – e is of class 1, then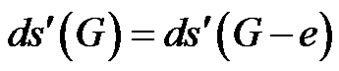
.
Proof
If e is γ'-fixed, then by Theorem 2.8, all of its neighbors are γ'-totally free. Then by Theorem 2.7, G is of class 2 and hence
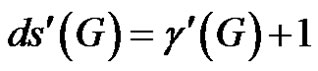(1)
As e is γ'-fixed, by Theorem 2.4,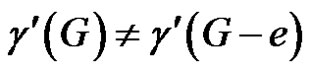
. If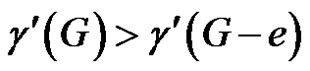
, then e is γ'-critical. Then by Lemma 2.11, e is γ'-free and this is a contradiction. Therefore
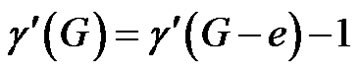. Since G is of class 2 and G – e is of class 1, we have
.
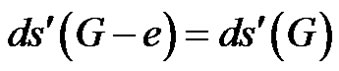2.14. Lemma
Let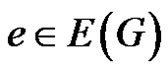
. If e is γ'-fixed and G – e is of class 2, then
.
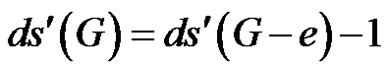Proof
By an argument analogous to that in Lemma 2.13, since G – e is of class 2, we have
.
2.15. Theorem
Let G be a graph without isolated edges. An edge e in G is ds'–-critical if and only if one of the following holds.
1) e is γ'-totally free and G – e is of class 1.
2) e is γ'-free, G is of class 2 and G – e is of class 1.
3) e is γ'-free and both G and G – e are of class 2.
Proof
Suppose e is ds'–-critical. Then
(1)
Let S be a γ'-set of G. Then we have the following cases:
Case (1). G and G – e are of class 1.
By (1),
. By theorem 2.3, e is γ'- free and no γ'-set of G-e contains any edge of N(e). Now every neighbor of e is γ'-totally free in G – e. Therefore G – e is of class 2, which is a contradiction.
Case (2). G is of class 1 and G – e is of class 2.
Then Equation (1) becomes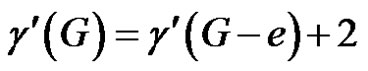
. But this is not possible.
Case (3). G is of class 2 and G – e is of class 1.
Then Equation (1) becomes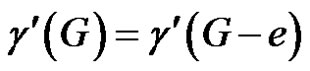
. Then either e is γ'-free or γ'-totally free.
Case (4). G and G – e are of class 2.
In this case, Equation (1) becomes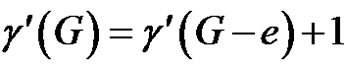
. Then by theorem 2.3, e is γ'-free.
From Lemmas 2.9, 2.11 and 2.12, the converse is true.
2.16. Theorem
Let G be a graph without isolated edges. An edge e in G is ds'+-critical if and only if one of the following holds.
1) e is γ'-free, G is of class 1 and G – e is of class 2.
2) e is γ'-fixed and G – e is of class 2.
Proof
Suppose e in G is ds'+-critical. Hence
(1)
Let S be a γ'-set of G. Then we have the following cases:
Case (1). G and G-e are of class 1.
From equation (1) 
and so G is γ'+-critical. Hence by Theorem 2.6, e is γ'-fixed, which is a contradiction.
Case (2). G is of class 1 and G – e is of class 2.
Now equation (1) becomes
. Then S must contain a neighbor of e. Since G is of class 1, e is γ'-free.
Case (3). G is of class 2 and G – e is of class 1.
Then Equation (1) becomes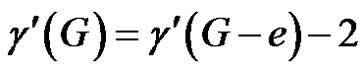
, which is not possible.
Case (4). G is of class 2 and G – e is of class 2.
In this case, Equation (1) becomes
. Then by Theorem 2.4, e is γ'-fixed.
Conversely, suppose if (1) or (2) is true. Then by case (1) of Lemma 2.12 and Lemma 2.14, the result follows.
3. Edge-Domsaturation Number of a Graph
Theorem
For any connected unicyclic graph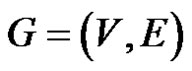 
with cycle C, 
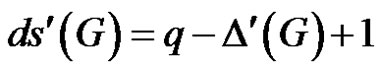if and only if one of the following holds.
1) 
, 
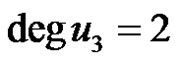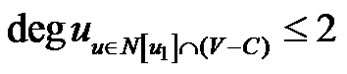and there exists
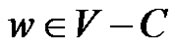such that
, i = 1, 2.
2) 
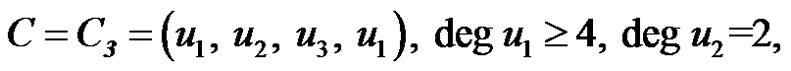
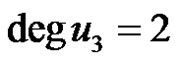, exactly one vertex w not on C has 
and remaining vertices are pendent vertices.
Proof
Suppose
.
Let 
be the unique cycle in G.
If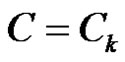
, then 
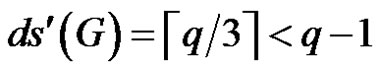for all n ≥ 3 and so
.
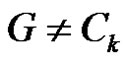Let S denote the set of all pendent edges of G and let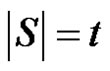
.
Claim 1: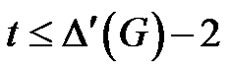
. Since 
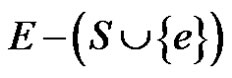is an edge dominating set for any edge e of C,
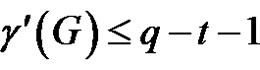. For any pendent edge f, 
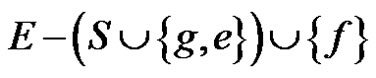is an edge dominating set of G containing f. Here g is an edge adjacent to f and e is any edge of the cycle. Hence
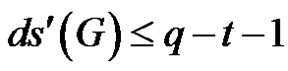, so that
.
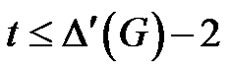Claim 2: e = uv is an edge with degree ∆'. Then either u or v lies on Ck.
Now let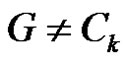 
and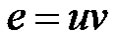 
be an edge of maximum degree ∆'. If e Î Ck, then for some edge 
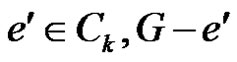is a tree T of G with at least 
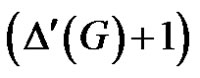pendent edges. If X is the set of all pendent edges of G – e, then 
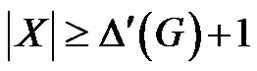. Then 
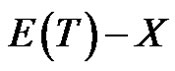is an edge dominating set of cardinality at most
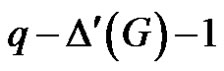. Therefore 
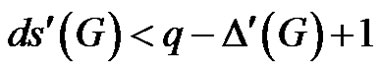, which is a contradiction.
Case (1). u or v lies on C.
Claim 3: 
is the union of P1 and P2. Suppose not. Then,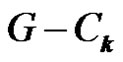 
contains
. Suppose 
lies on CK. Let 
be the maximal tree rooted at u1 not containing any edge of Ck. Clearly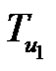 
has at least ∆'(G) – 2 pendent edges, say S. Then
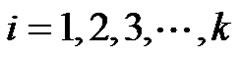
is an edge dominating set of cardinality less than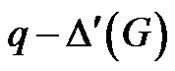
. Therefore
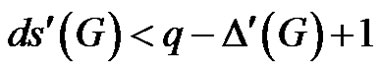, which is a contradiction.
In this case, G has at least ∆' – 2 pendent edges. Let W be the set of these pendent edges. Further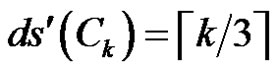 
and let Y denote a γ'-set of Ck. Let
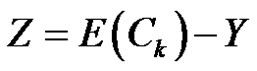. If k > 4, then 
is an edge dominating set of cardinality less than 
Hence 
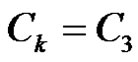or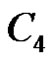
. Since
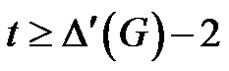. By claim 1,
.
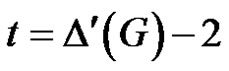Subcase (1).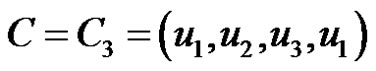 
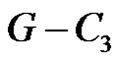is the union of P1 and P2. Also u or v lies on C. Let
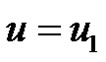. Therefore 
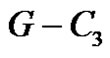contains at least one P2. Since
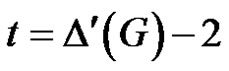, no other vertex other than u and v has degree > 3.
If G – C3 is the union of 
alone, then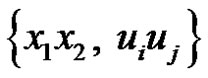 
or 
is an edge dominating set and every edge lies in a γ'-set. Therefore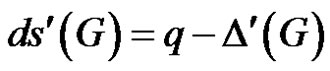
.
If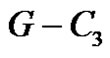 
is the union of 
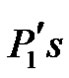and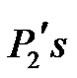
, then from Theorem 1.5,
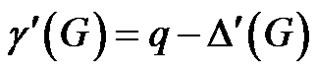. But pendent edges adjacent to u1 does not lie in any γ'-set. Therefore
.
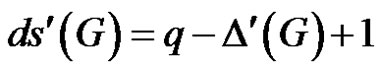Subcase (2).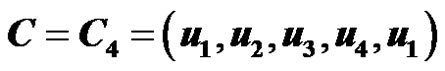 
As in subcase (1), G – C4 also contains P2. Then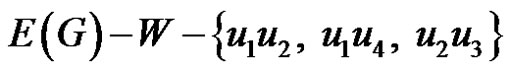 
is an edge dominating set of cardinality
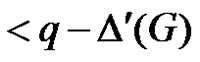. Therefore
.
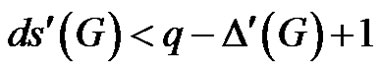Case (2). u and v lies on C.
Claim 4: G – Ck is the union of P1 and P2.
Suppose not. Then, G – Ck contains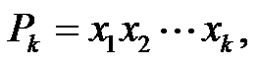 
. Suppose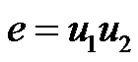 
lies on Ck. Let 
.
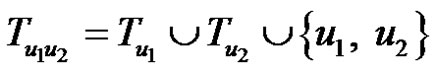Clearly 
has at least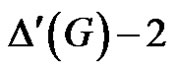 
pendent edges, say P.
Then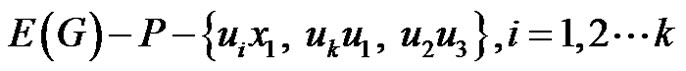 
is an edge dominating set of cardinality less than
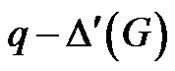. Therefore
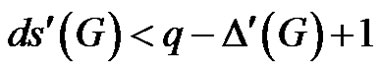, which is a contradiction.
As in case (1),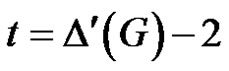
. Let 
be an edge of maximum degree.
Subcase (1). 
In this case, from Theorem 1.5, (3), u3u1 does not belong to any γ'-set. Therefore
.
Subcase (2). 
From Theorem 1.5, there does not exist an edge dominating set of cardinality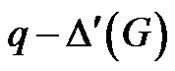
.
The converse is obvious.
4. Well-Edge Dominated Graph
A graph G is called well dominated if all minimal dominating sets have the same cardinality. This concept was introduced by Finbow, Hartnell and Nowakowski [6].
4.1. Definition
A graph G is well-edge dominated if every minimal edge dominating set of G has the same cardinality.
4.2. Lemma
If G is a well-edge dominated graph and e is an edge of G, then there exists a minimum edge dominating set containing e and a minimum edge dominating set not containing e.
Proof
To obtain an edge dominating set containing e, place e in the set D, delete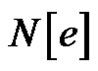 
from G and continue in this greedy fashion until there are no edges left. Then D is minimal and since G is well-edge dominated, it is minimum.
To obtain a minimum edge dominating set not containing e, we use the same greedy method except that we use a neighbor of e as our initial edge in D.
4.3. Theorem
If G is well-edge dominated, then G is of class 1.
Proof
From the above lemma, it is clear that every edge belongs to any one of the γ'-set. Therefore G is of class 1.