1. Introduction
In financial industry, the value-at-risk has been one of main tools for risk management (see, e.g., McNeil et al. [1] , and Föllmer and Schied [2] ). In this framework, the random variables for assets or asset returns are assumed to have distributions without uncertainty. In other words, it is implicitly assumed that there are true asset distributions and the estimation difficulty comes from our limited capability. However, it should be remarked that there is a possibility that the assets have the distribution uncertainty, i.e., the assets may have Knightian uncertainty (see Knight [3] ).
To capture the distribution uncertainty, the theory of sublinear expectation is introduced and developed (see Peng [4] [5] and the references therein). In this theory, the term probability is replaced by the ones of the upper and lower capacities induced by the upper and lower expectations, respectively, and the distribution uncertainty is described by the gap between the upper and lower expectations.
In this paper, we consider the value-at-risk type risk measure under the sublinear expectation, where the reference probability measure in the classical framework is replaced by the upper and lower capacities. We call these the upper and lower value-at-risk, respectively. Our aim is to study the asymptotic behavior of the historical value-at-risk under uncertainty. In doing so, we prove a generalization of Glivenko-Cantelli lemma under uncertainty, and then show that the historical value-at-risk eventually lies in between the upper and lower value- at-risks quasi surely.
This paper is organized as follows: In Section 2, we recall the theory of sublinear expectation. Section 3 is devoted to the statement of the main results and its proofs.
2. Sublinear Expectation and Capacities
In this section, we recall the basis of the sublinear expectation, introduced by Peng [4] . Let Ω be a given set and 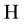 a linear space of
a linear space of 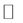 -valued functions on Ω. We assume that
-valued functions on Ω. We assume that  whenever
whenever 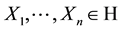 and
and 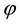 is a bounded function on
is a bounded function on 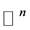 or
or  where
where  denotes the linear space of functions
denotes the linear space of functions  on
on 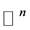 satisfying
satisfying

for some 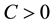 and
and  depending on
depending on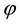 . We call an element in
. We call an element in 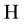 a random variable.
a random variable.
We consider a sublinear expectation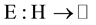 , in the sense of [4] . Namely, E is assumed to be satisfy the following conditions: for any
, in the sense of [4] . Namely, E is assumed to be satisfy the following conditions: for any![]() ,
,
1) Monotonicity: if ![]() then
then![]() .
.
2) Constant preserving: ![]() for
for![]() .
.
3) Subadditivity:![]() .
.
4) Positive homogeneity: ![]() for
for![]() .
.
Moreover, we assume that ![]() for
for ![]() with
with ![]() for each
for each![]() . Then, by Theorem 2.1 and Remark 2.2 in [5] , there exists a set
. Then, by Theorem 2.1 and Remark 2.2 in [5] , there exists a set ![]() of probability measures on
of probability measures on ![]() such that
such that
![]()
where ![]() denotes the linear expectation with respect to
denotes the linear expectation with respect to![]() . Then, the
. Then, the ![]() -valued set functions
-valued set functions
![]()
define capacities, where 1A denotes the indicator function of a set A. That is, each ![]() satisfies the following:
satisfies the following:
1)![]() ,
,![]() .
.
2) If ![]() satisfy
satisfy ![]() then
then![]() .
.
We refer to Denenberg [6] for the theory of capacities. Throughout this paper, we assume that each ![]() satisfies the following:
satisfies the following:
3) If ![]() satisfies
satisfies![]() , then
, then![]() .
.
4) If ![]() satisfies
satisfies![]() , then
, then![]() .
.
Let us recall several concepts in the sublinear expectation theory. The random variable ![]() is said to be independent of
is said to be independent of![]() , where
, where![]() ,
, ![]() , if
, if
![]()
We say that ![]() and
and ![]() have the same distribution if
have the same distribution if
![]()
A sequence ![]() is called the one of independent, identically distributed random variables if
is called the one of independent, identically distributed random variables if ![]() and
and ![]() have the same distribution, and if
have the same distribution, and if ![]() is independent of
is independent of ![]() for any
for any![]() . As in the linear case, we call a sequence of independent, identically distributed random variables an IID sequence. We say that the distribution of X has an uncertainty if
. As in the linear case, we call a sequence of independent, identically distributed random variables an IID sequence. We say that the distribution of X has an uncertainty if ![]() is nonlinear in
is nonlinear in![]() . In particular, set
. In particular, set
![]()
Then if![]() , then we say that X has the mean uncertainty. Similarly, X is said to have the volatility or variance uncertainty if
, then we say that X has the mean uncertainty. Similarly, X is said to have the volatility or variance uncertainty if![]() .
.
3. Main Resutls
For any![]() , we define the functions
, we define the functions ![]() by
by
![]() (1)
(1)
Proposition 3.1 Let ![]() and let
and let ![]() and
and ![]() be as in (1). Then each
be as in (1). Then each ![]() satisfies the following:
satisfies the following:
1) ![]() for
for ![]() with
with![]() .
.
2)![]() ,
,![]() .
.
3) ![]() for
for![]() .
.
Proof. The assertion (1) follows from the monotonicity of ![]() and
and![]() .
.
To prove (2), take ![]() and set
and set![]() . We will see
. We will see ![]() for any sequence
for any sequence ![]() with
with![]() . By setting
. By setting ![]() we have
we have ![]() and
and ![]() . It follows from the definition of the capacity that
. It follows from the definition of the capacity that
![]()
Similarly, ![]() follows.
follows.
Finally, by an argument similar to the proof of (2) with![]() , we can show
, we can show ![]() for any sequence
for any sequence ![]() with
with![]() , implying (3). □
, implying (3). □
The proposition above justifies the following definition:
Definition 3.2 For a random variable X, we call the function ![]() and
and ![]() as in (1) the upper and lower cumulative distribution functions of X, respectively.
as in (1) the upper and lower cumulative distribution functions of X, respectively.
For an IID sequence![]() , the empirical distribution function
, the empirical distribution function ![]() of
of ![]() is defined by
is defined by
![]()
If ![]() satisfies
satisfies ![]() for some
for some![]() , then by Strong law of large number under sublinear expectation (see Theorem 1 in Chen [7] ),
, then by Strong law of large number under sublinear expectation (see Theorem 1 in Chen [7] ),
![]() (2)
(2)
Indeed,
![]()
![]()
We show a stronger result, which is a generalization of Glivenko-Cantelli lemma.
Theorem 3.3 Let ![]() be an IID sequence with
be an IID sequence with ![]() for some
for some![]() . Denote by
. Denote by ![]() and
and ![]()
the upper and lower cumulative distribution functions of X respectively, and denote by ![]() the empirical disribution function of
the empirical disribution function of![]() . Then,
. Then,
![]()
We need the following lemma for the proof of the theorem.
Lemma 3.4 Under the assumtions imposed in Theorem 3.3, for![]() , there exist
, there exist ![]() and
and
![]() such that
such that ![]() and
and
![]()
where![]() ,
, ![]() , for each
, for each![]() .
.
Proof. Let![]() . Then there exists
. Then there exists ![]() such that
such that![]() . Starting with
. Starting with![]() , we recursively define
, we recursively define ![]() by
by
![]()
By this recursion, we can find ![]() such that
such that ![]() or
or ![]() hold, and set
hold, and set![]() . With this sequence, the lemma follows. □
. With this sequence, the lemma follows. □
With the help of Lemma 3.4, we can show Theorem 3.3.
Proof of Theorem 3.3. Let ![]() be fixed. By Lemma 3.4, there exists a partion of
be fixed. By Lemma 3.4, there exists a partion of ![]() such that
such that ![]() and
and
![]() (3)
(3)
By (2), we have, ![]() for any
for any![]() , where
, where
![]()
Thus, ![]() for any
for any ![]() and so
and so ![]() for any
for any![]() . Hence
. Hence
![]() ,
,
where for ![]()
![]()
So we have
![]()
Now, for any ![]() there exists j such that
there exists j such that![]() . Thus, by (3),
. Thus, by (3),
![]()
![]()
so
![]()
Therefore, on![]() , letting
, letting ![]() we get
we get ![]() and
and ![]() for all
for all![]() . Thus
. Thus
![]()
If we write ![]() for the event inside the brace above and denote
for the event inside the brace above and denote![]() , then by the continuity of the capacity
, then by the continuity of the capacity
![]()
meaning the assertion of the theorem. □
Recall that for a function ![]() satisfying (1)-(3) in Proposition 3.1 and for
satisfying (1)-(3) in Proposition 3.1 and for![]() , the
, the ![]() - quantile
- quantile ![]() of F is defined by
of F is defined by
![]()
Then we have the following:
Theorem 3.5 Let ![]() be an IID sequence with
be an IID sequence with ![]() for some
for some![]() . Denote by
. Denote by ![]() and
and ![]() the upper and lower cumulative distribution functions of X respectively, and denote by
the upper and lower cumulative distribution functions of X respectively, and denote by ![]() the empirical disribution function of
the empirical disribution function of![]() . Consider the upper, lower, and historical value-at-risk defined respectively by
. Consider the upper, lower, and historical value-at-risk defined respectively by
![]()
Suppose that for ![]()
![]() (4)
(4)
Then, the historical value-at-risk eventually lies in between the upper and lower value-at-risk, i.e.,
![]()
Proof. Consider the event A defined by
![]()
In view of Theorem 3.3, it suffices to show that for a given ![]() and
and ![]() there exists
there exists ![]() such that
such that
![]() (5)
(5)
To this end, fix ![]() and set
and set![]() ,
,![]() . By the definition of the infimum and the condition (4),
. By the definition of the infimum and the condition (4),
![]()
Thus we can take ![]() such that
such that
![]()
Next, take ![]() such that
such that
![]()
and set![]() . Then,
. Then,
![]()
So we have![]() . Similarly, we see
. Similarly, we see
![]()
leading to![]() . Thus (5) follows. □
. Thus (5) follows. □