Boundary Control for 2 × 2 Elliptic Systems with Conjugation Conditions ()
1. Introduction
So many optimal control problems governed by partial differential equations have been studied as in [1-3].
Systems governed by elliptic, parabolic, and hyperbolic operators have been considered, some of which are of distributed type as in [4-12], while some others are of boundary type as in [13-17].
Boundary control problems for non-cooperative  elliptic systems involving Laplace operator have been discussed in [17].
elliptic systems involving Laplace operator have been discussed in [17].
Here, using the theory of [3], we study the boundary control problem for 2 × 2 non-cooperative elliptic systems involving Laplace operator but under conjugation conditions.
Let us consider the following elliptic equations:
 (1.1)
(1.1)
the heterogeneous boundary Neumann conditions:
 (1.2)
(1.2)
and the conjugation conditions:
 (1.3)
(1.3)
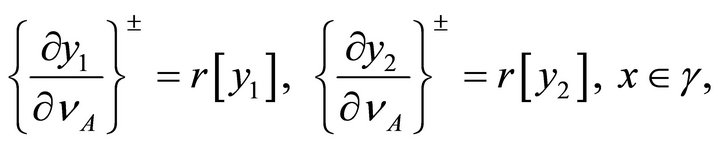 (1.4)
(1.4)
where we have the following notations:
Ω is a domain that consists of two open, non-intersecting and strictly Lipschitz domains Ω1 and Ω2 from an n-dimensional real linear space Rn i.e. Ω1,  are bounded, continuous, and strictly Lipschitz domains such that
are bounded, continuous, and strictly Lipschitz domains such that

Furthermore,  is a boundary of a domain
is a boundary of a domain ,
,  , and
, and  is a boundary of a domain
is a boundary of a domain , i = 1, 2.
, i = 1, 2.
In addition,
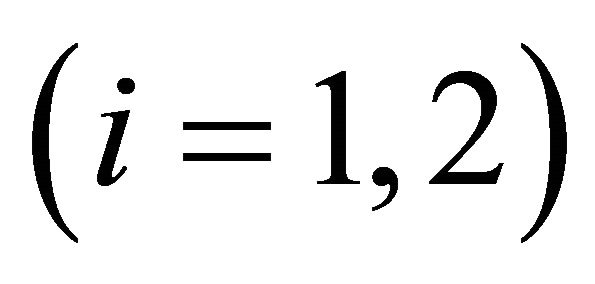
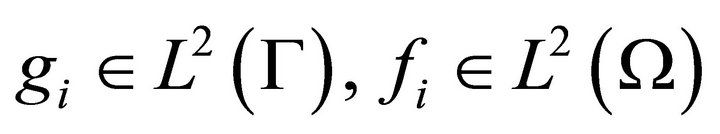 ,
,
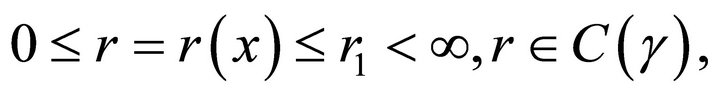
r1 = constant, and n is an ort of an outer normal to G.
Finally, 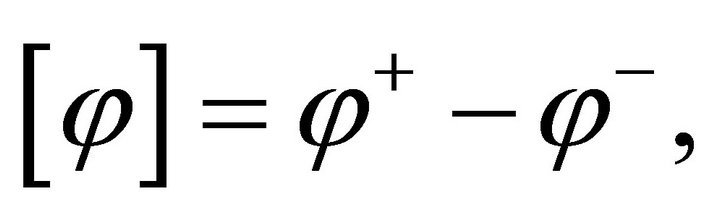
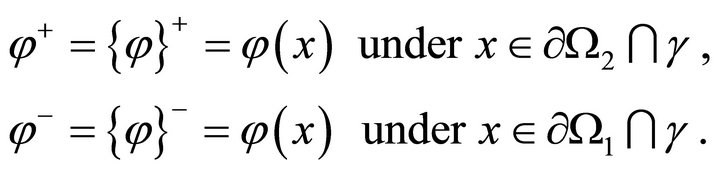
The model of system (1) is given by:
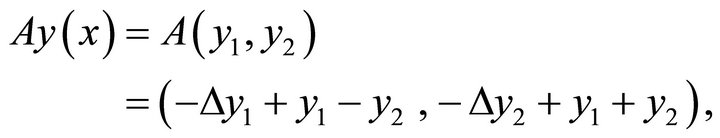
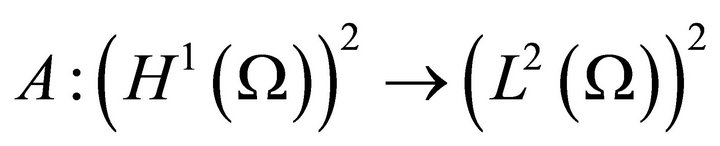
system (1) is called non-cooperative, since the coefficients took the previous form.
We first prove the existence and uniqueness for the state of system (1), then we formulate the control problem. We also prove the existence and uniqueness of the optimal control of boundary type, and we discuss the necessary and sufficient conditions of the optimality.
2. The Existence and Uniqueness for the State of System (1)
Since , then by Cartesian product we have the following chain [1]:
, then by Cartesian product we have the following chain [1]:
 .
.
On , we define the following bilinear form:
, we define the following bilinear form:
 (2)
(2)
The bilinear form (2) is continuous, since:
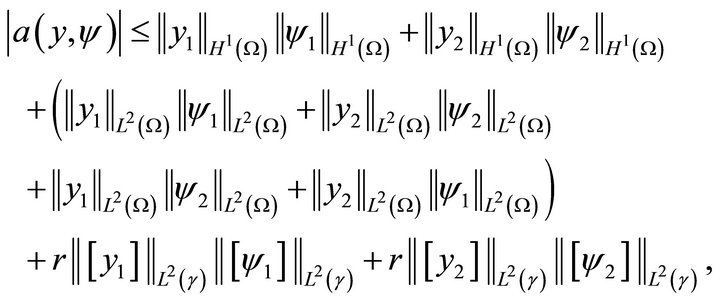
since the inequalities
 are true
are true
[3]. Then we have:
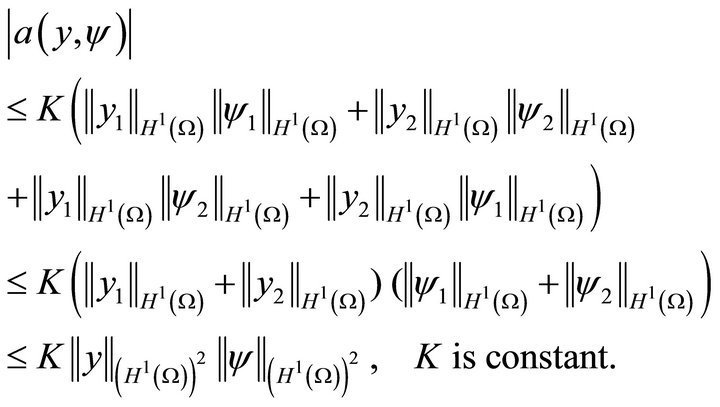
Now, we have the following lemma:
Lemma 1:
The bilinear form (2) is coercive on , that is, there exists l Î R, such that:
, that is, there exists l Î R, such that:

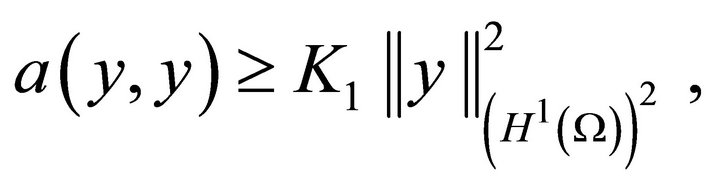
Proof:
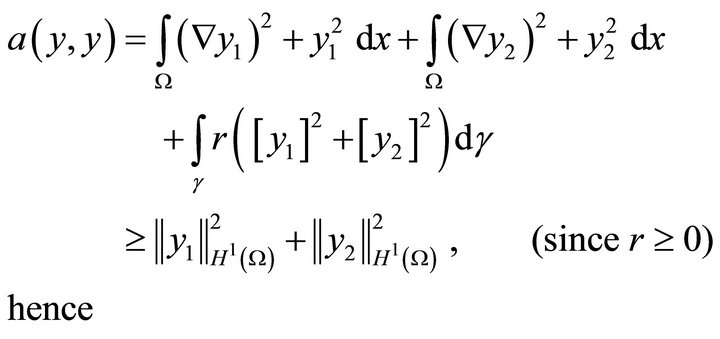
 (3)
(3)
which proves the coerciveness condition of the bilinear form (2). Then we have the following theorem:
Theorem 1:
For a given , there exists a unique solution
, there exists a unique solution  for system (1).
for system (1).
Proof:
Since (3) is hold, then by Lax-Milgram lemma, there exists a unique element
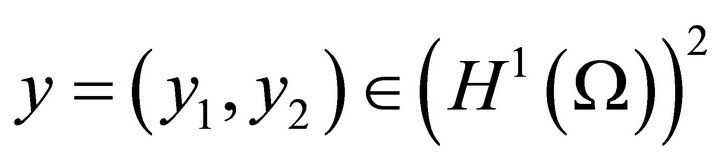

such that
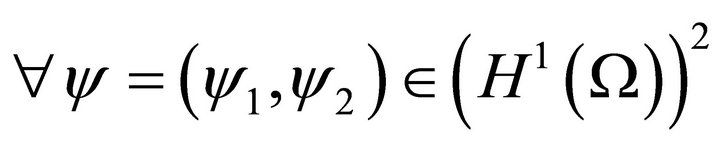
 , (4)
, (4)
where  is defined by:
is defined by:

 . (5)
. (5)
The linear form (5) is continuous, since:

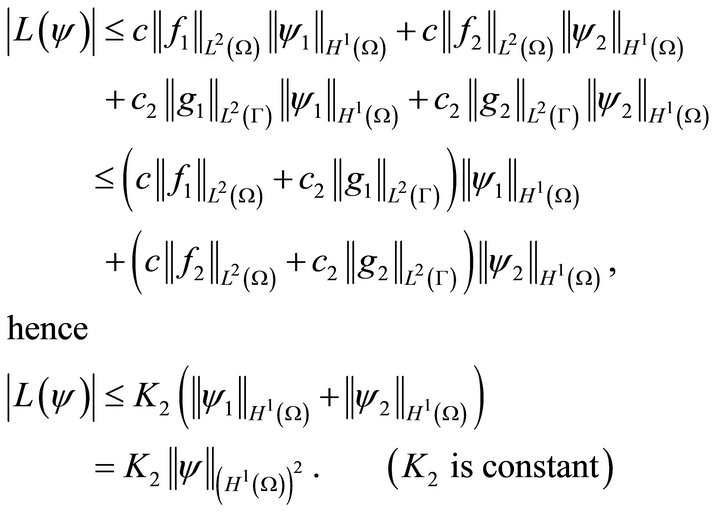
Now, let us multiply both sides of first equation of (1.1) by , and the second equation by
, and the second equation by 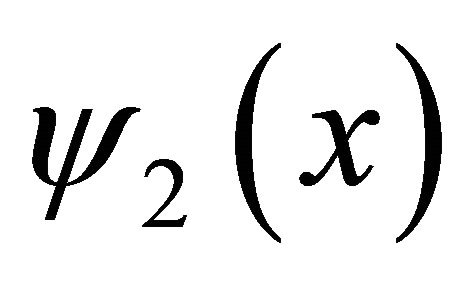 then integration over W, we have:
then integration over W, we have:
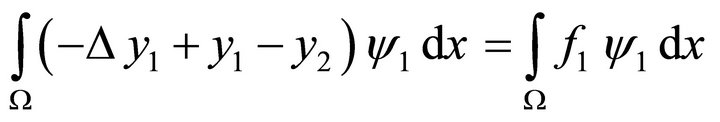 ,
,
 .
.
By applying Green’s formula:
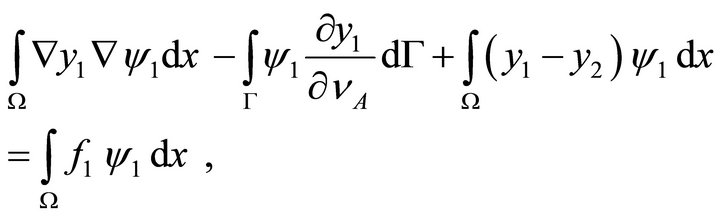

by sum the two equations, then comparing the summation with (2), (4) and (5) we obtain:
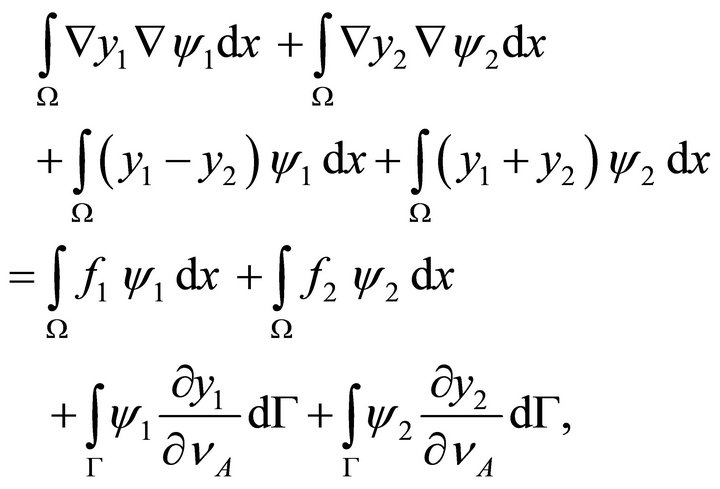
then we deduce (1.2), which completes the proof.
3. Formulation of the Control Problem
The space  is the space of controls. For a control
is the space of controls. For a control , the state
, the state
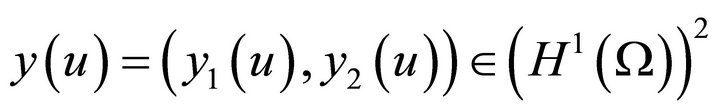 of system (1) is given by the solution of the following systems:
of system (1) is given by the solution of the following systems:
 (6.1)
(6.1)
 (6.2)
(6.2)
and the conjugation conditions:
 (6.3)
(6.3)
 (6.4)
(6.4)
Since there exists a generalized solution
 to the boundary value problem (6)then such solution is reasonable on
to the boundary value problem (6)then such solution is reasonable on  of
of , and
, and
 . (7)
. (7)
The observation equation is given by:
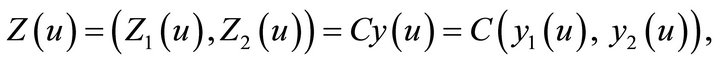
where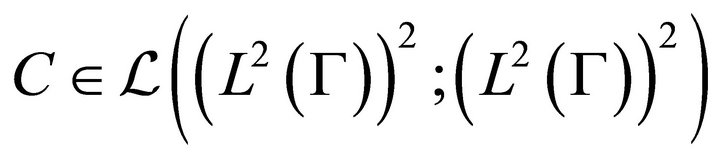 , namely:
, namely:
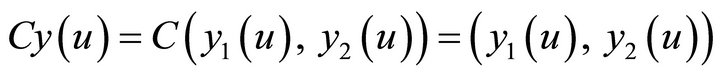
i.e.
 (8)
(8)
For a given , the cost function is given by
, the cost function is given by
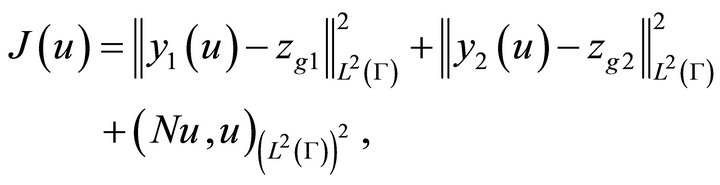 (9)
(9)
where 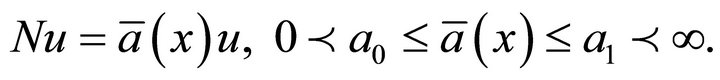
The function 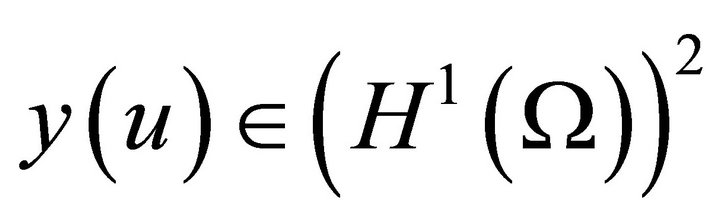 is specified on the domain
is specified on the domain  , minimizes the energy functional:
, minimizes the energy functional:
 (10)
(10)
on , and it is the unique solution in
, and it is the unique solution in
 to the weakly stated problem of finding an element
to the weakly stated problem of finding an element  that meets the following integral equation:
that meets the following integral equation:
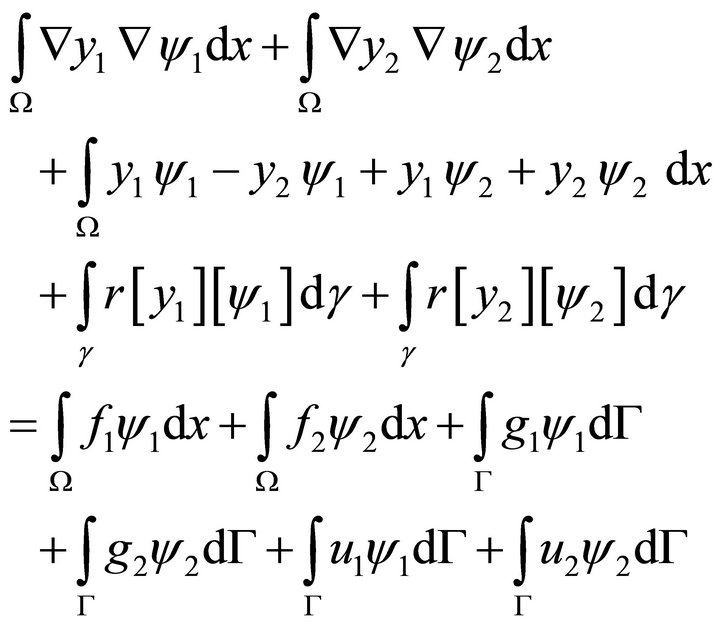
 . (11)
. (11)
The control problem then is to find  such that
such that , where Uad is a closed convex subset of
, where Uad is a closed convex subset of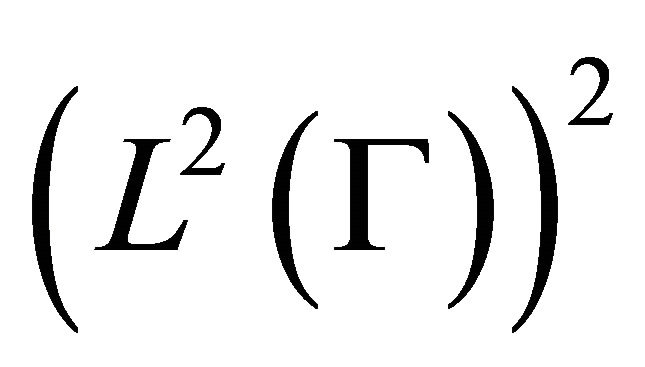 .
.
The cost function (9) can be written as (see [1]):
 (12)
(12)
In this case, the bilinear form 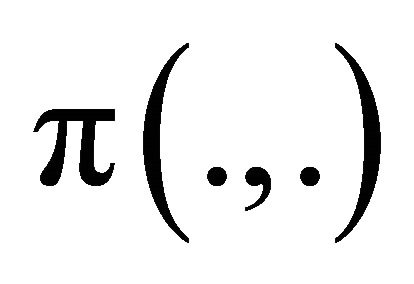 and the linear form
and the linear form  are expressed as:
are expressed as:
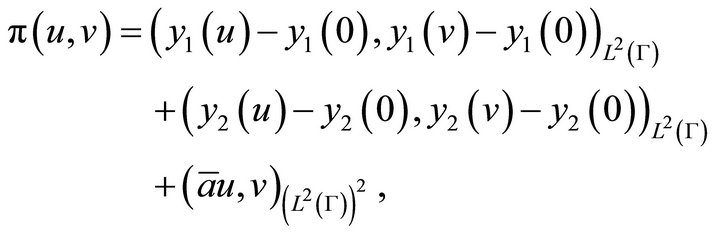 (13)
(13)
 (14)
(14)
Now, we prove the continuity of 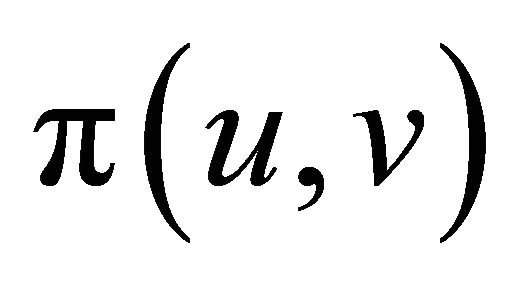 and
and 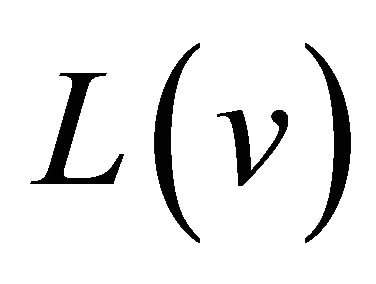
on  as follows [3]:
as follows [3]:
Let  and
and  be solutions from
be solutions from  to problem (11) under f = 0 and g = 0. Then from the bilinear form
to problem (11) under f = 0 and g = 0. Then from the bilinear form  which is given by (2), we can derive the following inequality:
which is given by (2), we can derive the following inequality:

Since , then:
, then:
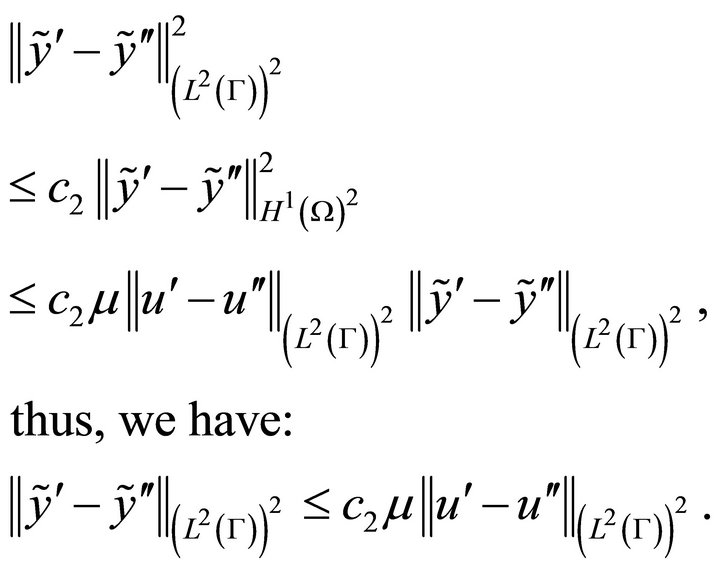
i.e. the function  is continuously dependent on u.
is continuously dependent on u.
Then the continuity of  and L(v) on
and L(v) on  is proved.
is proved.
The bilinear form  is coercive on
is coercive on 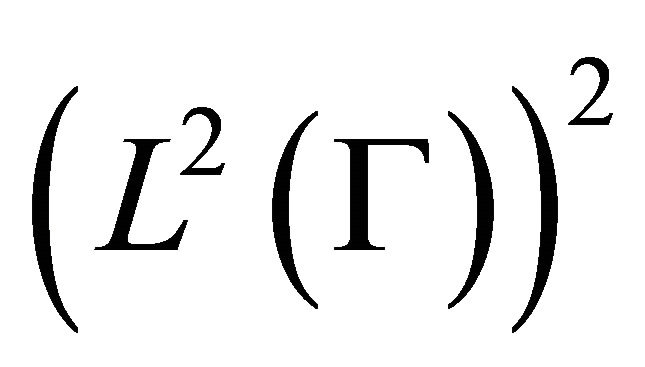
since Thus:
Thus:

Then by Lax Milgram lemma, the following theorem is proved. Moreover, it gives the necessary and sufficient conditions of optimality.
Theorem 2:
Assume that (3) holds, there exists a unique optimal control 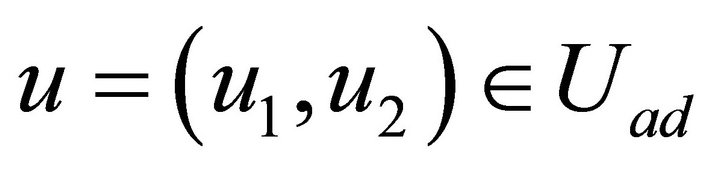 that is closed convex subset of
that is closed convex subset of  and it is then characterized by the following equations and inequalities:
and it is then characterized by the following equations and inequalities:
 (15.1)
(15.1)
 (15.2)
(15.2)
 (15.3)
(15.3)
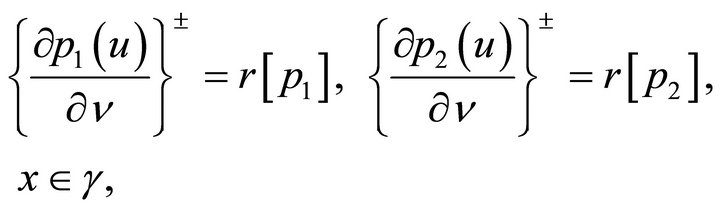 (15.4)
(15.4)
 (16)
(16)
together with (6), where
 is the adjoint state.
is the adjoint state.
Proof:
The optimal control  is characterized by (see [1])
is characterized by (see [1])
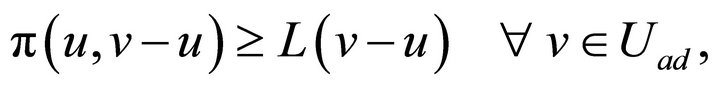 (17)
(17)
by (13), and (14):

thus:
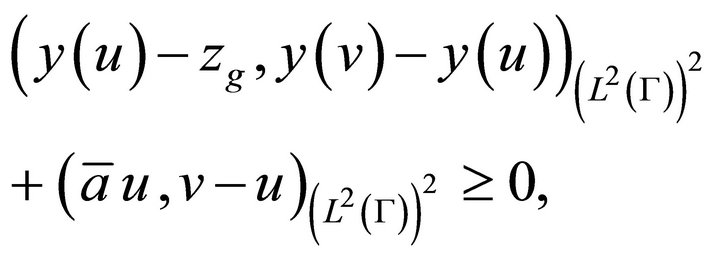
this inequality can be written as
 (18)
(18)
Now, since: , then:
, then:
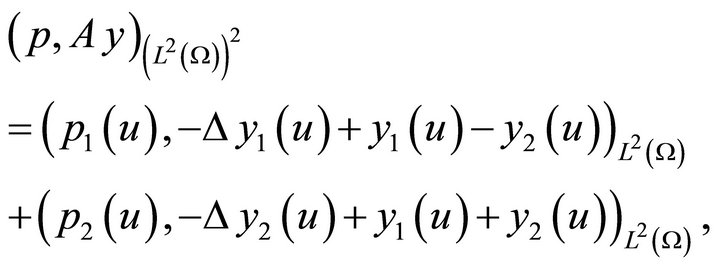
by using Green’s formula, we obtain:

then
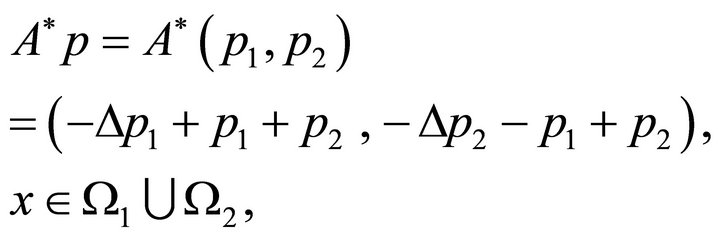 (19)
(19)
Since the adjoint system takes the form [3]:
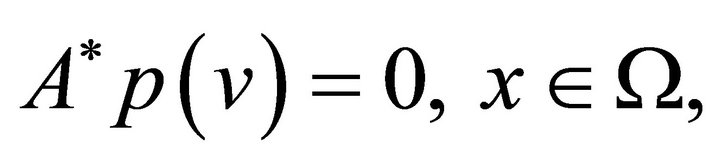 (20.1)
(20.1)
 (20.2)
(20.2)
 (20.3)
(20.3)
and by using (19), system (15) is proved .
From Green’s formula the following equations are true:
 (21)
(21)
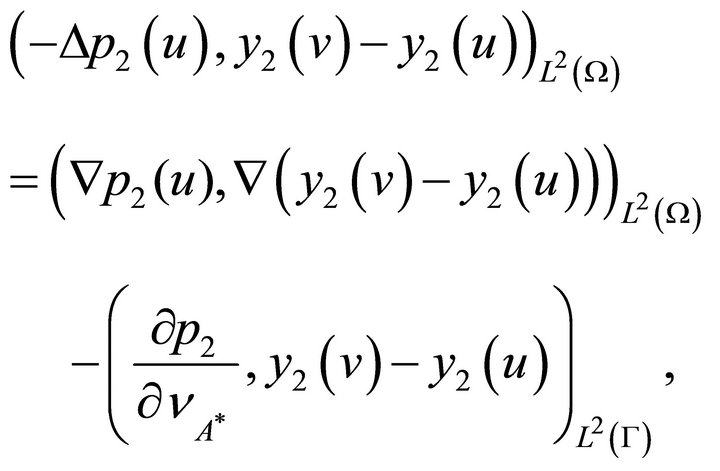 (22)
(22)
by adding
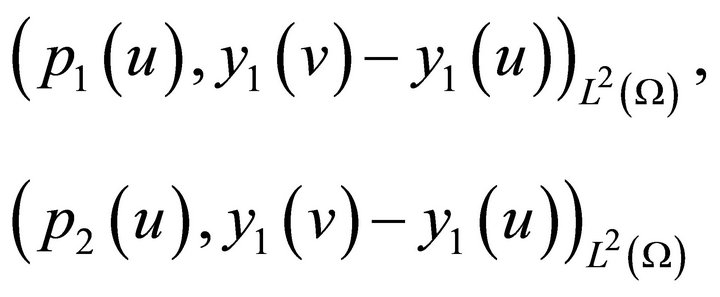
to the both sides of Equation (21), and

to the both sides of Equation (22), then by (15) we obtain:
 (23)
(23)
and
 (24)
(24)
Now, we transform (18) by using (15) as follows:
 (25)
(25)
by (23) and (24), we have:

by using (2):
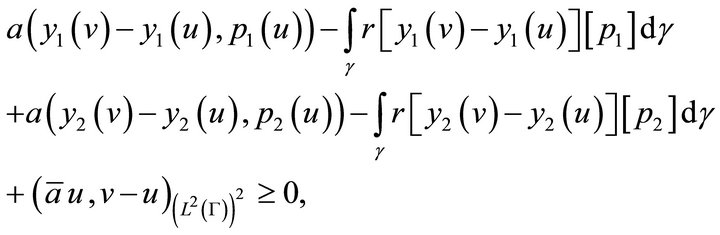
from (2), and using Green’s formula:

from (6), we obtain:

which proves (16).
Remark:
If the constraints are absent, i.e. when
 , then the equality:
, then the equality:
 follows from condition (16).
follows from condition (16).
Hence

 . (26)
. (26)
4. Conclusions
The main result of the paper contains necessary and sufficient conditions of optimality (of Pontryagin’s type) for 2 ´ 2 elliptic systems under Neumann conjugation conditions involving Laplace operator defined on bounded, continuous and strictly Lipschitz domain of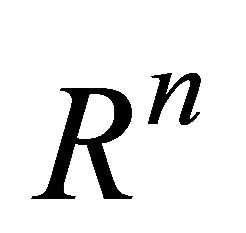 , that give characterization of optimal control.
, that give characterization of optimal control.
We can consider boundary control problems for 2 ´ 2 and n ´ n elliptic distributed systems with Dirichlet conjugation boundary conditions. Also we can consider boundary control problems for parabolic and hyperbolic distributed systems with Dirichlet and Neumann conjugation boundary conditions. The ideas mentioned above will be developed in forthcoming papers.
Also it is evident that by modifying:
- the boundary conditions- the nature of the control (distributed, boundary(- the nature of the observation- the initial differential systemmany of variations on the above problem are possible to study with the help of Lions formalism.
5. Acknowledgements
The authors would like to express his gratitude to Professor H. M. Serag, Mathematics Department, Faculty of Sciences, AL-Azhar University for suggesting the problem and critically reading the manuscript.