Reverse Building of Complete (k,r)-Arcs in PG(2,q)

Mina Ghareeb Faraj, Nada Yassen Kasm

Department of Mathematics, College of Education for pure Sciences, University of Mosul, Mosul, Iraq.

DOI: 10.4236/oalib.1105900                

Abstract

The purpose of this work is to study the construction of complete (ki,i)-arcs in PG(2,9), where i=q,9-1,...2 by eliminating points from a complete (kn,n)-arc to get a complete(km,m)-arc, where m < n. And we adopted a new sequential way to delete points [1] [2].

Keywords

Complete Arcs, Maximal Arcs, Galois Geometry

1. Introduction

A projective plane PG(2,q) over Galois field GF(p), where q is a prime number, consists of $q^2+q+1$ points and $q^2+q+1$, every line contains $q+1$ point and every point is on $q+1$ lines [3]. Any point of the plane has the form of a triple, where $X_0,X_1,X_2$ are elements in PG(q) with the exception of a triple consisting of three zero elements. Two triples $\left(X_0,X_1,X_2\right)$ and $\left(Y_0,Y_1,Y_2\right)$ represent the same point if there exists j in GF(P)/{0}, s.t. $\left(Y_0,Y_1,Y_2\right)=J\left(X_0,X_1,X_2\right)$.

The points in PG(2,q) have unique forms which are (1,0,0), (X,1,0), (X,Y,1) for all X,Y in GF(q). There exist one point of the from (1,0,0), q points of the from (X,1,0) and q² points of the from (X,Y,1) similarly any line in PG(2,q) has the form $\left(X_0,X_1,X_2\right)$, $X_0,X_1,X_2$ are elements in GF(q) with the exception of a triple consisting of three zero elements. Two triples represent the same line if thereexist J GF(q)\{0}, s.t $\left(Y_0,Y_1,Y_2\right)=J\left(X_0,X_1,X_2\right)$. A point $P\left(X_0,X_1,X_2\right)$ is incident with the line $\left(Y_0,Y_1,Y_2\right)$ iff $x_0{y}_0+x_1{y}_1+x_2{y}_2=0$ [4].

Finally, the points of PG(2,q) can be numerated as follows: the number of the point $\left(1,0,0\right)$ is 1, the point is $\left(X,1,0\right)$ numerated as X + 2, the point $\left(X,Y,1\right)$ is numerated as $\left(x+\left(x\cdot q\right)+q+2\right)$ [5].

Definition 1: A(K,n)-a in PG(2,q) is asset of K points such that NO n + 1 points them are collinear A(K,2)-arc which is called K-arc is a set of K points such that NO three of them are collinear [6].

Definition 2: A(k,n)-arc is a complete if it is not contained in a (k + 1,n)-arc [6].

Definition 3: The maximum number of points that a (K,2)-arc can have is m(2,q) and a (K,2)-arc with this number of points is an Oval. In the even case Ovals are complete [7].

Theorem 1: $M\left(2,q\right)=\{\begin{array}{l}q+1\text{for}q\text{isodd}\\ q+2\text{for}q\text{iseven}\end{array}$ [7].

Theorem 2: In PG(2,q), with q odd, every oval is conic [8].

Definition 4: The i-secant of a (K,n)-arc K is a line intersects the arc in exactly i points, a 0-secant is called an external line K, a 1-secant is called a uni secant line, 2-secant is called a bisecant line and 3-secant is called a trisecant line [9].

Corollary 1: A (k,n)-arc K is a maximal if and only if every line PG(2,q) is a 0-secant, or an N-secant [10] [11].

Theorem 3: Let m be a point of a (K,2)-arc K and let t(m) be the number of unisecants through m in PG(2,q) then $t=t\left(m\right)=q+2-k$ [12].

Proof: In PG(2,q), there exist exactly q + 1 lines through q. since M is on k then each line passing through M is either unisecant or bisecant of k. There exists exactly k-1 lines joining M with the remaining k-1 points of k which are bisecant of k. then the number of unisecant through M is $q+1-\left(k-1\right)=q+2-k$.

(i.e.). $t\left(m\right)=q+2-k=t$.

Corollary 2: If k is an oval then t(m) = 1 [13].

Theorom 4: Let k be a k-arc in PG(2,q) and let T_i be the number of i-secant of k in the plane, that is, T₂ is the number of bisecant, T₁ the number of unisecants, and T₀ the number of external line, then [13] :

1) $T_2=k\left(k-1\right)/2$ ;

2) $T_1=Kt$ ;

3) $T_0=q\left(q-1\right)+t\left(t-1\right)/2$.

Proof: 1) We have K points in arc K; we take two points from these k point to find the bisecant of k so.

2) There exist exactly K points on arc K. Each point of K has exactly $t=q+2-k$ line of K. The number of unisecants of K is exactly

$T_1=Kt=K\left(q+2-k\right)$.

3) Each line of the plane PG(2,q) is either bisecant, unisecant or external line. The number of lines is $\text{PG}\left(2,q\right)=q^2+q+1=T_0+T_1+T_2$.

$T_0=q^2+q+1-T_1–T_2=\frac{q\left(q-1\right)}{2}+\frac{t\left(t-1\right)}{2}$

Corollary: For a (q + 1)-arcs, t = 1, $T_0=\frac{q\left(q-1\right)}{2}$, $T_1=q+1$, $T_2=\frac{q\left(q+1\right)}{2}$ [13].

Definition 5: Let Q be a point of PG(2,q) not on the K-arc. Let S_i(Q) be the number of i-secants through Q. The number of bisecants S₂(Q) is called the index of Q with respect to K and the number of unisecant S₁(Q) is called the grade of Q with respect to K [14].

Lemma 1: For any point Q in PG(2,q)\K, then $S_1\left(Q\right)+2S_2\left(Q\right)=K$ [14].

Proof: Since each unisecant of K. passes through one point of the arc and each bisecant passing through two points of the arc, the number of the points of the arc is K, then $S_1\left(Q\right)+2S_2\left(Q\right)=K$.

Lemma 2: Let C_i be the number of points Q of index i. Then

1) ${\displaystyle {\sum }_{\alpha }^{\beta }{C}_i}=q^2+q+1-k$ ;

2) ${\displaystyle {\sum }_{\alpha }^{\beta }i{C}_i}=k\left(k-1\right)\left(q-1\right)/2$ ;

where $\alpha$ is smallest i for Which $C_i\ne 0$, and $\beta$ is the largest i for Which $C_i\ne 0$.

Proof: 1) ${\displaystyle \sum C_i}$ represents all the points of the plane not in K. Since the number of point in the plane is $q^2+q+1$, then ${\displaystyle {\sum }_{\alpha }^{\beta }{C}_i}=q^2+q+1-k$

2) ${\displaystyle {\sum }_{\alpha }^{\beta }i{C}_i}=C_1+2C_2+3C_3+\cdots$

{(Q,l)/Q ?l\k, l is a bisecant of K} each bisecant contains q − 1 points not in K.

There are 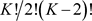Bisecant of K. Then there exist OF points satisfying the equation

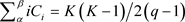
Remark: The (k,n)-arc K is complete if and only if,. Thus, K is complete if every point of PG(2,q) lines on some n-secant of K [15] [16].

2. The Additions and Multiplications Operations of GF(9)

To find the addition and multiplication tables, in GF(9), We have the order pier such that in GF(3), as follows:

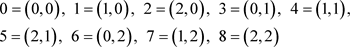
Put these points in one orbit (1,0) at the first point and by the principle of (0,1) A_i, 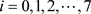and

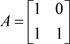
(1,0)

(0,1) (1,1) (1,2) (2,0) (0,2)(1,2) (2,2)

Now, in the left of the following Table 1 is the operation of multiplication, and in the right n is the operation of addition; in multiplication side, it writes the numeration of points as last, and the addition side take the normal sequence [15] [16].

Mod (8): In addition (Table 2), We have the following relation:.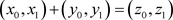

In multiplication (Table 3), we have the following relations 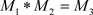. For Example: 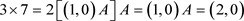where (2,0) is equal to 2 in multiplication side. Now we have the multiplication (Table 3).

Table 1. Operation of multiplication.

Table 2. Point addition.

Table 3. Point multiplication.

2.1. The Construction Complete (k_i,i)-Arc, Where 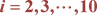in PG(2,9) over GF(9)

The projective plane PG(2,q) contains (91) points and (91) lines, every line contains (10) points and every point is on (10) lines. Any line in PG(2,q) can be constructed by means of variety V. let Pi and be the points and lines of PG(2,9), respectively. Let i stands for the point P_i[i] stands for the L_i whose coordinates are the same coordinates of the point P_i, and all the points and the lines of PG(2,9) are given in Table 4.

2.2. The Construction of (k₁₀,10)-Arc

If i = 10, the M(10,9) = 91 which is the maximal arc, since every line in PG(2,9) is a 10-secan of the (K,10)-arc. This arc contains The construction of (k₉,9)-arc, from the (k₁₀,10)-arc: all the points of the plane PG(2,9), So it is a complete arc. Now, we shall construct the (K,m)-arcs as given in Table 4.

2.3. The Construction of (k₉,9)-Arc, from the (k₁₀,10)-Arc

We eliminate one line from the (k₁₀,10)-arc, say the line L₁₁ = [1, 2, 3, 4, 5, 6, 7, 8, 9, 10]. On ather hand, in projective plane any two distinct lines are intersected in a unique point, the eliminating line intersects any line of PG(2,9) in exactly one point consequently, we eliminate one point from any line in the plane PG(2,9) The eliminated line is a 0-secant of K₉ and the remaining (90) lines are the 9 secants of the arc. We find:

1) K₉ is a maximal (81,9)-arc in PG(2,9), since every line in PG(2,9) is either 0-secant or a 9-secant of K₉, as given in Table 5.

2) K₉ is a complete (81,9)-arc since there are no point 0f index zero for K₉ i.e..

2.4. The Construction of (k₈,8)-Arc k from k₉

In this section, we construct (K₈,8)-arc K₈ from K₉ by eliminating the line L₂ = [11, 12, 13, 14, 15, 16, 17, 18, 19] and following points [20, 29, 38, 47, 56, 65, 74, 83] then find:

1) K₈ is not a maximal (64,8)-arc in PG(2,9) since every line in PG(2,9) is either 0-secant or a 8-secant of K, as given in Table 6.

2) K₈ is acomplete (64,8)-arc since there are no point of index zero for K₈ i.e.,.

2.5. The Construction of (k₇,7)-Arc K from K₈

We construct a (K₇,7)-arc K from K₈ by eliminating one line, the line L₂₉: [20, 21, 22, 23, 24, 25, 26, 27, 28] and the following points [30, 39, 48, 57, 66, 75, 84], then we find:

1) K₇ is not a maximal (49,7)-arc in PG(2,9) since every line in PG(2,9) is either 0-secant or a 7-secant of K₇, as geven in Table 7.

2) K₇ is a complete (49,7)-arc since there are no points of index zero for K₇, i.e..

Table 4. Point and Line of pG(2,9).

Table 5. Point and Line of pG(2,9).

Table 6. Point and Line of pG(2,9).

Table 7. Point and Line of pG(2,9).

2.6. The Construction of (K₆,6)-Arc k₆ from k₇

WE construct a (K₆,6)-arc K₆ from K₇ by eliminating one line, the line L₂₀ = [29, 30, 31, 32, 33, 34, 35, 36, 37] and the following points [40, 49, 58, 67, 76, 85], then we find:

1) K₆ is not a maximal (36,6)-arc in PG(2,9) since every line in PG(2,9) is either 0-secant or a 6-secant of K₆ as given in Table 8.

2) K₆ is a complete (36,6)-arc since there are no points of index zero for K₆, i.e.,.

Table 8. Point and Line of pG(2,9).

2.7. The Construction of (k₅,5)-Arc k₅ from k₆

We construct a (k₅,5)-arc K₅ from K₆ by eliminating one line, the line L₇₄ = [38, 39, 40, 41, 42, 43, 44, 45, 46] and following points [50, 59, 68, 77, 86], then we find:

1) K₅ is not a maximal (25,5)-arc in PG(2,9), since every line in PG(2,9) is either 0-secant or a 5-secant of K₅ as given in Table 9.

2) K₅ is a complete (25,5)-arc since there are no point of index zero for K₅, i.e.

.

2.8. The Construction of (k₄,4)-Arc k₄ from k₅

We construct a (k₄,4)-arc from k₅ by eliminating one line, the line L₄₇ = [47, 48, 49, 50, 51, 52, 53, 54, 55] and following points [60, 69, 78, 87], then we find:

1) K₄ is not a maximal (16,4)-arc inPG(2,9), since every line in PG(2,9) is either 0-secant or a 4-secant of K4 as given in Table 10.

2) K₄ is a complete (16,4)-arc since there are no points index zero for K₄, i.e.

.

2.9. The Constrcution of (k₃,3)-Arc k₃ from k₄

We construct (K₃,3)-arc K₃ from K₄ by eliminting one line, the line L₆₅ = [56, 57, 58, 59, 60, 61, 62, 63, 64] and following points [70, 79, 88], we find:

1) K₃ is not a maximal (9,3)-arc in PG(2,9), since every line in PG(2,9) is either 0-secant or a 3-secant of K₃, as given in Table 11.

2) K₃ is a complete (9,3)-arc since there are no points of index zero for K₃, i.e.

.

2.10. The Construction of (k₂,2)-Arc k₂ from k₃

We construct a (k₂,2)-arc K₂ from K₃ by eliminating one lines the line L₅₆ = [65, 66, 67, 68, 69, 70, 71, 72, 73] and following points [80, 89], then we find:

1) K₂ is not a maximal (4,2)-arc in PG(2,9), since some line in PG(2,9) which are 0-secant, 1-secants and 2-secant of K₂, as given in Table 12.

Table 9. Point and Line of pG(2,9).

Table 10. Point and Line of pG(2,9).

Table 11. Point and Line of pG(2,9).

Table 12. Point and Line of pG(2,9).

2) Since there are no points of index zero for K₂, i.e., (4,2)-arc is a complete arc and it is oval.

Conflicts of Interest

The authors declare no conflicts of interest regarding the publication of this paper.

References

[1]	Yahya, N.Y.K. and Sulaimaan, A.E.M. (2019) New Structural Engineering Con-struction Methods by Remove q Points in PG (2, q). Applied Mathematical Sci-ence, 13, 1048-1041. https://doi.org/10.12988/ams.2019.99134
[2]	Kasm, N.Y. and Ibrahim, H.S. (2019) The Optimal Size of {b, t}-Blocking Set When t = 3, 4 By Intersection the Tangents in PG (2, q). Modern Applied Science, 13, 15. https://doi.org/10.5539/mas.v13n7p15
[3]	Kasm, N.Y. and Ibrahim, H.S. (2019) The Possibility of Applying Rumen Research at the Projective Plane PG (2, 17). Modern Applied Science, 13, 150. https://doi.org/10.5539/mas.v13n8p150
[4]	Yahya, N.Y.K. and Sulaimaan, A.E.M. (2019) Linear Code Related ?-Blocking Sets. Applied Mathematical Science, 13, 1033-1040. https://doi.org/10.12988/ams.2019.99133
[5]	Yahya, N.Y.K. and Hamad, A.Z. (2019) A Geometric Construction of a (56, 2)-Blocking Set in PG (2, 19) and on Three Dimensional Linear [325,3,307]19 Griesmer Code. Rafidain Journal of Computer Sciences and Mathematics, 14, 13-27.
[6]	Yahya, N.Y.K. (2014) A Non PGL (3, q) k-arcs in the Projective Plane of Order 37. Tikrit Journal of Pure Science, 19, 135-145.
[7]	Yahya, N.Y.K. (2018) A Geometric Construction of Complete (k, r)-arc in PG (2, 7) and the Related Projective [n,3,d]7 Codes. Rafidain Journal of Computer Sciences and Mathematics, 12, 24-40.
[8]	Yahya, N.Y.K. and Salim, M.N. (2019) New Geometric Methods for Prove Existence Three-Dimensional Linear [97,3,87]11 and [143,3,131]13 Codes. Journal of Education and Science, 28, 312-333. https://doi.org/10.33899/edusj.2019.161057
[9]	Yahya, N.Y.K. and Sulaimaan, A.E.M. (2019) Linear Code Originates from Complete Arc by En-gineering Building Methods. Applied Mathematical Science, 13, 1013-1020. https://doi.org/10.12988/ams.2019.99132
[10]	Yahya, N.Y.K. (2012) Existence of Minimal Blocking Sets of Size 31 in the Projective Plane PG (2, 17). Journal of University of Babylon/Pure and Applied Sciences, 20, 1138-1146.
[11]	Yahya, N.Y.K. (2014) The Use of 7-Blocking Sets in Galois Geometries. Journal of University of Babylon/Pure and Applied Sciences, 22, 1229-1235.
[12]	Yahya, N.Y.K. and Salim, M.N. (2019) 17 New Existences Linear [n,3,d]19 Codes by Geometric Structure Method in PG (2, 19). Rafidain Journal of Computer Sciences and Mathematics, 13, 61-86.
[13]	Kasm, N.Y. and Hamad, A.Z. (2019) Applications of Algebraic Geometry in Cryptography. Modern Applied Science, 13, 130. https://doi.org/10.5539/mas.v13n5p130
[14]	Yahya, N.Y.K. and Salim, M.N. (2018) The Geometric Approach to Existences Linear [n,k,d]13 Code. Interna-tional Journal of Enhanced Research in Science, Technology and Engineer-ing.
[15]	Hirschfeld, J.W.P. (1979) Projective Geometries over Finite Fields. Ox-ford Clarendon Press, New York.
[16]	Kareem, F.F. (2010) Maximum Arc in Projective Plane PG (2, 9) over Galois Field. Journal of College of Education, No. 3, 306-317.