1. Introduction
In present tokamaks operating in high-confinement regimes (H-modes), the steep pressure gradients at edge are often observed to relax through frequent intermittent discharges of energy, known as ELMs. The physics of ELMs is a key issue for ITER operation. The onset of ELMs constrains the pressure at top of edge transport barrier (pedestal height). The ELMs events transport substantial heat and particle loads to plasma-facing materials. A predictive understanding of the onset of type-I ELMs has been gained via the development of peeling-ballooning modes [1] in which EL Ms are triggered by instabilities driven by the large pressure gradient and bootstrap current in the edge. High pressure is important for fusion efficiency. The bootstrap current can be changed.
The perpendicular heating in cyclotron waves tends to pile up the resonant particles toward the low magnetic field side. An electrostatic field may result [2] . Variations of the electrostatic potential at plasma edge are observed in HL
-2A
[3] . Full particle simulation is performed using the Boris algorithm [4] . The electrostatic field can make circulating particles trapped or make trapped particles circulating depending on the field direction. With the assumption neoclassical transport the population of the trapped particles and bootstrap current change accordantly. Modulating bootstrao current by changing the electrostatic field, mitigation of type-1 ELM is possible. The electrostatic potential needed for the mitigation is quantitatively calculated. Experiments by either ECRH or biasing [5] are being prepared to verify the theory.
2. Full Particle Orbit Simulation in Tokamaks
In particle simulations of magnetized plasmas, the Boris algorithm [4] is the standard for advancing a charged particle in an electromagnetic field in accordance with the equation of motion associated with the Lorentz force,
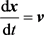 (1)
(1)
 (2)
(2)
where the magnetic field and electric field are given respectively by
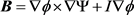 (3)
(3)
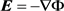 (4)
(4)
where
is the poloidal magnetic flux,
is the electrostatic potential. We proceed from Solov’ev solution
 (5)
(5)
where
, e is elongation, Q is related to tri-angularity.
We use ITER’s parameters: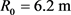 ,
,
, toroidal current
, aspect ratio
. So the tokamak magnetic field is well-determined. Full orbit simulations find electric trapping and de-trapping seen in Figure 1 and Figure 2 respectively.
,
,
, toroidal current
, aspect ratio
. So the tokamak magnetic field is well-determined. Full orbit simulations find electric trapping and de-trapping seen in Figure 1 and Figure 2 respectively.
![]()
Figure 1. (a) The electrostatic field E is zero, particle is circulating; (b) The electrostatic field E is 35 kv/m, particle is trapped. Ion energy is 60 kev, pitch angle is 72˚.
![]()
Figure 2. (a) The electrostatic field E is zero, particle is trapped; (b) The electrostatic field E is −35 kv/m, particle is turned to be circulating which is called as de-trapping. Ion energy is 60 kev, pitch angle 151˚.
Full particle orbit simulation is suitable to a multi-scale problem. The Boris algorithm [4] makes simulation in the long time simulation accurate.
3. Bootstrap Current
The gyro-averaged Hamiltonian has been given in Ref. [6] ,
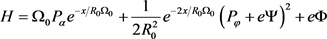 (6)
(6)
where the momenta
 (7)
(7)
(8)
 (9)
(9)
are conjugate to
, the gyrophase, φ, the toroidal angle, and x, expressed as
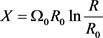 (10)
(10)
where R and Z are the coordinates of the guiding center in a cylindrical system, ρ is the Larmor radius, Ω is the toroidal gyro-frequency. The particle mass is taken to be unity for simplicity. The electrostatic potential is assumed in a form,
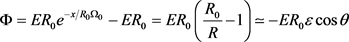 (11)
(11)
which is like the dipole potential produced by two close-point-charges, where
is the inverse aspect ratio.
From Equation (6) we have
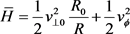 (12)
(12)
where
,
. For the large aspect-ratio approximation we have,
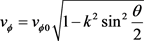 (13)
(13)
where
. For the trapped particles
and the bounce frequency is
 (14)
(14)
The ions with
are trapped, however, they are circulating without the electrostatic field. That is electrostatic trapping. For electrons the trapping condition is
 (15)
(15)
There is minimum of
for trapping. If equilibrium distribution-function is Maxwellian it is easy to calculate trapped-electron population. The fraction of trapped electrons is
. Comparing with neoclassical transport [7] which increase by a factor
. And the bootstrap current changes accordantly [8] ,
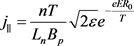 (16)
(16)
where
is the poloidal magnetic field, n is the density, T is plasma temperature,
is the density scale length. The gradients in the electron profiles contribute to typically 70% - 90% of the total bootstrap current [9] .
4. Peeling-Ballooning Modes
The criterion of peeling-ballooning modes can be expressed by the following formula [10] ,
![]() (17)
(17)
where
is the Mercier coefficient,
is the Mercier stability criterion, finite (positive) bootstrap current,
, is destabilizing and
is the derivative of the safety factor with respect to the poloidal magnetic flux. At pedestal the temperature is low, therefore, from Equation (16) bootstrap current is sensitive to the electrostatic potential.
Now we use Equation (17) to calculate the criterion. For a large aspect ratio and low
ordering Equation (17) can be written [1]
(18)
where
and e is the elongation [11] . We neglect triangularity,
, then Equation (17) becomes
(19)
If
we have the criterion for stability
(20)
which can be produced in the practical experiments [5] .
Electrostatic field, hopefully, can realize ELM-control like that in Ref. [12] and show synchronization of the ELM cycle with added electrostatic field. Electrostatic field, hopefully, can realize ELM-ree discharge which appears in I-mode of Alcator C-mod [13] .
5. Summary
Full particle simulation is suitable to a multi-scale problem. The Boris algorithm makes long-time simulation accurate. The perpendicular heating in cyclotron waves tends to pile up the resonant particles toward the low magnetic field side in which electrostatic field may result [2] . The electrostatic field can make circulating particles trapped or make trapped particles circulating depending on the field direction. The trapped-particle population and bootstrap current change accordingly in the process. Modulating bootstrap current, mitigation of type-1 ELM or ELM-free discharge is possible. Experiments by either ECRH or biasing [5] are being prepared to verify the theory in HL
-2A
Tokamak [14] .
Acknowledgements
Helpful discussions with Prof. S. Q. Liu, Dr. Y. Liu and Dr. X. S, Yang are greatly appreciated. This work is supported by the National Natural Science Foundation for Young Scientists of China (Grant No. 11605143), Chinese National Science Foundation (Nos. 11261140327, 11005035, 11205053, 11575055). National Key R&D Program of China under 2017YFE0300405.