Superheterodyne Amplification for Increase the Working Frequency ()
1. Introduction
Very high frequencies including gigahertz range are very promising for building active devices [1] [2] . This article is devoted to idea to use crystal GaAs like very wide material for obtain of high frequency of very simple method in monolithic material without of difficult nanostructure design which usually destroyed diffusion processes.
There are different effects of nonlinear interaction of electromagnetic waves which caused the increase of frequency. More frequently it is using the terminus superheterodyne mechanism for increasing the frequencies. It is useful to show how works all mechanisms on simplest case with analytical demonstration of superheterodyne amplification in case of GaAs thanks parametric connection of two transversal waves and charge wave. It is necessary to explain the role of space charge wave. Amplification of traveling space charge waves (SCW) of the microwave range in n-GaAs has been under investigations for many years [1] . When bias electric fields are higher than the critical value for observing negative differential conductivity (NDC), space charge waves have the possibility to take energy due to negative differential mobility. But the critical value of bias electric field in GaAs is 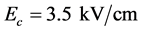 that limits the maximum values of space charge waves. Also, the frequency range of amplification of SCW in GaAs films is
that limits the maximum values of space charge waves. Also, the frequency range of amplification of SCW in GaAs films is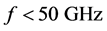 . At frequencies
. At frequencies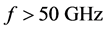 , it is better to use a new type of interaction. It is necessary to use the connection of space charge waves with electromagnetic one. This connection caused to study nonlinear interaction between space charge waves and electromagnetic one. First of all it is necessary to discuss a new type of interaction named superheyterodyne one, which analyzed very carefully in this article. In any case the negative mobility of crystal is very important for all our simulation of nonlinear interaction for different cases. The superheterodyne amplification is thanks going of the energy of crystal with battery and with current to energy of electromagnetic wave of high frequency in case of connection of electromagnetic and space charge waves.
, it is better to use a new type of interaction. It is necessary to use the connection of space charge waves with electromagnetic one. This connection caused to study nonlinear interaction between space charge waves and electromagnetic one. First of all it is necessary to discuss a new type of interaction named superheyterodyne one, which analyzed very carefully in this article. In any case the negative mobility of crystal is very important for all our simulation of nonlinear interaction for different cases. The superheterodyne amplification is thanks going of the energy of crystal with battery and with current to energy of electromagnetic wave of high frequency in case of connection of electromagnetic and space charge waves.
In order to obtain a negative differential mobility (NDM), the bias electric field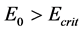 , which is different in different crystals.
, which is different in different crystals.
2. Idea and Model
Let’s consider el crystal GaAs In order to obtain a negative differential mobility (NDM) in GaAs, the bias electric field 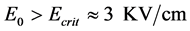 should be [1] [2] .
should be [1] [2] .
There are the two electromagnetic waves of THz range with frequencies and wave numbers 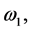
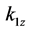 ,
, 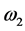 ,
,  with opposite directions of propagation and space charge wave with
with opposite directions of propagation and space charge wave with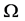 ,
, 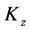 of the microwave range. The frequencies and wave numbers are connected by coupling conditions
of the microwave range. The frequencies and wave numbers are connected by coupling conditions
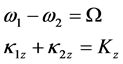 (1)
(1)
The wave synchronism is realized at the frequencies:
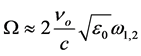 (2)
(2)
where![]() , c are the electron and light velocities,
, c are the electron and light velocities, ![]() is dielectric permittivity of GaAs. Let’s explain simplest case of interaction in the one dimensional model
is dielectric permittivity of GaAs. Let’s explain simplest case of interaction in the one dimensional model
![]() for analysis of Maxwell’s equations (in absolute units):
for analysis of Maxwell’s equations (in absolute units):
![]() (3)
(3)
and hydrodynamic equation of the motion for the electrons
![]() (4)
(4)
where ![]() and
and ![]() are the electron charge and mass,
are the electron charge and mass, ![]() is the collision frequency,
is the collision frequency, ![]() is the electron’s concentration. For electromagnetic waves the effective dielectric constant is
is the electron’s concentration. For electromagnetic waves the effective dielectric constant is
![]()
with plasma frequency![]() . The interaction of longitudinal wave
. The interaction of longitudinal wave
(![]() ) with transversal waves (
) with transversal waves (![]() ) is possible thanks nonlinear connection between waves. In the case of longitudinal wave (
) is possible thanks nonlinear connection between waves. In the case of longitudinal wave (![]() ) it is necessary use (3), and (4) in the case of frequency
) it is necessary use (3), and (4) in the case of frequency ![]() taking into account the differential negative mobility of electrons
taking into account the differential negative mobility of electrons
![]() (5)
(5)
It is necessary to use![]() ,
,
where![]() ,
, ![]() are the velocity electrons and diffe-
are the velocity electrons and diffe-
rential mobility ![]() of electrons, which is negative in case if the field is more the critical field
of electrons, which is negative in case if the field is more the critical field ![]() for GaAs. It is possible to take in calculations
for GaAs. It is possible to take in calculations ![]() like mobility in first valley of GaAs and
like mobility in first valley of GaAs and ![]() is initial concentration of electrons. The variable current
is initial concentration of electrons. The variable current ![]() is determined from (5)
is determined from (5)
![]() (6)
(6)
where ![]()
![]() is diffusion coefficient. Using (6) and (3) it is possible
is diffusion coefficient. Using (6) and (3) it is possible
obtain the equation for longitudinal variable wave
![]() (7)
(7)
where the right part is calculated like resonance part of longitudinal part,
![]() , is the relaxation frequency,
, is the relaxation frequency, ![]() is differential conduc-
is differential conduc-
tivity negative if field is more critical.
For transverse waves (![]() ) from (3) and (4) it is possible obtain the wave equation
) from (3) and (4) it is possible obtain the wave equation
![]() (8)
(8)
The right parts of (7) and (8) describe the nonlinear interaction of waves which is resonance (1) with respect to the wave equation.
3. Nonlinear Equations for Waves
The nonlinear interactions of waves are with longitudinal wave and transversal electromagnetic waves
![]() (9)
(9)
where![]() . The waves satisfy the parametric conditions (1).
. The waves satisfy the parametric conditions (1).
The nonlinear right parts in (7) and (8) were calculated using Bloembergen’s method [3] :
![]() (10)
(10)
where![]() . From (8) we have two nonlinear equations for transverse waves:
. From (8) we have two nonlinear equations for transverse waves:
![]() (11a)
(11a)
![]() (11b)
(11b)
where ![]() are group’s velocities of electromagnetic waves,
are group’s velocities of electromagnetic waves,
![]()
![]() and
and![]() . From (7) it is possible to ob-
. From (7) it is possible to ob-
tain the nonlinear equation for longitudinal wave
![]() (11c)
(11c)
4. Calculation of Superheterodyne Amplification
We analyze amplification by means of using constant pumping wave ![]()
![]() and negative differential mobility
and negative differential mobility![]() . It is necessary use the equa-
. It is necessary use the equa-
tions
![]() (12)
(12)
and the conditions![]() , where
, where ![]() is the diffusion frequency,
is the diffusion frequency, ![]() ,
, ![]() From (12a) and (12b) it follow the
From (12a) and (12b) it follow the
decisions ![]()
For ![]() it is possible obtain the equation
it is possible obtain the equation
![]()
And
![]()
The superheterodyne amplification is characterized by coefficients ![]() and
and![]() :
:
![]() (13)
(13)
If we have negative differential mobility ![]() and
and ![]() from (13) it is follow optimal for amplification length L of crystal:
from (13) it is follow optimal for amplification length L of crystal:
![]()
where![]() . For the next parameters of GaAs the initial volume con-
. For the next parameters of GaAs the initial volume con-
centration of electrons![]() , the collision frequency
, the collision frequency![]() , the velocity
, the velocity![]() ,
, ![]() , absence of domain, the frequencies
, absence of domain, the frequencies
![]() in THz range and
in THz range and ![]() in the microwave range,
in the microwave range,
the plasma frequency![]() ,
, ![]() , where mo is the mass of electron,
, where mo is the mass of electron, ![]() , the pumping intensity is
, the pumping intensity is![]() ,
,
![]() ,
, ![]() and on frequency
and on frequency ![]() GHz the superheterodyne amplification of about
GHz the superheterodyne amplification of about![]() . This amplification is significant
. This amplification is significant
for the case of superheterodyne interaction.
5. Simulation of Amplification in Gaas Films
The amplification is more perspective for integrated system so it is necessary to analyze amplification and multiplication for obtain increase of frequencies in GaAs films with space charge waves. It is possible by means of computer simulation and consideration of the parametric interaction of space charge waves with matching conditions is realized:
![]() . (14)
. (14)
The electron velocity in GaAs is the nonlinear function of an external electric field [4] [5] . The coordinate system is chosen as follows: X-axis is directed perpendicularly to the plane of film, the drift bias field is applied along Z one, exciting and receiving antennas are parallel to Y-axis. The sizes of the film are![]() ,
,![]() . In our model, it is considered 2D model of the electron gas in GaAs so as our consideration described the epitaxial film n-GaAs and i-GaAs substrate. Thus, 2D electron concentration is present only in the plane
. In our model, it is considered 2D model of the electron gas in GaAs so as our consideration described the epitaxial film n-GaAs and i-GaAs substrate. Thus, 2D electron concentration is present only in the plane ![]() (epitaxial n-GaAs), and an influence of transverse motion of carriers on space charge wave dynamics is neglected. Consider space charge waves having phase velocity equal to velocity of electrons drift equal to
(epitaxial n-GaAs), and an influence of transverse motion of carriers on space charge wave dynamics is neglected. Consider space charge waves having phase velocity equal to velocity of electrons drift equal to![]() ,
, ![]() , where
, where ![]() is the constant difference of potential, creating the bias electric field
is the constant difference of potential, creating the bias electric field ![]() in GaAs film. The following system for description of nonlinear space charge waves in quasi-stationary approximation is used:
in GaAs film. The following system for description of nonlinear space charge waves in quasi-stationary approximation is used:
![]() (15)
(15)
![]() (16)
(16)
The Equations (15), (16) are added by boundary conditions:
![]()
Here ![]() where
where ![]() is constant two-dimensional electron concentration,
is constant two-dimensional electron concentration, ![]() is its varying part of concentration, D is the diffusion coefficient which weakly depends on a drift field, e is the electron charge (the signs are changed for the positive charge);
is its varying part of concentration, D is the diffusion coefficient which weakly depends on a drift field, e is the electron charge (the signs are changed for the positive charge); ![]() is unit vector along direction of axis Z, and
is unit vector along direction of axis Z, and ![]() is the lattice dielectric permittivity of GaAs. A dependence of Z-component of electron velocity
is the lattice dielectric permittivity of GaAs. A dependence of Z-component of electron velocity ![]() is presented in a Figure 1. In the input antenna (
is presented in a Figure 1. In the input antenna (![]() ), the signal of the longitudinal electric field is present
), the signal of the longitudinal electric field is present ![]() at two separate microwave frequencies
at two separate microwave frequencies![]() ;
; ![]() are half-widths of input pulse. This signal excites space charge waves in 2D electron gas. First of all, amplification at the frequencies
are half-widths of input pulse. This signal excites space charge waves in 2D electron gas. First of all, amplification at the frequencies ![]() takes place in the case of negative differential mobility, NDM. Also, the parametric interaction of waves with matching conditions is realized like (14).
takes place in the case of negative differential mobility, NDM. Also, the parametric interaction of waves with matching conditions is realized like (14).
The output antenna is in![]() . The problem is to get the optimal conditions for releasing the output signal at the sum frequency in films of finite sizes.
. The problem is to get the optimal conditions for releasing the output signal at the sum frequency in films of finite sizes.
For a small monochromatic input signal (![]() ) in an unbounded film, it is possible to get the expression for the linear increment of temporal growth
) in an unbounded film, it is possible to get the expression for the linear increment of temporal growth![]() :
:
![]() (17)
(17)
In the case of NDM (![]() ), an instability takes place:
), an instability takes place: ![]() in a certain frequency range. A dependence of increment
in a certain frequency range. A dependence of increment ![]() on carrier frequency
on carrier frequency ![]() is presented in a Figure 2. It is seen that there exists the maximal value at an optimal frequency. A comparison of the value of increment obtained in local field approximation used here with calculation within the more general non-local Shur’s model [4] , [5] shows that under
is presented in a Figure 2. It is seen that there exists the maximal value at an optimal frequency. A comparison of the value of increment obtained in local field approximation used here with calculation within the more general non-local Shur’s model [4] , [5] shows that under![]() , the local field approximation is valid for
, the local field approximation is valid for![]() . Therefore, the sum frequency should be chosen within this range.
. Therefore, the sum frequency should be chosen within this range.
Simulations of wave mixing in GaAs films demonstrate a possibility to get the
![]()
Figure 1. Dependence ![]() (105 m/s, 105 V/m).
(105 m/s, 105 V/m).
signal at sum frequency under a wide range of amplitudes of input signals. The spectral distributions of output signal ![]() in films with different input antenna widths are presented in Figure 3 and Figure 4. The parameters are: 2D electron concentration is
in films with different input antenna widths are presented in Figure 3 and Figure 4. The parameters are: 2D electron concentration is![]() ,
, ![]() ,
,![]() ;
;![]() ,
, ![]() ,
,![]() ;
;![]() ;
; ![]() (Figure 3);
(Figure 3); ![]() (Figure 4). The input pulse duration is
(Figure 4). The input pulse duration is![]() ; input frequencies are
; input frequencies are![]() ,
,![]() ;
;![]() ,
, ![]() ,
,![]() . The output amplitudes of waves are ~
. The output amplitudes of waves are ~![]() . In the case of wider input antenna, the spectral line intensity corresponding to sum frequency is greater but the additional background spectrum is present. This can be explained in the following manner. The main obstacle for observation of wave mixing is a transition of instability into an essentially nonlinear regime, where a lot of extraneous spectral components are present. For a wider input antenna, such a transition takes place earlier. Thus, there exists the optimal width of the input antenna for observing wave mixing.
. In the case of wider input antenna, the spectral line intensity corresponding to sum frequency is greater but the additional background spectrum is present. This can be explained in the following manner. The main obstacle for observation of wave mixing is a transition of instability into an essentially nonlinear regime, where a lot of extraneous spectral components are present. For a wider input antenna, such a transition takes place earlier. Thus, there exists the optimal width of the input antenna for observing wave mixing.
![]()
Figure 2. Dependence of increment ![]() on a frequency (1010 s−1).
on a frequency (1010 s−1).
![]()
Figure 3. Fourier spectrum of ![]() (relative units) in the output antenna.
(relative units) in the output antenna.
![]()
Figure 4. Fourier spectrum in the output for wider input antenna.
A comparison of simulations with experiment on wave mixing in GaAs films [6] shows that there is a coincidence on the frequency interval and possible levels of input signals.
6. Conclusions
It is shown that superheterodyne amplification is realized by negative differential mobility in GaAs and nonlinear interaction two transversal and longitudinal waves. The pumping wave of very small amplitude helps to move the energy of battery to microwave. Usually level of noise is low in high of frequency and absent of domains. The advantage to use of transversal waves is in moving very easy from crystal. The pumping wave may be very low amplitude. The value of amplification is very big on the length ![]() without exciting of domain. The condition for exciting domain
without exciting of domain. The condition for exciting domain ![]() is not fulfilled. This mechanism of amplification is very promising in millimeter and submillimeter ranges. In these ranges it is absent good amplifiers ranges.
is not fulfilled. This mechanism of amplification is very promising in millimeter and submillimeter ranges. In these ranges it is absent good amplifiers ranges.
The mechanism of the mixing and the multiplication is a transition of instability into an essentially nonlinear regime. There exists the optimal width of input antenna for observing wave parametric and multiplication effects.
Comparison of simulations with experiment on wave mixing in GaAs films is to show a coincidence on frequency interval and possible levels of input signals.
It is possible future work to investigate some other crystal having negative differential mobility which it is realized now. For another hand it is possible to use the strongly nonlinear material like ![]() that is done [7] [8] [9] , and the results of this investigations are successful. Only it is one problem, from different materials including
that is done [7] [8] [9] , and the results of this investigations are successful. Only it is one problem, from different materials including![]() , it is necessary to use temperature of liquid nitrogen N. It is possible to use another method to increase the frequency using periodical systems and graphene [10] [11] .
, it is necessary to use temperature of liquid nitrogen N. It is possible to use another method to increase the frequency using periodical systems and graphene [10] [11] .