Numerical Study of 2-D Natural Convection in a Square Porous Cavity: Effect of Three Mode Heating ()
1. Introduction
The natural convection in confined porous media saturated by fluid is fundamental in the fields of engineering and physics. This interest arises from the importance of this heat transfer mode in various engineering fields such as storage of the thermal energy, solar energy collectors, thermal design for the buildings, cooling of electronic components (Alves and Altemani [1] , Kuznetsov and Sheremet [2] , Sheremet and Pop [3] ), the underground spread of pollutants (Bagchi and Kulacki [4] ). The literature on the convective flow in porous media is abundant. An excellent review of most of these studies is in the books (Nield and Bejan [5] , Pop and Ingham. [6] , Vafai [7] , Ingham and Pop [8] ) that give a complete overview of the current state of research in this area. However, most of these studies, theorical and experimental, on side convection, consider boundary conditions thermal constant (temperature or flux constant heat). Excellent review articles including one written by Baytas and Pop [9] , give detailed accounts results obtained with these boundaries conditions. However, these boundary conditions do not reflect what is encountered in many practical situations where the temperature gradient is a function of time. This is the case of electronic components that dissipate power intermittently during operation on/of. Mastering the gradient behavior heat in these real situations can be used to control the flow convective. For example, it may be used to control the quality and structure of a solid resulting from solidification of an alloy by influencing the process of transport. The effect of the modulation of temperature on natural convection in cavity fluid medium was several time studied. For example, Schaladow et al. [10] studied the case of a cavity subjected to a temperature which increases linearly each time. Both considered simulations (numerical and experimental) show that the flow and temperature field are very little affected by these boundary conditions. For their part, Kazmierczak and Chinoda [11] have numerically studied the effect of temperature varying sinusoidally in time on fluid flow and heat transfer in a square cavity. These authors showed that the heat transfer means in time is substantially insensitive to the periodic change of the wall temperature. Lage and Bejan [12] , studied enclosures with one sidewall heated using a pulsating heat flux and the other sidewall cooled at constant temperature. They showed that at high Rayleigh numbers, the buoyancy-driven flow has the tendency to resonate to the periodic heating that has been supplied from the side. Lage et al. [13] , have studied the phenomenon of flow interference caused by discrete, solid objects placed inside fluid saturated enclosures under natural con- vection. Their analysis confirms and quantifies the predominance of the horizontal interference when the enclosure becomes shallow ( ). Seyf and Rassoulinejad-Mousavi [14] proposed a new analytical solution for 2D Darcy-Brink- man equations in porous channels filled with porous media subjected to various boundary conditions at walls. The obtained ODE is solved analytically using homotopy perturbation method. It was shown that there is an excellent agreement between the presented models and the results of the CFD and previous works. Rassoulinejad-Mousavi and Yaghoobi [15] studied the effect of form drag term on the viscous dissipation through a parallel plate channel packed with a porous medium with isoflux or isothermal condition at the walls. Abourida et al. [16] , have examined the effect of imposed sinusoidal temperatures and the thermophysical ones on the fluid flow and heat transfer within the cavity. They showed that all the obtained solutions concerning the fluid flow are periodic, with a period identical to that imposed to the variable temperatures. Note that many of these works consider cavities in which the temperature of cold wall is maintained constant. However, very little work is done on natural convection in a cavity filled with a porous medium with boundary conditions thermal periodic in time and using the Darcy model. Some of the documents dealing with this problem are, Saeid [17] , Malomar et al. [18] . The main objective of the present study is to contribute to the enrichment of the kind of problem by examining the effect of the imposed sinusoidal temperatures parameter (frequency) on the structure of the flow and transfer thermal. We study numerically natural convection unsteady in a square cavity, filled with a porous medium and the vertical walls are subjected at least to a temperature varying sinusoidally with time. The horizontal walls are thermally adiabatic and Darcy model was used.
). Seyf and Rassoulinejad-Mousavi [14] proposed a new analytical solution for 2D Darcy-Brink- man equations in porous channels filled with porous media subjected to various boundary conditions at walls. The obtained ODE is solved analytically using homotopy perturbation method. It was shown that there is an excellent agreement between the presented models and the results of the CFD and previous works. Rassoulinejad-Mousavi and Yaghoobi [15] studied the effect of form drag term on the viscous dissipation through a parallel plate channel packed with a porous medium with isoflux or isothermal condition at the walls. Abourida et al. [16] , have examined the effect of imposed sinusoidal temperatures and the thermophysical ones on the fluid flow and heat transfer within the cavity. They showed that all the obtained solutions concerning the fluid flow are periodic, with a period identical to that imposed to the variable temperatures. Note that many of these works consider cavities in which the temperature of cold wall is maintained constant. However, very little work is done on natural convection in a cavity filled with a porous medium with boundary conditions thermal periodic in time and using the Darcy model. Some of the documents dealing with this problem are, Saeid [17] , Malomar et al. [18] . The main objective of the present study is to contribute to the enrichment of the kind of problem by examining the effect of the imposed sinusoidal temperatures parameter (frequency) on the structure of the flow and transfer thermal. We study numerically natural convection unsteady in a square cavity, filled with a porous medium and the vertical walls are subjected at least to a temperature varying sinusoidally with time. The horizontal walls are thermally adiabatic and Darcy model was used.
2. Materials and Methods
2.1. Basic Equations
A schematic geometry of the problem is shown in Figure 1, where  and
and 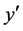 are the cartesian coordinates and
are the cartesian coordinates and 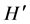 is the size of the walls. This is a square porous cavity two-dimensional and the horizontal walls are assumed to be ther- mally adiabatic. All walls of the cavity are assumed to be impermeable. At the same, the vertical walls, left wall (hot
is the size of the walls. This is a square porous cavity two-dimensional and the horizontal walls are assumed to be ther- mally adiabatic. All walls of the cavity are assumed to be impermeable. At the same, the vertical walls, left wall (hot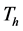 ) and right wall (cold
) and right wall (cold ) are subjected at least to a temperature varying sinusoidally with time such that:
) are subjected at least to a temperature varying sinusoidally with time such that:
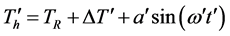 (1)
(1)
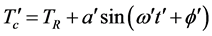 (2)
(2)
where  is the reference temperature (in our study
is the reference temperature (in our study ),
), 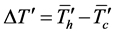 is the average temperature difference,
is the average temperature difference, ![]() represents the amplitude of the
represents the amplitude of the
![]()
Figure 1. Schematic diagram of the physical model and co- ordinate system.
modulation, ![]() the phase angle and
the phase angle and ![]() the modulation frequency. The fol- lowing three cases are considered: 1) only the left wall’s temperature is mo- dulated, the right wall is held at constant temperature; 2) the temperatures modulation are out-of-phase, i.e.
the modulation frequency. The fol- lowing three cases are considered: 1) only the left wall’s temperature is mo- dulated, the right wall is held at constant temperature; 2) the temperatures modulation are out-of-phase, i.e.![]() ; 3) the temperatures modulation are in-phase, i.e.
; 3) the temperatures modulation are in-phase, i.e.![]() . The Darcy-Boussinesq approximation is employed. Iso- tropy, homogeneity and local thermal equilibrium in the porous medium are assumed. Under these assumptions, the equations governing the problem is the equation continuity, the equation of motion and the energy equation, res- pectively (see Nield and Bejan [5] ):
. The Darcy-Boussinesq approximation is employed. Iso- tropy, homogeneity and local thermal equilibrium in the porous medium are assumed. Under these assumptions, the equations governing the problem is the equation continuity, the equation of motion and the energy equation, res- pectively (see Nield and Bejan [5] ):
![]() (3)
(3)
![]() (4)
(4)
![]() (5)
(5)
where ![]() and
and ![]() are the velocity components along
are the velocity components along ![]() and
and![]() ,
, ![]() is the fluid temperature,
is the fluid temperature, ![]() is the time,
is the time, ![]() is the coefficient of thermal expansion. The quantities
is the coefficient of thermal expansion. The quantities ![]() and
and ![]() are defined by
are defined by ![]() and
and ![]() where
where ![]() is the thermal conductivity (solid phase + fluid phase).
is the thermal conductivity (solid phase + fluid phase).
Equations (3)-(5) are subject to the following boundary conditions and initial:
![]() (6)
(6)
One can introduce a stream function ![]() defined by
defined by ![]() and
and
![]() so that Equation (3) is satisfied. Then the governing Equations (3)-(5) can be rewritten with the following dimensionless variables:
so that Equation (3) is satisfied. Then the governing Equations (3)-(5) can be rewritten with the following dimensionless variables:
![]() (7)
(7)
![]() represents the dimensional period and is connected to the modulation fre- quency dimensional by the following equation
represents the dimensional period and is connected to the modulation fre- quency dimensional by the following equation![]() .
.
Substituting (7) in (3)-(5) we obtain the following dimensionless governing equations:
![]() (8)
(8)
![]() (9)
(9)
associated with initial and boundary conditions
![]() (10)
(10)
where ![]() et
et ![]() and
and ![]() represents the Rayleigh number.
represents the Rayleigh number.
At each time t the average Nusselt numbers at the vertical walls (hot and cold) are defined by, respectively:
![]() (11)
(11)
2.2. Numerical Method
The equations of motion (8) and energy (9) associated with the boundary con- ditions (10) are discretized by a finite difference scheme, centred and accurate to the second order. The energy equation is then solved by the implicit method of alternating directions (ADI). The linear discretized equations were solved by Thomas algorithm. For equation of motion, the obtained linear discretized equation was solved by the sucessive over-relaxation method. Uniform grids have been selected in both the ![]() and
and ![]() direction. We have developed a nu- merical code with Fortran 95.
direction. We have developed a nu- merical code with Fortran 95.
The calculation stops when between two time steps, the following condition is satisfied by the stream function:
![]() (12)
(12)
Preliminary tests on the influence of the mesh have allowed us to retain a uniform mesh size of 120*120. The time step used is![]() . The present nu- merical code have been validated against the works of Walker and Homsy [19] , Bejan [20] , Beckerman et al. [21] , Moya et al. [22] ; Manole and Lage [23] , Baytas and Pop [9] for the steady state natural convection in a square porous cavity with isothermal vertical and adiabatic horizontal walls. Table 1 shows the values of the average Nusselt number computed for various Rayleigh numbers in the range
. The present nu- merical code have been validated against the works of Walker and Homsy [19] , Bejan [20] , Beckerman et al. [21] , Moya et al. [22] ; Manole and Lage [23] , Baytas and Pop [9] for the steady state natural convection in a square porous cavity with isothermal vertical and adiabatic horizontal walls. Table 1 shows the values of the average Nusselt number computed for various Rayleigh numbers in the range ![]() in comparison with other authors.
in comparison with other authors.
Note that all numerical simulations are initialized by considering a conductive state and constant heating conditions. When steady regime is established, we introduce the excitatory temperatures and expecte the establishment of a periodic regime.
3. Results and Discussion
3.1. Influence of the Modulation Frequency
To highlight the effect of ![]() frequency modulation, we present the temporal evolution of the functions
frequency modulation, we present the temporal evolution of the functions![]() ,
, ![]() ,
, ![]() and
and ![]() for the three types of oscillatory heating under the following conditions
for the three types of oscillatory heating under the following conditions![]() ;
; ![]() and
and
![]() . Note that Figure 2(a), Figure 3(a) and Figure 4(a) show the temporal evolutions of
. Note that Figure 2(a), Figure 3(a) and Figure 4(a) show the temporal evolutions of ![]() and
and ![]() for
for![]() .
.
Thus Figures 2(b)-(e) respectively show the temporal evolution of the
![]()
Table 1. Comparison of the average Nusselt number of the hot wall.
functions![]() ,
, ![]() ,
, ![]() and
and ![]() in the case where only the temperature of the hot wall (
in the case where only the temperature of the hot wall (![]() ) is modulated. The curves of Figures 2(b)-(e) show that all the solutions obtained are periodic, of periods equal to those of the excitation imposed temperature. In the frequency range
) is modulated. The curves of Figures 2(b)-(e) show that all the solutions obtained are periodic, of periods equal to those of the excitation imposed temperature. In the frequency range![]() , the amplitudes of functions
, the amplitudes of functions![]() ,
, ![]() and
and ![]() remain very close while those of
remain very close while those of ![]() in- creases when
in- creases when ![]() increases. We also note that the nature of sinusoidal oscil- lations is kept in
increases. We also note that the nature of sinusoidal oscil- lations is kept in![]() . Figure 2(e) shows that for
. Figure 2(e) shows that for![]() ,
, ![]() takes nega- tive values during a brief portion of the cycle.
takes nega- tive values during a brief portion of the cycle.
In the case where the temperatures excitatory evolve in phase opposition, Figures 3(b)-(e) show that all the solutions obtained are periodic, periods equal to those the imposed excitatory temperatures. In the frequency range![]() , the amplitudes of the functions presented remain very close except for
, the amplitudes of the functions presented remain very close except for ![]() where there is a notable decrease in the amplitude when
where there is a notable decrease in the amplitude when ![]() go of 20 to 30. For this heating mode is also observed better competition between cells rotating in clockwise direction and cells rotating in counterclockwise direction, resulting in a cancellation of
go of 20 to 30. For this heating mode is also observed better competition between cells rotating in clockwise direction and cells rotating in counterclockwise direction, resulting in a cancellation of ![]() during brief part of the cycle. During this time interval, the flow is monocellular, characterized by a presence of cell rotating in a clockwise
during brief part of the cycle. During this time interval, the flow is monocellular, characterized by a presence of cell rotating in a clockwise
direction and of which intensity decreases when ![]() tends to 0. Unlike the case where only the temperature of the hot wall is variable, the sinusoidal nature of the excitation is not conserved for the different functions. Figures 3(d)-(e) show that during part of the cycle, the average Nusselt numbers
tends to 0. Unlike the case where only the temperature of the hot wall is variable, the sinusoidal nature of the excitation is not conserved for the different functions. Figures 3(d)-(e) show that during part of the cycle, the average Nusselt numbers ![]() and
and ![]() take negative values. These negative values found, are justified by the fact that for amplitudes (
take negative values. These negative values found, are justified by the fact that for amplitudes (![]() ) the cold wall acquires temperatures higher than the wall supposed to be hot. This then results in a transfer of heat from the cold wall inwardly of the cavity as soon as this wall becomes hotter than the surrounding fluid. Thereafter the fluid surrounding the hot wall becomes hotter than this wall it results a heat transfer from the fluid outwardly the cavity via the hot wall.
) the cold wall acquires temperatures higher than the wall supposed to be hot. This then results in a transfer of heat from the cold wall inwardly of the cavity as soon as this wall becomes hotter than the surrounding fluid. Thereafter the fluid surrounding the hot wall becomes hotter than this wall it results a heat transfer from the fluid outwardly the cavity via the hot wall.
For the last mode of heating studied where excitatory temperatures are in phase, Figures 4(b)-(e) show that only functions ![]() and
and ![]() retain the sinusoidal look and the period imposed on excitatory temperatures while
retain the sinusoidal look and the period imposed on excitatory temperatures while ![]() and
and ![]() are periodic, with a period which is half that of excitatory tem- peratures. These figures also show that the amplitudes of functions increase when
are periodic, with a period which is half that of excitatory tem- peratures. These figures also show that the amplitudes of functions increase when ![]() increases except
increases except ![]() where the amplitudes are very close to the range of frequencies considered. However, we note that the most important effects of frequency modulation
where the amplitudes are very close to the range of frequencies considered. However, we note that the most important effects of frequency modulation ![]() are rated for high values of this parameter (
are rated for high values of this parameter (![]() ). But for convenience we chose to study the functions for
). But for convenience we chose to study the functions for ![]() because even for this range frequency, important differences qualitative and quantitative are observed.
because even for this range frequency, important differences qualitative and quantitative are observed.
3.2. Streamlines and Isotherms
To understand the details of flow and heat transfer in the cavity, we produced streamlines and isotherms for each of the three modes of heating, during a flow cycle for![]() ;
; ![]() and
and![]() .
.
Before discussing the results for the oscillatory regime, we produced stream- lines and isotherms in steady state (![]() ) for
) for![]() . Note that in the case of constant heating (
. Note that in the case of constant heating (![]() ), the flow in the cavity remains monocell, consisting of cell rotating in clockwise direction and having a symmetry relative to the center of the cavity (see Figure 5).
), the flow in the cavity remains monocell, consisting of cell rotating in clockwise direction and having a symmetry relative to the center of the cavity (see Figure 5).
In the case where only the hot temperature (![]() ) is modulated, Figures 6(a)-(f) show the streamlines (left) and isotherms (right) at times corresponding to the letters a, b, c, d, e and f in Figure 2. When the temperature of the hot wall is at its maximum (
) is modulated, Figures 6(a)-(f) show the streamlines (left) and isotherms (right) at times corresponding to the letters a, b, c, d, e and f in Figure 2. When the temperature of the hot wall is at its maximum (![]() ) the flow field is dominated by a cell rotating in clockwise direction and occupying the entire cavity (Figure 6(a)). The maxi-
) the flow field is dominated by a cell rotating in clockwise direction and occupying the entire cavity (Figure 6(a)). The maxi-
mum of the absolute value of ![]() (
(![]() ) being located in the neighboring
) being located in the neighboring
of center-left of the cavity and compared to the constant heating case (![]() ), symmetry is broken. The structure of the isotherms show the existence of high thermal gradients in the vicinity of active walls, which leads to the maximum heat transfer and Nusselt numbers at this time (a). It is also observed that when
), symmetry is broken. The structure of the isotherms show the existence of high thermal gradients in the vicinity of active walls, which leads to the maximum heat transfer and Nusselt numbers at this time (a). It is also observed that when
![]() decreases,
decreases, ![]() moves and is located in the neighboring of center-right
moves and is located in the neighboring of center-right
of the cavity (Figure 6(b)). The gradual reduction of ![]() is accompanied by a decrease in the intensity of this cell rotating in clockwise and promotes the appearance of a secondary cell rotating counter-clockwise and localized to the upper left corner of the cavity (Figure 6(c) and Figure 6(d)). This is explained by the existence of a quantity of fluid surrounding the hot wall and having a higher temperature than the hot wall. At the same moment the structure of
is accompanied by a decrease in the intensity of this cell rotating in clockwise and promotes the appearance of a secondary cell rotating counter-clockwise and localized to the upper left corner of the cavity (Figure 6(c) and Figure 6(d)). This is explained by the existence of a quantity of fluid surrounding the hot wall and having a higher temperature than the hot wall. At the same moment the structure of
![]()
Figure 5. Streamlines (left) and isotherms (right) for![]() : Steady state solution.
: Steady state solution.
isotherms show a spacing between isotherms at the hot wall, which results in significant reduction in heat transfer, demonstrated by the average Nusselt numbers are near their minimum values. Once that the temperature of the hot wall again believes in leaving its minimum value, the secondary cell rotating in counterclockwise direction decreases in intensity and eventually disappear in favor of the formation of a cell rotating in clockwise direction, near the hot wall and which is added to the cell rotating in clockwise direction already in the vicinity of the cold wall (Figure 6(e)). The structure of isotherms show that heat transfer are near to minimum values. Beyond the moment (e), it is gradually forms a main cell rotating in clockwise direction and occupying the entire cavity in end of the cycle (Figure 6(f)).
In the case where temperatures evolve in phase opposition, Figures 7(a)-(f) show streamlines (left) and isotherms (right) at times corresponding to the letters a, b, c, d, e and f in Figure 3. We note that at the moment (a) where the hot wall is at its maximum value, ![]() and the cold wall is at its minimum value,
and the cold wall is at its minimum value, ![]() the flow regime is monocell, dominated by one cell rotating in a clockwise direction and occupying the entire cavity. We observe that also at time (a) the structure of isotherms shows stratification of the temperature around the center of the cavity and of high gradients thermal around the active
the flow regime is monocell, dominated by one cell rotating in a clockwise direction and occupying the entire cavity. We observe that also at time (a) the structure of isotherms shows stratification of the temperature around the center of the cavity and of high gradients thermal around the active
walls (Figure 7(a)). Evolving in the cycle, ![]() decreases and
decreases and ![]() increases, there is a weakening of the main cell. This weakening of the main cell is further complicated by the occurrence of two recirculation cells rotating in a counterclockwise direction, one at the upper left corner and the other at the lower right corner (Figure 7(b) and Figure 7(c)). The appearance of these two negative cells cause a spacing of the isotherms in the vicinity of active walls whence a heat transfer reduction. These secondary cells expands along the active walls at the expense of the cell rotating in a clockwise direction and causing a reduction in heat transfer (Figure 7(d)). Continuing in the cycle, the flow will consist of three cells rotating in counterclockwise (Figure 7(e)) and thereafter for a short time, the flow is dominated by one cell rotating in a counterclockwise direction (not shown here). Between moments (d) and (e) structure of isotherms attests that heat transfer are virtually reduced to zero. In the second part of the cycle, the cell rotating in a clockwise direction will play a role similar to the one played by the cell rotating in a counterclockwise direction during the first part of the cycle. However the two recirculation cells rotating in a clockwise direction appear in the corner of level upper right and lower left (Figure 7(f)). Conse- quently, these secondary cells rotating in a clockwise direction expands along the active walls to the detriment of the cell rotating in a counterclockwise direction and thereafter a cycle with a monocellular cell rotating in a clockwise direction starts again.
increases, there is a weakening of the main cell. This weakening of the main cell is further complicated by the occurrence of two recirculation cells rotating in a counterclockwise direction, one at the upper left corner and the other at the lower right corner (Figure 7(b) and Figure 7(c)). The appearance of these two negative cells cause a spacing of the isotherms in the vicinity of active walls whence a heat transfer reduction. These secondary cells expands along the active walls at the expense of the cell rotating in a clockwise direction and causing a reduction in heat transfer (Figure 7(d)). Continuing in the cycle, the flow will consist of three cells rotating in counterclockwise (Figure 7(e)) and thereafter for a short time, the flow is dominated by one cell rotating in a counterclockwise direction (not shown here). Between moments (d) and (e) structure of isotherms attests that heat transfer are virtually reduced to zero. In the second part of the cycle, the cell rotating in a clockwise direction will play a role similar to the one played by the cell rotating in a counterclockwise direction during the first part of the cycle. However the two recirculation cells rotating in a clockwise direction appear in the corner of level upper right and lower left (Figure 7(f)). Conse- quently, these secondary cells rotating in a clockwise direction expands along the active walls to the detriment of the cell rotating in a counterclockwise direction and thereafter a cycle with a monocellular cell rotating in a clockwise direction starts again.
In case where the two temperatures are changing in phase, Figures 8(a)-(l) show the streamlines (left) and isotherms (right) corresponding to the instants a, b, … and l in Figure 4. At time (a), ![]() and
and ![]() are around them maximum, the flow in cavity is monocellular, characterized by the presence of a cell rotating in a clockwise direction (Figure 8(a)). Evolving in the cycle, at end of first half of the flow cycle, we note with decreasing temperatures the appearance of a secondary cell rotating counterclockwise in the upper left corner of the cavity.
are around them maximum, the flow in cavity is monocellular, characterized by the presence of a cell rotating in a clockwise direction (Figure 8(a)). Evolving in the cycle, at end of first half of the flow cycle, we note with decreasing temperatures the appearance of a secondary cell rotating counterclockwise in the upper left corner of the cavity. ![]() is located in the center right of the cavity (Figures 8(b)-(e)). The appearance of this secondary cell is explained by the existence of a quantity of fluid surrounding the hot wall and having a higher temperature than the hot wall. Beyond the moment (e), the secondary cell is weakened (Figure 8(f)) and eventually disappear at the end of flow cycle (Figure 8(g)) when
is located in the center right of the cavity (Figures 8(b)-(e)). The appearance of this secondary cell is explained by the existence of a quantity of fluid surrounding the hot wall and having a higher temperature than the hot wall. Beyond the moment (e), the secondary cell is weakened (Figure 8(f)) and eventually disappear at the end of flow cycle (Figure 8(g)) when ![]() and
and ![]() take minimum values. At time (a) the structure of the isotherms show relatively high thermal gradients around the active walls unlike the moments b, c, d, e and f where it has a spacing of the isotherms at the upper half of the hot wall, which leads the reduction of heat transfer. Figure 8(a) and Figure 8(g) show a similar flow state qualitatively but different from the quantitative standpoint as illustrated by the temporal variations of
take minimum values. At time (a) the structure of the isotherms show relatively high thermal gradients around the active walls unlike the moments b, c, d, e and f where it has a spacing of the isotherms at the upper half of the hot wall, which leads the reduction of heat transfer. Figure 8(a) and Figure 8(g) show a similar flow state qualitatively but different from the quantitative standpoint as illustrated by the temporal variations of ![]() and
and![]() . Continuing to evolve in the cycle,
. Continuing to evolve in the cycle, ![]() and
and ![]() grow again, we see that
grow again, we see that ![]() moves to the left center of the cavity, which leads to creation of a secondary cell (Figures 8(i)-(k)). However the secondary cell that forms and rotating in a counterclockwise is located in the lower right corner. His apparition is due to the existence of a quantity of fluid in the vicinity of the cold wall whose temperature is lower than the cold wall. This secondary cell is weakened (Figure 8(l)) and disappear at the end of flow cycle. We also noticed that flow conditions (Figures 8(g)-(l)) can be obtained from the flow conditions (Figures 8(a)-(f)) respectively, by rotating the cavity in its plan of 180 degree. The structure of isotherms shows a spacing of the isotherms at the lower half of the cold wall.
moves to the left center of the cavity, which leads to creation of a secondary cell (Figures 8(i)-(k)). However the secondary cell that forms and rotating in a counterclockwise is located in the lower right corner. His apparition is due to the existence of a quantity of fluid in the vicinity of the cold wall whose temperature is lower than the cold wall. This secondary cell is weakened (Figure 8(l)) and disappear at the end of flow cycle. We also noticed that flow conditions (Figures 8(g)-(l)) can be obtained from the flow conditions (Figures 8(a)-(f)) respectively, by rotating the cavity in its plan of 180 degree. The structure of isotherms shows a spacing of the isotherms at the lower half of the cold wall.
4. Conclusions
The numerical study of unsteady natural convection in a porous cavity square whose side walls are subjected at least to a temperature varying sinusoidally with time was investigated. The mathematical model used is that of Darcy in the Boussinesq approximation. The algorithm was validated by direct comparison with previously published work and the results were considered in good agree- ment. Streamlines and isotherms were produced for![]() ,
, ![]() et
et ![]() for each of the three mode heating.
for each of the three mode heating.
Based on the results found in this study, we remark that the oscillatory heating causes the appearance of secondary flow, whose amplification depends on the frequency of modulation of the imposed temperature but also of the heating mode. In addition the imposed oscillatory heating improves thermal transfer compared to the constant heating and constitutes the best way to remove the
heat to the outside environment. The evolution of temperatures in phase oppo- sition is the best way to remove the heat to the outside environment. When oscillatory regime is imposed, important differences are noted in terms of flow structure and of transfers heat unlike the case of constant heating; depending on the purpose, these differences can be exploited by the modeller.
Acknowledgements
We thank the Editor and the referee for their comments.
Nomenclature
![]() , Dimensionless amplitude
, Dimensionless amplitude
![]() , Gravity intensity,
, Gravity intensity, ![]()
![]() , Permeability of the porous medium,
, Permeability of the porous medium, ![]()
![]() , Dimensionless time
, Dimensionless time
![]() , Dimensionless temperature
, Dimensionless temperature
![]() , Dimensionless coordinates
, Dimensionless coordinates
![]() , Rayleigh number for porous medium
, Rayleigh number for porous medium
![]() , Average temperature difference,
, Average temperature difference, ![]()
![]() , Average Nusselt number at the cold wall
, Average Nusselt number at the cold wall
![]() , Average Nusselt number at the hot wall
, Average Nusselt number at the hot wall
Greek Symbols
![]() , Effective thermal diffusivity,
, Effective thermal diffusivity, ![]()
![]() , Coefficient of thermal expansion,
, Coefficient of thermal expansion, ![]()
![]() , Dynamic viscosity,
, Dynamic viscosity, ![]()
![]() , Dimensionless phase angle
, Dimensionless phase angle
![]() , Ratio of heat capacity of porous medium to that of fluid
, Ratio of heat capacity of porous medium to that of fluid
![]() , Dimensionless frequency
, Dimensionless frequency
![]() , Dimensionless period
, Dimensionless period
![]() , Dimensionless stream function
, Dimensionless stream function
![]() , Reference density of fluid,
, Reference density of fluid, ![]()
Superscript
('), Dimensional variables
(-), Average values
Subscript
c, Cold
h, Hot
f, Fluid
min, Minimum value
max, Maximum value