Microcalorimetric Study of Acetylcholine and Acetylthiocholine Hydrolysis by Acetylcholinesterase ()
1. Introduction
Acetylcholinesterase (AChE) (3.1.1.7) is one of the best studied enzymes found in scientific literature, partly due to its physiological role in the neurotransmission process and also to the remarkably high efficiency displayed by AChE, which has a large turnover number [1] [2] . AChE is responsible for the cleavage of acetylcholine (ACh) within the neuromuscular junction and the synaptic cleft in the central nervous system of both vertebrates and invertebrates [3] [4] . It is highly conserved along evolution. Due to its importance in insects, it is a target for many substances used as pesticides [5] . AChE inhibitors have pharmaceutical and commercial importance, and side effects should be an important issue to be considered [6] .
Carbaryl is a carbamate organic compound and classical noncompetitive inhibitor of AChE, with widespread use as insecticide [7] . Acute intoxication of this pesticide class primarily affects agricultural workers, causing short- and long-term health damages [8] . Clinical conditions include symptoms referred altogether as cholinergic syndrome, embracing among others sialorrhea, diarrhea, bronchial hypersecretion and, in severe cases, mental disorder and even death [8] [9] .
Carbaryl intoxication may be confirmed by assaying AChE activity. Spectroscopic assays comprise the standard approach to determine parameters from AChE activity. They are widely used in laboratories around the world. Ellman’s method [10] is the usual manner to obtain indirectly the kinetic values of AChE using an artificial substrate, acetylthiocholine (ATCh) [11] . As for several enzymes, the product and substrate of AChE are not suitable for direct evaluation by UV-VIS absorbance or fluorescence, thereby, the cleavage of ACh as long the reaction process cannot be observed by spectrophotometric techniques. On the other hand, calorimetric assays by isothermal titration calorimetry (ITC), although not as easily performed, return values considerably accurate [12] . The advantages of ITC are the use of enzyme and substrate only, not requiring any additional compound to generate measurable signal, and the possibility to analyze the natural substrate reaction of AChE [13] .
ITC measures the heat exchange in a physical-chemical process and is used typically for binding assays; however, this technique also allows the determination of enzyme kinetics [14] . When a reaction occurs in the assay cell, there is liberation or absorption of heat by the system inside the cell. This causes a drop (exothermic reaction) or increase (endothermic reaction) of power supplied by the electric devices that maintain the temperature inside the cell. This generates a thermogram showing the heat flow (μcal/s) applied by the equipment [12] .
When comparing the initial reaction rates (v0) with the initial substrate concentration, for most of the enzymes studied a rectangular hyperbole arises; those are called Michaelis-Menten (M-M) enzymes [15] . In the literature, linear approaches to evaluate kinetics from M-M enzymes are widely used. However, this linearization lacks precision, i.e. primarily because results obtained at low substrate concentrations overwhelmingly affect the fitted parameters [16] .
The present study evaluates the kinetics of AChE by ITC, which allowed the comparison of its activity with the natural (ACh) and the artificial (ATCh) substrates. To achieve better precision, the integrated form of Michaelis-Menten equation was used to determinate experimental kinetic values. Additionally, the effect of the pesticide carbaryl, a well-known inhibitor, was also assessed and compared with the conventional methods [5] .
2. Experiment
2.1. Materials
The enzyme acetylcholinesterase, AChE (EC. 3.1.1.7), extracted from electric-fish (Electrophorus electricus), lyophilized, was obtained from Sigma-Aldrich®. The substrates used were acetylcholine chloride (A6625) and acetylthiocholine chloride (A5626), both obtained from Sigma-Aldrich®.
2.2. Enzyme
The concentration of the enzyme stock solution was determined by spectrophotometric assay at 280 nm, using 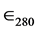 = 125.730 M−1∙cm−1, and the result, confirmed by colorimetric assay using Ellman’s method [10] , was 3.0 mM (± 0.50). The concentration of use, 30.0 pM, was the same for all experiments and obtained by dilution from that stock. All assays were performed in Tris-HCl buffer 0.05 M with ionic strength 0.148 M by the addition of NaCl 0.05 M and MgCl2 0.01 M. The buffer with the enzyme also contained 0.1 mg/L of ultrapure bovine serum albumin (Sigma-Aldrich). The experiments were performed at pH 7.4 and 37˚C (310.15 K), except when otherwise stated.
= 125.730 M−1∙cm−1, and the result, confirmed by colorimetric assay using Ellman’s method [10] , was 3.0 mM (± 0.50). The concentration of use, 30.0 pM, was the same for all experiments and obtained by dilution from that stock. All assays were performed in Tris-HCl buffer 0.05 M with ionic strength 0.148 M by the addition of NaCl 0.05 M and MgCl2 0.01 M. The buffer with the enzyme also contained 0.1 mg/L of ultrapure bovine serum albumin (Sigma-Aldrich). The experiments were performed at pH 7.4 and 37˚C (310.15 K), except when otherwise stated.
2.3. Substrates and Inhibitor
The substrates ACh and ATCh were prepared and stocked at 0.1 M, and diluted for use in Tris-HCl buffer 0.05 M, pH 7.4 at 37˚C, with MgCl 10 mM and NaCl 100 mM. Carbaryl analytical standard (Brand Sevin® 99.6%) was stored at a concentration of 10 M in a solution of methanol 12.38 M in deionized water. The final concentration of methanol was 0.1 mM in each sample, the same concentration was added to control solutions.
2.4. ITC Assays
Assays were performed in isothermal titrating microcalorimeter VP-ITC (MicroCal® GE). Periodic calibration of the device was conducted by the author and by laboratory team using the procedure described in the equipment manual provided by GE. The default values are described in the literature [17] . The enthalpy was measured by adding the substrates (4.0 mM ATCh and 10 mM ACh) with 15 successive injections of 8.0 μL into the sample cell in the presence of AChE, with an interval of 120 s between the injections. The reaction was buffered in Tris-HCl buffer 0.05 M, NaCl 0.05 M and MgCl2 0.01 M (pH 7.4) at a temperature of 310.15 K. Control experiments were conducted in the absence of AChE.
2.5. Enzymatic Assay on ITC
Experimental solutions were degassed with a vacuum pump (ThermoVac, MicroCal®) and let to reach thermal equilibrium for 5 min prior to experimental run. The heating reference was 30 μcal/s, and the stirring speed was 215 rpm. To analyze the substrate hydrolysis, sequential injections of 1, 2, 4, 6, and 8 μl, the last until the end of the assay, with an interval of 120 s between them, of 10 mM ACh. For ATCh assay, the same protocol was used.
Todd and Gómez in 2011 [18] developed a method for direct measurement of the rate of product formation, from dq/dt, the heat flow per time, which is proportional to the power variation supplied by ITC device to the system analyzed, as follows in Equation (1):
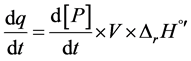 (1)
(1)
where [P] is the molar concentration of product generated, within a certain volume (V). The power variation provided by the temperature controller maintains the temperature at the desired value (310.15 K). By Rearranging Equation (1), the reaction rate can be determined knowing the change of power provided by the VP-ITC, as the rate of heat generated by the enzyme (dq/dt) was equivalent to the variation in instrumental thermal power divided by reaction enthalpy (∆rH˚’) and volume [18] . Thus, in Equation (2):
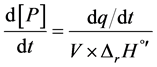 (2)
(2)
The variation of enthalpy can be obtained from the area definite by the power curve until it returns to the baseline, after consuming the whole substrate (STotal), and it is equal to the total heat of reaction [18] , as shown in Equation (3):
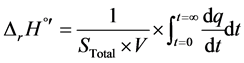 (3)
(3)
After these steps, the values of rate and substrate concentration could be used to determine the kinetic parameters, kcat, the first-order rate constant, and Km, Michaelis-Menten constant, by applying to Michaelis-Menten Equation (4). It is also possible to determine the thermodynamic parameters of activation by Eyring Equation (5):
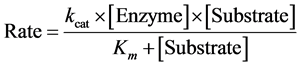 (4)
(4)
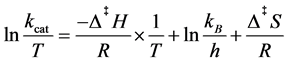 (5)
(5)
where kB is the Boltzmann constant (1.3805 × 10−23 J/K), h the Planck’s constant (6.6256 × 10−34 J/s), Δ‡H and Δ‡S the enthalpy and entropy of activation, T the temperature and R the gas constant (8.3145 J/K mol) [19] .
2.6. Integrated Michaelis-Menten
Since its formulation, it is possible to write Michaelis-Menten as a superposition of linear and logarithmic function obtained by integrating the equation [20] . In an enzyme kinetic method, it is important observing the concentrations of substrates and products throughout the time from start until the end of reaction. The integrated M-M equation is a more appropriate approach for the enzymatic reaction, since a usually kinetic reaction is expressed as either product or substrate concentration dependent of time [21] . Main advantages of integrated Michaelis-Menten includes the absence of differentiating data in order to obtain initial velocities, and the ability to determine these initial velocities with high accuracy [22] .
Nonetheless, the function is implicit, with several variables, which can be solved by numerical method with the help of basic software programs [21] [22] [23] , in Equation (6):
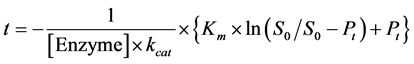 (6)
(6)
where, t is time observed, S0 is the initial substrate concentration and Pt is the total product formed until a time t, by a certain concentration of enzyme, [Enzyme]. To a reaction with a competitive inhibitor, inhibition constant, Ki, can be calculated the knowing the inhibitor concentration, I, and the turnover number, kcat, by Equation (7):
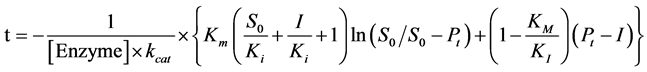 (7)
(7)
2.7. Analysis
The calorimetry data were analyzed with Origin 9.0, and with graphs generated with Origin 9.0, GraphPad Prism 6.01 or Microsoft Office Excel 2013.
The experiments were performed in triplicate. For each result, a statistical weighed analysis was used, which took into account the errors associated with each variable. The F-test with Akaike’s information criteria (AIC) was used to verify the similarity between regressions. Normality was tested using Shapiro- Wilk test, given P-values always superior than 0.05, and Student’s t-test was used to evaluate two groups. Linear regressions had the weight 1/y2 adjusted for each analysis. Data are expressed as mean (±S.E.M.).
3. Results
3.1. Ionization Enthalpy
The value of the ionization enthalpy ΔiH˚ was obtained from the literature [24] [25] [26] . Thus, the apparent enthalpy of the reaction, ΔrH˚’, is a sum of the enthalpy of reaction and enthalpy of ionization for a given number of moles. As a result, the value of ΔiH˚ = ?12.00 (±0.66) kJ/mol was used.
The hydrolysis of ACh by AChE gives a ΔrH˚= ?29.76 (±2.19) kJ/mol (Figure 1(a)). Similarly, the hydrolisis of ATCh shows a value of ΔrH˚ = ?24.81 (±1.70) kJ/mol (Figure 1(b)) and it was slightly lower when compared with the value observed with ACh.
3.2. Activation Energy
The linear analysis from Eyring equation was used 1/y2 as an adjusting of weight, for decreasing the sum of squares of errors, since the y-axes, ln(kcat/T), carry
![]()
Figure 1. Enthalpy energy obtained from ITC for both substrates. In (a) there is the isothermal curve for ACh. The standard enthalpy was obtained using 4 mM of ACh, in two different injections protocols in the same experiment. The system was allowed to reach thermal equilibrium at 37˚C. After a 120 s after the equilibrium period, successive injections of 8 μl of 40.0 mM ACh were made every 10 min. The enthalpy was calculated by Equation (3) and a baseline corresponding to heat of dilution was subtracted from the data, and correct to the concentration of AChE 30.0 pM. In (b) the isothermal curve for ATCh 10.0 mM in a protocol only with 8 μL injections by the syringe and titrating them in the cell containing 30.0 pM of AChE, with buffer.
most experimental error than the x-axis, 1/T. The results from calorimetric assays in different temperatures could be observed in Table 1, notably the pronounced similarity in Δ‡G˚ values exhibited by both substrates.
The experiment was conducted in different temperatures; thus, it was possible to evaluate the increase in kcat value with the rising of temperature. The Student’s t-test shown a similar result for both substrate’s Δ‡G˚ (p = 0.85), due to the structural resemblance they have. Nevertheless, the values for Δ‡H˚ and TΔ‡S˚ are significantly distinct from each other, displaying a different driven reaction for acetylcholine (Figure 2(a)) compared with acetylthiocholine (Figure 2(b)).
3.3. Kinetic Parameters
The simultaneous nonlinear regression analysis (SNLR) uses a concurrent analysis of the data adjusted for M-M equation, the result of inhibition kinetic parameters is seen in Figure 3, and it shows the results obtained for kcat and Km values. The kcat could be defined as the number of molecules converted by an enzyme per time unit, or a first-order constant, otherwise, Km is the ratio among direct and inverse reaction constants [19] [27] . Enzymological analysis is typically examined from the point of view of the “catalytic efficiency”, wherein the ratio kcat/Km is seen as a useful indicator of the relative processing power for an enzyme [28] . In presence of different carbaryl concentrations, a classic competitive inhibitor, it is possible to calculate the value of Ki [29] . The Ki may be thought as the amount of inhibitor required to decrease the reaction; smaller this value, the inhibitor would be more effective [30] . The results for ACh and ATCh are shown in the graphs from Figure 3. The kcat values for both analyzes were statistically equal when made F-test with Akaike’s information criterion (AIC) where ACh had a probability >88.21% to be equal in all experiments and to ATCh, they had all probability >61.77% being equal.
![]()
Table 1. Values of kcat and activation thermodynamic parameters obtained in ACh and ATCh catalysis kinetics at different temperatures.
![]()
Figure 2. Eyring linear analysis of catalytic constants obtained in different temperatures. Analysis performed at five temperatures for ACh and four for ATCh and adjusted to Eyring equation, Equation (5), where is possible to obtain the thermodynamic parameters of activation, Δ‡H and Δ‡S. The graph (A) represents the linear regression for ACh and has a correlation coefficient, r = −0.9926. In (B) is the setting for ATCh with r = ?0.9747.
3.4. Kinetic Parameters by Integrated Michaelis-Menten Equation
The integrated form of Michaelis-Menten equation simultaneous analyze various concentration of product formed per time directly by the initial substrate concentration after each injection. This is an iterative nonlinear analysis method and is fitting by the method of least squares, returning the kcat and Km values. For this, a table is made in a simple program, such as Microsoft Office Excel®, so that all values of t, S0, Pt, and [Enzyme] are aligned in columns side by side [23] [31] . Using the Solver tool, within the program, you can perform the analysis using the Equations (6) and (7). Results are seeing in Table 2.
4. Discussion
From the data obtained, the values of Δ‡G˚ ACh and ATCH are very similar for both groups (p = 0.8426), which is plausible since both have a structural similarity, therefore, requiring resembling chemical steps to reach the transition state. However, the replacement of an oxygen by a sulfur in ATCh reflects the difference in the enthalpy factor activation of a substrate to another (p = 0.0001), where Δ‡H˚ for ACh is almost twice that observed for ATCh. This may be due to a greater enzyme specificity for the transition state to the original substrate than for the modified. Although the value of activation entropy, Δ‡S˚, increases in both cases, comments on this variation must be careful, even in a general base catalysis, as occurred in the enzyme deacylation. This reaction has a transitional state with a lot of spatial freedom, although the variation of entropy in the activated state depends not only on factors intrinsic to the reaction, but also the environment in which this occurs [32] .
![]()
Figure 3. Nonlinear regression analysis of M-M Equation (4) for substrates ACh and ATCh. Kinetic graphs at 310.15 K in the presence and absence of inhibitor aside their ITC graphs. In (a) SNLR for M-M equation for ACh, (b) ITC assays for ACh, (c) SLNR of M-M equation for ATCh, and (d) ITC assays for ATCh. The concentration of inhibitor is μmol/L. Calorimetric assays were performed with successive injections spaced at 150 s between them, containing increasing volumes of 1, 2, 4, 6, and 8 μl until finishing, 20 mM ACh or ATCh, after the equipment has reached thermal equilibrium at 37˚C. The change in thermal power was acquired from the baseline shift, transformed to rate using equation (2). The rate was further used to obtain kinetics values through MM-equation, Equation 4. The SNLR analysis was performed concomitantly, resulting in a Ki value, as well the Km and kcat.
![]()
Table 2. Results from mathematical analysis using the integrated Michaelis-Menten equation.
The activation value of the standard enthalpy change in the working Cabib and Wilson (1956) [33] was between 140 - 190 kJ/mol, however, they had determined that activation energy changes with temperature. As shown, the activation entropy is high, which shows great clutter between enzyme and substrate in the transition state, a possible step used by AChE in order to increase the rate of reaction [33] . However, the entropic factor is also preeminent, which would, at a first glance, bringing the idea that the reaction is listless, which is not the case.
The enzyme velocity value using ATCh is lower when compared with ACh, in all analyses. This possibly occurs by the fact the enzyme has more interaction with the transition state from its natural substrate than for the synthetic substrate. As already mentioned, the catalytic efficiency of an enzyme would be given by kcat/Km, which is the value whose second-order constant, k1, assumes whether the limiting step of the reaction was the collisional frequency between substrate and enzyme. This could only be assumed when the kcat value is large enough to not be considered as limiting step, and the approach given to situations where [S] is very small, the second-order constant, k−1, for the enzyme- substrate complex dissociation to free enzyme and substrate is negligible.
The values from integrated M-M are similar to those obtained by nonlinear regression analysis for both substrates. However, since only one curve was evaluated in each assay for integrated M-M, the deviation observed by the experimental fluctuation cannot be taken into account.
Analyzing all the results, it can be verified a similarity between Km values for both substrates in the absence of inhibitor, motivated by the similarity in affinities to the substrates by the enzyme. As Km is an apparent ratio measured of enzyme-substrate complex formation ratio to substrate catalysis into product, a low Km value means that a lower concentration of substrate is required to achieve maximum rate of kinetics. Similar values of Km explicit similar affinities, but only part of the problem is solved by this interpretation [34] .
The values obtained for Ki allow to say that the inhibition constants of carbaryl are low and similar for both substrates. This may be a reflection of how carbaryl acts as an inhibitor, entering the active site of the enzyme, interacting with the aromatic amino acids of the anionic site of the enzyme, and blocking the entry of the substrate into the enzyme’s gorge [4] . Considering Ki as the amount of inhibitor required to slow down the reaction; lower that value, more effective is an inhibitor. Thus, as the specificity of AChE to ACh is large, higher inhibitor concentrations are needed to displace ACh from the active site, but with ATCh the opposite occurs, a lower concentration of inhibitor is already able to carry carbaryl out from AChE active site, when compared to ACh.
Remarkably, the values from ordinary nonlinear regression are very similar to those obtained by using the integrated M-M analysis for both substrates. In recent fields of enzymology, there is the integrated form of the classical Michaelis-Menten equation, where the solution to catalytic constants can be reached directly by the concentration of product formed as a function of time [21] . However, the way found by direct integration of the equation is implicit, where the mathematical process to obtain it is more exhausting. The method used in this work is supported elsewhere [23] , where the concentration of product formed in near zero time are measured in function of time without the need to use differential for determining velocities. In this method, information is equal to that required to provide a more classical test, but in a single analysis can determine the catalytic constant and varying the inhibitor concentration, also the inhibition constant.
5. Conclusion
Although the difference observed in both substrates structure, there is a slightly change of the values of constants because of their distinct interactions with the enzyme. This is also evidenced in different Ki values, although very close, the values are higher for ACh, showing that more inhibitor should be necessary to move the natural substrate of the enzyme’s active site. An alternative analysis that can save time and return more precise values is the integrated form of the Michaelis-Menten, although in this work, the implicit form was used, requiring more robust computations. The values obtained by this analysis are comparable with those obtained by classical analyses of open explicit form of the equation. Thus, the robustness of ITC enlightens an enzymatic kinetics and thermodynamic parameters values of a reaction, and even better with a more precise mathematical method. Although the traditional spectrophotometric assay is more viable to be used, this work shows comparable kinetics values between ACh and ATCh, while not precisely identical, and any approximation even if accompanied by an error is correct.
Acknowledgements
This study is supported by Brazilian Ministry of Health (n. 17217.9850001/12-025). The authors posthumously thank Professor Marcelo M. Santoro for his immeasurable help in this work.