
1. Introduction
Based on a formal definition of a causal model of an area of physics (see [1] and [2] ), a local causal model of quantum theory (QT) including quantum field theory (QFT) is described where “quantum objects” are defined as the elementary units of causality and locality. Quantum objects run autonomously with system state update frequency based on their local proper time and with no or minimal dependency on external parameters. The independence of quantum objects from external parameters is limited by 1) quantum objects interaction with other quantum objects and by 2) quantum objects interrelationship with space. Interactions between quantum objects are extensively discussed in [2] . The quantum objects interrelationship with space is only roughly discussed in [2] and is the main subject of this article.
In general, a causal model does not aim at providing a new theory with new predictions in an area of physics, but rather aims for another view or interpretation of the existing theories with as much compatibility as possible with the predictions of the existing standard theories. However, as it turned out, with certain aspects this new interpretation of the existing standard theory led to the detection of (partly already known) deficiencies, holes or uncertainties in the existing theories which need to be resolved before the new interpretation (i.e., the causal model) can be completed. This holds true also for the causal model related to spacetime considerations. The starting point for the causal model related spacetime considerations had to be the relativity theories (special relativity theory [SRT] and general relativity theory [GRT]). The guiding principles of SRT and GRT such as the constant speed of light (SRT), Lorentz invariance (SRT) and the Equivalence Principle (GRT) concern relationships between systems moving with different relative speeds or between systems in different gravitational environments and different acceleration. From the laws concerning global inter-system relationships, the laws for the local systems dynamics have been deduced. For example, the law that an inertial system has its local proper time has been deduced from the overall law of Lorentz invariance of SRT.
In contrast to the standard interpretation of SRT and GRT, the causal model related interpretation of SRT and GRT starts with the assumption of the autonomy of quantum objects. This means that the dynamics of quantum objects is determined purely by local laws (i.e., laws that depend on the local state only). Overall inter-system relationships should be deducible from the quantum-object- local laws together with laws concerning the interactions between quantum objects and the interface of quantum objects with space. Ultimately, the predictions that can be derived this way should be compatible with the predictions of SRT and GRT.
Although the integration of space and time as introduced with the theories of relativity in general remains valid, in the causal model of QT/QFT there exist a number of aspects where the treatment of time differs from that of space. As the major consequence of the autonomy of quantum objects, the time struc- ture is composed of local time units based on the quantum objects proper time. In contrast, the space serves as the global medium for all inter-object relationships.
The goal of this paper is to show how the causal dynamics of quantum objects (as described in [2] ) can interface with the causal dynamics of spacetime (as described in this paper). This includes refinements of the causal model described in [2] . The assumption made for the spacetime model―that space curvature begins and is relevant already with the basic processes of quantum field theory―enables a refined treatment of interactions between quantum objects (Section 6).
It is well known that for the discussion of spacetime structures in relation to QT/QFT, pure GRT is not sufficient. The causal model uses the theory of causal dynamical triangulation (CDT) (see [3] ) as one of its bases because CDT matches a number of features that are also supported by the causal model of QT/QFT as described in [2] :
・ CDT is a “background-independent” spacetime theory.
・ CDT contains major elements in support of causality. For example, in CDT spacetime emerges.
・ CDT assumes discretized spacetime.
Although GRT and CDT are mandatory (resp. suitable) bases for the spacetime considerations in a causal model of QT/QFT, they need to be supplemented by additional specifications in order to obtain a causal model.
Sections 2, 3 and 4 mainly summarize what is already described in [1] and [2] to the extent necessary for the understanding of the remaining sections which describe the proper subject of the article. Section 5 describes the major processes for the evolution of space (e.g., the expansion of space). Based on the assumption that the processes involved in the changes of spacetime structure have to be applied already at the level of quantum objects, in Section 6 the causal model of the evolution of space is applied to the process of QFT-interactions. QFT- interactions play a key role in the causal model of QT/QFT described in [1] and [2] .
Although the described spacetime model has been developed with a focus on quantum objects relationship to space, the model may also be applied to larger-scale spacetime considerations such as questions about the possibility of a (static or varying) cosmological constant. Some of these topics are roughly discussed in Section 7. More detailed discussions are outside the scope of this article.
2. Causal Models
The specification of the formal model of a theory of physics consists of (1) the specification of the system state and (2) the specification of the laws of physics that define the possible state transitions when applied to the system state. For the formal definition of a causal model of a physical theory, the laws of physics are represented by a “physics engine”. The physics engine acts upon the state of the physical system. The physics engine continuously determines new states in uniform time steps. For the formal definition of a causal model of a physical theory, the continuous repeated invocation of the physics engine to realize the progression of the state of the system is assumed.
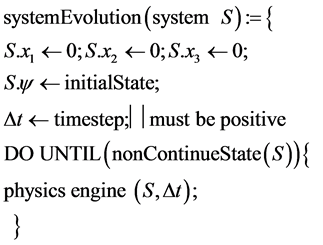
The refinement of the statement
defines how an “in” state s evolves into an “out” state s.
...
The “in” conditions
specify the applicability of the state transition function
in basic formal (e.g., mathematical) terms or refer to complex conditions that then have to be refined within the formal definition.
The state transition function
specifies the update of state s in basic formal (e.g., mathematical) terms or refers to complex functions that then have to be refined within the formal definition.
To enable non-deterministic theories (“causal” does not imply deterministic) an elementary function RANDOM (value range, probability distribution) may also be used for the specification of a state transition function.
The set of laws
has to be complete, consistent and reality conformal (see [4] for more details).
In addition to the above described basic forms of specification of the laws of physics by
, other forms are also imaginable and sometimes used in this article.1
Note the following comments on the notation used for the specification of causal models. While in mathematics and in programming languages the “=” sign is used for three different purposes, in this article three different notations are used for different purposes:
1. “=“ indicates a relation, as in
, reading a equals b.
2. “:=” means “is defined as”. For example,
means spacepoint is defined as ...
3. “
” indicates a value assignment.
means that the value of the expression on the right-hand side (y) is assigned to the item on the left-hand side (x).
This notational distinction is used only in causal model specifications. In traditional mathematical expressions occurring in this paper, the traditional meaning of the “=” sign is used. The distinction of the three types of “equality” has significant implications for the specification of a causal model. It means that
typical mathematical equations, such as
(see below), must not be
taken unchanged for the laws of physics
. The traditional symmetric equal sign appearing in “
” has to be replaced by the asymmetric “
”.
Example 1―A causal model: Many areas of physics can be described by starting with a specific Lagrangian. For a description of the causal relationships, i.e., the evolution of the system state, the equation of motion is the major law. The equation of motion can be derived from the Lagrangian by using the Euler-Lagrange equation.
The Lagrangian for classical mechanics is
with
.
The Euler-Lagrange equation leads to the equation of motion
.
The specification of the laws of classical mechanics can be given by a list (
) that distinguishes different cases or by a single general law. The single general law is
Thus, the system state has to contain
Types and Properties of Causal Models
Spatial causal model: A causal model of a theory of physics is called a spatial causal model if (1) the system state contains a component which represents a space, and (2) all other components of the system state can be mapped to the space.
There exist numerous textbooks on physics (mostly in the context of Relativity theory) and on mathematics which define the essential features of a “space”. For the purpose of the present article a more detailed discussion is not required. For the purpose of this article and the subject locality it is sufficient to request that the space (assumed with a spatial model) supports the notions of position, coordinates, distance, and neighborhood.
Example 2―A spatial causal model: A possible type of a spatial causal model is the cellular automaton (CA). The classical CA consists of a k-dimensional grid of cells. The state of the CA is given by the totality of the states of the individual cells.
With traditional standard CAs, the cell states uniformly consist of the same state components
Typically, the number of state components, j, is 1, and the possible values are restricted to integer numbers. The dynamical evolution of the CA is given by the “update-function”, which computes the new state of a cell and of the neighbor cells as a function of the current cell state.

The full functionality and complexity of a particular CA is concentrated in the update-function. As Wolfram (see [5] ) and others (see, e.g., [6] ) have shown, a large variety of process types (e.g., stable, chaotic, pseudo-random, and oscillating) can be achieved with relatively simple update-functions.
Local causal model: The definition of a local causal model presupposes a spatially causal model (see above). A (spatially) causal model is understood to be a local model if changes in the state of the system depend on the local state only and affect the local state only. The local state changes can propagate to neighboring locations. The propagation of the state changes to distant locations; however, they must always be accomplished through a series of state changes to neighboring locations.2
Based on a formal model definition of a causal model, a formal definition of locality can be given. We are given a physical theory and a related spatially causal model with position coordinates x and position neighborhood dx (or
in case of discrete space-points).
A causal model is called a local causal model if each of the laws
applies to no more than a single position x and/or to the neighborhood of this position
.
In the simplest case, this arrangement means that
has the form
The position reference can be explicit (for example, with the above simple case example) or implicit by reference to a state component that has a well-defined position in space. References to the complete space of a spatially extended object are considered to violate locality. References to specific properties of spatially extended objects do not violate locality.
3. The Causal Model of Quantum Theory and Quantum Field Theory
The causal model of QT/QFT (i.e., quantum theory and quantum field theory) consists of the specification of the system state and of the laws of physics that proceed the system state. Both items are here only roughly described. More details can be found in [1] and [2] . The system state consists of the following components:
;
The laws of physics that progress the system state are embedded in the following overall process:

The evolution of quantum objects, i.e., “update-quantum-objects”, is the major subject of the causal model of QT/QFT. It is partly described below in Sections 4 and 6. More details can be found in [1] and [2] . “update-space”, i.e., the dynamics of space is the main subject of the present article.
The causal model of QT/QFT assumes a separate physics engine associated to each quantum object and a single further physics engine associated to the space.
4. The Quantum Object
The quantum object is the most important entity for the description of the causal model of QT/QFT. A particle may occur as a separate quantum object or be part of a quantum object. The following three properties distinguish quantum objects from other objects that typically occur in physics:
1) Quantum objects are composed of multiple alternative paths with associated probability amplitudes. With the interactions (including the measurements), the multiple paths may be reduced to a single path.
2) Quantum objects may consist of multiple spatially separated particles.
3) Quantum objects have global attributes that apply to all of the paths and particles of the quantum object.
The combination of these three properties makes quantum objects special within physics.
A quantum object may be viewed as having a two-dimensional structure. One of the dimensions represents the collection of quantum object elements, which typically consists of 1 to n particles.

In the second dimension, the quantum object consists of the set of alternatives that may be selected during the evolution of the quantum object, for example, by a measurement. In this paper, these alternatives are called “paths”.

The two-dimensional structure is supplemented by global attributes. Whereas global quantum-object attributes are attributes that apply to the complete quantum object, particle attributes apply to the complete particle. “Amplitude” is the single attribute that applies to a complete path. The only space-point-local attributes are the attributes labeled “particle-i.path-j” in Figure 1. Global attributes disturb space-point localities. Similarly, the inclusion of global attributes may be unavoidable for the construction of causal models in theories that contain non-lo- calities. The confinement of the non-localities of QT/QFT within quantum objects (by assuming the global attributes) supports the view of quantum objects as the elementary units of causality and locality.
Examples of Quantum Objects Different types of quantum objects can be distinguished:
![]()
Figure 1. Example of a quantum object which consists of two entangled particles.
・ A single particle
A single particle represents the simplest type of quantum object. The idea of representing a particle by a set of paths was introduced by Feynman (see [7] ) with the formulation of quantum electrodynamics (QED).
・ Collections of (entangled) particles
Collections of particles that can be described by a common wave function where only specific attribute combinations can occur as measurement results represent a quantum object. Thus, the particle collection is represented by a set of paths, and each path contains the attribute combinations for all of the particles and an associated probability amplitude (see Figure 1). Arbitrary particle collections whose common wave function would be the product of the individual wave functions do not constitute quantum objects (As a consequence, considering the whole universe as a single large quantum object would not be in accordance with the definition of the term quantum object given in this paper).
The following additional types of quantum objects are special examples of particle collections.
・ Interaction object
The interaction object is a type of quantum object that was described in [8] as part of a functional model of QFT-interactions. It is created at the beginning of an interaction. At the end of the interaction, the interaction object is transformed into an interaction-result quantum object.
・ Interaction result object
The result of a QFT-interaction (see Section 6.1) is a quantum object containing all of the particles resulting from the interaction and the probability amplitudes for the resulting paths. The causal model of QT/QFT assumes that the interaction object develops into the interaction result object.
・ Bound system quantum object
Composite objects such as hadrons, nuclei, and atoms that are built from (elementary) particles are incorporated into the concept of a quantum object. The elements of the composite quantum object may be grouped to form an internal structure. For example, the atom consists of the nucleus and electrons, and the nucleus consists of hadrons. If this type of a hierarchical structure is given, only the complete (outermost) entity is called a quantum object within this paper.
Quantum objects are dynamically created, split and combined in specific processes such as interactions and decays.
5. The Causal Model of Spacetime
In Einsteins theories of relativity, space and time are united into the four- dimensional spacetime. In the causal model of a theory of physics (see Section 2), space and time are treated very differently. Time and the progression of time is an inherent feature of the physics engine associated with each individual quantum object. Time is a quantum object-local property. In contrast, in the causal model, space is the global object that is referenced by quantum objects whenever global interrelationships among quantum object have to be implemented. The assumption/requirement that the space itself changes dynamically (e.g., expands and changes its curvature) resulted in the association of a separate physics engine to the space. The physics engine assigned to the (global) space determines the speed of changes (e.g., expansions and curvature changes) of the space.
Thus, the general causal model of a theory of physics shows a rather non- united picture for space and time. The (specific) causal model of QT/QFT including spacetime considerations, however, leads to an integration of the two concepts such that compatibility with GRT and SRT is largely maintained. The (re-)integration of space and time in the causal model of QT/QFT is caused by the model assumption that all space dynamics (e.g., expansions and curvature changes) are triggered by events of the quantum objects (e.g., creation and movement of quantum objects) and the assumption that the events of the quantum objects occur according to the quantum objects local time.
Space: In the proposed causal model, the following assumptions are made concerning space:
・ The dynamics of space (i.e., creation and expansion of space, change of space curvature) evolves from local sources to the largest possible scope (i.e., to the whole universe).
・ The space changes start at the elementary units of causality and locality, the quantum objects (see [2] ). Large-scale changes and large-scale sources of changes are aggregations of changes triggered by quantum objects.
・ Space changes propagate with limited speed, the speed of light.
・ The space is an active object, i.e., its dynamics, is driven by a physics engine with a global proper time interval.
Time: In the causal model, time (in contrast to space) is not a component of the system state, but an inherent property of the physics engine. Individual physics engines are assigned to quantum objects and to the (global) space.
5.1. Basic Assumptions
The model of spacetime is based on certain assumptions that are taken over from GRT, causal dynamical triangulation and the causal model of QT/QFT described in [2] .
Basic assumptions derived from GRT: The spacetime structure of the causal model of QFT/QT aims at maximum compatibility with GRT. The following is a list of some of the major assumptions derived from GRT:
・ The energy (including mass) distribution within the universe determines the structure (i.e., curvature) of spacetime.
・ The structure of spacetime determines the movement of free particles (i.e., particles that are not affected by any forces except gravity) within space.
・ Spacetime is a dynamical object. It may expand and it may change its structure (i.e., curvature).
・ Changes of the spacetime structure propagate with limited speed, namely, the speed of light, c.
Basic assumptions taken from the causal model of QT/QFT described in [2] : The causal model of QFT/QT described in [2] assumes the following:
・ Discretized space, time, and paths of quantum objects.
・ Quantum objects run autonomously, which means that the state progression of a quantum object is performed with the quantum objects individual proper time.
・ Space is viewed as an additional physical object which is shared by all quantum objects.
・ The dynamical changes of spacetime are triggered by the changes (e.g., move- ment) of quantum objects. Spacetime changes due to larger compact physical objects are aggregations of the collections of quantum objects.
Causal dynamical triangulation: In [9] , causal dynamical triangulation is described as follows:
“Causal dynamical triangulation (abbreviated as CDT) invented by Renate Loll, Jan Ambjorn and Jerzy Jurkiewicz, and popularized by Fotini Markopoulou and Lee Smolin, is an approach to quantum gravity that like loop quantum gravity is background independent”.
CDT assumes triangles (two-dimensional) and tetrahedrons (three-dimensional) as discrete building blocks of spacetime and offers a theory of the dynamical evolution of such a spacetime. The assumption of discrete background independent spacetime together with some causal theory of the evolution of spacetime makes CDT an attractive candidate base for the spacetime structure of the causal model of QFT/QT. The use of CDT as the base for the spacetime structure of the causal model of QFT/QT, however, resulted in the necessity for additional specifications (e.g., the initial space object for space expansions), adjustments of CDT, and specializations of the original CDT described in [3] [10] and [11] . As the major deviation from standard CDT, in the causal model of QFT/QT the evolution of space is considered separately from the evolution of time. The reintegration of space and time is obtained by the fact that the evolution of space is caused by the evolution of the quantum objects and the evolution of the quantum objects occurs with the quantum objects local proper time.
5.2. System State Components of Space
;
;
;
refnumber and connections serve for the specification of references to spacepoints and neighborhood relationships in a causal model. For a specific causal model, these two variables can be replaced by the coordinates of coordinate systems. In GRT, the curvature of the space is represented by the suitable tensor.
Roughly speaking, the space consists of (a) the space structure (i.e., refnumber, connections, curvature) and (b) the space contents (i.e.,
). The space contents may be provided by a quantum object (e.g., a particle) or by a field.
5.3. Creation and Expansion of Space Resulting from a Single Source Quantum Object
For a causal model, which aims for compatibility with GRT, it is reasonable to assume that the changes of space have their origin at the smallest units of causality and locality from where they propagate through the whole space. In the proposed causal model of QT/QFT, the smallest units of causality and locality are the quantum objects. Large-scale space changes, typically associated with GRT, have to be aggregations of the small-scale changes originating at the quantum objects.
Space creation and expansion of space is performed in terms of elementary building blocks which, in CDT, are called simplexes. In two dimensions, the building blocks are triangles; in three dimensions, they are tetrahedrons. In contrast to CDT, higher than three-dimensional simplexes are not considered in the causal model of QFT/QT because the time dimension is treated separately. Starting from a specific source (i.e., a quantum object), space is assumed to expand spherically until the expanding area of space overlaps with other areas of space that are generated by other sources (the case of multiple sources is discussed in Section 5.4).
Two-dimensional space: The basic space element is an equilateral triangle. Space expansion is performed in steps. With each step, a further spherical layer of space elements is added. The objective of the algorithm for the expansion of two-dimensional space is that the space resulting from a single source at each time (i.e., after each expansion step) remains as close as possible to a compact spherical manifold. The assumption that space expansion is performed in terms of uniform space elements (i.e., triangles) eases the provision of linear speed of the space extension. An algorithm that satisfies these requirements/assumptions is non-trivial, but feasible. In order to prevent the special case treatment in the algorithm for the first evolution step, the initial space is not equal to the basic space element (i.e., the triangle), but the collection of six triangles shown in Figure 2. Figure 2 also shows the space after 3 expansion steps.
![]()
Figure 2. Evolution of space in 2 dimensions.
Figure 2 shows the evolution of space in terms of spacepoints and their connections. It does not show the space curvature (i.e., curvature in the spacepoint state). The space curvature has to be specified as well. For the causal model, it is assumed that a uniform curvature is assigned to all spacepoints generated by an expansion step. The curvature is decreasing with each expansion step. GRT requests that curvature is a function of source energy (including mass). The dependency between the curvature and the source energy can be accomplished directly either by using source energy as the parameter or by making curvature a function of the sources proper time (which is also dependent on the source energy).
For the specification of a causal model, it is also necessary to specify the relationship between the quantum object that triggers the space creation and expansion and the space that performs the space expansion and modification. This is a non-trivial relationship because it involves two different physics engines with differing proper times. As a schematic view, it is assumed that the quantum object (i.e., its physics engine) performs the update of the layer of space that immediately surrounds it. Further propagation of the space update is performed by the (global physics engine of the) space. If a cosmological constant
is to be supported, this will cause the inclusion of an additional layer of space (see below “Creation and expansion of space within existing space”).
Three-dimensional space: The creation and expansion of a new three-di- mensional space resulting from a single source is the more realistic and therefore more interesting case for the causal model. Most of what has been described in the preceding paragraph with respect to requirements and assumptions for the two-dimensional case applies unchanged to the three-dimensional case.
In three-dimensional space, the basic building blocks are tetrahedrons (instead of triangles). As shown in Figure 3, the initial space of the space generation process is not the single tetrahedron, but the collection of 12 tetrahedrons shown in Figure 3. The expansion of the initial space towards larger spherical space manifolds by maintaining the requirements and assumptions described above for the two-dimensional cases is more difficult. The type of algorithm developed by the author is called a heuristic algorithm in computer science, i.e., an algorithm that finds solutions among possible ones. In addition, with the three-di- mensional case, non-uniform tetrahedrons have to be used during the space evolution process.
![]()
Figure 3. Evolution of space in 3 dimensions.
(3 + 1)-Dimensional spacetime: As mentioned above, space and time are treated separately in the causal model of QFT/QT. Therefore, four-dimensional simplexes and the evolution of four-dimensional spacetime are not considered. However, the (major) implications of the four-dimensional spacetime model of GRT have to be maintained. Also, the CDT rule that the time coordinate of the simplex evolution proceeds in uniform time steps is adopted (as described in [2] ).
Not regarding four-dimensional spacetime evolution does not exclude considerations on the joined progression of time and space. (This is just the main subject of a causal model of spacetime.) However, because the causal model of QFT/QT assumes discrete steps for the progression of time as well as for the changes of the space structure, mathematical models that assume a continuous four-dimensional spacetime manifold are not appropriate. The view of a continuous four-dimensional spacetime manifold is additionally disturbed by the assumption that the causal model always contains only a single time slice of system state as input for the computation of the follow-on state.
Creation and expansion of space within the existing space: Space creation within the existing space implies that the existing space is adapted to changes in the energy distribution within the space. In general, for the causal model of QT/ QFT, maximal compatibility with SRT and GRT is aimed. With respect to space changes, this implies that Einsteins famous equation
(1)
has to be supported by the space expansion process. As can be seen, Equation (1) contains the cosmological constant
, which was not contained in Einsteins original equation. The question arises whether
or something equivalent should be supported by the causal model, and if yes, how this affects the causal model. The author decided that the causal model should support
by assuming that space expansions that are caused by the changes in the energy distribution are always additive. This implies that the continuous invocation of the space update function also implies a continuous (not necessarily uniform) expansion of space.
The space change starts at the quantum object with the insertion of a new layer of space immediately surrounding the quantum object. From there, the space change propagates with the speed of light c in all directions within the existing space.

This means update-space proceeds from the layer that surrounds the quantum object (the layer which has been inserted in support of
) to the next outer layer. For the determination of the “nearest-neighbors-layer”, the curvature parameter associated with the spacepoint is utilized.
The dynamics of space curvature: The creation/modification of space within the existing space means, first of all, the creation/modification of the structure, i.e., curvature of the space. In Section 5.2, the curvature is specified as a parameter associated with each spacepoint. The curvature parameter of the spacepoint subsumes the role of the respective GRT tensor (e.g., metric tensor, Riemann tensor, or Ricci tensor). With single-source space expansion (Section 5.3), the assignment of the curvature parameter to the spacepoint is relatively simple. The curvature is uniformly set for all spacepoints generated during an expansion step. With increasing expansion, the curvature decreases. With multiple-source space expansion, the curvature assignment is more complex (discussed later).
For a simplified visualization of the curvature of a two-dimensional space, the curvature may be reduced to a scalar parameter and represented as an additional (i.e., third) dimension (see Figure 4).
The dynamics of space content: In the causal model of QT/QFT, two types of space contents are distinguished: 1) quantum objects and 2) fields. Fields are considered to be the attributes of spacepoints. This means fields participate in the dynamics of space, but may perform additional dynamical functions.
Quantum objects internal dynamics is unaffected by the dynamics of the surrounding space. The motion of quantum objects is performed in relation to space and as a function of the space curvature. In addition, the interaction of quantum objects (in particular when quantum objects collide) is determined by the space and quantum object interactions affecting the internal dynamics of space.
Complete vacuum, i.e., space without content (neither fields nor quantum objects) is, according to QFT, not possible. There exists at least some field in the ground state, which produces the vacuum fluctuations. In the causal model of QT/QFT, the ground state field is created in conjunction with the space generation.
![]()
Figure 4. Visualization of 2-dimensional space including curvature.
5.4. Creation and Expansion of Space Resulting from Multiple Source Quantum Objects
Space changes caused by multiple quantum objects (e.g., which constitute a larger compact object) start as multiple single-source changes in the way described in Section 5.3. After the space changes have been initiated by the multiple-source quantum objects, further propagation of the space changes are performed by the physics engine of the space as a single repetitive process.
The propagating space changes will ultimately overlap. This requires a generalization of the space expansion process described in Section 5.3.
The major additional task of the generalized expansion process is the determination of the accumulated curvature for the overlapping areas of space expansion. The propagation of the space including its curvature must be conformal to the GRT Equation (1). For the causal model of QT/QFT, the computation of the propagation of space curvature in addition has to be integrated with the process of triangulation discussed in Section 5.3.
6. Quantum Field Theory in Curved Discretized Space
Spacetime structures in the causal model of QT/QFT are the main subject of the present paper. In Section 5, the spacetime structures and processes related to spacetime dynamics have been described. It is now possible to analyze how the described spacetime structures may influence the overall causal model of QT/ QFT. The major assumption of the causal model of spacetime is that the spacetime dynamics (i.e., creation and expansion of space, curvature modification) begins at the quantum objects. The progression of the quantum object is accompanied by a repetitively invoked space update process. It is worthwhile to look into the possible closer relationship between quantum object dynamics and space(-time) dynamics.
The major items that request/enable adjustments of the causal model of QT/ QFT described in [1] and [2] are:
・ The assumption that space curvature formation and propagation begins already at the space around the quantum objects.
Although QFT is a relativistic theory, QFT treats space like an Euclidean space.3 The assumption that the formation of curvature and the expansion of space begins at the quantum objects opens new opportunities for the construction of a causal model of QT/QFT. Possible implications are:
(a) Increased probability for the unification of alternative paths
(b) A possible model for the end of the “Feynman phase” (see below) and the transition to the next phase.
(c) The occurrence of a temporary confinement phase.
・ The assumption that space is continuously expanded and modified concurrently with the progression of the quantum object has implications for both, (1) the model of spacetime dynamics, and (2) the model of quantum object progression. For the model of spacetime dynamics it means that the interval between space updates differs depending on the differing proper times of the quantum objects. For the causal model of quantum object progression the assumption of continuous (but discretized) space updates concurrently with the quantum object progression enables the retention of the static space model in between the space update cycles.
・ The usage of causal dynamical triangulation as the basic discretized space structure.
One of the arguments for using in this paper causal dynamical triangulation as the basis for the spacetime structure has been the “background independence” of the causal dynamical triangulation. Background independence here means that for the space there exists no predefined structure, coordinate system, topology, etc. Space emerges in conjunction with some physical process(es). (After the space has emerged, it may be considered to represent a background.)
For the construction of a low level causal model, background independence theoretically should allow for models which do not need to refer to coordinates. It should be sufficient to refer to the parameters which constitute the space structure of causal dynamical triangulation (e.g., neighborhood relationships). Whether this extreme form of background independence can be realized in the causal model of QT/QFT has still to be worked out.
In Section 3, the progression of the QT/QFT system state (“update-system- state”) is specified as consisting of “update-quantum-object”, “update-fields” and “update-space”. The present section gives further details on “update-quantum- object” and its relation to “update-space”.

With update-quantum-object two major cases are distinguished: 1) the normal progression (“progress-quantum-object()”) and 2) the processing of a QFT- interaction. In both cases, the update cycle of the quantum object is finished by signaling to the space that the space needs to be updated (see Sections 5.3 and 5.4).
Interactions between Quantum Objects
For two reasons, the interactions between quantum objects are key for the causal model of QT/QFT:
1) In the causal model of QT/QFT, the proposed solution for certain “QT problem areas” (the measurement problem, the “interference collapse rule” and entanglement) is based on the model of interactions between quantum objects.
2) Because quantum objects are assumed to run autonomously, their global relationships are mainly determined by the information exchange with interactions.
Typical examples of interactions are particle scatterings such as electron-photon scattering. In general, an interaction between two quantum objects may change the interacting quantum objects to differing extents. The changes may range from the changed attributes (e.g., momenta and spins) to changes in the numbers and types of the particles. The interactions that change only the particle attributes can be described by the laws of (classical) quantum theory and are called “volatile interactions” in this paper. In contrast to volatile interactions, there are also more complex interactions where the “out” quantum objects and/or the “out” particles (which are contained in the “out” quantum objects) may differ from the “in” quantum objects and particles. Because such interactions require QFT (e.g., a scattering matrix and Feynman diagrams) in their description, they are called “QFT-interactions” within this paper. With QFT-interactions, only a single path of the “in” particles determines the interaction result.
QFT-Interactions: With QFT-interactions, the paths (i.e., space points) that triggered the interaction exclusively determine the outcome of the interaction. In the causal model of QT/QFT described in [1] and [2] , the paths that do not participate in the interaction are discarded, and the interacting particles (not necessarily the interacting quantum objects) are replaced by a single new quantum object called the interaction object. The destruction of the interacting particles and their replacement by the interaction object may be viewed as the collapse of the wave functions. The creation of the interaction object implies that further expansion and modification of the space is now dependent on the further processing of the interaction object. The detailed laws of physics for the treatment of QFT- interactions are given by QFT. In [2] , the interaction process is described as consisting of several phases, namely
1) Creation of the quantum object (i.e., the interaction object)
2) Exchange of virtual particles (“Feynman phase”)
3) Creation of the interaction result object (i.e., entangled real particles)
Part of these phases is assumed to occur also with the dynamics of other quantum objects such as bound systems quantum objects. In particular, the Feynman phase (exchange of virtual particles) is generally applicable.
QFT provides three alternative approaches for the computation of the results of QFT-interactions such as particle scatterings: 1) diagrammatic perturbative computation in momentum space, 2) diagrammatic perturbative computation in position space, and 3) computation based on lattice gauge theory. Each approach has different advantages, weaknesses and limitations, thus different areas of applicability. For the specification of a causal model, the standard QFT formalism has to be mapped into a sequence of system state changes. The causal model described in [1] and [2] is mainly derived from the computation in momentum space (i.e., Feynman diagrams). The inclusion of spacetime consideration as described in the present paper enables a more detailed causal model. The improved level of detail, however, cannot be achieved with the momentum space orientation. The inclusion of space considerations requires concepts and techniques similar to those available in QFT position space computation or in lattice gauge theory. Lattice gauge theory (see [12] ) is especially suited as a base for a more detailed causal model of QT/QFT because it supports discretized space.
Processes: The causal model of QFT has to support the following processes:
・ Space/curvature update (see Section 5)
・ Field propagation
In standard QFT, the propagation of fields is typically described by the pertinent field equation or the equation of motion. For example, for the Dirac-field, the field equation is
. (2)
The creation of the causal model requires the transformation of the field equations such as Equation (2) to a causal model that refers to the discrete space elements of CDT.
・ Quantum object normal progression
The “normal” progression of quantum objects is understood as the progression of the wave function and is determined by the Hamiltonian derived from the Schrödinger equation
. (3)
In [13] a mapping of the Schrödinger equation to discrete space points (although not to the space elements of CDT) is described.
・ QFT-interaction
A causal model of QFT-interactions is extensively addressed in [1] [2] [8] and [13] . The model described has to be adapted to include the spacetime concepts described in the present paper.
・ Bound system quantum object progression
The internal dynamics of bound system quantum objects such as hadrons, nuclei, and atoms is most difficult to model in terms of diagrammatic pertubative QFT. Lattice gauge theory has been more successful in the treatment of hadrons. Therefore, the author expects that the increased utilization of the concepts and techniques of lattice gauge theory enables the inclusion of bound system quantum objects in the causal model of QT/QFT.
7. Discussions
7.1. Causal Models
The value of and need for causal models: The construction of a causal model leads to specific difficulties that may be avoided with theories that do not aim at the complete specification of causal relationships. Therefore, the question arises whether the goal of a complete specification of causal relationships is worth the effort to handle these difficulties. The author sees the following arguments in support of causal models:
1) Since the appearance of the first theories of physics until today, the determination of causal relationships, including the prediction of the results of physical experiments, has been the primary goal for doing physics.
2) Our inability to specify detailed causal relationships in certain areas of physics may be tolerated for some time, but should not lead to the explicit negation of causality or to making allowances for the lack of a better understanding. There are different areas of physics where this argument is considered (by the author) to apply to a differing degree: a) With statistical mechanics and thermodynamics, the causal relationships are understood, although not computable at a detailed level. b) The assumption of indeterminism in QT is a good working assumption, but declaring the indeterminism of QT as an inherent feature of QT (which could even be proven mathematically) is at least premature. c) Neglecting the measurement problem of QT as something where a suitable solution is highly desirable (or even necessary) is incomprehensible to the author.
3) The goal of the construction of a causal model limits the set of possible theories and models; however, this limitation may lead to models that are more realistic. For example, the author has the impression that causal models almost necessarily lead to discreteness (at least) of time. Discreteness at the lowest level may be a good assumption in general.4
The time arrow in a causal model: Causal models, as defined in Section 2, imply a direction of time that is reflected in 1) the semantic definition of the “physics engine” and 2) by not requesting only bijective functions to appear in the list of the laws of physics. Physicists who believe that the laws of physics are (by nature) time symmetric may argue therefore that the definition of the causal model given in Section 2 may be more general than necessary and suitable. This suspicion may be enforced when it is shown that, in some cases, the formulation of a causal model even necessitates the transformation of an (apparently) time symmetrical law of physics into an asymmetric sequence of causal steps.
In contrast to this possible view, the author considers causal models as the more realistic models also with respect to the specification of temporal relationships. The causal model uncovers causal relationships and the existence of an arrow of time which existed already without the causal model. The hiding of the causal relationships by the typically time-symmetric equations of physics may result in more compact and more elegant equations, but this should not be used as a proof for the non-existence of an arrow of time.
Are the laws of physics fixed forever? In Section 2, a precise definition of the type of laws of physics that are suitable for the specification of a causal model of an area of physics is given. Let us, for example, assume that the laws listed in the causal model of QT/QFT assume that the speed of light is a constant c, and it is then detected that this assumption is true for the present state of our universe, but (probably) not true for an early phase in the development of the universe. In such an assumed case, the causal model would have to be adjusted. The laws referring to a constant c had to be replaced by laws that refer to a variable c plus some laws that specify the dependency of c on other parameters of the system state. The adjusted model, including the adjusted laws of physics, however, would represent an evolution of our understanding of the laws of physics, not an evolution of the laws of physics. The example can be generalized to the detection of further possible more dynamical laws of physics.
7.2. From the Very Small Scale (Back) to the Very Large Scale
The main subject and focus of this article are spacetime considerations in the context of a proposed causal model of QT/QFT. This requires an application of the concepts of GRT, which mainly have been applied and verified at the very large scale (i.e., cosmology), to the very small scale (i.e., to QT/QFT). The resulting causal model contains a number of features that are largely derived from GRT, but which are not completely identical with those of GRT. It may be interesting to look at the possible implications if the spacetime concept of the causal model of QT/QFT is applied to large-scale physics again. Several questions arise. These questions cannot be discussed in detail within this article (and have not yet been sufficiently thought through by the author). Nevertheless, they are deemed to be worthy of a brief discussion within this article. The large-scale GRT related questions are discussed from the point of view of the causal model described in this paper. Whether the statements made are in accordance with the existing GRT and with the newest findings in cosmology is partly not clear (to the author). In many cases, GRT apparently does not have a clear position on the respective questions.
Are gravitational waves possible and detectable? The possibility of gravitational waves and the feasibility of testing gravitational waves is a typical question about a causal model. GRT and Einsteins equations (e.g., Equation (1) in Section 5.3) have to be the basis for a causal model of gravitation and thus for discussing gravitational waves. However, pure GRT is obviously not sufficient to provide generally agreed upon answers.
The causal model of spacetime described in Section 5 is based on the GRT and the assumption that changes to the structure of spacetime (i.e., expansion of space and changes of space curvature) occur at a definite source location in space from where they propagate with the speed of light through space. Gravitational waves depend on the same assumptions. Thus, the spacetime concept of the causal model of QT/QFT supports the possibility of gravitational waves, if “wave” is not understood to be the typical kind of wave that occurs in other areas of physics such as electromagnetism. In a causal model of spacetime that is compatible with GRT, it should be possible that spacetime changes that occur at location L1 propagate to location L2 (see Section 5.3). If the process at the source produces periodical spacetime changes (e.g., rotating pairs of stars), the resulting effects at location L2 may have some similarity with waves, although the waves amplitude decreases with increasing distance from the source.
Theoretically, it should also be possible to observe “waves” that are generated by (periodical) gravitational effects. Details about the criteria for the feasibility of processes that may generate gravitational waves and for the possible experiments for the detection of gravitational waves are not subject of the present paper.
Phases in the evolution of the universe: In [14] , the Big Bang theory is described in view of new findings in QFT. As the major improvement of the refined theory, the process that includes and follows the Big Bang is subdivided into a number of phases and phase transitions such as inflation, hadronization, occurrence of empty space, etc. The major cause for the various phase transitions is spontaneous symmetry breaking due to the continuous expansion of the space of the universe.
In [2] and in Section 6.1, the process that is performed with QFT-interactions, such as particle scatterings, is also described as consisting of multiple phases. The phases described in Section 6.1 are partly comparable with the phases assumed for the Big Bang theory. With both models, the phases are related to the expansion of the space. A special feature of the causal model of QFT-interactions described in Section 6 is the impact of space curvature even at small-scale QFT processes. It may be worthwhile to study whether space curvature may also affect the Big Bang related processes.
The expansion of the universe, dark energy: Recent findings in cosmology indicate that the expansion of our universe is faster than that so far assumed and possibly accelerating. For a possible explanation, the term dark energy is introduced and some cosmologists equate dark energy with the availability of vacuum energy or of a (nonzero) cosmological constant
in Einsteins equation (see Equation (1)). In Section 5.4, the effect of a cosmological constant
is achieved by making the effect of the continuous space expansions additive. This enables one to have different values for
, including varying
depending on the more detailed model of the development of the space curvature of our universe. Whether a more detailed analysis can lead to calculations that are in accordance with the observations is completely left open.
Phases in the formation of a black hole: Models of the Big Bang related processes (see [14] ) and of the process of QFT-interactions (see Section 6.1) assume a number of phases, which are partly comparable. An obvious idea is to apply the same phases to the process of the formation of a black hole, though in reverse order. This could eliminate the singularity problem with purely GRT- derived models. At a closer look, it becomes clear that simply reversing the process does not make sense. The major argument against a reverse Big Bang is the fact that the mentioned Big Bang phases are tightly connected to the expansion of space. The formation of a black hole, however, is not (according to the generally agreed upon theory) accompanied by a shrinking of the space (only by a shrinking of the space curvature).
Nevertheless, it may be worthwhile to look into the possible applicability of some of the features of the causal model of spacetime dynamics described in this paper. The major candidates seen by the author for the application to the black hole formation process are: (1) identification of phases in the formation of a black hole, (2) the assumption of discretized space and time, (3) treating the black hole as a quantum object, which implies among others a uniform (nonzero) proper time.
8. Conclusions
The causal model of QT/QFT described in [1] and [2] has been refined and extended to include aspects concerning spacetime and the dynamics (i.e., causal relationships) of spacetime evolution. The basis for the development of the causal model of QT/QFT (including spacetime aspects) has been GRT and the model described in [1] and [2] . The major assumption taken from GRT is the assumption that changes of the spacetime structure (e.g., space expansions and changes in curvature) always propagate with final speed (i.e., the speed of light), starting from definite local sources. As an assumption from the causal model of QT/ QFT, it is assumed that the initial source for the changes in the spacetime structure is the quantum objects. Large-scale changes and large-scale sources (e.g., large compact objects) are aggregations of the changes in collections of quantum objects.
Another major assumption from the causal model of QT/QFT is the assumption of discrete space and time. This assumption led to the inclusion of the concepts of the causal dynamical triangulation theory in the causal model for the evolution of spacetime described in this paper.
The major model characteristics derived from the above-described assumptions can be described by two items:
1) For the quantum object internal dynamics: The assumption that changes of the spacetime structure (expansion and curvature) starts at the quantum object means that quantum object internal processes are already executing within (dynamically changing) curved space. This enables new models for QFT processes such as scatterings (which play a major role in the overall causal model of QT/ QFT).
2) For the overall causal model of the world around the quantum objects: The mutual causal dependency of the spacetime structure on state changes of the quantum object (collections) and vice versa resulted in an overall causal model with very high spacetime dynamics. As an example, the model may imply an evolution of spacetime that is equivalent to the assumption of a (static or varying) cosmological constant. Implications on various open questions in the area of cosmology (such as dark energy) are obvious. These subjects, however, were not considered as the focal topic of this paper and are therefore only briefly discussed in Section 7 (Discussions).
A further conclusion of the work described in this paper is that models of physics areas which assume discreteness of the main parameters and causal models at a low level of detail, can only be partly evaluated and analyzed in terms of mathematical equations that are typical in traditional physics. Major analyses can only be performed by use of numerical computation and computer simulations. Under the assumption that the discrete model is the suitable (i.e., the more realistic) model, computer simulations are not a second-choice solution for solving complex (e.g., nonlinear) mathematical equations, but may even provide the more
accurate results. This is particularly true when the model contains functions that require (partly heuristic) algorithms rather than mathematical equations. The work described in this article has been accompanied by computer simulations from the very beginning.5
NOTES
1This article does not contain a proper definition of the used causal model specification language. The language used is assumed to be largely self-explanatory.
2Special relativity requests that the series of state changes does not occur with a speed which is faster than the speed of light. This requirement is not considered within the present article.
3The possible interpretation of QFT based on Minkowsky space leads to a number of open questions.
4Admittedly, this argument works both ways. The assumption of discreteness may also deviate from the realistic model.
5The figures shown have been generated as output of the computer simulations.