Transformation Formulas for the First Kind of Lauricella’s Function of Several Variables

Fadhle B. F. Mohsen¹, Ahmed Ali Atash², Hussein Saleh Bellehaj²

¹Department of Mathematics, Faculty of Education-Zingibar, Aden University, Aden, Yemen.
²Department of Mathematics, Faculty of Education-Shabwah, Aden University, Aden, Yemen.

DOI: 10.4236/jamp.2016.46115                

Abstract

Very recently Atash and Al-Gonah [1] derived two extension formulas for Lauricella’s function of the second kind of several variables and . Now in this research paper we derive two families of transformation formulas for the first kind of Lauricella’s function of several variables and with the help of generalized Dixon’s theorem on the sum of the series obtained earlier by Lavoie et al. [2]. Some new and known results are also deduced as applications of our main formulas.

Keywords

Transformation Formulas, Lauricella’s function, Dixon’s Theorem, Kampé de Fériet Function

Received 16 May 2016; accepted 24 June 2016; published 27 June 2016

1. Introduction

In 1994, Lavoie et al. [2] , obtained the following generalization of the classical Dixon’s theorem for the series: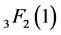

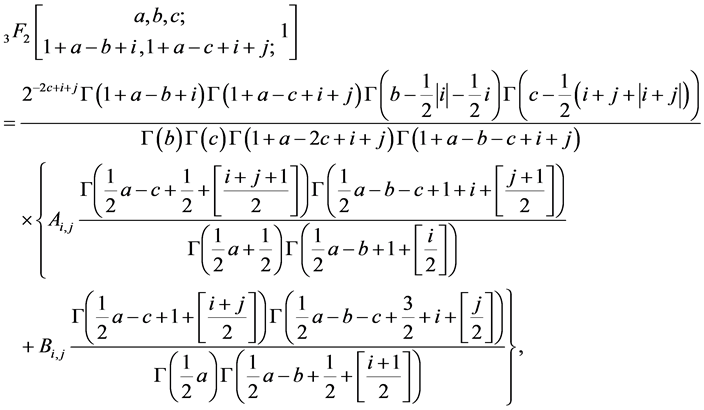(1.1)

,

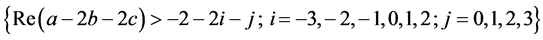where 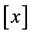denotes the greatest integer less than or equal to x and 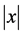denotes the usual absolute value of x. The coefficients 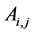and 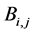are given respectively in [2] . When, (1.1) reduces immediately to the classical Dixon’s theorem [3] , (see also [4] )

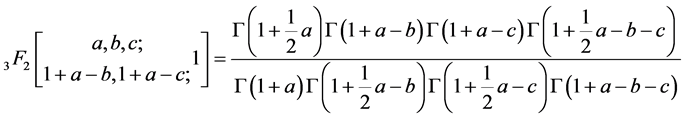(1.2)

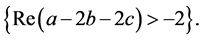
We recall that the first kind of the Lauricella hypergeometric function of -variables is defined as [5] :

(1.3)

,

where is the Pochhammer’s symbol defined by [5]

(1.4)

When, (1.3) reduces to the Lauricella function of 2r-variables

(1.5)

.

Clearly, we have, where F₂ is Appell’s double hypergeometric function [5]

(1.6)

Next, we recall that the generalized Lauricella function of several variables is defined as [5] :

(1.7)

where

(1.8)

the coefficients, ,;,; for all

are real and positive; abbreviates the array of A parameters; abbreviates

the array of parameters for all with similar interpretations for

and . Note that, when the coefficients in Equation (1.7) equal to 1, the genera-

lized Lauricella function (1.7) reduces to the following multivariable extension of the Kampé de Fériet function [5] :

(1.9)

where

. (1.10)

In our present investigation, we shall require the following results [5] :

(1.11)

(1.12)

(1.13)

(1.14)

(1.15)

(1.16)

2. Main Result

In this section, the following transformation formula will be established:

Theorem 2.1. For, the following formula for Lauricella’s function holds true:

(2.1)

where

(2.2)

(2.3)

(2.4)

The coefficients and can be obtained from the tables of A_i,j and B_i,j given in [2] by replacing a and c by and, also the coefficients and can be obtained from the same tables of A_i,j and B_i,j by replacing a and c by and respectively.

Proofs.

In order to prove the Theorem 2.1, let us first prove the following result:

(2.5)

To prove (2.5), denoting the left hand side of (2.5) by I, expanding in a power series as in (1.6) and using the result [5] :

,

we have

.

Now, using the elementary identities [5]

,

we have

.

This completes the proof of (2.5).

Proof of Theorem 2.1. Denoting the left hand side of (2.1) by S, expanding in a power series as in (1.3), adjusting the parameters, using the results (1.11) and (2.5) and by repeating this procedure r-times, we have

where

Now, separating into even and odd powers of by using the elementary identity [5]

,

we have

Finally, if we use the result (1.1), then we obtain the right hand side of the Theorem 2.1. This completes the proof of the Theorem 2.1.

Remark. Taking x = 0 in (2.1), we deduce the following formulas:

Corollary 2.1. For, the following formula for Lauricella’s function holds true:

(2.6)

3. Applications

1) In (2.1) if we take r = 1, then we get a known extension formulas [6] for Lauricella’s function of three variables for

2) In (2.1), if we take, we have

(3.1)

Now, in (3.1) if we use the results (1.12)-(1.16) and simplify, we obtain the following transformation formula:

(3.2)

which for, reduces to

(3.3)

3) Similarly, in (2.6), if we take, we have

(3.4)

which is a generalization of a known result of Bailey [7]

. (3.5)

Further, in (3.4) if we take, then we get

(3.6)

4. Conclusion

We conclude our present investigation by remarking that the main results established in this paper can be applied to obtain a large number of transformation formulas for the first kind of Lauricella’s function of several variables. Further, in the formulas (2.1) and (2.6), if we take, then we can obtain two new families of transformation formulas for Lauricella’s functions of several variables

and

for.

Conflicts of Interest

The authors declare no conflicts of interest.

References

[1]	Atash, A.A. and Al-Gonah, A.A. (2016) On Two Extension Formulas for Lauricella’s Function of the Second Kind of Several Variables. Journal of Applied Mathematics and Physics, 4, 571-577. http://dx.doi.org/10.4236/jamp.2016.43062
[2]	Lavoie, J.L., Grondin, F., Rathie, A.K. and Arora, K. (1994) Generalizations of Dixon’s Theorem on the Sum of a 3F2. Mathematics of Computation, 62, 267-276.
[3]	Bailey, W.N. (1935) Generalized Hypergeometric Series. Cambridge University Press, Cambridge.
[4]	Rainville, E.D. (1960) Special Functions. The Macmillan Company, New York.
[5]	Srivastava, H.M. and Manocha, H.L. (1984) A Treatise on Generating Functions. Halsted Press, New York.
[6]	Atash, A.A. (2015) Extension Formulas of Lauricella’s Functions by Applications of Dixon’s Summation Theorem. Applications and Applied Mathematics, 10, 1007-1018.
[7]	Bailey, W.N. (1953) On the Sum of Terminating . Quarterly Journal of Mathematics: Oxford Journals, 2, 237-240. http://dx.doi.org/10.1093/qmath/4.1.237