Optimization of the Angle of Frog in Mouldboard Tillage Operations in Sandy Clay Soil ()
Received 28 September 2015; accepted 8 December 2015; published 11 December 2015
1. Introduction
Tillage is a necessary action on soil to prepare favorable conditions for plant growth however, it is costly and time consuming [1] . In soil tilth preparation, primary tillage is considered as the largest power consumer operation [2] . For this reason tillage power optimization is still one of the main research fields [3] . Research has been carried out to optimize performance of soil implements and reduction of tillage power through various methods: optimization of tool geometry [4] -[6] and strip tillage [7] . Accurate modelling is necessary for the design of energy efficient soil implements in different operating conditions [8] . Draught force of a mouldboard plough is dependent on the plough geometry, soil properties and operation factors (cutting speed and depth) [5] .
Soil-cut interactions have been studied experimentally and analytically [5] [9] . Empirical formulas are also used to study soil-cut interactions however they are limited to relatively simple geometries of the working tool [10] . With the advancements in computer science, numerical methods are now used to predict draught forces. Numerical methods are further divided into [11] : Finite Element Model (FEM), Computational Fluid Dynamics (CFD) and Discrete Element Model (DEM). This study used DEM model as it considers soil failure, deformation and can handle large particle displacement.
[12] developed DEM for the study of rock mechanics. DEM describes mechanical behavior of granular materials through the study of the contact forces between finite number particles and their interactions hence suitable for modelling soil-cut interactions [13] . DEM allows for creation and breakage of contact between elements and the study of formation of cracks propagation involved in the field operation of a soil engaging implement. It also allows for the study of the relationship between micro and macro behavior. DEM has been in use in the past for various applications: [11] [14] -[18] . The objective of this paper is to optimize the design parameters of the mouldboard plough and in particular the frog angle. Altering the frog angle affects how the soil is cut and inverted impacting the draught forces. Numerical formula (DEM) was used and the results were compared to the analytical formula (Saunders Equation).
In contrast to the analytical formulas, use of DEM allows for the prediction of draught forces for complex tool geometrics hence optimizing performance for the mouldboard ploughs.
2. Development of Soil Interaction Model
2.1. EDEMTM Model
EDEMTM is a modeling platform in-built in DEM. It is based on the Hertz Mindlin contact force model and in particular the parallel particle bond model as shown in Figure 1. Equations (1) and (2) show the governing equations of the model.
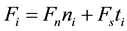(1)
(2)
The contact forces, normal force (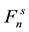
) and tangential force (
) shown in Equations (1) and (2) between particles are computed using the Hertz Mindlin contact law. A damping force is added to the normal damping force (
) and the tangential damping force (
) to show the viscous behavior. The contact forces are defined as functions of the normal and tangential stiffness (Kn and Kt), normal and tangential relative displacements. While the damping forces are determined as functions of the damping coefficient and the relative velocity as per [19] , the friction is well modeled using the Coulomb’s law of friction.
![]()
Figure 1. Schematic representation of the contacts.
Table 1 shows the global settings of the EDEMTM, the units and values of the different parameters. These parameters are found in the main window and are kept constant for all the iterations of simulation performed [20] .
2.2. Particle Modelling
The soil particles were remodeled in EDEM using optimal imaging techniques as shown in the below images. Figure 2 shows a pictorial representation of soil while Figure 3 shows the simulated soil particle.
A virtual soil bin was created using the EDEM as shown in Figure 4. The particles filled the soil bin at a rate of 3000 particles per second until the bin filled. The particles were set to be distributed in a log-normal manner and were placed randomly in the bin at a velocity of 20 m/s. the time step was set at 15 seconds with a time interval of 0.1 seconds as per Figure 5.
EDEMTM was calibrated using the angle of repose. Values of surface energy, coefficient of restitution, coefficient of static friction and coefficient of rolling were adjusted iteratively until the value of the angle of repose in simulation was close to the experimental value. Figure 6 shows how the angle of repose was measured in simulation. Macro mechanical strength parameters were determined using the standard tests e.g. the shear test.
![]()
Figure 2. Soil particles to be remodeled.
![]()
Figure 5. Particles dropping from the particle factory.
![]()
Figure 6. Slumped soil mass in EDEM showing the angle of repose.
2.3. Experimental
Verifications of the simulated results was carried out through experimental work and calculating the total draught force as per the Saunders Equation as explained below.
Saunders Equation
[5] [21] outlined the basis of this model. The model predicts draught force in a semi-rigorous manner. The equation calculated the draught force as a sum of all the forces acting on the plough point, the plough share, the force due to the mouldboard soil momentum change, increase in soil potential energy, friction forces and the lateral forces at the share, mouldboard and that due to the soil lateral movement. It considered soil parameters and plough geometric factors. Figure 7 is a diagrammatical representation of the different components attributing to the total draught force.
Equation (3) is quadratic equation that shows the relation between draft, speed, plough design characteristics and the soil conditions according to [5] [13] .
(3)
where:
Ht is the total draught force in KN.
Hp is the draught force due to plough point.
Hs is the draught force due to plough share.
Hmc is the draught force due to mouldboard soil momentum change and draught force friction along the mouldboard.
He is the draught force due to the increase in soil potential energy and the mouldboard
Hcs and Hms are the draught force arising from friction forces due to lateral forces at the share and at the mouldboard.
Hfs is the draught force arising from lateral forces at the mouldboard because of the lateral soil movements.
The above model aims at predicting the total plough draught forces in a semi rigorous manner. The constituents of Equation (3) were further broken down as shown in Equations (4)-(9).
(4)
![]()
(5)
![]()
Figure 7. Diagram of the components of the draught force acting on the plough.
![]()
(6)
![]()
(7)
![]()
(8)
![]()
(9)
3. Results and Discussion
EDEM simulation was performed by conducting iterations for each variable of frog angle, cutting depth and speed on the same type of soil. A VBA was developed and used to perform the rigorous mathematical Saunders equation. All the results were transferred to excel sheets for smoothening.
Sandy Clay soil was used and the soil parameters as determined by the shear test are outlined in Table 2.
Table 3 shows the parameters of the mould plough used. The mouldboard had only one plough.
The soil type was not varied. However, the speed, depth and frog angle varied as shown in Table 4.
The EDEM simulated total draught force results compared ± 15.95% to those determined through Saunders Equation.
3.1. Effect of Speed on Draught Force
The draught force increased as the speed increased. The relationship between draught force and speed was seen as 2nd degree polynomial quadratic equation.
*Soil soil friction angle was determined as Tan of the shearing resistance angle.
![]()
Table 3. Plough Geometric parameters.
![]()
Table 4. Assumed operating conditions for optimization.
*The depth of tillage was varied from 0.1 to 0.3 m with intervals of 0.1 m per range. **The speed of tillage was broadly divided into low speeds (≤2.5 m/s) and high speeds (2.5 m/s > 5 m/s). ***The frog angles used were 30˚, 40˚ and 50˚.
Figure 8 shows the behavior of draught force determined by EDEM simulation across all the frogs. Draught force increased as speed increased for frog 30˚ and 40˚. For frog 50˚ the draught force increased slightly and only picked at speeds higher than 4 m/s showing a more stable relationship with increase in speed.
Figure 9 shows the behavior of draught force determined by Saunders Equation across all the frogs. Draught force increased as speed increased across all the frog angles. At speeds above 3.25 m/s there was an increase of draught force of about 45.8% per each increase of 0.5 m/s of speed.
As the speed increased across the frog angles used, the draught force increased. The optimum speed of operation according to the results was 1.6 m/s which was agreeable with the various literature materials [8] [13] [15] .
3.2. Effect of Cutting Depth to Draught Force
As the depth increased the draught force also increased linearly. Figure 10 and Figure 11 are of draught force determined through Simulation (EDEM), and Saunders equation Vs. Depth of tillage respectively. The draught force in the graphs is of the three depths: 8 cm, 16.25 cm and 24.25 cm they show how draught force increase linearly as depth of tillage increases.
The two methods used to determine the draught force showed a linear relationship between the draught force and the depth. The depth of tillage is a determinant of the crop being planted.
4. Conclusions
A mathematical model (Saunders Equation) of a mouldboard was used to describe the draught force with emphasis on the different forces acting on the mouldboard parts contributing to the total draught force. DEM model was used to simulate the tillage process in a controlled environment. The simulations were iterated to achieve
![]()
Figure 8. Simulation draught force vs speed.
![]()
Figure 10. Simulation draught force vs depth.
![]()
Figure 11. Saunders equation draught force vs depth.
the optimal operating parameters of the plough. The draught forces determined by the Saunders Equation were verified through DEM simulation showing a variance of ±0.15.
Statistical analyses of the draught forces determined by the two methods showed there was minimal significant difference between the measured and simulated data. It was observed that the mouldboard required more draught force at higher speeds and cutting depth. At higher speeds the Saunders Equation was not able to describe draught force as reliably.
The results determined that DEM is an effective tool of determining draught force as it is fast and reliable. 30˚ frog angle was the optimum angle at a speed of 1.6 m/s. DEM predicted draught forces at this angle was in good agreement with the measured values with an error range of 7.6% to 14.5% for a speed range of 1.5 m/s to 1.8 m/s.
Acknowledgements
The authors respectfully acknowledge the support from the University of Nairobi support staff for the field work assistance. Sincere gratitude to the professional assistance I received from the Department of Environmental and Biosystems Engineering.
NOTES
![]()
*Corresponding author.