Magneto Hydrodynamics Stagnation Point Flow of a Nano Fluid over an Exponentially Stretching Sheet with an Effect of Chemical Reaction, Heat Source and Suction/Injunction ()
1. Introduction
Stagnation point is of considerable importance in most of industrial applications. The stagnation point is the one where fluid becomes stationary. In certain situations, flow is stagnated by a solid wall while in others; there is a line interior to a homogeneous fluid domain or the interface between two immiscible fluids [1] -[3] . A good amount of research is done drawing the attention of several researchers [4] -[14] .
A new kind of fluid known as Nano fluid is first time proposed in Argonne National Laboratory in US by Chai at the time of investigations on coolants techniques and cooling processes. The Nano fluids are of nanometre sized particles that are metals, oxides and nanotubes.
The properties of Nano fluids are of special importance over the base fluid since thermal conductivity and convective properties of the Nano fluid predominant over the properties of the base fluid. Thermal conductivity is observed to be more effectively enhanced in the range of 15% - 40% over the base fluid. Stagnation point flow is most significant in various fields of science and technology. The study of stagnation point flow was carried out by Hiemenz in 1911 [15] who concentrated his studies on the two-dimensional stagnation point problem. Magyari and Keller [16] investigated the steady boundary layers flow on a stretching continuous surface with exponential temperature distribution while Partha et al. and M. Shakhaoath Khan [17] [18] analysed the effects of viscous dissipation on the mixed convection heat transfer from an exponentially stretching surface. However, researchers are getting interested in studying the boundary layer flow of Nano fluids with different aspects [19] - [36] .
In the present study, we have investigated the viscous dissipation, heat source, suction for different values of velocity ratio parameter and observed that the nanoparticle volume decreases with the increase of chemical reaction parameter for γ = 0.1 and 2.1.
2. Mathematical Formulation
Consider a steady, two-dimensional boundary layer stagnation-point flow of a Nano fluid over an exponentially stretching sheet, the stretching and free stream velocities are assumed to be of the forms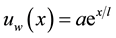
, and
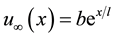, respectively. Where a > 0 and b are constants, x is the coordinate measure along the stretching surface and l is length of the sheet. A non-uniform transverse magnetic field of strength 
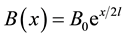is imposed parallel to the y-axis, where Bo is a uniform magnetic field strength. It is assumed that the induced magnetic field due to the motion of an electrically conducting field is negligible. Further it is also assumed that the external electric field is zero and the electrical field due to polarization of charges is negligible [22] .
Figure 1 shows that the temperature T and the Nano-particles fraction C take forms Tω(x) and Cω(x), respectively whereas the ambient values of temperature T∞ and Nano-particle fraction C∞ are obtained when y tends to infinity.
The governing boundary layer equations of the conservation Law of mass, momentum, energy and concentration in the flow as follows:
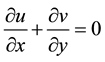(1)
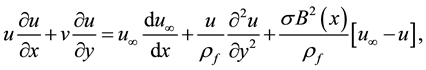(2)
![]()
Figure 1. Physical flow model and coordinate system.
(3)
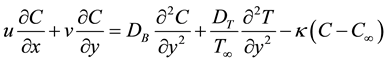(4)
Here u and v are the velocity components in the x and y directions respectively, µ is the viscosity, ρ is the
density of the base field and σ is the electrical conductivity.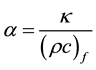 
where k is the thermal conductivity and 
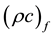is the heat capacitance of the base fluid and 
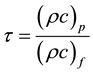where 
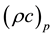is the heat capacitance of
the nanoparticle. DB is the Brownian diffusion coefficient, DT is the thermophoresis diffusion coefficient and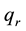 
is the radiation flux. The Rosseland approximation is defined as [23] [24] ;
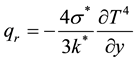(5)
where σ∗ is the Stefan-Boltzmann constant and k∗ is the mean absorption coefficient. It is assumed that the temperature difference between the free stream T∞ and local temperature T is small enough expanding T4 in a Taylor series about T∞ and neglecting higher order terms results;
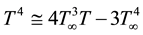. (6)
After substituting Equations (5) and (6) in Equation (3), it will be reduced to
![]()
(7)
the subjected boundary conditions are
![]()
. (8)
The prescribed temperature and concentration on the surface of the sheet is assumed to be ![]()
and ![]()
where T0, C0 are the reference temperature and concentration respectively, now, the non-linear partial differential equations for the purpose of a stream function ![]()
is defined as
![]()
(9)
where the continuity Equation (1) is satisfied identically. A similarity transformation is defined as [20] follows
![]()
(10)
as such Equation (10), Equations (2), (4) and (7) reduce to the following system of nonlinear ordinary differen- tial equations.
![]()
(11)
![]()
(12)
![]()
(13)
where
![]()
(14)
![]()
![]()
![]()
Here, prime denote the differentiation with respect to ![]()
is the velocity ratio parameter, ![]()
is the kinematic viscosity of the fluid, Pr is the Prandtl number, Le is the Lewis number, M is the magnetic parameter, A is the heat source parameter, S is the suction parameter.
![]()
where ![]()
is the radiation parameter.
![]()
where Nb is the Brownian motion parameter and Nt is the thermophoresis parameter, Ec Eckert number, γ is chemical reaction parameter; The corresponding boundary conditions Equation (8) are transformed into
![]()
. (15)
The parameters of practical interest in the formulated problem are velocity, heat and mass transfer respectively, which are presented in terms of Skin friction Cf, Nusselt number Nu and Sherwood numbers Sh. Using the transformed variables (10), the non-dimensional expressions for the Skin friction coefficient![]()
, the reduced Nusselt number ![]()
and the reduced Sherwood number ![]()
respectively are defined as;
Skin friction coefficient:
The Skin friction coefficient ![]()
is defined by
![]()
where τω is the local wall Shear stress, ρ is the fluid density.
![]()
Nusselt Number:
In heat transfer at a boundary within a fluid, the Nusselt number (Nu) is the ratio of convection to conductive heat transfer across the boundary
![]()
(16)
Sherwood Number:
The Sherwood number (Sh) is also called the mass transfer Nusselt number. It represents the ratio of convective to diffusive mass transport
![]()
where ![]()
is the local Reynolds number based on the stretching velocity.
3. Numerical Procedure
The equations (11)-(14) subject to the boundary conditions (15) are solved numerically using an implicit finite-difference scheme known as Keller box method. The method has the following four basic steps.
1) Reduce equations (11)-(14) to first order equations;
2) Write the difference equations using central differences;
3) Linearize the resulting algebraic equations by Newton’s method and write them in matrix-vector;
4) Use the Block-tridiagonal elimination technique to solve the linear system.
The system of ordinary differential equations (11)-(13) has been solved numerically using Keller-box method. From the numerical computation, the main physical quantities of interest namely the local skin friction coefficient, the local Nusselt number and the local Sherwood number are obtained and the results are presented in Table 1, Table 2 and Table 3.
From Table 2, it is observed that with the increase in heat source parameter, there is a decrease in rate of heat transfer and increase in mass transfer.
From Table 3, it is observed that with increase in suction parameter, there is a increase in rate of heat transfer, mass transfer and skin friction coefficient.
Figure 2 shows the effects of the suction parameter “s” on the flow field velocity f′(η) for three different values of the velocity ratio parameter B, B = 0.1, 1 and 2.
When B = 0.1 the velocity profile and the boundary layer thickness decrease with an increases in “s”. When s = 2.0, higher value of the Lorentz force further reduces the velocity and consequently the thickness of boundary
![]()
Table 1. Comparison of the reduced Nusselt number ![]()
when![]()
.
![]()
Figure 2. Velocity profile against η for different values of S.
layer reduces. When B = 1, there is no influence of source on f′(η) and f′(η) attains a constant value of “1” for any values of η indicating that there is no boundary layer of fluid, as shown by a thick (-) line in Figure 2.
Figure 3 and Figure 4 demonstrate the effects of suction parameter on temperature and concentration. It is clear that the fluid velocity decreases significantly with increasing values of suction parameter while it is found to enhance with blowing. The presence of suction would result in the reduction of the thickness of the boundary layer. So the Nano fluid temperature and concentration decreases with an increase in the suction parameter.
Figure 5 shows effects of the heat source parameter on temperature. Increasing the heat source parameter from −0.3 to 0.5 increases the temperature profile. Because the presence of source of heat enhances thermal energy, as a result of this, temperature profile increases. Figure 6 shows the heat source parameter significantly decreases the concentration profile.
Figure 7 and Figure 8 shows the effects of viscous dissipation parameter “Ec” on Temperature and concentration profile. Eckert number is the ratio of the kinetic energy of the flow to the boundary Layer enthalpy differ-
![]()
Figure 3. Temperature profile against η for different values of S.
![]()
Figure 4. Concentration profile against η for different values of S.
![]()
Figure 5. Temperature profile against η for different values of A.
ence. The effect of viscous dissipation on flow field is to increase the energy, yielding a greater fluid temperature and as consequence greater buoyancy force. The increase in the buoyancy force due to an increase in the dissipation parameter enhances the temperature. While concentration decreases with an increase in the viscous dissipation parameter.
The influence of chemical reaction parameter “γ” on concentration profile is shown in Figure 9. Concentration decreases with an increase in the chemical reaction parameter indicating that the nanoparticle volume fraction decreases with the increase of chemical reaction parameter, while effect of chemical reaction parameter γ is
![]()
Figure 6. Concentration profile against η for different values of A.
![]()
Figure 7. Temperature profile against η for different values of Ec.
![]()
Figure 8. Concentration profile against η for different values of Ec.
not significant on the temperature profile.
4. Conclusions
A numerical study corresponding to the flow and heat transfer in a steady flow region of Nano fluid over an exponential stretching surface and effects of heat source, suction parameter and Eckert number are examined and
![]()
Figure 9. Concentration profile against η for different values of γ.
discussed in detail. The main observation of the present study as follows:
1) It is interesting to note that an increase in the suction parameter reduces the velocity profile and increase the temperature and concentration;
2) An increase in the heat source parameter increases the temperature profile while it reduces the concentration profile;
3) An increase in the Eckert number increases the temperature profile while it reduces the concentration profile.
NOTES
*Corresponding author.