1. Introduction
Earlier Mielke and Romero [1] have shown that Cartan spacetime torsion [2] in the chiral anomaly induces a dynamical axion coupled with gravitation. This torsion-induced pseudo-scalar is given in such way that its gradient yields the torsion vector. Earlier Campanelli et al. [3] have investigated the primordial oscillation of axions and respective magnetic fields when CP-violating dynamos [4] in QCD are present. In early universe torsion effects are stronger than usual [3] which justifies the introduction of torsion in the dynamo equation [5] . In this work we consider QCD era and we are very far any galaxy formation. Therefore instead of the usual classical Maxwell electrodynamics non-minimally coupled with photon-torsion coupling in the realm of quan- tum electrodynamics (QED) we use early universe electrodynamics. In this paper a FRW universe is given as background for axion dynamo equation with torsion. It is shown that torsion oscillations enhance cosmic axion oscillations computed by Campanelli and Gianotti [4] . Primordial axion oscillations due to torsion yields a magnetic field of 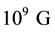 at Nucleosynthesis epoch. This is obtained due to a decay of BBN magnetic field of
at Nucleosynthesis epoch. This is obtained due to a decay of BBN magnetic field of 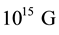 induced by torsion. In the last section of the paper a dynamo equation is obtained from axion scalar torsion string where huge magnetic fields are obtained from
induced by torsion. In the last section of the paper a dynamo equation is obtained from axion scalar torsion string where huge magnetic fields are obtained from 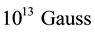 seed fields.
seed fields.
2. Axions, Photons and Torsion
Parity violation in gravity has been investigated recently by B Mukhopadhayaya, S Sen and Sur [6] which conclude that on a torsion-axion duality arising in a string scenario via Kalb-Ramond field leads to parity-
violating interactions for spin-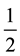 fermions. More recently the author [7] has investigated the role of parity vio-
fermions. More recently the author [7] has investigated the role of parity vio-
lation in torsion has been used to built dynamo equation. By analogy photon-axion coupling may happen giving rise to magnetic fields that eventually may be amplified giving rise to the 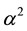 -dynamos addressed in the next section.
-dynamos addressed in the next section.
3. Efficiency of CP-Violating Dynamos
In this section we shall consider the solution the CP-violation dynamos and its efficiency on a torsion back- ground. Let us start by considering the dynamo equation as [1]
 (1)
(1)
where H is the Hubble parameter, a is the expansion of the universe, S represents the torsion vector and 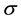 is the conductive. Here
is the conductive. Here 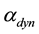
 (2)
(2)
the metric is given by
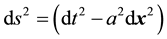 (3)
(3)
Here 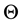 represents the axion field primordial field. The Fourier analysis of first equation becomes
represents the axion field primordial field. The Fourier analysis of first equation becomes
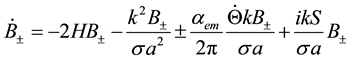 (4)
(4)
From this solution one notes that the torsion oscillating length is complex representing a true oscillation. Here k is the wave coherent scale number. The solution of this equation is
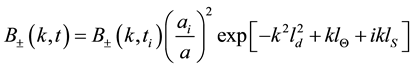 (5)
(5)
where the oscillation lengths are
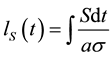 (6)
(6)
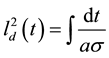 (7)
(7)
 (8)
(8)
Here ![]() is the dynamo length [2] . Here
is the dynamo length [2] . Here ![]() is the dissipation constant. Magnetic energy is then given by
is the dissipation constant. Magnetic energy is then given by
![]() (9)
(9)
The magnetic helicity contribution of torsion oscillation is
![]() (10)
(10)
Note that the magnetic helicity generation now has a contribution of the torsion oscillation length. Finally let us compute the torsion oscillation new term to compare it with the dynamo length to see if the torsion dissipative term may damp the dynamo length. Then
![]() (11)
(11)
when the argument is small this expression reduces to
![]() (12)
(12)
and for Big Bang Nucleosynthesis (BBN)
![]() (13)
(13)
where we have used the seed field for![]() . In the case of the seed field
. In the case of the seed field ![]() one obtains
one obtains
![]() (14)
(14)
which is able to seed galactic dynamos. Dynamo efficiency is given by![]() . This ratio reads
. This ratio reads
![]() (15)
(15)
where torsion field ![]() [5] and
[5] and![]() . Here we have used
. Here we have used![]() . Thus one may conclude that the torsion field is not high enough to avoid the dynamo efficiency of the dynamo by torsion damping of magnetic fields.
. Thus one may conclude that the torsion field is not high enough to avoid the dynamo efficiency of the dynamo by torsion damping of magnetic fields.
4. Dynamo Equation from Torsion Axion Anomalies
Earlier Duncan et al. [7] investigated axion hair anomalies in Riemann-Cartan spacetime where torsion was given by the gradient of axion scalar f. In this section instead of using the a dynamo equation of the previous one we shall addopt this approach and obtain a simpler dynamo equation which solution gives rise to stronger magnetic fields starting from the magnetic field of ![]() of the last section. The Maxwell equation obtained by Duncan et al. [7]
of the last section. The Maxwell equation obtained by Duncan et al. [7]
![]() (16)
(16)
where star in front of the Maxwell tensor F means that we are taking the dual of F given by![]() . Taking the approximation of Minkowski space plus torsion to emphasize the torsion role one obtains the dynamo equation as
. Taking the approximation of Minkowski space plus torsion to emphasize the torsion role one obtains the dynamo equation as
![]() (17)
(17)
By taking the scaled version of this equation in Fourier space one obtains
![]() (18)
(18)
which solution yields
![]() (19)
(19)
From this expression one sees that the torsion helicity sign ![]() together with the coupling constant l are fundamental to check for the efficiency of the dynamo; when torsion helicity is positive the magnetic field decays while when is negative dynamo effect is enhanced. By considering the approximation
together with the coupling constant l are fundamental to check for the efficiency of the dynamo; when torsion helicity is positive the magnetic field decays while when is negative dynamo effect is enhanced. By considering the approximation
![]() (20)
(20)
Here the coupling constant ![]() and
and ![]() is the axion decay constant. Taking the seed field as
is the axion decay constant. Taking the seed field as
high as in the next section ![]() one obtains for 1 kpc scale and a torsion field
one obtains for 1 kpc scale and a torsion field![]() . Taking again
. Taking again ![]() a magnetic field as high as
a magnetic field as high as![]() . Which upon substitution of l yields
. Which upon substitution of l yields ![]() Gauss which is one order of magnitude greater than the domain wall primordial magnetic field obtained by Cea and Tedesco [8] . Actually if one uses
Gauss which is one order of magnitude greater than the domain wall primordial magnetic field obtained by Cea and Tedesco [8] . Actually if one uses ![]() one obtains for the B-field
one obtains for the B-field ![]() which is a much stronger limit for the DW primordial magnetic field. This is extremely stronger than the limit found by Kisslinger from walls obtained from chiral phase transition [9] . Thus one may conclude that the strenght of the magnetic field shall depend upon the strenght of the coupling constant.
which is a much stronger limit for the DW primordial magnetic field. This is extremely stronger than the limit found by Kisslinger from walls obtained from chiral phase transition [9] . Thus one may conclude that the strenght of the magnetic field shall depend upon the strenght of the coupling constant.
5. Discussions and Conclusions
Torsion fields introduced in CP-violating cosmic axion ![]() -dynamos by the author [2] in order to obtain Lorentz violating bounds for torsion are revisited. These time oscillating axion solutions of the dynamo equation with torsion modes [3] are obtained taking into account dissipative torsion fields. Magnetic helicity torsion oscillatory contribution is also obtained. Note that the torsion presence guarantees dynamo efficiency when axion dynamo length is much stronger than the torsion length. Primordial axion oscillations due to torsion yields a magnetic field of
-dynamos by the author [2] in order to obtain Lorentz violating bounds for torsion are revisited. These time oscillating axion solutions of the dynamo equation with torsion modes [3] are obtained taking into account dissipative torsion fields. Magnetic helicity torsion oscillatory contribution is also obtained. Note that the torsion presence guarantees dynamo efficiency when axion dynamo length is much stronger than the torsion length. Primordial axion oscillations due to torsion yields a magnetic field of ![]() at Nucleosynthesis epoch. This is obtained due to a decay of BBN magnetic field of
at Nucleosynthesis epoch. This is obtained due to a decay of BBN magnetic field of ![]() induced by torsion [6] . Taking the Duncan et al. [7] torsion axion anomaly, the magnetic field strenght is huge depending on how strong is the coupling between torsion axions and photons.
induced by torsion [6] . Taking the Duncan et al. [7] torsion axion anomaly, the magnetic field strenght is huge depending on how strong is the coupling between torsion axions and photons.
Acknowledgements
We would like to express my gratitude to D. Sokoloff and A. Brandenburg for helpful discussions on the subject of this paper. I thank Prof. C. Sivaram for initiating me on the problem of dynamos and torsion. Financial support from CNPq. and University of State of Rio de Janeiro (UERJ) are grateful acknowledged.