Equivalent Martingale Measure in Asian Geometric Average Option Pricing ()
1. Introduction
Asian option, also known as the average price of options, was one of the derivatives of the stock options, and was firstly introduced by the American Bankers Trust Company (Bankers Trust) in Tokyo, Japan, on the basis of the lessons learned from the option implementations, such as real options, virtual options and stock options. It was a kind of exotic options, which was the most active one in financial derivative market, with the difference of the limitation of the exercise price from the usual stock option, that is, its exercise price was the average secondary market price of the stock price implemented during the current six months.
In this paper, after the Black-Scholes [1] Option Pricing Model was fully understood, the pricing of Asian options was discussed: It was assumed that the underlying asset price was driven by the geometric Brownian motion, that is, lognormal distribution. By using the random variables with the same Second moment driven by the lognormal distribution to approximate the arithmetic average of the underlying asset price, the approximate solution of the arithmetic average price of Asian put and call option with fixed exercise price was obtained, and the application of the equivalent martingale measure in the pricing of financial derivatives was further expanded [2] .
2. Model and Formulas
Generally, the stock market could be described as a probability space with a  -stream, that is,
-stream, that is, ; It was supposed that the market could meet the following conditions:
; It was supposed that the market could meet the following conditions:
(1) The market was an efficient frictionless market including two assets: one was the risk-free assets, known as the bonds, whose price process was denoted by ; another was the risky assets, called stocks, the price process was denoted by
; another was the risky assets, called stocks, the price process was denoted by . They satisfied the following formula separately:
. They satisfied the following formula separately:
 (1)
(1)
 (2)
(2)
where,  denotes the expectation of the yield rate,
denotes the expectation of the yield rate,  denotes firm-value process volatility, T denotes time to expiration of option, r denotes the risk-free interest rate and they all are constants.
denotes firm-value process volatility, T denotes time to expiration of option, r denotes the risk-free interest rate and they all are constants.  denotes the instantaneous increment of the Brownian motion under the probability measurer P at time t;
denotes the instantaneous increment of the Brownian motion under the probability measurer P at time t;
(2) Security trading is continuous and there are no transactions costs or taxes;
(3) There are no dividends to be payoff during options being held.
Definition 1 Let 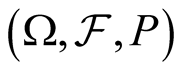 be a probability space and
be a probability space and  be an increasing chain of σ- fields spanning
be an increasing chain of σ- fields spanning , which
, which  and
and  be a standard Brownian motion.
be a standard Brownian motion.  be a σ- algebra of spanning
be a σ- algebra of spanning .
.
Let measures Q satisfy:

and set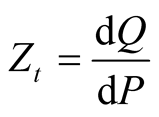 , we know that
, we know that  is a martingale since
is a martingale since . Then measure Q is defined a martingale measure equivalent to measure P, where
. Then measure Q is defined a martingale measure equivalent to measure P, where  denotes the expectation of random variable under probability measure P [3] .
denotes the expectation of random variable under probability measure P [3] .
Lemma 1 The dynamics of the share price under probability measure Q:
 where S denotes the share price now and
where S denotes the share price now and ,
, .
.
Prove Because the share price process satisfy the formula (1), using Ito’s theorem, we have:
 (3)
(3)
Then we get the dynamics of the share price under probability measure P:
 (4)
(4)
where  and
and .
.
Let , by definition 1 and Girsanov’s theorem we get that the random process
, by definition 1 and Girsanov’s theorem we get that the random process
 is a Brownian motion on
is a Brownian motion on  and:
and:
 (5)
(5)
 (6)
(6)
where  denotes the expectation of random variable in probability measure P and
denotes the expectation of random variable in probability measure P and  denotes the probability of random variable in measure P,
denotes the probability of random variable in measure P,  is an indicator function of set A. Substituting (5) into (3), we get:
is an indicator function of set A. Substituting (5) into (3), we get:

Thus we have that under probability measure Q:

The proof is completed.
Lemma 2 Let ,
,  ,
,  , then the distribution function of
, then the distribution function of  is:
is:

Definition 2 Using a bond as the denominated unit,  was the process of the discount factor, and
was the process of the discount factor, and  was the value process of discounted assets.
was the value process of discounted assets.
3. Asian Geometric Average Options Pricing
In general, for the contingent claim, the risk-neutral pricing principle [4] was obtained as followed.
Theorem 1 It was supposed that the market was arbitrage-free, so that the value of the process of any asset  at time
at time  was:
was:
 .
.
Considering one bearish Asian option, its return at expiration time was: , where,
, where,
 .
.
Under the conditions of arbitrage-free market and from Theorem 1, the price at time  was:
was:
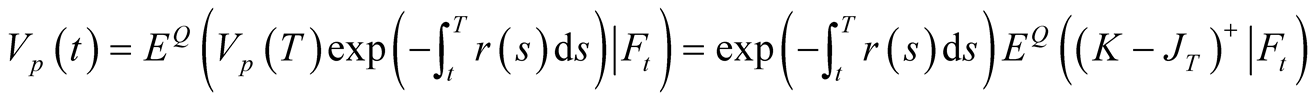
To get the specific expression of , the key was to obtain the distribution of
, the key was to obtain the distribution of  under the condition
under the condition
 . Made
. Made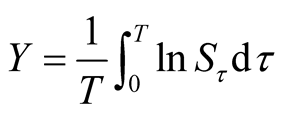 , then
, then , and:
, and:

Thereby,

Therefore,

Written as:
 ,
,
 Then,
Then,
 .
.
Theorem 2  was driven by the normal distribution
was driven by the normal distribution where,
where, .
.
Note: It could be deduced from the Lemma 1.
Theorem 3 It was supposed that the market was arbitrage-free, then the price of Asian put options 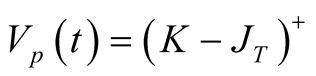 at any valid time
at any valid time  was:
was:

where, .
.
Prove

The proof was completed.
Similarly, the price of Asian call options  at any time
at any time  could be obtained:
could be obtained:

where, .
.
Deduction 1 For Asian geometric average options, the parity relationship between call option and put option was:
 .
.
Deduction 2 When the interest rate  and the volatility of stock returns
and the volatility of stock returns  were constant, there was:
were constant, there was:

Acknowledgements
Natural Research Foundation of Education Bureau of Hubei Province of China, NO. 20101202, Yichang Municipal Science and Technology Planning Project, NO. A2010-302-18.