Electromagnetic—Pure Gravity Connection via Hardy’s Quantum Entanglement ()
“Cosmology’s dark apparitions raise the question of whether Einstein’s theory is the right one”.
Jacob Bekenstein
1. Introduction
The extraordinary importance of Hardy’s quantum entanglement in clarifying a host of fundamental problems in theoretical physics and cosmology was researched extensively in the last three years [1] - [6] . Hardy’s result or rather theorem which states that the maximal probability of entanglement for two quantum particles is given by 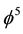 where
where 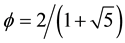 was confirmed by numerous theoretical analysis and experimental tests with very high accuracy [7] [8] . In addition this result was generalized within the framework of a fractal-Cantorian spacetime theory to encompass n particles leading to a simple general expression for quantum entanglement [8] [9] . The key point in obtaining and understanding these results is undoubtedly the surreal nature of the “Cantorian” points representing the space in question which is a physical realization of the mathematical E-infinity theory [10] [11] . A point of this KAM-like Cantorian space where zero measure thin fractals are interwoven with positive measure fat fractals [12] - [14] is impossible to locate. It is something of a geometrical topological “Scarlet Pimpernel” [15] for being here and there and everywhere simultaneously so to speak [8] [13] [16] . The physical mechanics behind this almost esoterically unreal quasi manifold is the fundamental fact of quantum entanglement [8] .
was confirmed by numerous theoretical analysis and experimental tests with very high accuracy [7] [8] . In addition this result was generalized within the framework of a fractal-Cantorian spacetime theory to encompass n particles leading to a simple general expression for quantum entanglement [8] [9] . The key point in obtaining and understanding these results is undoubtedly the surreal nature of the “Cantorian” points representing the space in question which is a physical realization of the mathematical E-infinity theory [10] [11] . A point of this KAM-like Cantorian space where zero measure thin fractals are interwoven with positive measure fat fractals [12] - [14] is impossible to locate. It is something of a geometrical topological “Scarlet Pimpernel” [15] for being here and there and everywhere simultaneously so to speak [8] [13] [16] . The physical mechanics behind this almost esoterically unreal quasi manifold is the fundamental fact of quantum entanglement [8] .
In what follows we will see how gauging pure gravity in terms of Hardy’s quantum entanglement leads directly to the exact theoretical value of the inverse electromagnetic fine structure constant introduced to fundamental physics by the teacher of both Wolfgang Pauli and Werner Heisenberg, namely Arnold Sommerfeld [3] [17] - [22] . The physical implication of this new result in the context of the unification program of the fundamental interactions as well as ordinary and dark energy is discussed in some details.
2. Hardy’s Self Entanglement of a Single Quantum Particle
The general formula for Hardy’s entanglement is given by the multiplication of two parts, the local 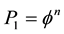 and the global
and the global  where n is the number of particles [8] . Thus from
where n is the number of particles [8] . Thus from
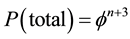 (1)
(1)
we find that for n = 2, Hardy’s celebrated result follows [8]
 (2)
(2)
where . On the other hand for a single particle we obviously have [8] [9] [23]
. On the other hand for a single particle we obviously have [8] [9] [23]
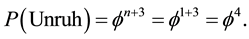 (3)
(3)
We note that this particular result is essentially a statement about the magnitude and nature of the dimensionless topological Unruh thermal temperature which a Rindler observer experiences [9] [13] . Once we have established the magnitude of the degrees of freedom of pure gravity in D = 8 super space of unification [22] [23] we will be gauging it in terms of the Hardy-Unruh 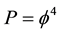 as mentioned in the introduction [8] [9] [23] . We also note that
as mentioned in the introduction [8] [9] [23] . We also note that 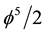 gives that exact value for the ordinary energy density of the cosmos while its Legendre transformation, i.e.
gives that exact value for the ordinary energy density of the cosmos while its Legendre transformation, i.e. 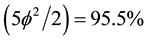 gives the measured cosmic dark energy density [1] - [6] .
gives the measured cosmic dark energy density [1] - [6] .
3. Pure Gravity in Unification Super Space
The degrees of freedom of the state of pure gravity, i.e. gravity in the absence of matter field are given dependently of the dimension d of spacetime by [24] [25]
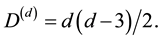 (4)
(4)
For d = 8 one finds
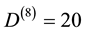 . (5)
. (5)
This happens to be numerically identical to the number of independent components of the Riemann tensor in four dimensional space. In the next section we will see how gauging D(8) in terms of P(Hardy) of oneself entangled quantum particle 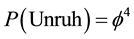 [8] [9] [12] will lead to the fundamental Sommerfeld “constant” [16] [17] .
[8] [9] [12] will lead to the fundamental Sommerfeld “constant” [16] [17] .
4. Electromagnetism from Pure Gravity via Quantum Entanglement
The electromagnetic fine structure constant is one of the most fundamental “constants” in nature and has a direct bearing on the existence and appearance of life and obviously on our entire range of modern technological civilization [22] . There are in the meantime numerous ways to derive the exact E-infinity value of the inverse fine structure constant  where
where 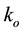 is a small irrational tail given by k = 0.082033925 which is directly related to Hardy’s quantum entanglement as we will see momentarily [2] - [9] . In fact
is a small irrational tail given by k = 0.082033925 which is directly related to Hardy’s quantum entanglement as we will see momentarily [2] - [9] . In fact ![]() could easily be reconstructed from the theoretical value of the electroweak couplings using the exact renormalization equation of E-infinity theory as follows [16] :
could easily be reconstructed from the theoretical value of the electroweak couplings using the exact renormalization equation of E-infinity theory as follows [16] :
![]() (6)
(6)
where![]() ,
, ![]() ,
, ![]() ,
, ![]() and
and![]() . Inserting in
. Inserting in ![]() one finds
one finds
![]() (7)
(7)
We note that ![]() and
and ![]() are very close indeed to the corresponding electroweak value. However
are very close indeed to the corresponding electroweak value. However ![]() of the strong interaction is on the other hand slightly off the experimental value due to the nature of quarks confinement. On the other hand
of the strong interaction is on the other hand slightly off the experimental value due to the nature of quarks confinement. On the other hand ![]() corresponding to quantum gravity Planck coupling does not enter into the conventional approximate renormalization equation of
corresponding to quantum gravity Planck coupling does not enter into the conventional approximate renormalization equation of![]() . In our present exact equation however
. In our present exact equation however ![]() is essential for the consistency of our theory. This can be seen from three subtle points. First the P-Adic expansion of
is essential for the consistency of our theory. This can be seen from three subtle points. First the P-Adic expansion of ![]() gives us the following T-duality relation between
gives us the following T-duality relation between ![]() weak range and
weak range and ![]() Planck range as is obvious from [26]
Planck range as is obvious from [26]
![]() (8)
(8)
where![]() ,
, ![]() and
and![]() . In other words [16] [26]
. In other words [16] [26]
![]() . (9)
. (9)
where T stands for Witten’s T-duality [23] . Second we know from previous fundamental results that the ultra high Planck energy Epl = 1019 Gev corresponds to a topological value ![]() which is nothing else but Hardy’s probability of quantum entanglement [13] [14] [23] . Our point became manifestly clear when we notice that the irrational tail in
which is nothing else but Hardy’s probability of quantum entanglement [13] [14] [23] . Our point became manifestly clear when we notice that the irrational tail in ![]() is given exactly by
is given exactly by
![]() (10)
(10)
Thus ![]() is the intersection of quantum entanglement
is the intersection of quantum entanglement ![]() as well as quantum disentanglement
as well as quantum disentanglement ![]() showing the deep involvement of
showing the deep involvement of ![]() with Hardy’s entanglement as well as the theory of prime numbers because of the remarkable exact value of
with Hardy’s entanglement as well as the theory of prime numbers because of the remarkable exact value of![]() , namely [16] [26]
, namely [16] [26]
![]() (11)
(11)
However this is by no means the only surprising aspect between the geometrical fusion and overcoming spacial separation by quantum entanglement and the attractive force of opposite charges of electromagnetic interaction. To show this we recall our earlier results regarding pure gravity in unification d = 8 super symmetric space and gauge the corresponding degrees of freedom in terms of a single quantum particle self entanglement, namely![]() . That way we find
. That way we find
![]() (12)
(12)
This is a remarkable result confirming a new aspect of the undeniable correctness of the unification program of high energy physics of quantum gravity [23] [25] and the dark energy mystery of cosmology [1] - [28] .
5. Conclusion
Unification is not only a simple and powerful idea which follows directly from Mach’s principle of Denk Economie taken to its ultimate but it is also a visible reality which can be inferred directly from the experimental facts of the standard model [9] [22] [25] . In the present short work we showed yet again the fundamental role of Hardy’s magnificent discovery of the exact value of probability of entanglement of two quantum particles [7] [8] [23] . Seen via the mental picture afforded by Hardy’s work, quantum entanglement [7] is a consequence of the transfinite fractal-Cantorian nature of quantum spacetime [1] [6] which glues the various fundamental interactions to a monolithic unity where electromagnetism and pure gravity are but two different sides of the same coin as amply demonstrated by the present new derivation of ![]() [3] [4] . In this sense we could extend the quotation of Prof. J. Bekenstein adorning the present work that Einstein’s pure gravity implicitly contains quantum mechanics and electromagnetism in a way which would have surprised even Einstein himself [27] [28] .
[3] [4] . In this sense we could extend the quotation of Prof. J. Bekenstein adorning the present work that Einstein’s pure gravity implicitly contains quantum mechanics and electromagnetism in a way which would have surprised even Einstein himself [27] [28] .