Interactive Fuzzy Programming for Random Fuzzy Two-Level Integer Programming Problems through Fractile Criteria with Possibility ()
1. Introduction
Decision making problems in hierarchical managerial or public organizations are often formulated as two-level mathematical programming problems [1,2]. In the context of two-level programming, the decision maker at the upper level first specifies a strategy, and then the decision maker at the lower level specifies a strategy so as to optimize the objective with full knowledge of the action of the decision maker at the upper level. In conventional multi-level mathematical programming models employing the solution concept of Stackelberg equilibrium, it is assumed that there is no communication among decision makers, or they do not make any binding agreement even if thereexists such communication [1,3-5]. Compared with this, for decision making problems in such as decentralized large firms with divisional independence, it is quite natural to suppose that there exists communication and some cooperative relationship among the decision makers [2].
For two-level linear programming problems or multilevel ones such that decisions of decision makers in all levels are sequential and all of the decision makers essentially cooperate with each other, Lai [6] and Shih et al. [7] proposed fuzzy interactive approaches. In their methods, the decision makers identify membership functions of the fuzzy goals for their objective functions, and in particular, the decision maker at the upper level also specifies those of the fuzzy goals for the decision variables. The decision maker at the lower level solves a fuzzy programming problem with a constraint with respect to a satisfactory degree of the decision maker at the upper level. Unfortunately, there is a possibility that their method leads a final solution to an undesirable one because of inconsistency between the fuzzy goals of the objective function and those of the decision variables. In order to overcome the problem in their methods, by eliminating the fuzzy goals for the decision variables, Sakawa et al. have proposed interactive fuzzy programming for two-level or multi-level linear programming problems to obtain a satisfactory solution for decision makers [8,9]. Extensions to two-level linear fractional programming problems [10], decentralized two-level linear programming problems [11,12], two-level linear fractional programming problems with fuzzy parameters [13], and two-level noncomvex programming problems with fuzzy parameters [14] were provided. Further extensions to two-level linear programming problems with random variables, called stochastic two-level linear programming problems [15,16], two-level integer programming problems [17], and twolevel linear programming problems involving fuzzy random variables, called fuzzy random two-level programming problems [18,19], have also been considered. It should be noted here that fuzzy random variables [20-22] are considered to be random variables whose realized values are not real values but fuzzy numbers or fuzzy sets. Arecent survey paper of Sakawa and Nishizaki [23] is devoted to reviewing and classifying the numerous major papers in the area of so-called cooperative multilevel programming.
On the other hand, from a viewpoint of ambiguity and randomness different from fuzzy random variables [20-22], by considering the experts’ ambiguous understanding of means and variances of random variables, a concept of random fuzzy variables was proposed, and mathematical programming problems with random fuzzy variables were formulated together with the development of a simulation-based approximate solution method [24].
Under these circumstances, as a first attempt to tackle decision making problems in hierarchical organizations under random fuzzy environments, assuming cooperative behavior of the decision makers, we have formulated random fuzzy two-level linear programming problems [25]. To deal with the formulated random fuzzy two-level linear programming problems, considering the probabilities that the decision makers’ objective function values are smaller than or equal to target variables, we introduce fuzzy goals of the decision makers for the probabilities. Then we adopt fractile criteria [26] to optimize the target variables under the condition that the degrees of possibility with respect to the attained probabilities are greater than or equal to certain permissible levels. Interactive fuzzy programming to obtain a satisfactory solution for the decision maker at the upper level in consideration of the cooperative relation between decision makers is presented [25].
However, in real-world decision making situations, it is often found that decision variables in random fuzzy two-level linear programming problems are not continuous but rather discrete. From such a viewpoint, in this paper, we formulate random fuzzy two-level integer programming problems as natural extensions of random fuzzy two-level linear programming problems with continuous variables [25]. Through fractile criteria with possibility, by considering the cooperative relation between decision makers, we present interactive fuzzy programming for random fuzzy two-level integer programming problems. Itis shown that all of the problems to be solved in the proposed interactive fuzzy programming become nonlinear integer programming problems and approximate optimal solutions can be obtained through the genetic algorithms with double strings for nonlinear integer programming. An illustrative numerical example is provided to demonstrate the feasibility and efficiency of the proposed method.
2. Random Fuzzy Variables
In the framework of stochastic programming, it is implicitly assumed that the uncertain parameter which well represents the stochastic factor of real systems can be definitely expressed as a single random variable. This means that the realized values of random parameters under the occurrence of some event are assumed to be definitely represented with real values.
Depending on the situations, however, it is natural to consider that the possible realized values of these random parameters are often only ambiguously known to the experts. In this case, it may be more appropriate to interpret the experts’ ambiguous understanding of the realized values of random parameters as fuzzy numbers. From such a point of view, a fuzzy random variable was first introduced by Kwakernaak [20], and its mathematical basis was constructed by Puri and Ralescu [21]. An overview of the developments of fuzzy random variables was found in the recent article of Gil et al. [27].
From the expert’s experimental point of view, however, the experts may think of a collection of random variables to be appropriate to express stochastic factors rather than only a single random variables. In this case, reflecting the expert’s conviction degree that each of random variables properly represents the stochastic factor, it would be quite reasonable to assign the different degrees of possibility to each of random variables. For handling such an uncertain parameter, a random fuzzy variable was defined by Liu [24] as a function from a possibility space to a collection of random variables, which is considered to be an extended concept of fuzzy variable [28]. It should be noted here that the fuzzy variables can be viewed as another way of dealing with the imprecision which was originally represented by fuzzy sets. Although we can employ Liu’s definition, for consistently discussing various concepts in relation to the fuzzy sets, we define the random fuzzy variables by extending not the fuzzy variables but the fuzzy sets.
Definition 1 (Random fuzzy variables) Let  be a collection of random variables. Then, a random fuzzy variable
be a collection of random variables. Then, a random fuzzy variable  is defined by its membership function
is defined by its membership function
 (1)
(1)
In Definition 1, the membership function 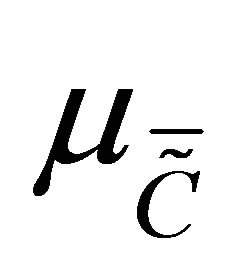 assigns each random variable
assigns each random variable  to a real number
to a real number . It should be noted here that if Γ is defined as
. It should be noted here that if Γ is defined as , then (1) becomes equivalent to the membership function of an ordinary fuzzy set. In this sense, a random fuzzy variable can be regarded as an extended concept of fuzzy sets. On the other hand, if
, then (1) becomes equivalent to the membership function of an ordinary fuzzy set. In this sense, a random fuzzy variable can be regarded as an extended concept of fuzzy sets. On the other hand, if  is defined as a singleton
is defined as a singleton  and
and , then the corresponding random fuzzy variable
, then the corresponding random fuzzy variable  can be viewed as an ordinary random variable.
can be viewed as an ordinary random variable.
When taking account of the imprecise nature of the realized values of random variables, it would be appropriate to employ the concept of fuzzy random variables. However, it should be emphasized here that if mean and/ or variance of random variables are specified by the expert as a set of real values or fuzzy sets, such uncertain parameters can be represented by not fuzzy random variables but random fuzzy variables.
As a simple example of random fuzzy variables, we consider a Gaussian random variable whose mean value is not definitely specified as a constant. For example, when some random parameter  is represented by the Gaussian random variable
is represented by the Gaussian random variable  where the expert identifies a set
where the expert identifies a set  of possible mean values as
of possible mean values as
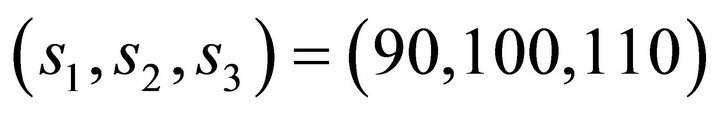 , if the membership function
, if the membership function  is defined by
is defined by

then  is a random fuzzy variable. More generally, when the mean values are expressed as fuzzy sets or fuzzy numbers, the corresponding random variable with the fuzzy mean is represented by a random fuzzy variable.
is a random fuzzy variable. More generally, when the mean values are expressed as fuzzy sets or fuzzy numbers, the corresponding random variable with the fuzzy mean is represented by a random fuzzy variable.
3. Random Fuzzy Two-Level Integer Programming Problems
As natural extensions of random fuzzy two-level linear programming problems with continuous variables [25], throughout of this paper, consider random fuzzy two-level integer programming problems. Realizing that the realworld decision making problems are often formulated as mathematical programming problems with integer decision variables, we consider the random fuzzy two-level integer programming problems formulated as
 (2)
(2)
where the two objective functions  and
and  are those of DM1 and DM2, respectively, and“
are those of DM1 and DM2, respectively, and“ ” and “
” and “ ” mean that DM1 and DM2 areminimizers for their objective functions. Moreover,
” mean that DM1 and DM2 areminimizers for their objective functions. Moreover,  is an
is an  dimensional integerdecision variable column vector for the decision maker at the upper level (DM1),
dimensional integerdecision variable column vector for the decision maker at the upper level (DM1),  is an
is an 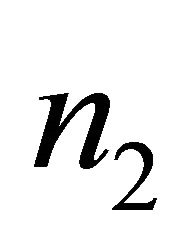 dimensional integer decision variable column vector for the decision maker at the lower level (DM2),
dimensional integer decision variable column vector for the decision maker at the lower level (DM2),  are
are  coefficient matrices,
coefficient matrices,  l = 1,2, are positive integer values, and
l = 1,2, are positive integer values, and  is an m dimensional column vector.
is an m dimensional column vector.
Observing that the real data with uncertainty are often distributed normally, from the practical point of view, we assume that each of  of
of 
 is the Gaussian random variable with fuzzy mean value
is the Gaussian random variable with fuzzy mean value  which is represented by an L-R fuzzy number characterized by the membership function
which is represented by an L-R fuzzy number characterized by the membership function
 (3)
(3)
where the shape functions L and R arenonincreasing continuous functions from  to
to ,
,  is the mean value, and
is the mean value, and  and
and 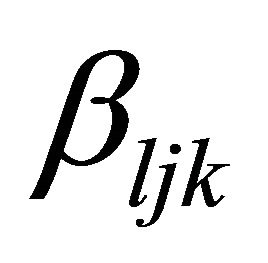 are positive numbers which represent left and right spreads. Figure 1 illustrates an example of the membership function
are positive numbers which represent left and right spreads. Figure 1 illustrates an example of the membership function .
.
Let Γ be a collection of all possible Gaussian random variables  where
where 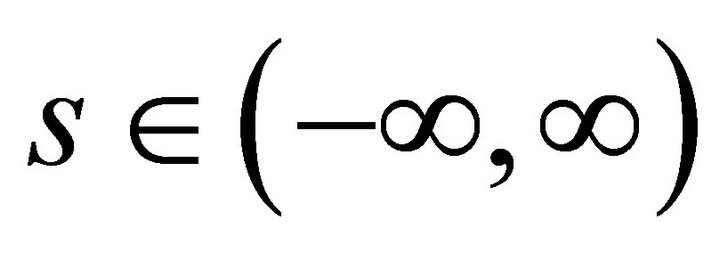 and
and 
 . Then,
. Then,  is expressed as a random fuzzy variable with the membership function
is expressed as a random fuzzy variable with the membership function
 (4)
(4)
Through the Zadeh’s extension principle, in view of (4), the membership function of a random fuzzy variable corresponding to each of objective functions 
 is given as
is given as

Figure 1. An example of the membership function .
.
 (5)
(5)
where ,
,
 , and
, and
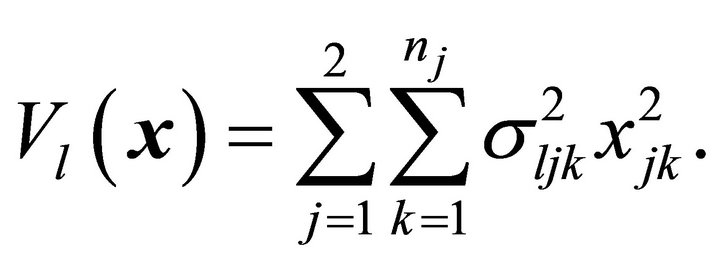
4. Fractile Criteria with Possibility Incorporating Fuzzy Goals
Assuming that the decision makers (DMs) concerns about the probabilities that their own objective function values  are smaller than or equal to certain target values
are smaller than or equal to certain target values , we introduce the probabilities
, we introduce the probabilities
 which are expressed as fuzzy sets
which are expressed as fuzzy sets
 with the membership functions
with the membership functions
 (6)
(6)
where  are the initial target values specified by the DMs as constants.
are the initial target values specified by the DMs as constants.
Considering the imprecise nature of the DMs’ judgments for the probabilities  with respect to the random fuzzy objective values
with respect to the random fuzzy objective values , we introduce the fuzzy goals
, we introduce the fuzzy goals  such as “
such as “ should be greater than or equal to a certain value.’’ Such fuzzy goals
should be greater than or equal to a certain value.’’ Such fuzzy goals  can be quantified by eliciting corresponding membership functions
can be quantified by eliciting corresponding membership functions
 (7)
(7)
where  are nondecreasing functions. Figure 2 illustrates a possible shape of the membership function for the fuzzy goal
are nondecreasing functions. Figure 2 illustrates a possible shape of the membership function for the fuzzy goal .
.
Recalling that the membership function is regarded as a possibility distribution, the degree of possibility that the probability  attains the fuzzy goal
attains the fuzzy goal  is expressed as
is expressed as
 (8)
(8)
Figure 3 illustrates the degree of possibility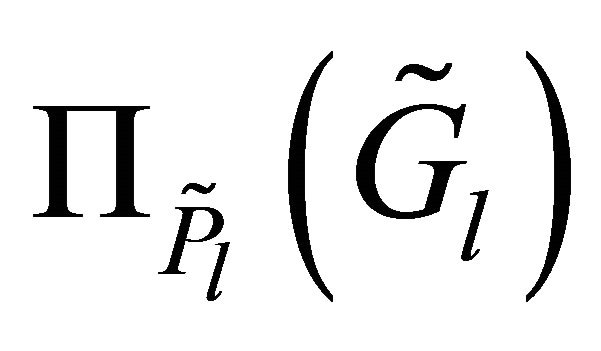 .
.
Now, assuming that the DMs are willing to maximize the degrees of possibility with respect to the attained probability, we consider the possibility-based probability model for random fuzzy two-level programming problems formulated as

Figure 2. An example of a membership function  of a fuzzy goal
of a fuzzy goal .
.
 (9)
(9)
or equivalently
 (10)
(10)
where  and
and  are permissible possibility levels specified by the DMs, and
are permissible possibility levels specified by the DMs, and  and
and 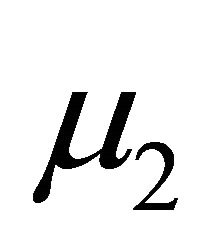 are the membership functions of the fuzzy goals for the target variables
are the membership functions of the fuzzy goals for the target variables  and
and , respectively.
, respectively.
It should be noted here that the bilevel programming problem (10) involves the possibility constraints  . Fortunately, however, the following theorem holds for the constraints
. Fortunately, however, the following theorem holds for the constraints 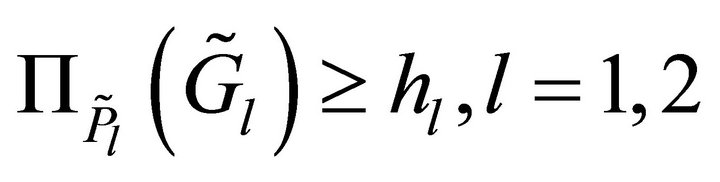 in (10).
in (10).
Theorem 1. Let  denote a probability distribution function of the standard Gaussian random variable N(0, 1). Then,
denote a probability distribution function of the standard Gaussian random variable N(0, 1). Then,  in (10) is equivalently transformed into
in (10) is equivalently transformed into

where  is a pseudo inverse functions defined as
is a pseudo inverse functions defined as  and
and  is the inverse function of
is the inverse function of .
.
Proof From (8), the constraints  in (10) is equivalently replaced by the condition that there exists a p such that
in (10) is equivalently replaced by the condition that there exists a p such that  and
and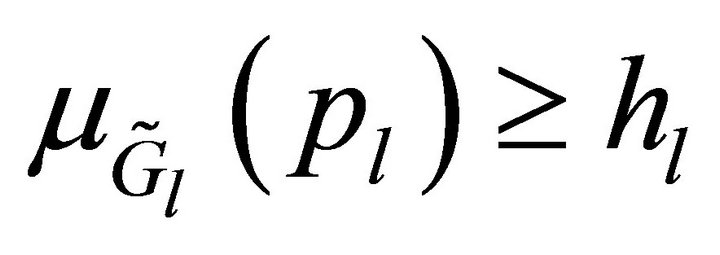 , namely,
, namely,
 (11)
(11)
and , where
, where  are pseudo inverse functions defined as
are pseudo inverse functions defined as
 . This implies that there exists a vector
. This implies that there exists a vector  such that
such that

 whichcan be equivalently transformed into the condition that there exists a vector
whichcan be equivalently transformed into the condition that there exists a vector  such that
such that

 . (12)
. (12)
In view of (3), it follows that

 where
where  and
and 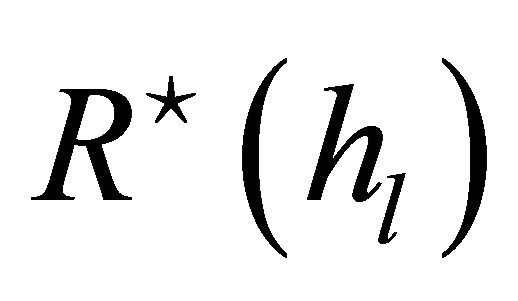 are pseudo inverse functions defined as
are pseudo inverse functions defined as and
and
 . Hence, (12) is rewritten as theequivalent condition that there exists a
. Hence, (12) is rewritten as theequivalent condition that there exists a  such that
such that
 ,
,
 (13)
(13)
Since  is transformed into
is transformed into

in consideration of

(13) isequivalently transformed as
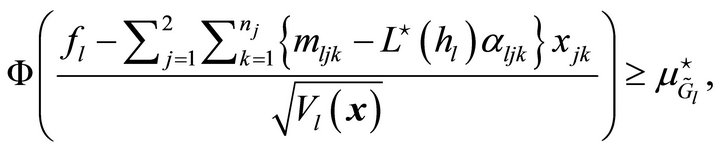 (14)
(14)
where  is a probability distribution function of the standard Gaussian randomvariable
is a probability distribution function of the standard Gaussian randomvariable .
.
From the monotone increasingnessof , (14) is rewritten as
, (14) is rewritten as
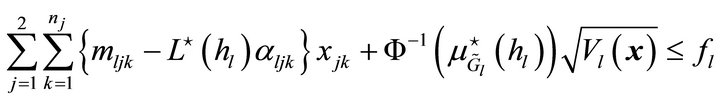 (15)
(15)
where  is the inverse function of
is the inverse function of .
.
From (11)-(15), it holds that
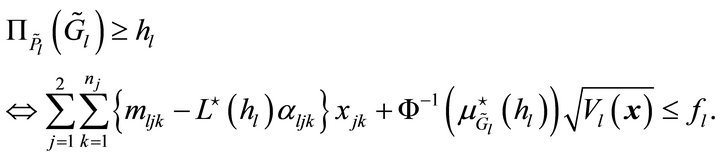 (16)
(16)
This completes the proof of the theorem.
Due to Theorem 1, the two-level integer programming problem with the possibility constraints (9) is equivalently transformed into (17)
or equivalently (18)
 (17)
(17)
 (18)
(18)
where
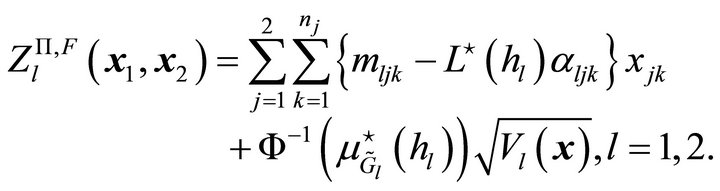 (19)
(19)
It should be emphasized here that (18) is a deterministic two-level nonlinear integer programming problem.
5. Interactive Fuzzy Programming
In order to obtain an initial candidate for an overall satisfactory solution to (9) or (17), it would be useful for DM1 to find a solution which maximize the smallerdegree of satisfaction between the two DMs by solving the maximin problem
 (20)
(20)
By introducing an auxiliary variable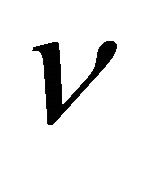 , this problem is written as
, this problem is written as
 (21)
(21)
Although the membership function does not always need to be linear, for the sake of simplicity, we adopt a linear membership function which characterizes the fuzzy goal of each decision maker. The linear membership functions  are defined as
are defined as
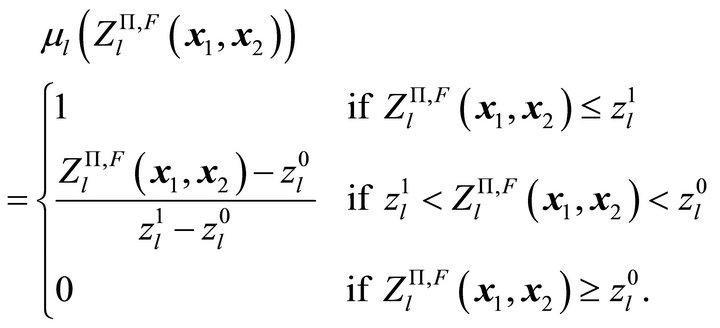 (22)
(22)
Then, (21) is equivalently transformed as (23)
If DM1 is satisfied with the membership function values , the corresponding opti mal solution
, the corresponding opti mal solution  to (21) is regarded as the satisfactorysolution. Otherwise, by introducing the constraint that
to (21) is regarded as the satisfactorysolution. Otherwise, by introducing the constraint that 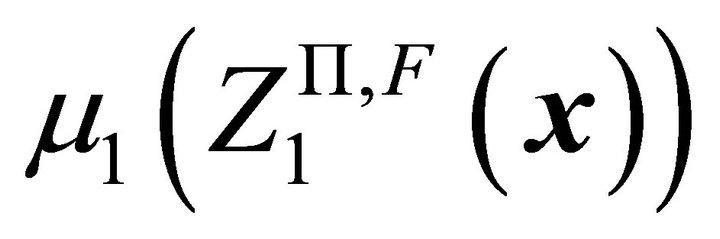 is larger than or equal to the minimal satisfactory level
is larger than or equal to the minimal satisfactory level  specified by DM1, we consider the problem of maximizing the membership function
specified by DM1, we consider the problem of maximizing the membership function formulated as
formulated as
 (24)
(24)
or equivalently (25)
In general, when the objective functions of DM1 and DM2 conflict with eachother, it should be noted here that the larger the minimal satisfactory level δ for  is specified by DM1, the smaller the satisfactory degree for
is specified by DM1, the smaller the satisfactory degree for  becomes, whichmay lead to the improper satisfac
becomes, whichmay lead to the improper satisfac (23)
(23)
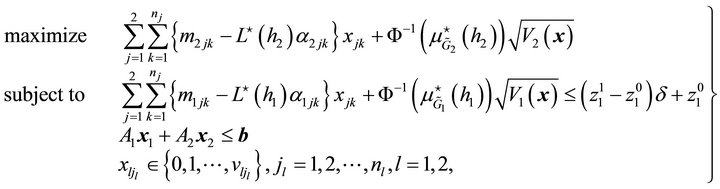 (25)
(25)
tory balance between DM1 and DM2 due to the large difference between the membership function values of both DMs.
In order to derive the satisfactory solution which has well-balanced membership function values between both DMs, by introducing the ratio Δ expressed as
 (26)
(26)
the lower bound  and the upper bound of
and the upper bound of  of
of , specified by DM1, are introduced to determine whether or not the ratio Δ is appropriate. To be more explicit, if it holds that
, specified by DM1, are introduced to determine whether or not the ratio Δ is appropriate. To be more explicit, if it holds that

then DM1 regards the corresponding solution as a preferable candidate for the satisfactory solution with wellbalanced membership function values.
Now we summarize a procedure of interactive fuzzy programming through fractile criteria with possibility in order to derive a satisfactory solution.
Interactive Fuzzy Programming through Fractile Criteria with Possibility
Step 0: Ask DMs to specify the initial target values , and determine the membership functions
, and determine the membership functions .
.
Step 1: Ask DM1 to specify the permissible possibility levels .
.
Step 2: Ask DMs to determine the membership functions .
.
Step 3: For the current , solve the maxmin problems (20).
, solve the maxmin problems (20).
Step 4: DM1 is supplied with the current values of the membership functions  and
and  for the optimal solution obtained in step 3. If DM1 is satisfied with the current membership function values, then stop. If DM1 is not satisfied and prefers to update
for the optimal solution obtained in step 3. If DM1 is satisfied with the current membership function values, then stop. If DM1 is not satisfied and prefers to update , ask DM1 to update
, ask DM1 to update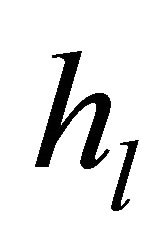 , and return to step 3. Otherwise, ask DM1 to specify the minimal satisfactory level
, and return to step 3. Otherwise, ask DM1 to specify the minimal satisfactory level for
for
 and the permissible range
and the permissible range 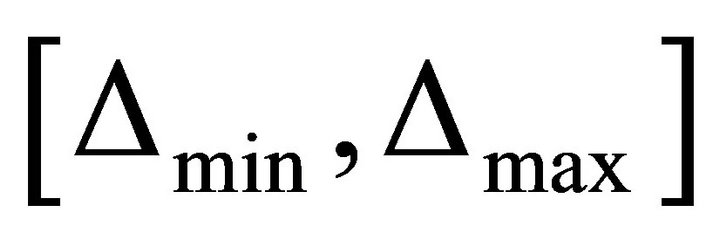
of the ratio .
.
Step 5: For the current minimal satisfactory level δ, solve the membership function maximization problem (25).
Step 6: DM1 is supplied with the current values of the membership function ,
,  and the ratio
and the ratio . If
. If  and DM1 is satisfied with the current membership function values, then stop. Otherwise, ask DM1 to update the minimal satisfactory level δ, and return to step 5.
and DM1 is satisfied with the current membership function values, then stop. Otherwise, ask DM1 to update the minimal satisfactory level δ, and return to step 5.
In the proposed interactive fuzzy programming method, it is required to solve the nonlinear integer programming problems (20) and (25), which is apparently difficult to solve compared to linear integer programming problems and 0-1nonlinear programming problems. In order to solve such difficult problems, in the following section, we introduce genetic algorithms designed for nonlinear integer programming problems.
6. Genetic Algorithms for Nonlinear Integer Programming
For solving linear integer programming problems on the framework of geneticalgorithms, Sakawa proposed GADSLPRRSU [29]. GADSLPRRSU is an abbreviation for genetic algorithms with double strings based on linear programming relaxation and reference solution updating. This method includes three key ideas: double strings (DS), linear programming relaxation (LPR), and reference solution updating (RSU). Unfortunately, however, due to nonlinearity, we cannot directly apply GADSLPRRSU for solving (20) and (25). However, we can introduce the revised GADSLPRRSU where GENOCOPIII [30,31] is employed for solving a nonlinear continuous relaxation problem.
As an efficient approximate solution method, the revised GADSLPRRSU are designed for nonlinear integer programming problems formulated as:
 (27)
(27)
where  is an
is an  dimensional integer decision variable column vector. Furthermore,
dimensional integer decision variable column vector. Furthermore,  and
and  may be nonlinear.
may be nonlinear.
Quite similar to genetic algorithms with double (GADS) [29], an individual is represented by a double string shown in Figure 4. In Figure 4, for a certain 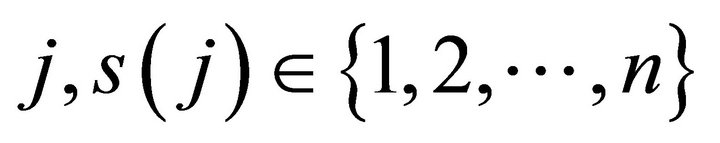 represents an index of decision variable
represents an index of decision variable  in the solution space, while
in the solution space, while  does the integer value among
does the integer value among 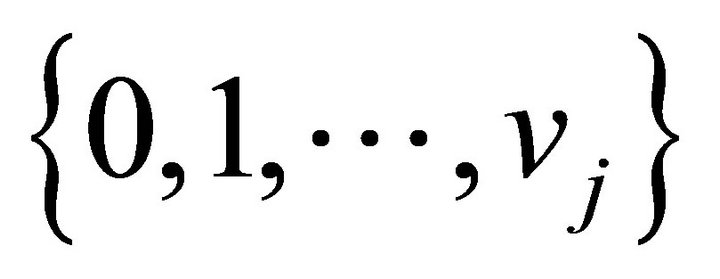 of the
of the 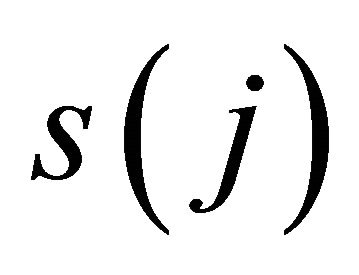 th decision variable
th decision variable .
.
Now we can summarize the computational procedures of the revised GADSLPRRSU as follows.
Computational Procedures of the Revised GADSLPRRSU
Step 0: Determine values of the parameters used in the genetic algorithm. Set the generation counter  at 0.
at 0.
Step 1: Generate the initial population consisting of
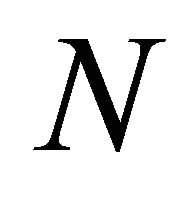 individuals based on the information of the optimal solution to the continuous relaxation problem.
individuals based on the information of the optimal solution to the continuous relaxation problem.
Step 2: Decode each individual in the current population and calculate its fitness based on the corresponding solution.
Step 3: If the termination condition is fulfilled, stop. Otherwise, let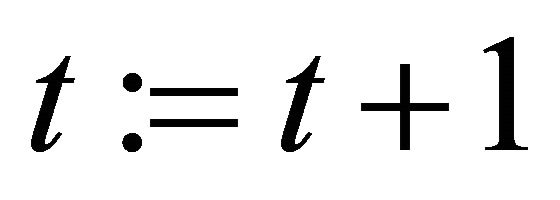 .
.
Step 4: Apply reproduction operator using elitist expected value selection after linear scaling.
Step 5: Apply crossover operator, called PMX (Partially Matched Crossover) for double string.
Step 6: Apply mutation based on the information of a solution to the continuous relaxation problem.
Step 7: Apply inversion operator, return to Step 2.
Further details of GADSLPRRSU and the revised GADSLPRRSU can be found in [17,29,32].
7. Numerical Example
To demonstrate the feasibility and efficiency of the proposed method, consider the following two-level integer programming problem involving random fuzzy variable coefficients:
 (28)
(28)
Table 1 shows values of coefficients of constraints  and
and  and Table 2 shows
and Table 2 shows