Further Results for General Financial Equilibrium Problems via Variational Inequalities ()
1. Introduction
In the previous papers [1] and [2], a general equilibrium model of financial flows and prices is considered. The model is assumed evolving in time. The equilibrium conditions are considered in dynamic sense and the governing variational inequality formulation is presented. Precisely, the variational inequality we are working with is the following one:


where  is the set of feasible assets and liabilities for each sector
is the set of feasible assets and liabilities for each sector  given by
given by

 is the total financial volume held by sector
is the total financial volume held by sector  as assets,
as assets,  is the total financial volume held by sector
is the total financial volume held by sector  as liabilities,
as liabilities,  is a measure of the risk of the financial agent,
is a measure of the risk of the financial agent,  is the tax rate levied on sector
is the tax rate levied on sector ’s net yield on financial instrument
’s net yield on financial instrument 
 is a nonnegative function,
is a nonnegative function,  is the portion of financial transaction per unit employed to cover the expenses of the financial institutions,
is the portion of financial transaction per unit employed to cover the expenses of the financial institutions,  is the set of feasible instrument prices given by
is the set of feasible instrument prices given by

where  and
and  are assumed to be in
are assumed to be in .
.
Setting, for the sake of simplicity,
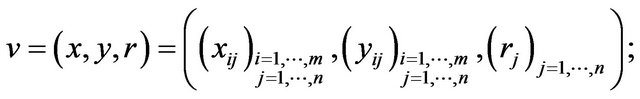

defined as:
 (1)
(1)
 (2)
(2)
then variational inequality (1) becomes the problem:

Variational inequality (1) proved to be a very useful tool which enables us to study the financial equilibrium of an economy evolving in time and in the previous papers [1] and [2] the authors provided an interesting and useful output as the deficit formula, a general balance law and the liability formula which could be of great importance for the theory of equilibrium problems evolving in time. For the reader’s convenience we recall such important formulas:
1) Deficit formula
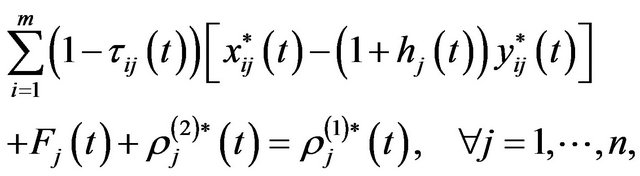
where  and
and  are the Lagrange variables associated to the price bounds:
are the Lagrange variables associated to the price bounds:

The meaning of  is that it represents the deficit per unit whereas
is that it represents the deficit per unit whereas  is the positive surplus per unit;
is the positive surplus per unit;
2) Balance law
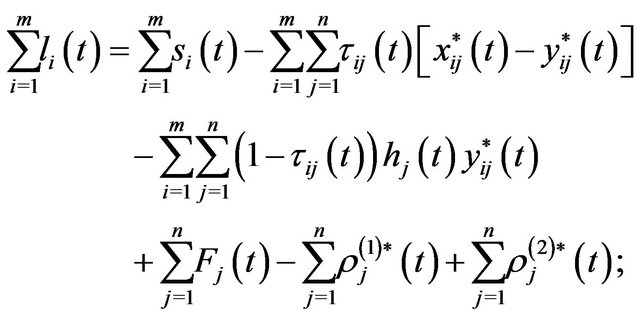
3) Liability formula
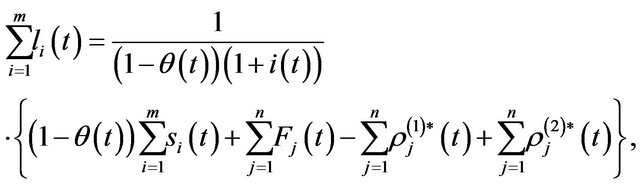
where  and
and  are the averages of
are the averages of  and
and
 , namely
, namely  and
and
 , respectively.
, respectively.
These suggested formulas could be of topical utility for the management of the world economy and to this aim in [2] the authors give some suggestions for the achievement of the world financial equilibrium and for finding the necessary way to follow in order to reach an improvement of the economy. It is worth reminding such suggestions:
Proposition 1.1 When the prices are minimal, namely they coincide with the floor prices, the economy collapses.
Proposition 1.2 Minimal prices imply the increase in the public debt.
Proposition 1.3 Minimal prices produce an economic recession. There is no incentive to the economic efforts.
Proposition 1.4 Even if it could be shocking, the development of the economy and of the employment results from an increase in the prices.
The value of these propositions can be realized by taking into account that the increase in prices indicated in Proposition 1.4 has been forecasted many months before (June 2011) it happened in our days.
Moreover, in [2] an “Evaluation Index”, that we denoted by , has been introduced as a useful and simple tool for the evaluation of an economy, given by
, has been introduced as a useful and simple tool for the evaluation of an economy, given by

where we set

We remark that if  is greater or equal to 1 the evaluation of the financial equilibrium is positive (better if
is greater or equal to 1 the evaluation of the financial equilibrium is positive (better if  is proximal to 1) whereas if
is proximal to 1) whereas if  is less than 1 the evaluation of the financial equilibrium is negative.
is less than 1 the evaluation of the financial equilibrium is negative.
The aim of this paper is to provide new theoretical and numerical results about solutions of financial equilibrium problems. In particular, we will prove a continuity result with respect to time of the solution, namely:
Theorem 1.1 Let , let
, let
 , let
, let  and let
and let
 be a strongly monotone mapnamely there exists
be a strongly monotone mapnamely there exists  such that, for
such that, for ,
,
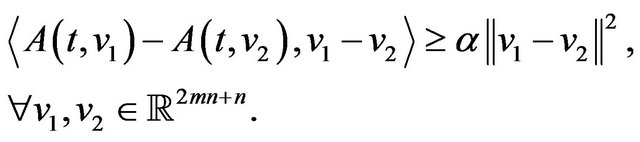
Then variational inequality (1) admits a unique continuous solution.
Furthermore, we prove the following Lipschitz continuity result:
Theorem 1.2 Let  be strongly monotone, namely there exists
be strongly monotone, namely there exists  such that, for
such that, for 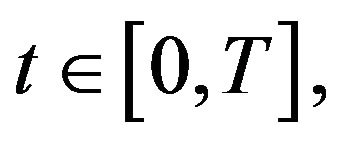
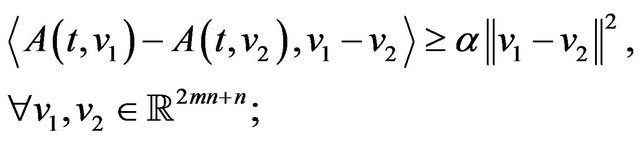
Lipschitz continuous with respect to , namely there exists
, namely there exists  such that, for
such that, for 

and Lipschitz continuous with respect to , namely there exists
, namely there exists  such that, for
such that, for 

Let  be two Lipschitz continuous functions and let
be two Lipschitz continuous functions and let  be two Lipschitz continuous functions. Then, the unique financial equilibrium solution
be two Lipschitz continuous functions. Then, the unique financial equilibrium solution  is Lipschitz continuous in
is Lipschitz continuous in . Moreover, let
. Moreover, let , the following estimate holds:
, the following estimate holds:
 (3)
(3)
where  and
and

The continuity and Lipschitz continuity of solutions to financial equilibrium problems is a very important property for applications. Indeed, it is fundamental to establish numerical approximate solutions. Such numerical solutions can be obtained making use of a modified version of the algorithms (see for instances [3-7]). It is worth remarking that the Lipschitz continuity allows us to calculate the error in approximating the solution.
In order to clearly illustrate theoretical results, some significant examples are provided and, in such a way, the impact that the components of the model have on the equilibrium are highlighted.
It is worth mensioning that even in this case variational inequalities are able to express the time-dependent equilibrium conditions. Then, applying delicate tools of nonlinear analysis (see [8-11]), it is possible to prove existence results and qualitative analysis. For other economic problems where the time plays an important role we refer to the papers devoted to the Walrasian equilibrium problem [12-15], to the oligopolistic market equilibrium problem [16,17], to the weighted traffic equilibrium problem [18,19], and to [20].
The paper is organized as follows. In Section 2 we present the general financial model. In Section 3 we study the continuity results of the solution to the variational inequality which characterizes the financial model. In Section 4 we provide a Lipschitz continuity result for the solution. Finally, in Section 5 we propose a numerical examples from which we deduce that the solution, computed by means of the direct method (see [21]), is Lipschitz continuous.
2. The Model
We consider a financial economy consisting of  sectors, with a typical sector denoted by
sectors, with a typical sector denoted by , and of
, and of  instruments, with a typical financial instrument denoted by
instruments, with a typical financial instrument denoted by , in the time interval
, in the time interval . Let
. Let  denote the total financial volume held by sector
denote the total financial volume held by sector  at time
at time  as assets, and let
as assets, and let  be the total financial volume held by sector
be the total financial volume held by sector  at time
at time  as liabilities. Then, unlike previous papers (see [22-27]), we allow markets of assets and liabilities to have different investments
as liabilities. Then, unlike previous papers (see [22-27]), we allow markets of assets and liabilities to have different investments  and
and  respectively. Since we are working in the presence of uncertainty and of risk perspectives, the volumes
respectively. Since we are working in the presence of uncertainty and of risk perspectives, the volumes  and
and  held by each sector cannot be considered stable with respect to time and may decrease or increase depending on unfavorable or favorable economic conditions. At time
held by each sector cannot be considered stable with respect to time and may decrease or increase depending on unfavorable or favorable economic conditions. At time , we denote the amount of instrument
, we denote the amount of instrument  held as an asset in sector
held as an asset in sector ’s portfolio by
’s portfolio by  and the amount of instrument
and the amount of instrument  held as a liability in sector
held as a liability in sector ’s portfolio by
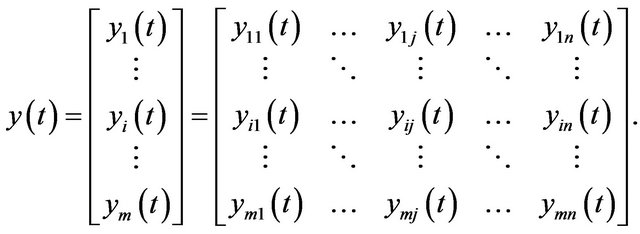
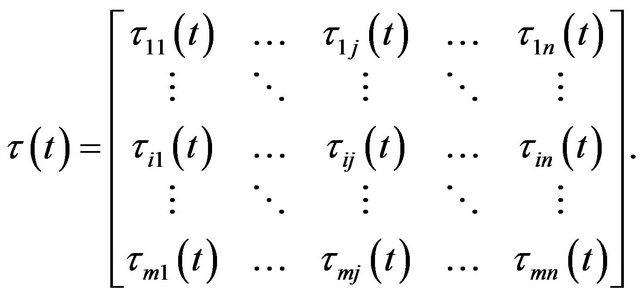
’s portfolio by . The assets and liabilities in all the sectors are grouped into the matrices
. The assets and liabilities in all the sectors are grouped into the matrices

and
We denote the price of instrument j held as an asset at time t by  and the price of instrument j held as a liability at time
and the price of instrument j held as a liability at time  by
by , where h is a nonnegative function defined into
, where h is a nonnegative function defined into  and belonging to
and belonging to . We introduce the term
. We introduce the term  because the prices of liabilities are generally greater than or equal to the prices of assets so that we can describe, in a more realistic way, the behaviour of the markets for which the liabilities are more expensive than the assets. In such a way, this paper appears as an improvement in various directions of the previous ones [22-27]. We group the instrument prices held as assets into the vector
because the prices of liabilities are generally greater than or equal to the prices of assets so that we can describe, in a more realistic way, the behaviour of the markets for which the liabilities are more expensive than the assets. In such a way, this paper appears as an improvement in various directions of the previous ones [22-27]. We group the instrument prices held as assets into the vector
 and the instrument prices held as liabilities into the vector
and the instrument prices held as liabilities into the vector

In our problem the prices of each instrument appear as unknown variables. Under the assumption of perfect competition, each sector will behave as if it has no influence on the instrument prices or on the behaviour of the other sectors.
In order to express the time-dependent equilibrium conditions by means of an evolutionary variational inequality, we choose as a functional setting the very general Lebesgue space . Then, the set of feasible assets and liabilities for each sector
. Then, the set of feasible assets and liabilities for each sector , is
, is 
Now, in order to improve the model of competitive financial equilibrium described in [1], we consider the possibility of policy interventions in the financial equilibrium and incorporate them in form of taxes and price controls.
To this aim, denote the ceiling price associated with instrument  by
by  and the nonnegative floor price associated with instrument
and the nonnegative floor price associated with instrument  by
by , with
, with
 , a.e. in
, a.e. in . The meaning of the constraint
. The meaning of the constraint  a.e. in
a.e. in  is that to each investor a minimal price
is that to each investor a minimal price  for the assets held in the instrument
for the assets held in the instrument  is guaranteed, whereas each investor is requested to pay for the liabilities not less than the minimal price
is guaranteed, whereas each investor is requested to pay for the liabilities not less than the minimal price . Analogously each investor cannot obtain for an asset a price greater than
. Analogously each investor cannot obtain for an asset a price greater than  and as a liability the price cannot exceed the maximum price
and as a liability the price cannot exceed the maximum price .
.
Denote the given tax rate levied on sector ’s net yield on financial instrument
’s net yield on financial instrument , as
, as . Assume that the tax rates lie in the interval
. Assume that the tax rates lie in the interval  and belong to
and belong to  . Therefore, the government in this model has the flexibility of levying a distinct tax rate across both sectors and instruments.
. Therefore, the government in this model has the flexibility of levying a distinct tax rate across both sectors and instruments.
Let us group the instrument ceiling prices  into the column vector
into the column vector , the instrument floor prices
, the instrument floor prices  into the column vector
into the column vector
 , and the tax rates
, and the tax rates  into the matrix
into the matrix
The set of feasible instrument prices is 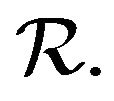
In order to determine for each sector  the optimal composition of instruments held as assets and as liabilities, we consider, as usual, the influence due to riskaversion and the process of optimization of each sector in the financial economy, namely the desire to maximize the value of the asset holdings and to minimize the value of liabilities. Then, we introduce the utility function
the optimal composition of instruments held as assets and as liabilities, we consider, as usual, the influence due to riskaversion and the process of optimization of each sector in the financial economy, namely the desire to maximize the value of the asset holdings and to minimize the value of liabilities. Then, we introduce the utility function , for each sector
, for each sector , in this way
, in this way
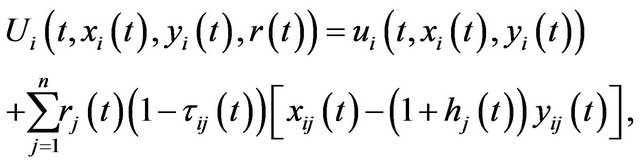
where the term  represents a measure of the risk of the financial agent and
represents a measure of the risk of the financial agent and
 represents the value of the difference between the asset holdings and the value of liabilities. We suppose that the sector’s utility function
represents the value of the difference between the asset holdings and the value of liabilities. We suppose that the sector’s utility function  is defined on
is defined on
 , is measurable in
, is measurable in  and is continuous with respect to
and is continuous with respect to  and
and . Moreover we assume that
. Moreover we assume that  and
and  exist and that they are measurable in
exist and that they are measurable in  and continuous with respect to
and continuous with respect to  and
and . Further, we require that
. Further, we require that 
 and a.e. in
and a.e. in  the following growth conditions hold true:
the following growth conditions hold true:
 (4)
(4)
and
 (5)
(5)
where ,
,  ,
,  are non-negative functions of
are non-negative functions of
 . Finally, we suppose that the function
. Finally, we suppose that the function
 is concave.
is concave.
In order to determine the equilibrium prices, we establish the equilibrium condition which expresses the equilibration of the total assets, the total liabilities and the portion of financial transactions per unit  employed to cover the expenses of the financial institutions including possible dividends, as in [1]. Hence, the equilibrium condition for the price
employed to cover the expenses of the financial institutions including possible dividends, as in [1]. Hence, the equilibrium condition for the price  of instrument
of instrument  is the following:
is the following:
 (6)
(6)
In other words, the prices are determined taking into account the amount of the supply, the demand of an instrument and the charges , namely if there is an actual supply excess of an instrument as assets and of the charges
, namely if there is an actual supply excess of an instrument as assets and of the charges  in the economy, then its price must be the floor price. If the price of an instrument is greater than
in the economy, then its price must be the floor price. If the price of an instrument is greater than , but not at the ceiling, then the market of that instrument must clear. Finally, if there is an actual demand excess of an instrument as liabilities and of the charges
, but not at the ceiling, then the market of that instrument must clear. Finally, if there is an actual demand excess of an instrument as liabilities and of the charges  in the economy, then the price must be at the ceiling.
in the economy, then the price must be at the ceiling.
Now, we can give different but equivalent equilibrium conditions, each of which is useful to illustrate particular features of the equilibrium.
Definition 2.1 A vector of sector assets, liabilities and instrument prices  is an equilibrium of the dynamic financial model if and only if
is an equilibrium of the dynamic financial model if and only if 
 and a.e. in
and a.e. in  it satisfies the system of inequalities
it satisfies the system of inequalities
 (7)
(7)
 (8)
(8)
and equalities
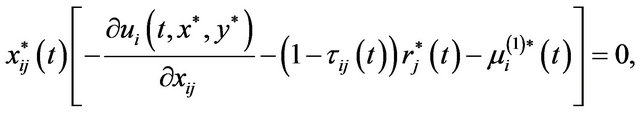
(9)
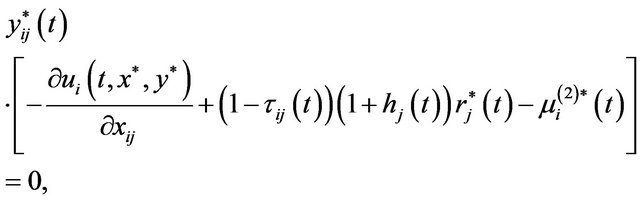
(10)
where  are Lagrange functions, and verify conditions (6) a.e. in
are Lagrange functions, and verify conditions (6) a.e. in .
.
Let us explain the meaning of the above conditions. To each financial volumes  and
and  held by sector
held by sector , we associate the functions
, we associate the functions , related, respectively, to the assets and to the liabilities and which represent the “equilibrium disutilities” per unit of the sector
, related, respectively, to the assets and to the liabilities and which represent the “equilibrium disutilities” per unit of the sector . Then, (7) and (9) mean that the financial volume invested in instrument
. Then, (7) and (9) mean that the financial volume invested in instrument  as assets
as assets  is greater than or equal to zero if the
is greater than or equal to zero if the  -th component
-th component
 of the disutility is equal to
of the disutility is equal to , whereas if
, whereas if
 , then
, then
 . The same occurs for the liabilities and the meaning of (6) is already illustrated.
. The same occurs for the liabilities and the meaning of (6) is already illustrated.
The functions  and
and  are Lagrange functions associated a.e. in
are Lagrange functions associated a.e. in  with the constraints
with the constraints
 and
and , respectively. They are unknown a priori, but this fact has no influence because we will prove in the following theorem that Definition is equivalent to a variational inequality in which
, respectively. They are unknown a priori, but this fact has no influence because we will prove in the following theorem that Definition is equivalent to a variational inequality in which  and
and  do not appear.
do not appear.
Theorem 2.1 A vector  is a dynamic financial equilibrium if and only if it satisfies variational inequality (1).
is a dynamic financial equilibrium if and only if it satisfies variational inequality (1).
Moreover, we recall the result about Lagrange multipliers (see [2]):
Theorem 2.2 Let 
be a solution to variational inequality (1). Then there exist
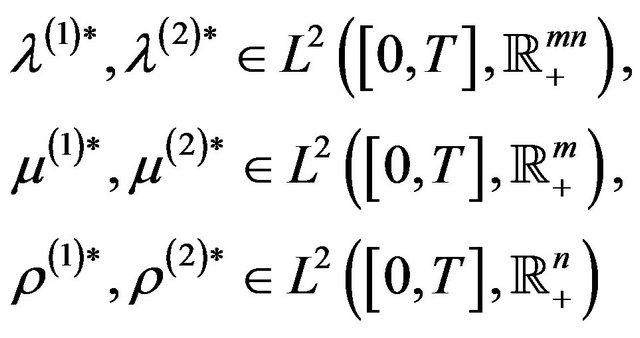
such that a.e. in ,
,
 (11)
(11)
 (12)
(12)
 (13)
(13)
 (14)
(14)
3. Continuity Results for Financial Equilibrium Solutions
In order to show the continuity result for the financial equilibrium problem, first of all, let us recall the wellknown property of set convergence due to K. Kuratowski (see [28]), that is a generalization of the classical Hausdorff definition of a metric for the space of closed subsets of a (compact) metric space.
Let  be a metric space and let
be a metric space and let  be a sequence of subsets of
be a sequence of subsets of . Recall that
. Recall that


where eventually means that there exists  such that
such that  for any
for any , and frequently means that there exists an infinite subset
, and frequently means that there exists an infinite subset  such that
such that  for any
for any . Finally we can remind the set convergence in Kuratowski’s sense.
. Finally we can remind the set convergence in Kuratowski’s sense.
Definition 3.1 We say that  converges to some subset
converges to some subset  in Kuratwoski’s sense, and we briefly write
in Kuratwoski’s sense, and we briefly write , if
, if . Thus, in order to verify that
. Thus, in order to verify that , it suffices to check that
, it suffices to check that
(K1) , i.e. for any
, i.e. for any , there exists a sequence
, there exists a sequence  converging to x in X such that
converging to x in X such that  lies in
lies in  for all
for all ;
;
(K2) , i.e. for any subsequence
, i.e. for any subsequence
 converging to x in X, such that
converging to x in X, such that  lies in
lies in 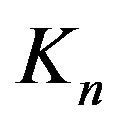
for all , then the limit
, then the limit  belongs to
belongs to .
.
Now, let us prove that the set of feasible vectors satisfies the property of the set convergence in Kuratowski’s sense.
Proposition 3.1 Let , let
, let
 , let
, let  and let
and let
 be a sequence such that
be a sequence such that , as
, as . Then, the sequence of sets
. Then, the sequence of sets
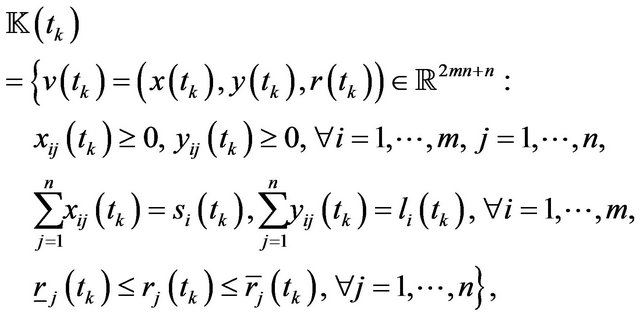
 , converges to
, converges to

as , in Kuratowski’s sense.
, in Kuratowski’s sense.
Proof In order to prove that the sequence  converges to
converges to  in Kuratowski’s sense, for any sequence
in Kuratowski’s sense, for any sequence  such that
such that , as
, as , it is enough to show that conditions (K1) and (K2) hold.
, it is enough to show that conditions (K1) and (K2) hold.
Let  be fixed and let us consider the sequence
be fixed and let us consider the sequence
 , such that
, such that
 ,
,  ,
,  ,
,

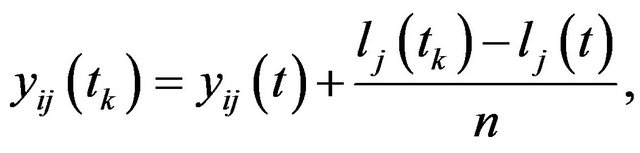
and ,
,  ,
,

Let us verify that ,
, . Taking into account that
. Taking into account that
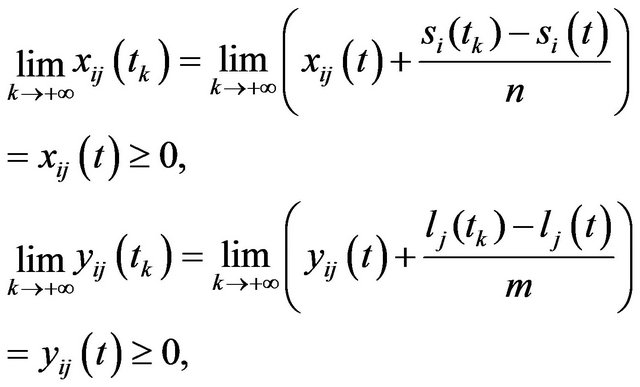
there exist two index  and
and  such that for
such that for  we get
we get

and for  we have
we have
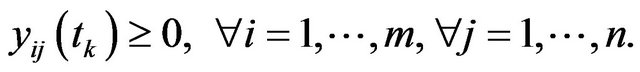
Since ,
,
 , it results
, it results ,
, . Moreoverbeing
. Moreoverbeing
 ,
,
 , it follows
, it follows ,
, . Finally, it results
. Finally, it results

Then we can consider a sequence  such that for
such that for ,
,  ,
,  ,
,
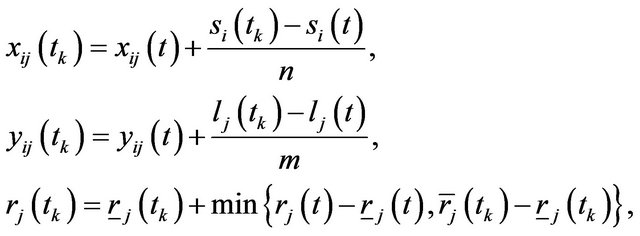
and for ,
,  ,
,  ,
,
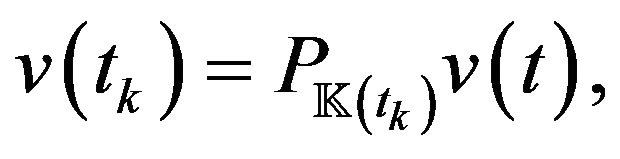
where  denotes the Hilbertian projection on
denotes the Hilbertian projection on .
.
We have  and for
and for 
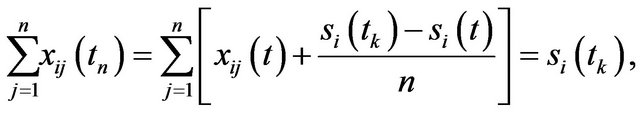
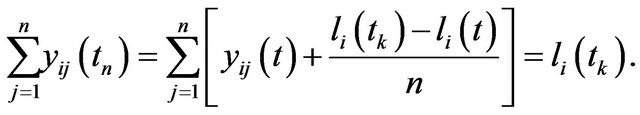
Then the first condition has been shown.
For the second one, let  be a fixed sequence, with
be a fixed sequence, with ,
,
 , such that
, such that  in
in ,
,
 in
in ,
,  in
in . We want to prove that
. We want to prove that . Since
. Since
 ,
,  , it results
, it results
 (15)
(15)
 (16)
(16)
 (17)
(17)
Passing to the limit as  in (15), (16) and (17), we obtain
in (15), (16) and (17), we obtain

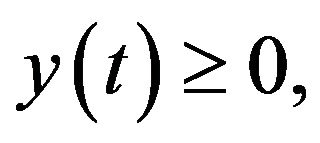
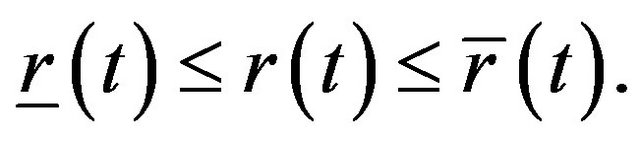
Then .
.
The claim is, now, achieved.□
For what follows, it is convenient to recall that variational inequality (1) can be rewritten in the equivalent parameterized form:
 (18)
(18)
where the constraint set ,
,  , is a closed convex and nonempty subset of
, is a closed convex and nonempty subset of ,
,
 is a mapping and
is a mapping and  denotes the scalar product in
denotes the scalar product in . Moreover, we recall that under general assumptions existence theorems have been proved in [2] (see Section 6).
. Moreover, we recall that under general assumptions existence theorems have been proved in [2] (see Section 6).
Taking into account the general continuity result for solutions to parameter variational inequalities in reflexive Banach spaces (see [29], Theorem 4.1) and Proposition, we obtain Theorem 1.1 of Section 1.
Theorem 1.1 Let , let
, let
 , let
, let  and let
and let
 be a strongly monotone map, namely there exists
be a strongly monotone map, namely there exists  such that, for
such that, for ,
,

Then variational inequality (1) admits a unique continuous solution.
4. Lipschitz Continuity Result
The aim of this section is to provide a Lipschitz continuity result for the financial equilibrium solution. For this reason, we recall a general result proved in [30] for the solutions to the parameterized variational inequality (18). More precisely, the following result holds (see [30], Theorem 1):
Theorem 4.1 Let  be strongly monotone, Lipschitz continuous with respect to
be strongly monotone, Lipschitz continuous with respect to , Lipschitz continuous with respect to
, Lipschitz continuous with respect to , and there exists
, and there exists  such that, for
such that, for ,
,

where ,
,  , denotes the projection onto the set
, denotes the projection onto the set . Then, the unique solution
. Then, the unique solution ,
,  , to (18) is Lipschitz continuous in
, to (18) is Lipschitz continuous in ,
,  , the following estimate holds:
, the following estimate holds:
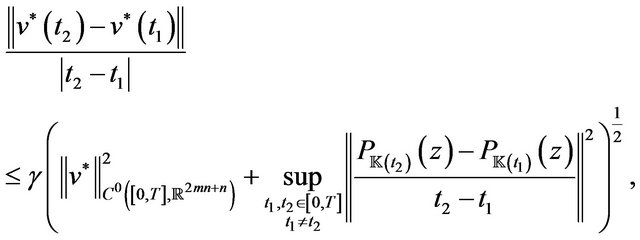
where .
.
For the sake of simplicity, we set
 .
.
Before applying the previous result to our dynamic financial equilibrium problem, it is necessary to estimate the variation rate of projections onto time-dependent constraint set  describing the problem. It is useful to note that
describing the problem. It is useful to note that  can be rewritten as the Cartesian product of the following set:
can be rewritten as the Cartesian product of the following set:
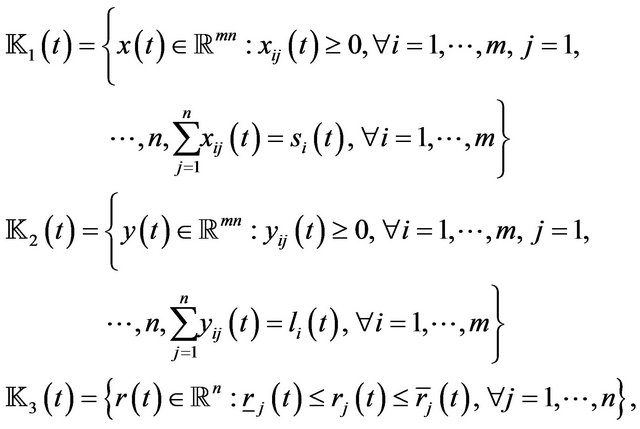
namely
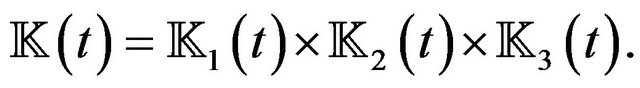
Making use of Proposition 1 in [30], we can show that assuming ,
,  , is Lipschitz continuous with Lipschitz constant
, is Lipschitz continuous with Lipschitz constant , for
, for , it results
, it results
 (19)
(19)
Moreover, under the assumption that ,
,  , is Lipschitz continuous with Lipschitz constant
, is Lipschitz continuous with Lipschitz constant , for
, for , we have
, we have
 (20)
(20)
Now, taking into account Proposition 4.1 in [31] and assuming that  and
and ,
,  , are Lipschitz continuous with Lipschitz constants
, are Lipschitz continuous with Lipschitz constants  and
and , respectively, for
, respectively, for , we can prove
, we can prove
 (21)
(21)
where .
.
We can conclude that Proposition 4.1 Let , let
, let  be two Lipschitz continuous functions and let
be two Lipschitz continuous functions and let  be two Lipschitz continuous functions. Let
be two Lipschitz continuous functions. Let  be an arbitrary point in
be an arbitrary point in . Then it results to be
. Then it results to be
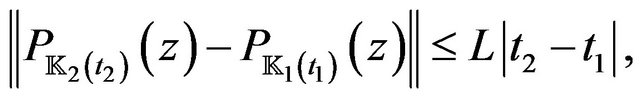
where  is the positive constant as in (3).
is the positive constant as in (3).
As a consequence, it results
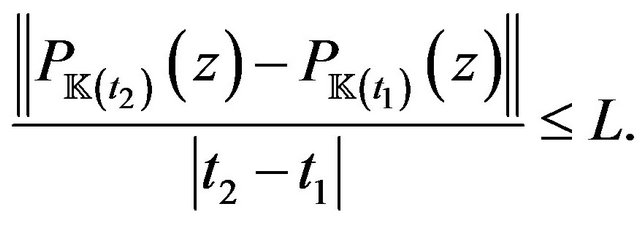
Hence, applying Theorem 4.1, we get the following result.
Theorem 1.2 Let  be strongly monotone (with constant
be strongly monotone (with constant ), Lipschitz continuous with respect to
), Lipschitz continuous with respect to  (with constant
(with constant ), Lipschitz continuous with respect to
), Lipschitz continuous with respect to  (with constant
(with constant ), and let
), and let  be two Lipschitz continuous functions and let
be two Lipschitz continuous functions and let  be two Lipschitz continuous functions. Then, the unique financial equilibrium solution
be two Lipschitz continuous functions. Then, the unique financial equilibrium solution  is Lipschitz continuous in
is Lipschitz continuous in . Moreover, let
. Moreover, let , the following estimate holds:
, the following estimate holds:
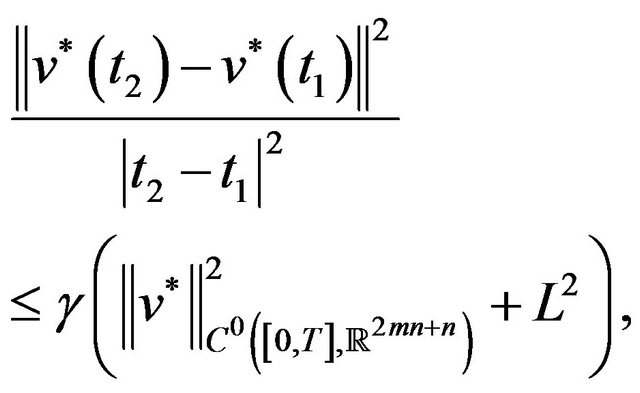
where .
.
5. Some Numerical Examples
Let us study some numerical financial examples in which we consider as the risk aversion function the one suggested by H.M. Markowitz in [32] and [33], which expresses at each instant  the risk aversion by means of variance-covariance matrices denoting the sector’s assessment of the standard deviation of prices for each instrument. We will see that the solutions of the examples are regular and we illustrate the impact of the components of the model on the financial equilibrium, especially for what regards
the risk aversion by means of variance-covariance matrices denoting the sector’s assessment of the standard deviation of prices for each instrument. We will see that the solutions of the examples are regular and we illustrate the impact of the components of the model on the financial equilibrium, especially for what regards  and
and  and the deficit and the surplus.
and the deficit and the surplus.
Let us consider an economy with two sectors and two financial instruments, as shown in Figure 1, but this setting is not restrictive since we can consider a general economy by an iteration of this significant case, and assume that the variance-covariance matrices of the two sectors are the following:
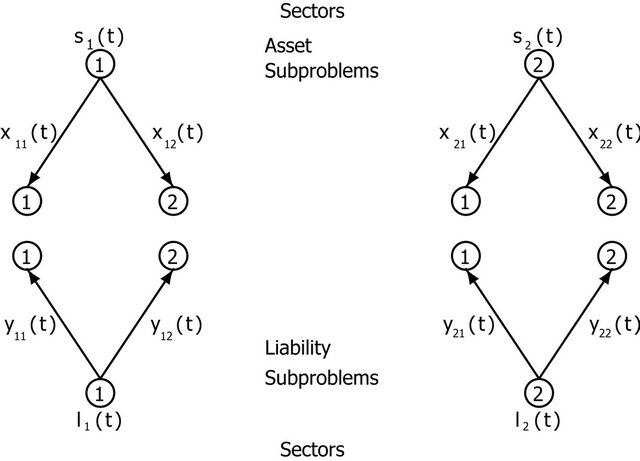
Figure 1. Two sectors and two financial instruments network a.e. in [0,1].

Let us choose as the feasible set
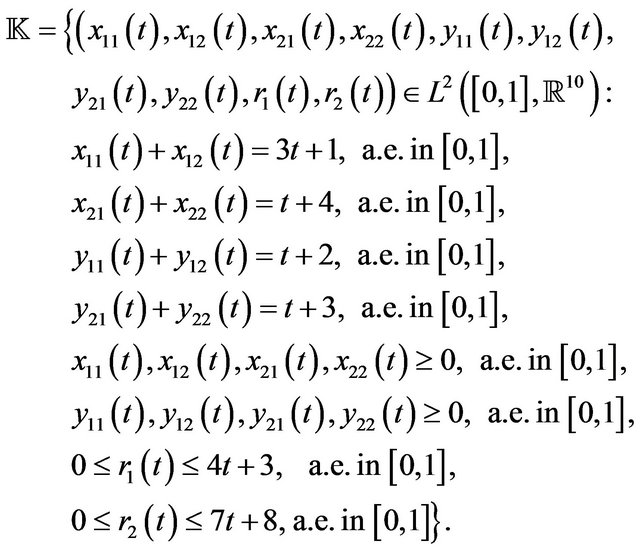
The variational inequality
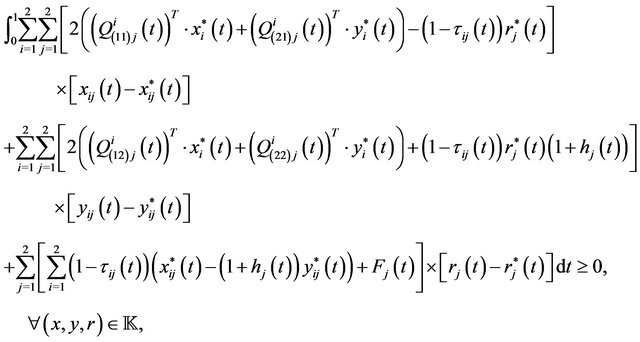
which expresses the financial equilibrium conditions, becomes
 (22)
(22)
Following the direct method (see [21]) in order to find the solution, we can derive from the constraints of the convex set  the values of some variables in terms of the others, namely we obtain, a.e. in
the values of some variables in terms of the others, namely we obtain, a.e. in ,
,
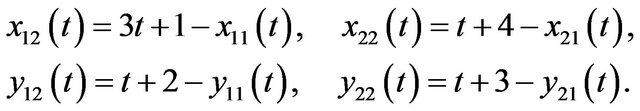
Then, setting
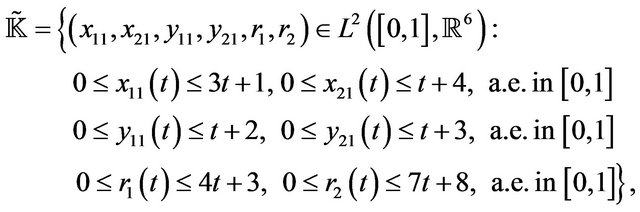
variational inequality (22) can be expressed in the equivalent form:

Let us set
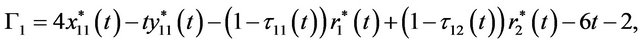
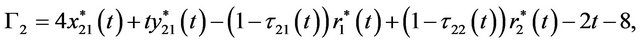



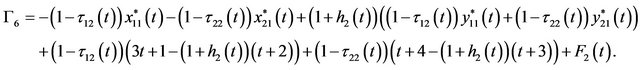
As the first step of the direct method suggests, let us search solutions obtained from the system

We get the values of the variables  and
and  in terms of
in terms of  and
and , namely
, namely
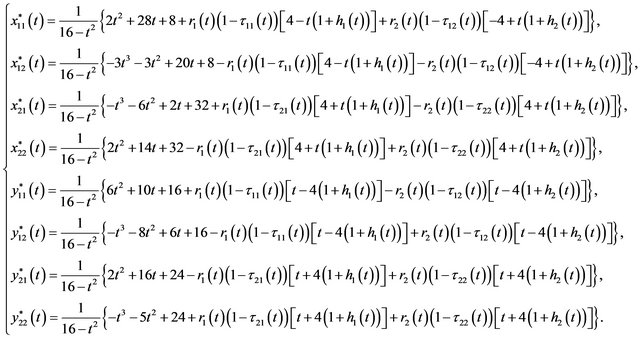
For the sake of simplicity, we assume

We get

In the following, we study various examples of financial equilibrium for which the deficit (namely ) and surplus (namely
) and surplus (namely ) are different from zero in certain time intervals. Such examples depend on the choose of suitable values of the data. In the first example, we consider values of
) are different from zero in certain time intervals. Such examples depend on the choose of suitable values of the data. In the first example, we consider values of  such that
such that
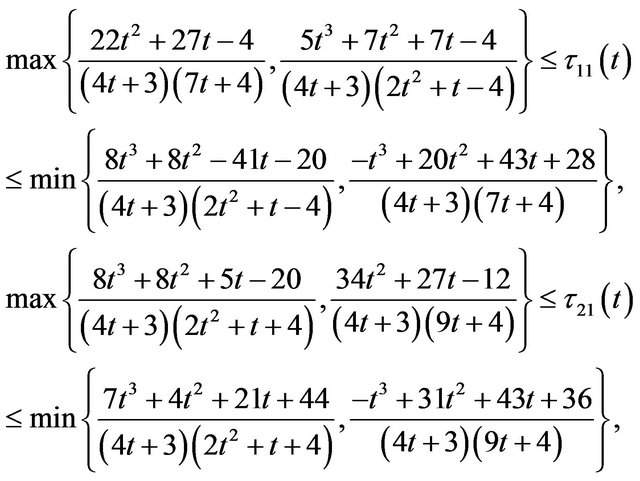
as Figures 2 and 3 show.
In particular, we assume
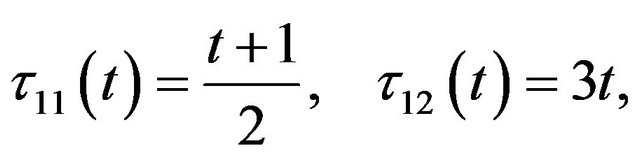

There exist  and
and , such that the vector
, such that the vector
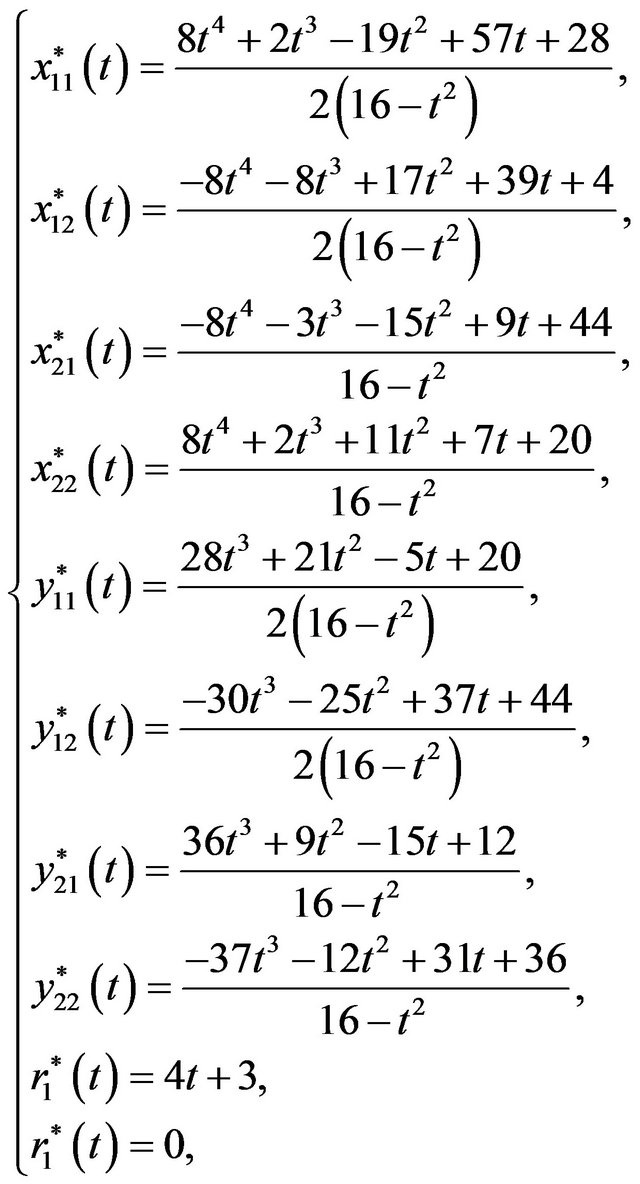
is solution of the variational inequality in the interval , because
, because  fulfil the constraints and
fulfil the constraints and
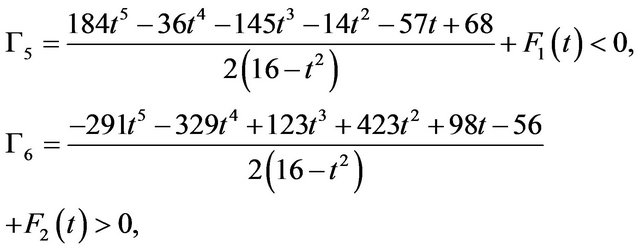
for  small enough and for any
small enough and for any  non negative. In fact, if we consider
non negative. In fact, if we consider

we obtain that they are negative and positive respectively, as it can be verified in Figure 4 where the graphs of the numerators are represented.
Let us remember that, by virtue of Theorem 2.2,  , the following relationships are satisfied
, the following relationships are satisfied
 (23)
(23)
 (24)
(24)
Taking into account that, in our case,
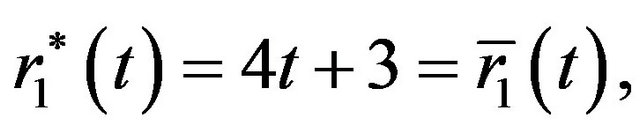

from (23), we obtain
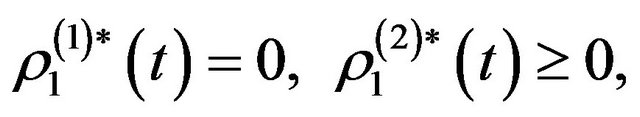
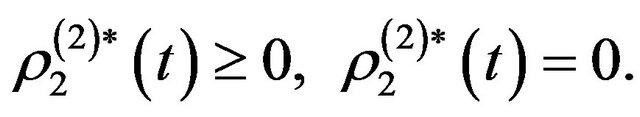
So, from (24), we get
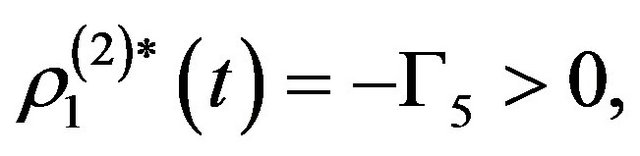
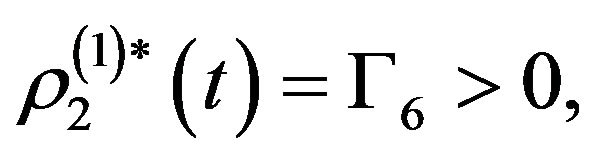
provided that  is small enough and for any
is small enough and for any  non negative.
non negative.
The importance of this example remains in the fact that, in the interval , for
, for  small enough, price
small enough, price  is maximum and the financial market has a surplus given by
is maximum and the financial market has a surplus given by
Whereas, for the instrument , we have minimal price and the deficit is given by
, we have minimal price and the deficit is given by
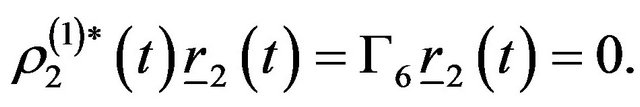
Now, we would like to calculate the Evaluation Index for this financial problem. More precisely, we have
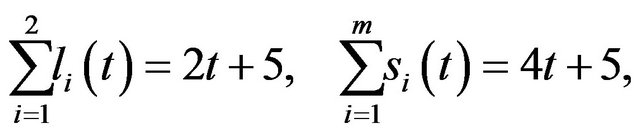

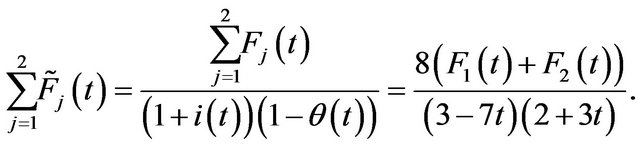
As a consequence, the Evaluation Index is given by:

In the interval , if
, if

the economy has a positive average evaluation. The same situation happens if
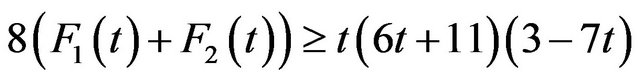
in the interval  (where
(where ).
).
Now let us consider the case where the values of  are such that
are such that

as Figures 5 and 6 show.
In particular, we assume
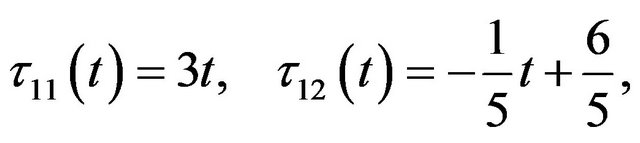

There exist  and
and , such that the vector
, such that the vector
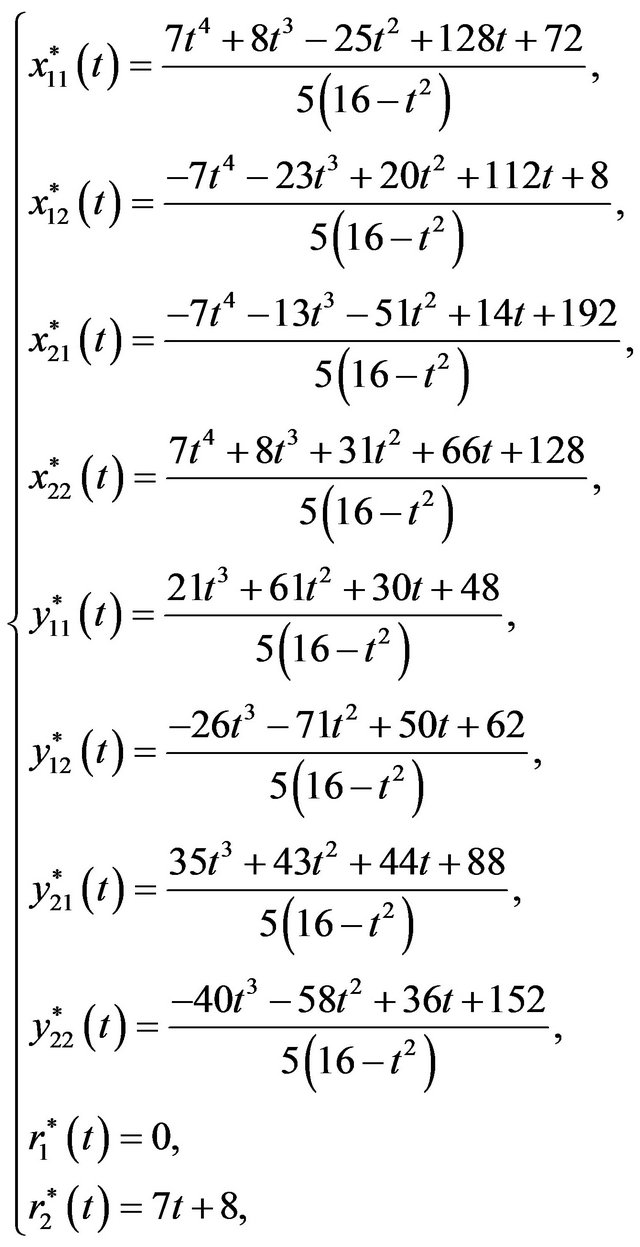
is solution of the variational inequality in the interval , because
, because  fulfil the constraints and
fulfil the constraints and

for any  non negative and for
non negative and for  small enough. In fact, if we consider
small enough. In fact, if we consider
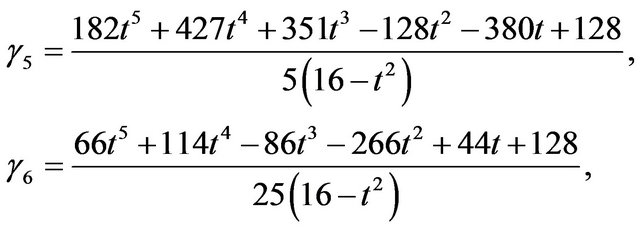
we obtain that they are positive and negative respectively, as it can be verified in Figures 7 and 8 where the graphs of the numerators are represented in detail.
If we remember that, in this case,


from (23), we obtain


So, from (24), we get

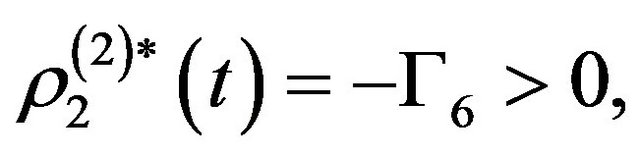
provided that  is small enough and for any
is small enough and for any  non negative.
non negative.
In this situation, we can assert that, in the interval , for the instrument
, for the instrument , we have minimal price and the deficit is given by
, we have minimal price and the deficit is given by

Whereas, for  small enough, price
small enough, price  is maximum and the financial market has a surplus given by
is maximum and the financial market has a surplus given by
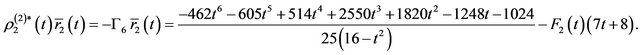
Now let us proceed to the calculation of the Evaluation Index. We have
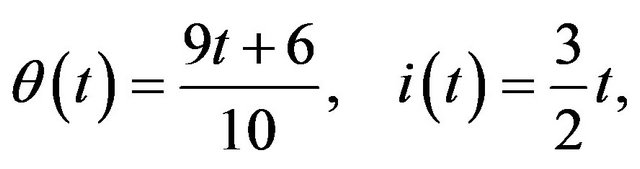

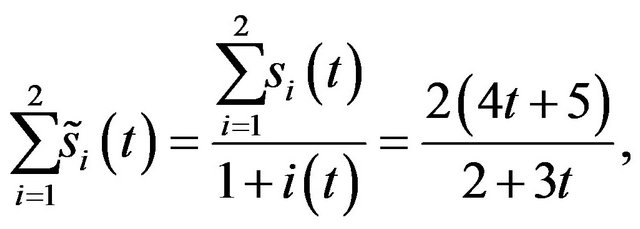
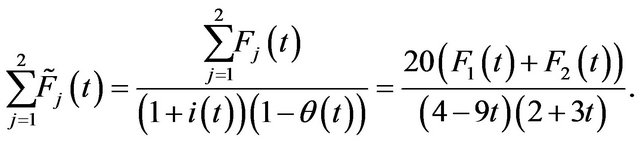
Then the Evaluation Index is
As a consequence, in the interval , if
, if

the economy has a positive average evaluation. The same situation happens if
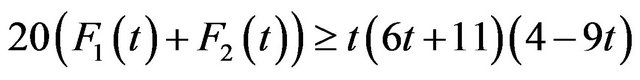
in the interval  (where
(where ). From this example we see that we can get useful information from the
). From this example we see that we can get useful information from the , which requires simple calculations.
, which requires simple calculations.
Now, we would like to study another example in which we assume
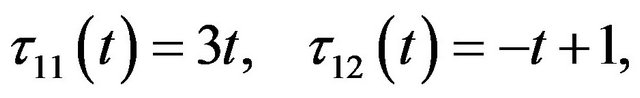

Under these assumptions, there exist  and
and , such that the vector
, such that the vector
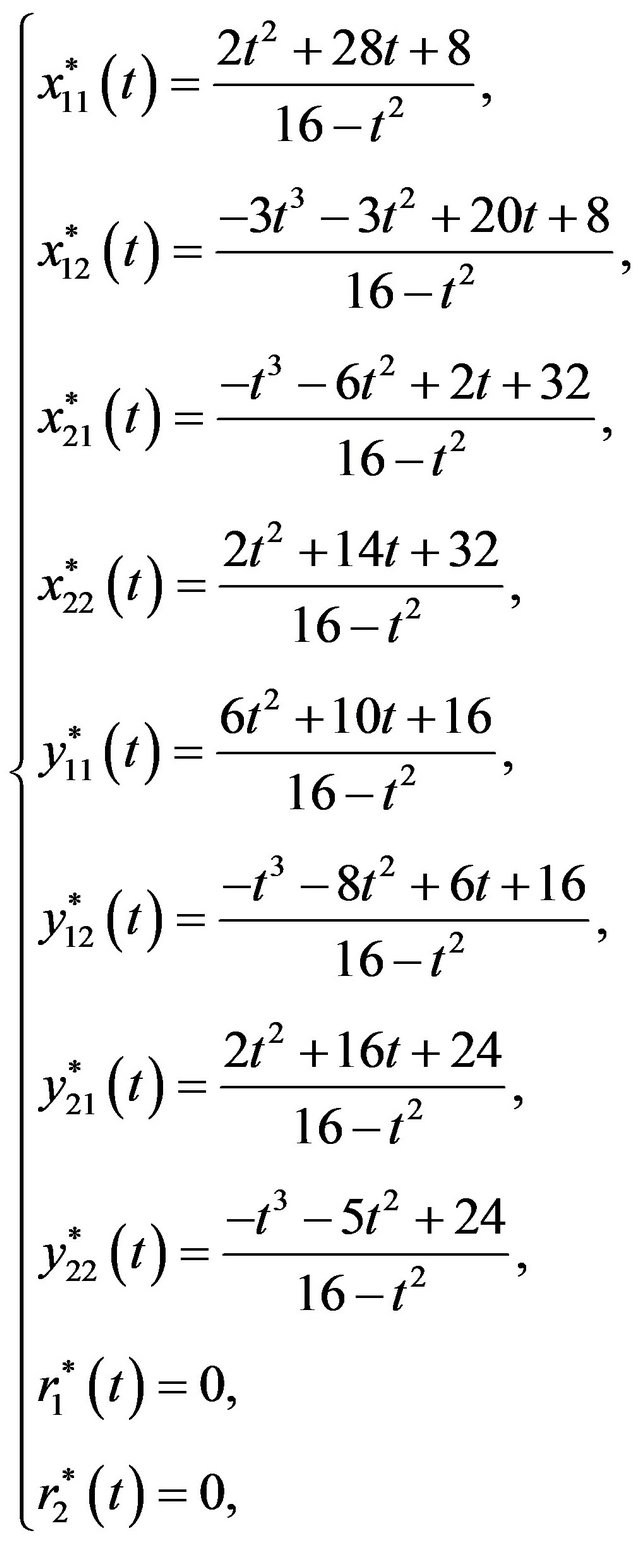
is solution of the variational inequality in the interval , because
, because  fulfil the constraints and
fulfil the constraints and
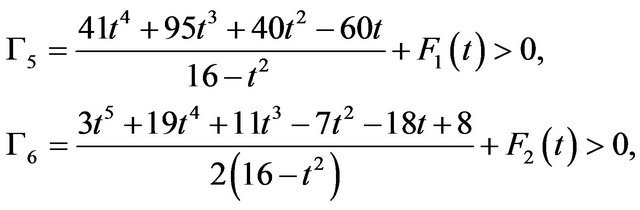
for  and
and  non negative. In fact, if we consider
non negative. In fact, if we consider
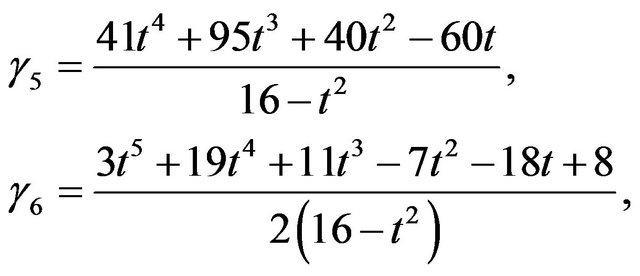
we obtain that they are both positive, as it can be verified in Figure 9 where the graphs of the numerators are represented.
We observe that, in our case


so, from (23), it follows that
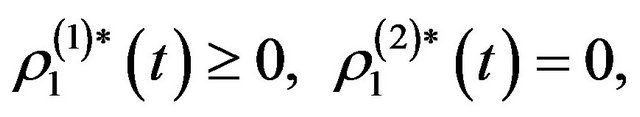

Then, from (24), we obtain

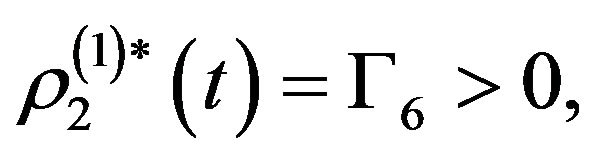
for any  and
and  non negative.
non negative.
As consequence of the meaning of , in the interval
, in the interval , for both the instruments, we have minimal price and the deficit is given, respectively, by
, for both the instruments, we have minimal price and the deficit is given, respectively, by
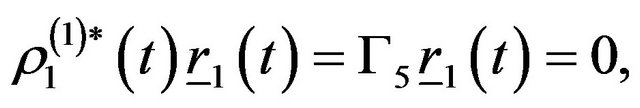

As regards the calculation of the Evaluation Index, we have

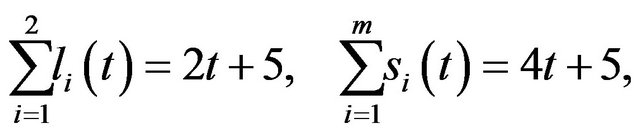

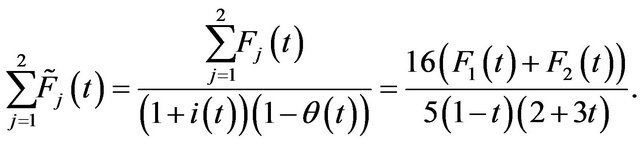
As a consequence, the Evaluation Index is

Then, in the interval , if
, if
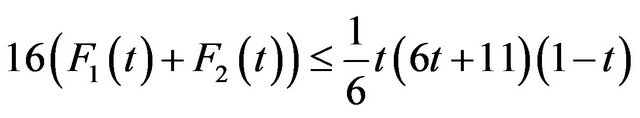
the economy has a positive average evaluation because . From this example we see that it is easier to achieve financial balance if
. From this example we see that it is easier to achieve financial balance if  and
and  are more or less equal.
are more or less equal.