1. Introduction
In [1], Olga Taussky introduces a group operation on the set of primitive pythagorean triples
 that is, the set of primitive integral triangles
that is, the set of primitive integral triangles  in which the angle
in which the angle 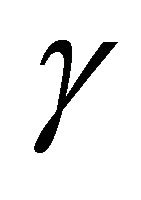 opposite side c is a right angle. Ernest J. Eckert spells out the details of the group operation in [2]:
opposite side c is a right angle. Ernest J. Eckert spells out the details of the group operation in [2]:
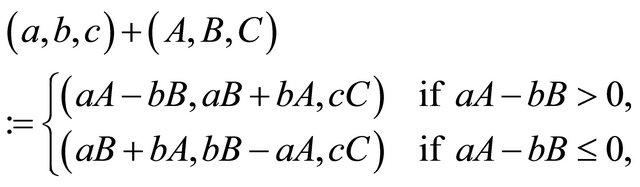
where we reduce the answer by a common factor if necessary to obtain a primitive triple. The group has identity ; the inverse of
; the inverse of  is
is .
.
The construction arises from the famous identity on sums of squares:
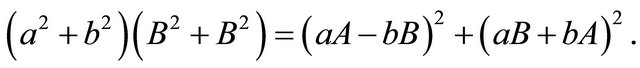
This identity, of course, corresponds to the rule  for complex numbers, and the group law on pythagorean triples has a similar geometric motivation: we identify the triangle
for complex numbers, and the group law on pythagorean triples has a similar geometric motivation: we identify the triangle  with the complex number
with the complex number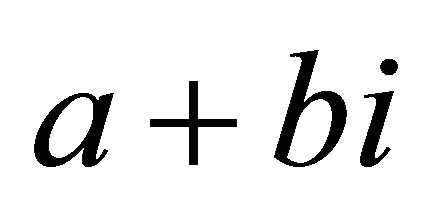 , and the group operation is then just complex multiplication.
, and the group operation is then just complex multiplication.
The argument of  is the angle
is the angle  opposite side b of the triangle. Since we add the arguments of complex numbers when we multiply the numbers, we can interpret the group operation as addition of the angles
opposite side b of the triangle. Since we add the arguments of complex numbers when we multiply the numbers, we can interpret the group operation as addition of the angles  and
and  to find a primitive triple whose corresponding angle is
to find a primitive triple whose corresponding angle is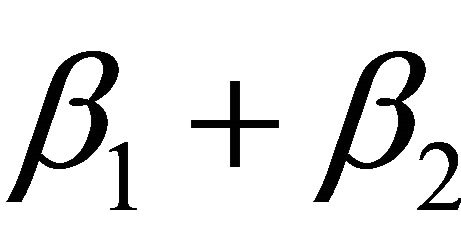 . A problem arises when
. A problem arises when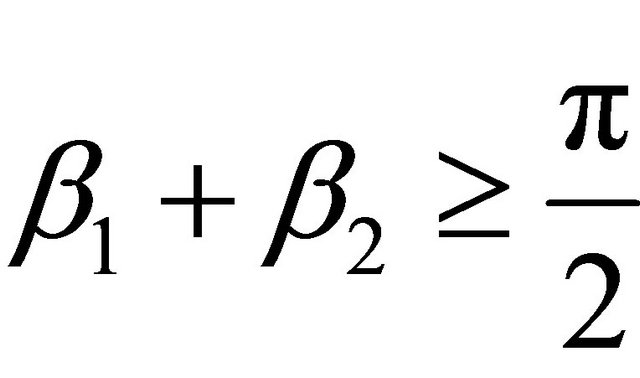 i.e. when
i.e. when , since this no longer corresponds to a pythagorean triple with positive coordinates. To fix this, we mod out the angle by
, since this no longer corresponds to a pythagorean triple with positive coordinates. To fix this, we mod out the angle by ; this is why we have the two cases in the definition of the group operation above.
; this is why we have the two cases in the definition of the group operation above.
This group has been the subject of much interest over the years. In [3], Jean Mariani studies the group of homogeneous linear transformations with integral coefficients that preserve the triangles above as a set. In [4], Barbara Margolius uses a sequence of pythagorean triples derived from the group law above to prove that Plouffe’s constant  is transcendental, a special case of Hilbert’s seventh problem.
is transcendental, a special case of Hilbert’s seventh problem.
Eckert’s main result in [2] is that this group is free abelian, that is, isomorphic to a direct sum of copies of the integers. The sum is indexed by the triangles , where
, where  and p is prime with
and p is prime with . In particular, the group is torsion-free, that is, there do not exist any nontrivial elements
. In particular, the group is torsion-free, that is, there do not exist any nontrivial elements  with
with  for
for .
.
In [5], Eckert and Preben Dahl Vestergaard generalize the construction above to the case when  is no longer a right angle. We define an angle
is no longer a right angle. We define an angle  to be pythagorean if
to be pythagorean if  or
or 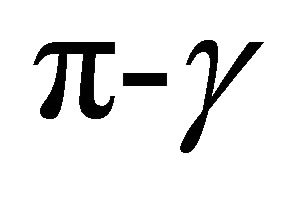 is an angle in a pythagorean triangle, or, equivalently, if
is an angle in a pythagorean triangle, or, equivalently, if  and
and  are both rational. For a fixed pythagorean angle
are both rational. For a fixed pythagorean angle , we define
, we define  to be the set of primitive integral triangles
to be the set of primitive integral triangles  for which the angle opposite side c is
for which the angle opposite side c is . We can add two triangles by adding their values for
. We can add two triangles by adding their values for , modulo
, modulo :
:
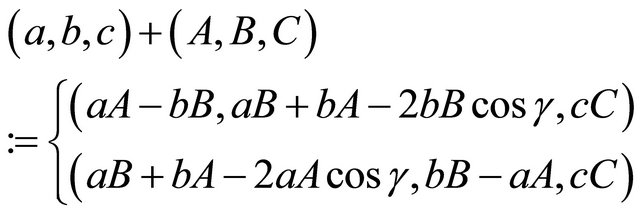
We use the first formula above in the case when , which corresponds to
, which corresponds to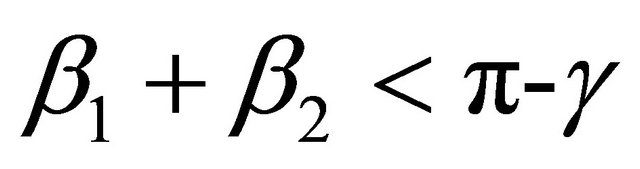 , and the second when
, and the second when , which corresponds to
, which corresponds to . As before, we stipulate that we will scale the answer by a common factor if necessary to obtain a primitive integral triangle. Eckert and Vestergaard prove that this construction again gives us a group, still with identity
. As before, we stipulate that we will scale the answer by a common factor if necessary to obtain a primitive integral triangle. Eckert and Vestergaard prove that this construction again gives us a group, still with identity  and with the inverse of
and with the inverse of  being
being . Note that the original group considered in [2] is just the special case
. Note that the original group considered in [2] is just the special case , whose structure we know completely.
, whose structure we know completely.
Eckert and Vestergaard ask a number of interesting questions about the structure of  in general. They mention that when
in general. They mention that when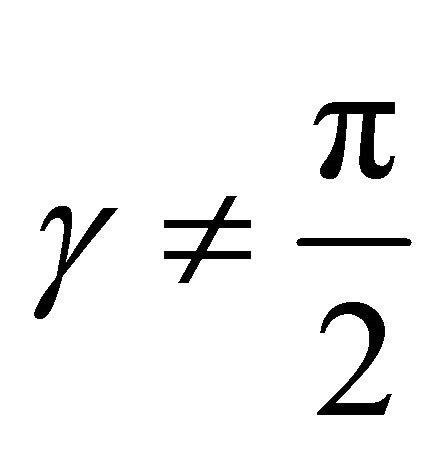 ,
,  can have nontrivial torsion elements, that is, elements
can have nontrivial torsion elements, that is, elements  such that
such that  for some
for some , but they do not give details. In this paper, we pursue the study of torsion elements in
, but they do not give details. In this paper, we pursue the study of torsion elements in . Our main results give necessary and sufficient conditions on
. Our main results give necessary and sufficient conditions on  for
for 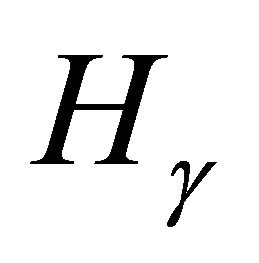 to have two-torsion and threetorsion. From these theorems, it will be easy to see that the set of values of
to have two-torsion and threetorsion. From these theorems, it will be easy to see that the set of values of  for which
for which  has two-torsion is dense in the interval
has two-torsion is dense in the interval , and similarly for threetorsion. We will also show that there is at most one copy of either
, and similarly for threetorsion. We will also show that there is at most one copy of either 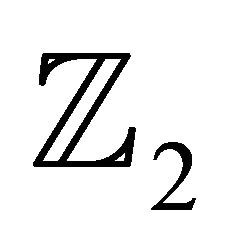 or
or  in
in . Finally, we will give some examples of higher-order torsion elements in
. Finally, we will give some examples of higher-order torsion elements in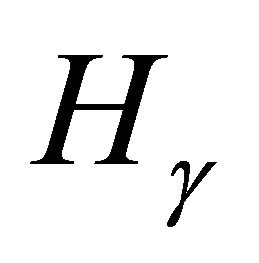 .
.
2. Two-Torsion
Our results on two-torsion and three-torsion are similar in flavor, but the theorem on two-torsion is simpler and significantly easier to prove, so we will start there.
Theorem 1. 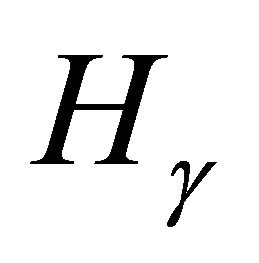 has a point of order two iff
has a point of order two iff 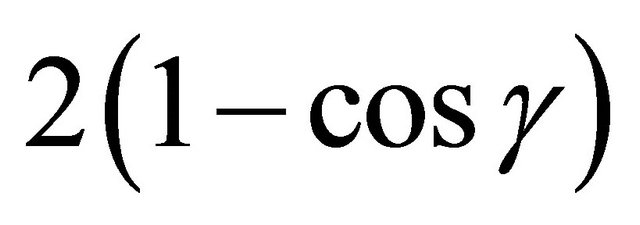 is a rational square. When this is true, the point of order two is unique.
is a rational square. When this is true, the point of order two is unique.
Proof of Theorem 1. Since the inverse of  is
is , a point has order two iff it has the form
, a point has order two iff it has the form .
.
Suppose first that 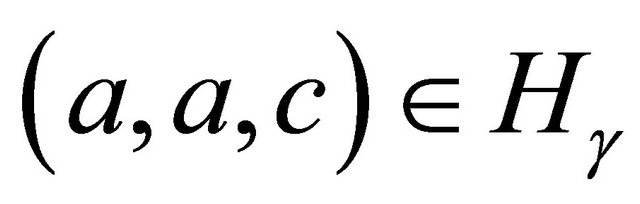 is a point of order two. We invoke the Law of Cosines:
is a point of order two. We invoke the Law of Cosines:

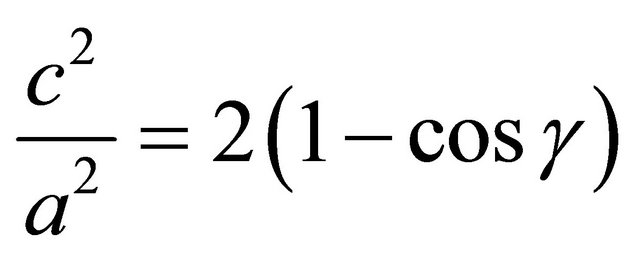
So  is a rational square, as desired.
is a rational square, as desired.
Conversely, suppose that , where a,
, where a,
 are relatively prime. Note that
are relatively prime. Note that  , so
, so , so
, so  is a triangle, and the computation above shows that by the Law of Cosines, the angle opposite side c is
is a triangle, and the computation above shows that by the Law of Cosines, the angle opposite side c is . Hence
. Hence  is a point of order two.
is a point of order two.
To show that only one point of order two can exist, we note that any two triangles 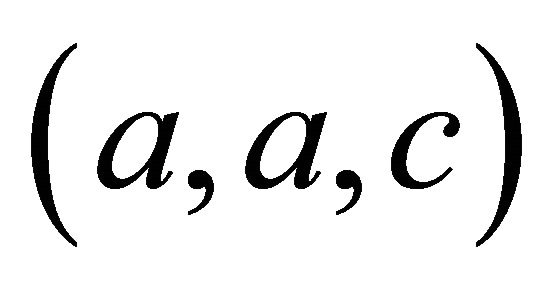 and
and  in
in  would have to be scalar multiples of each other, and would therefore be represented by a single primitive integral triangle. ∎
would have to be scalar multiples of each other, and would therefore be represented by a single primitive integral triangle. ∎
We illustrate Theorem 1 with some quick examples:
1) For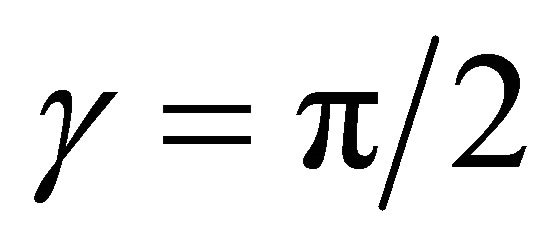 , we have
, we have , and as shown in [2], has no point of order two.
, and as shown in [2], has no point of order two.
2) For , we have
, we have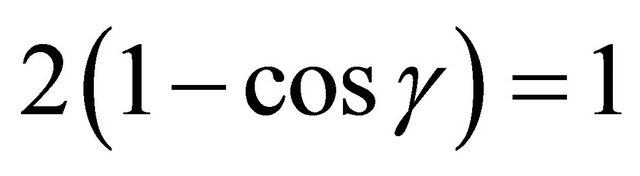 , and
, and  contains the point (1, 1, 1) of order two.
contains the point (1, 1, 1) of order two.
3) For , we have
, we have , and
, and  contains the point (2, 2, 3) of order two.
contains the point (2, 2, 3) of order two.
Corollary 2. The set of  for which
for which  contains a point of order two is dense in
contains a point of order two is dense in .
.
Proof. This follows from the fact that  is a continuous function from
is a continuous function from onto
onto  and the rational squares are dense in
and the rational squares are dense in . ∎
. ∎
3. Three-Torsion
Our result for three-torsion follows the same general spirit as that for two-torsion, but is significantly harder. We first prove the easy result that  contains at most one copy of
contains at most one copy of ; this proof will help set the stage for the proof of our main theorem.
; this proof will help set the stage for the proof of our main theorem.
Theorem 3.  contains at most two points of order three.
contains at most two points of order three.
Proof of Theorem 3: Suppose 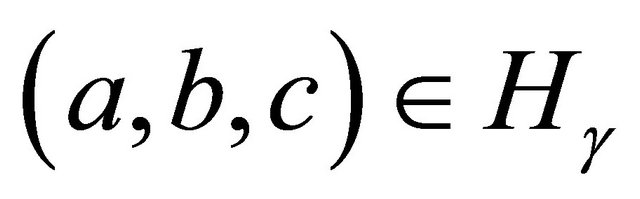 has order three. Note that its inverse
has order three. Note that its inverse  also has order three, so we may assume without loss of generality that
also has order three, so we may assume without loss of generality that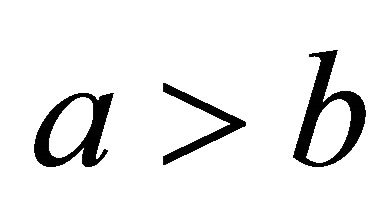 . (If
. (If , then as we saw above,
, then as we saw above,  has order two, so it cannot have order three). Let
has order two, so it cannot have order three). Let  and
and  be the angles opposite sides a and b respectively. Since
be the angles opposite sides a and b respectively. Since , we have
, we have , so
, so . Thus, when we compute
. Thus, when we compute , we are adding
, we are adding  to itself, and there is no modding out by
to itself, and there is no modding out by . The resulting triangle is
. The resulting triangle is , which has angle
, which has angle  opposite side a, so
opposite side a, so . But since
. But since  is fixed, the equation
is fixed, the equation  completely determines the three angles of the triangle, so there is only one primitive triple with these angles. (The other point of order three, of course, is the inverse
completely determines the three angles of the triangle, so there is only one primitive triple with these angles. (The other point of order three, of course, is the inverse ). ∎
). ∎
We will continue to use some of the ideas above in the proof of our main theorem on three-torsion.
Theorem 4.  has a point of order three iff the cubic
has a point of order three iff the cubic

has a rational solution .
.
The cubic criterion gives easy answers to two questions: First, given a specified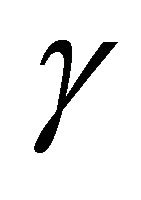 , we can ask whether
, we can ask whether  has three-torsion. Assuming that
has three-torsion. Assuming that  is pythagorean, we can use the Rational Root Theorem to check quickly whether the cubic above has a rational solution between 0 and 1. If it does, the proof below will give a constructive algorithm to produce a triangle
is pythagorean, we can use the Rational Root Theorem to check quickly whether the cubic above has a rational solution between 0 and 1. If it does, the proof below will give a constructive algorithm to produce a triangle  of order three.
of order three.
Second, if we don’t have a specific  in mind, we can use the cubic criterion to find values of
in mind, we can use the cubic criterion to find values of 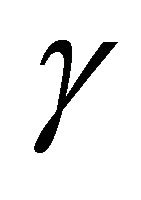 for which
for which 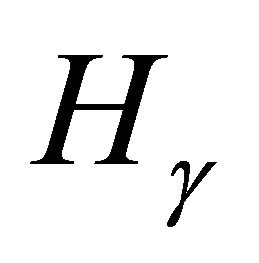 has three-torsion. Indeed, we can solve the equation above for
has three-torsion. Indeed, we can solve the equation above for :
:

The rational function on the right takes values less than −1 for 0 < x < 1/2, and it maps the interval 1/2 ≤ x ≤ 1 continuously onto the range . Therefore, to find angles whose groups have three-torsion, we can take any rational 1/2 ≤ x ≤ 1 and then find the corresponding
. Therefore, to find angles whose groups have three-torsion, we can take any rational 1/2 ≤ x ≤ 1 and then find the corresponding .
.
Proof of Theorem 4. First, suppose that 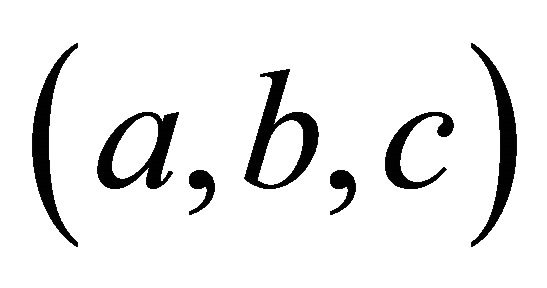 has order three. As in the proof of Theorem 3 above, we may assume that a > b and that when we compute
has order three. As in the proof of Theorem 3 above, we may assume that a > b and that when we compute , there is no modding out by
, there is no modding out by . So we use the first set of equations for the group operation:
. So we use the first set of equations for the group operation:
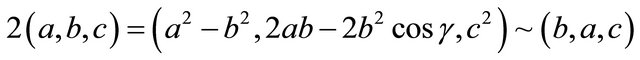
The common factor between the two triangles on the right is c, giving us the following “scaling equations”:
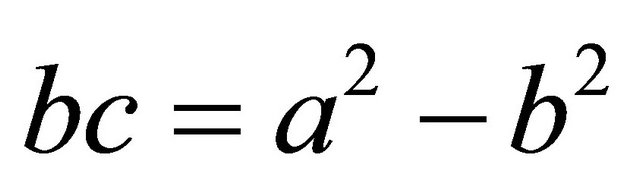

Solve the second scaling equation for c:
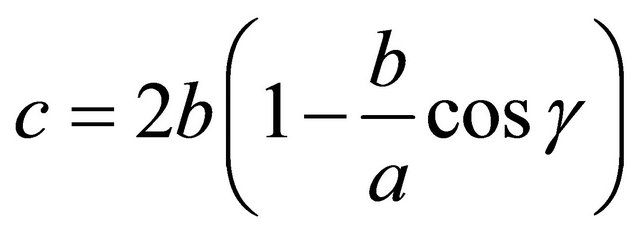
Plug this into the Law of Cosines:
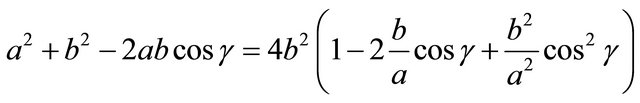
Multiply by :
:
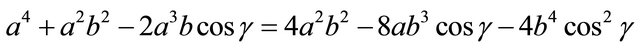
Rearrange:
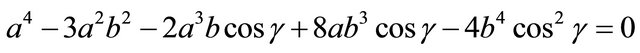
Factor:

We claim that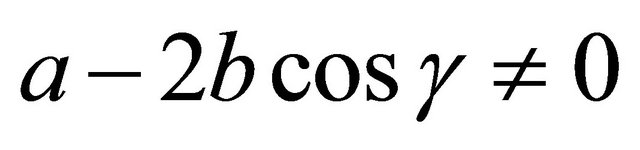 . To prove this claimsuppose that
. To prove this claimsuppose that ; then
; then . Plugging this into the second scaling equation, we get ac = ab, so b = c. Plugging this into the first scaling equation, we get
. Plugging this into the second scaling equation, we get ac = ab, so b = c. Plugging this into the first scaling equation, we get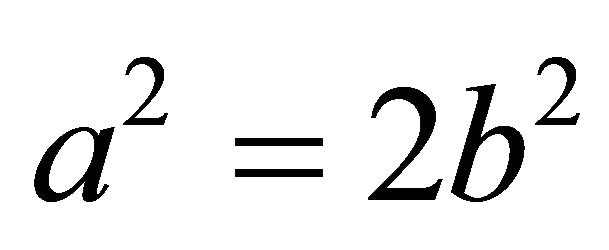 , contradicting the fact that a and b are both integers. This proves our claim, so we may cancel the factor of
, contradicting the fact that a and b are both integers. This proves our claim, so we may cancel the factor of :
:
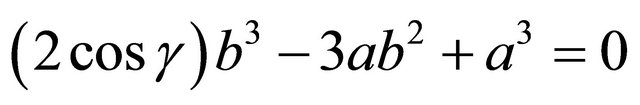
Divide by 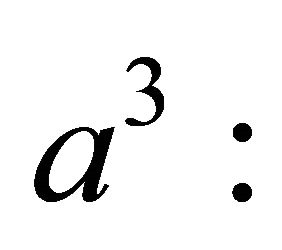
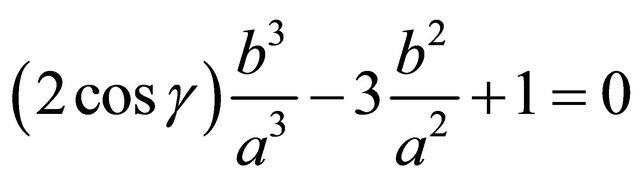
Set , which is a rational number with 0 < x < 1, and we have
, which is a rational number with 0 < x < 1, and we have
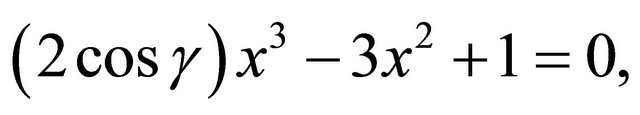
as desired.
Conversely, suppose we have a rational solution 0 < x < 1 to
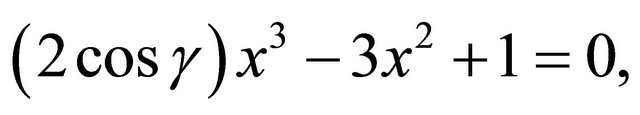
Define  to be positive relatively prime integers such that
to be positive relatively prime integers such that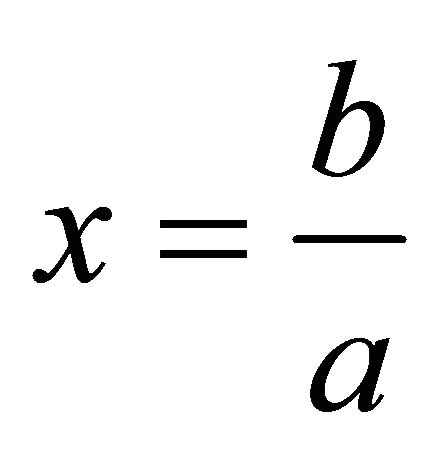 , and define c to satisfy
, and define c to satisfy
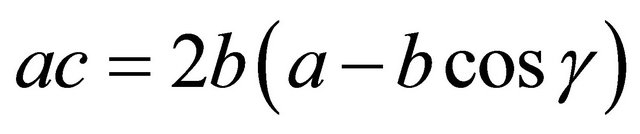 . (Note that c > 0). Scale a, b, and c by a common factor, if necessary, to be relatively prime integers; note that this scaling will preserve both
. (Note that c > 0). Scale a, b, and c by a common factor, if necessary, to be relatively prime integers; note that this scaling will preserve both 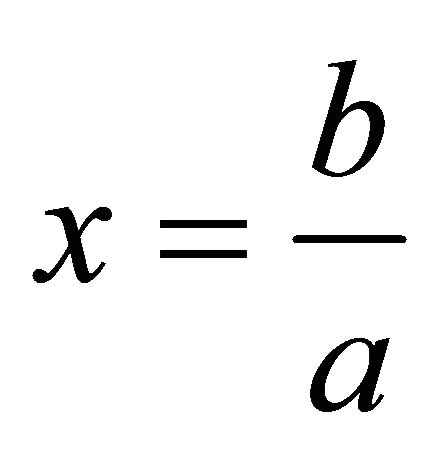
and .
.
By reversing the equations from the forward direction of the proof, we can work back to
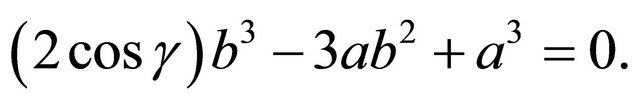
We can solve this for  and then build up to
and then build up to  as follows:
as follows:




Substitute:

Simplify:

Now we check that  is a triangle. First, we already saw that
is a triangle. First, we already saw that , so
, so . Second, to see that
. Second, to see that , we can reorganize the equation immediately above:
, we can reorganize the equation immediately above:

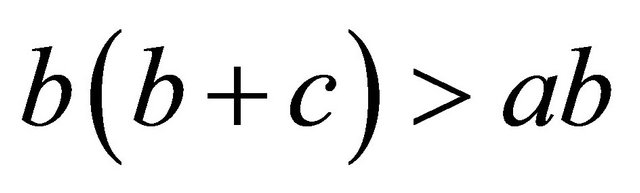
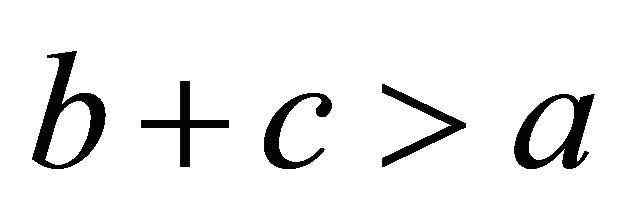
Third, we can continue to reverse the equations from the forward direction of the proof until we get back to
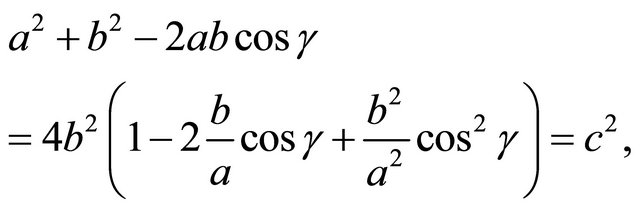
which gives us . It also shows by the Law of Cosines that the triangle
. It also shows by the Law of Cosines that the triangle , has angle
, has angle  opposite side c, so
opposite side c, so .
.
Finally, we have already established the scaling equations
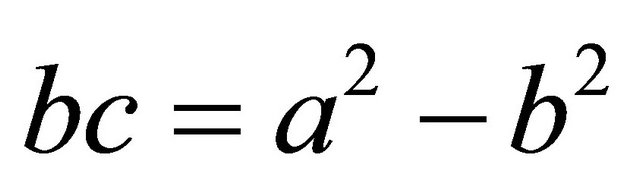
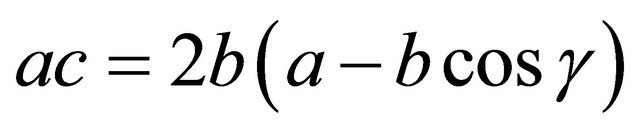
which show that
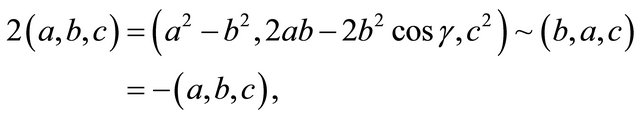
so  has order three, as desired. ∎
has order three, as desired. ∎
Here are some sample applications of Theorem 4:
1) Let . Then the cubic degenerates to
. Then the cubic degenerates to  , which has no rational solutions at all, and
, which has no rational solutions at all, and  has no point of order three.
has no point of order three.
2) Let . Then the cubic becomes
. Then the cubic becomes
 , which has the rational solution
, which has the rational solution .
.
Following this through the proof above leads to the triangle , which has order three.
, which has order three.
3) Let . Then the cubic becomes
. Then the cubic becomes , which has the rational solution
, which has the rational solution . This leads to the triangle
. This leads to the triangle , which has order three.
, which has order three.
Although it is not quite so obvious, we have the same corollary as for two-torsion:
Corollary 5. The set of 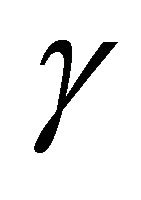 for which
for which 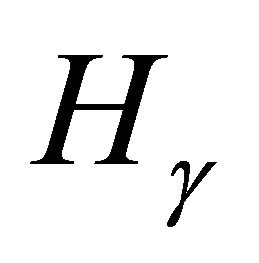 contains a point of order three is dense in
contains a point of order three is dense in .
.
Proof. The rationals are dense in the interval 1/2 ≤ x ≤ 1, which, as noted above, is mapped continuously by
 onto
onto  and in turn by arccos onto 0 ≤ y ≤
and in turn by arccos onto 0 ≤ y ≤
π. Hence the set of  corresponding to a rational x is dense in
corresponding to a rational x is dense in . ∎
. ∎
4. Examples of Higher Order Torsion
We close with some examples of higher order torsion points in :
:
1) Let . Then the triangle (10, 8, 3) has order four in
. Then the triangle (10, 8, 3) has order four in .
.
2) Let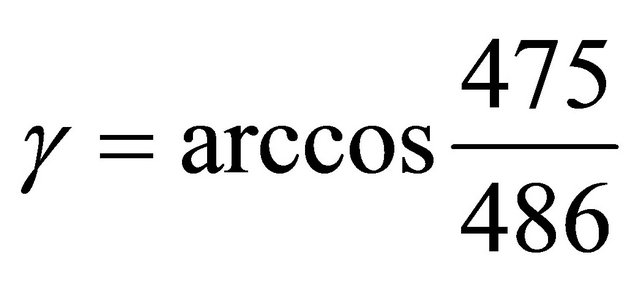 . Then the triangle (105, 81, 31) has order five in
. Then the triangle (105, 81, 31) has order five in .
.
3) Let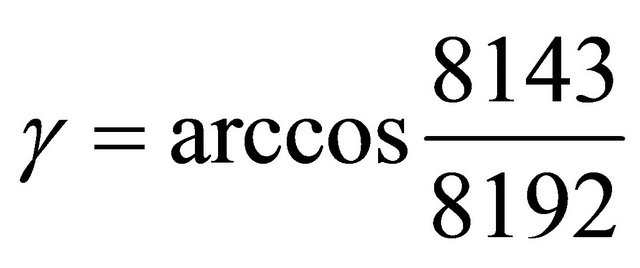 . Then the triangle (1220, 1024, 231) has order six in
. Then the triangle (1220, 1024, 231) has order six in .
.
I found these essentially by a brute force search on a computer, finding multiples of integral triangles and seeing which ones have finite order. As patterns start to emerge for smaller values, we can narrow and speed up the search for larger values by restricting to those triangles that satisfy similar patterns. In particular, there is a clear pattern in the values of b here:  From computer-generated evidence, the pattern on the exponents seems to be genuine, but the pattern on the bases is a red herring: other examples of points of order six are
From computer-generated evidence, the pattern on the exponents seems to be genuine, but the pattern on the bases is a red herring: other examples of points of order six are
(5555, 3125, 3024) for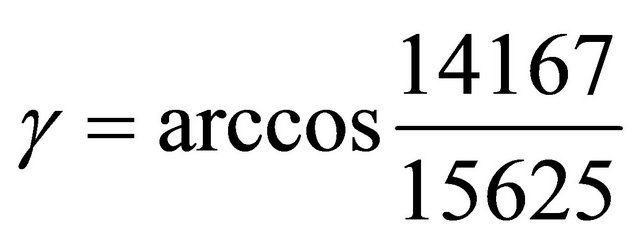 , (17214, 7776, 12155) for
, (17214, 7776, 12155) for , and (42833, 16807, 34320) for
, and (42833, 16807, 34320) for . The consistent pattern seems to be that a point of order n must have the form
. The consistent pattern seems to be that a point of order n must have the form  (or, of course,
(or, of course,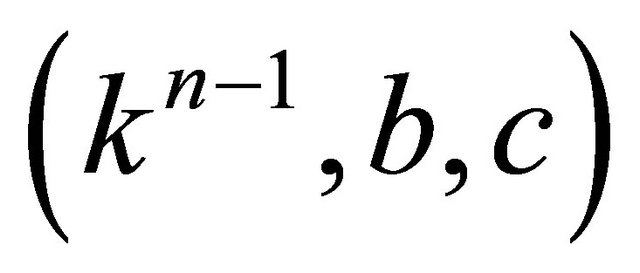 ), but I do not have a proof of this. I invite you to prove this or find a counterexample.
), but I do not have a proof of this. I invite you to prove this or find a counterexample.
5. Conclusions and Further Questions
We have seen that although  is torsion-free, there are many values
is torsion-free, there are many values  for which
for which  has torsion points of various orders. In fact, the set of
has torsion points of various orders. In fact, the set of  for which
for which  has two-torsion (respectively, three-torsion) is dense in
has two-torsion (respectively, three-torsion) is dense in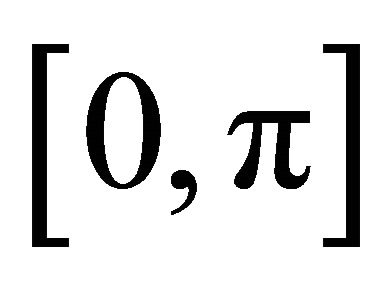 , and we can easily characterize all such
, and we can easily characterize all such  in terms of conditions on
in terms of conditions on . We have also seen that in such cases,
. We have also seen that in such cases,  contains a unique copy of
contains a unique copy of 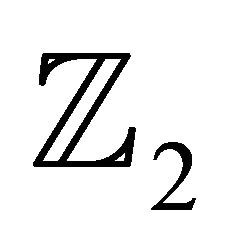 (respectively,
(respectively, ).
).
There remain many intriguing open questions on . Obviously we would like to have a complete determination of the isomorphism type of
. Obviously we would like to have a complete determination of the isomorphism type of  for all
for all , but since that doesn’t seem feasible in the near future, there are plenty of more approachable issues:
, but since that doesn’t seem feasible in the near future, there are plenty of more approachable issues:
1) For what values of n do there exist  with n-torsion?
with n-torsion?
2) For any n for which this is true, how can we characterize the  for which
for which  has n-torsion?
has n-torsion?
3) Is the set of such  dense in
dense in ? (Note that in all the examples of higher order torsion above, we had
? (Note that in all the examples of higher order torsion above, we had ).
).
4) Is it possible for  to contain more than one copy of
to contain more than one copy of ?
?