An Error Modeling Framework for the Sun Azimuth Obtained at a Location with the Hour Angle Method ()
1. Introduction
Finding the locations of points often depends on angular measurements and directions of lines [1]. Determination of the directions of lines is crucial in many engineering applications. In order to determine the direction of a line, three requirements need to be met including a reference line, direction of angular measurement, and the value of the angle. The direction of a line is described by the horizontal angle between the reference line commonly known as meridian and the line of interest. If this angle is measured between the meridian’s north or south directions, the angle is then referred to as the north or south azimuth.
Azimuth is generally defined as the angle in the horizontal plane measured from the meridian’s north (or south) to the location of the line in question. It is common however to refer to the north azimuth as “azimuth” without saying north or south azimuth. There are several types of meridians in use including true or geodetic, astronomic, magnetic, grid, record, assumed, etc. The true or geodetic meridian is the line that passes through a mean position between the earth’s geographic north and south poles. The astronomic meridian is obtained through astronomic observations of the Sun or North Star (Polaris) and it is one instance of the true or geodetic meridian. The astronomic meridian’s location is a function of the direction of gravity and the axis of rotation of the Earth and it determined from a mathematical approximation of the Earth’s shape [2]. Although the magnetic azimuth of a line can be obtained easily in the field by using a compass, it’s desired to prepare engineering maps and plans based on true/geodetic meridian. It’s not unusual to conduct mapping surveys based on magnetic meridian and convert the lines directions to true/geodetic azimuths given the magnetic declination at the time of the survey. The magnetic declination at a location is the horizontal angle measured to the east or west of the true/geodetic meridian’ north (or south) to the location of the magnetic meridian’s north (or south).
It is naturally common that measurements are subject to variations especially we tend to repeat measurement to improve the precision of the measurement process. These variations from a so-called “true value” of the measurement are known as errors, but because the true value is never known, we use the most probable value of the measurements, which is equivalent to the arithmetic mean, but the errors are then called residuals. Errors are commonly classified into three categories: personal, systematic, and random errors. Personal errors result due to carelessness of the surveyor when collecting surveying data in the field. This type of errors can be eliminated by implementing field procedures that are designed to reduce mistakes. Systematic errors take place due to instrumental faults or due to a natural causes for example; changes in temperature, pressure, humidity, etc. This type of errors can be eliminated by the adopting calibration procedures that check and identify the values of the instrumental errors and/or applying corrections to the measurements that are influenced by the natural conditions in the field. Random errors are the errors that remain in the measurement after both of personal and systematic errors are corrected. Unlike personal and systematic errors, random errors have random behavior because they have unknown deterministic nature, and therefore can only be modeled [3]. In this study, an error modeling framework for random errors result in the process of determining the sun azimuth using the hour angle method is presented.
Following Buckner [4], the azimuth of the sun; L measured clockwise from astronomic north is given by:
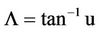 (1)
(1)
where: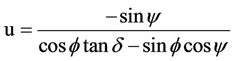 , y is the local hour angle of the sun, d is the declination of the sun, and f is the latitude of the observer.
, y is the local hour angle of the sun, d is the declination of the sun, and f is the latitude of the observer.
The azimuth of the sun; L is normalized from 0˚ to 360˚ by adding algebraically a correction from Table 1 shown below.
In the field, the horizontal angles from a line to the sun are obtained from direct and reverse (face left and face right or face I and face II pointing taken on the backsight mark and the sun). It is suggested that repeating the odolites be used as directional instruments with one of two general measuring procedures being followed, which are: 1) A single foresight pointing on the sun for each pointing on the back-sight mark: here the sighting sequence is: direct on mark, direct on sun, reverse on sun, and reverse on mark, with times being recorded for each pointing on the sun. The two times and four horizontal circle readings constitute one set of data. An observation consists of one or more sets. A minimum of 3 sets is recommended. This procedure is similar to that of meas-
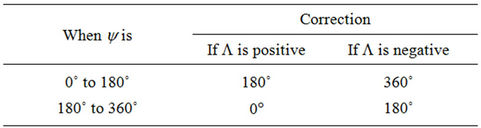
Table 1. Corrections for the normalized azimuth of the sun.
uring an angle at a traverse station using a directional theodolite. This procedure is based on the assumption that pointings on the sun are of approximately the same accuracy as pointings on the back-sight mark. The single foresight procedure imparts itself to proper procedure for incrementing the horizontal circle and micrometer settings on the back-sight. 2) Multiple foresights pointing on the sun for each pointing on the back-sight mark: here, the sighting sequence is: direct on mark, several direct on the sun, an equal number reverse on sun, reverse on mark, with times being recorded for each pointing on the sun. A minimum of 6 pointing (3D and 3R) on the sun is recommended. The multiple times, multiple horizontal circle readings on the sun and the two horizontal circle readings on the back-sight mark constitute one observation. The multiple foresight procedure is based on the assumption that pointings on the sun are significantly less accurate than back-sight pointings. The multiple foresight procedure allows for a greater number of pointings on the sun during a shorter time span [5].
Since a large difference usually exists between the vertical angle to the back-sight mark and the vertical angle to the sun, it is imperative that an equal number of both direct and reverse pointings be taken. This is even more important when using an objective lens filter. Also, the filter should not be removed or rotated between direct and reverse pointings on the sun. When reducing the data to compute the horizontal angles, the direct reading on the back-sight mark should always be subtracted from the direct foresight reading on the sun. Likewise, the reverse back-sight reading should always be subtracted from the reverse foresight reading, and add 360˚ if the resulting angle is negative. The vertical angle to the sun is usually larger than for typical surveying work. This increases the importance of accurately leveling the instrument. Because of this and other errors, it is recommended that observations not be made when the altitude of the sun is greater than approximately 45˚ [6].
2. Field Procedure and Data Collection
A Sun observation for azimuth field work was carried out on April 17, 2007 in a study area located in the University of Central Florida campus in Orlando, Florida (Figure 1). The geodetic coordinates of Station Rogers that marks the beginning of the line Rogers-P as shown in the figure are (E(l): 82˚22′16.82717″, N(Φ): 36˚18′00.43217″). The multiple foresight field procedure described below was used and three sets of data were collected with the telescope direct and reversed for the line in question (Table 2).
The field procedure started by switching the power of the shortwave receiver on. The correction DUT is encoded over the WWV station by using double clicks after
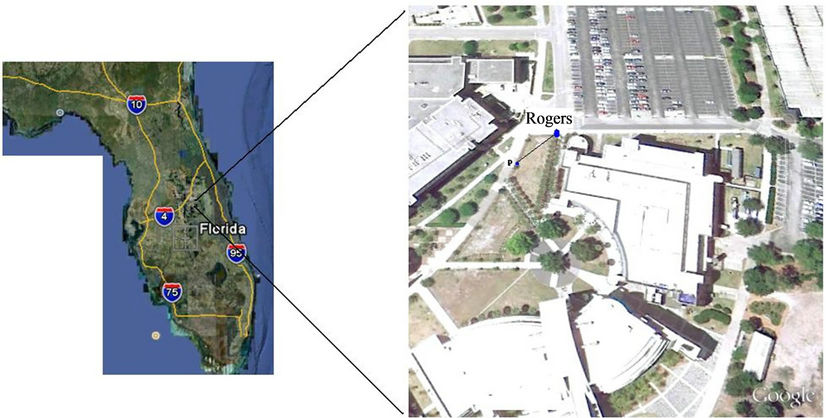
Figure 1. Study area—UCF main campus, Orlando, FL.
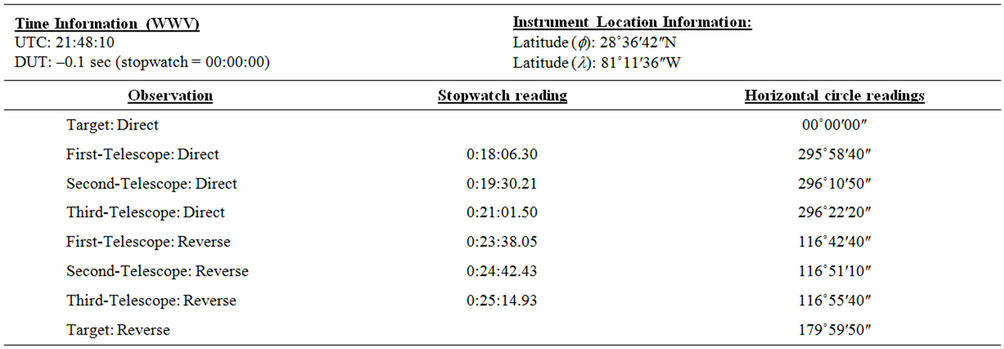
Table 2. Sun observation for azimuth data: stopwatch readings and horizontal Circle’s readings.
the start of each minute. If one hears double clicks for the 1st, 2nd and 3rd second, for example, the DUT correction is +0.3 seconds. Up to the 8th second, each double c1ick represents a correction of +0.1 seconds. To assign a negative sign to the correction, the code is to note what is heard starting with the 9th second. If no double clicks are heard during the first 8 seconds, one must count the double clicks, if any, from the 9th second onward to determine negative DUT and the amount. For example, if the 9th, 10th, 11th and 12th clicks are doubled, the DUT correction is –0.4 seconds, each double-click representing –0.l seconds correction.
The stop watch was started at the tone of the WWV station and the “start time” was recorded. A Trimble® M3 total station with a sun filter was set up at station P which was in clear view of the sun. Then, a backsight (BS) was taken on station Rogers and the Horizontal Angle (HA) were set to 00˚00′00″. The telescope was turned to the sun and while it is in direct (D) position, the trailing edge of the sun was pointed by allowing it to move into the vertical cross hair. Pointings are made with the vertical portion of the vertical cross hair without regard to the location of the horizontal cross hair. The HA was recorded as well as the time when readings are recorded as soon as the vertical cross hair becomes tangent to the trailing edge of the sun. To record the time that corresponds to the HA reading when the vertical hair is tangent to the trailing edge of the sun, the “split push button” of the stopwatch was used (three readings with telescope direct were taken). Then with the telescope is in this reverse (R) position, the trailing edge of the sun was pointed by allowing it to move into the vertical cross hair. The HA and the times when the readings were taken were recorded as soon as the vertical cross hair becomes tangent to the trailing edge of the sun (three readings with telescope reverse were taken). Then, a Foresight (FS) was taken at the target and the HA reading was recorded in order to be used to compute error in the total station horizontal circle.
3. Field Data Manipulation and Azimuth Computation
Below is the computations performed on the data of Table 2 in order to compute the azimuth of sun and further azimuth of the line Rogers-P shown in Figure 1 above. The summary of the computed values of the Sun’s local hour angle (y), declination (d), and azimuth (L) at the observation latitude (f) for the field data in Table 2 is shown in Table 3.
Correction to stopwatch equals UT1 when stopwatch was started:
UTC = 21 h 48 m 10.0 s DUT= –0.1 s UT1= 21 h 48 m 9.9 s (at stopwatch = 0:00:00)
From Table 5 (Appendix A), which shows Sun and Polaris ephemeris on the day Sun observation was be performed:
GHA 0 h = 180˚03′32.8″
GHA 24 h = 180˚06′59.4″
Decl 0 h = 10˚15′55″
Decl 24 h = 10˚37′03.5″
Sun’ semi-diameter = 0˚15′56.1″.
3.1. First Pointing with Telescope Direct
UT1 = 18 m 6.3 s + 21 h 48 m 9.9 s = 22 h 6 m 16.2 s GHA = GHA 0 h + (GHA 24 h – GHA 0 h + 360) (UT1/24) = 180˚03′32.8″ + (180˚06′59.4″ – 180˚03′32.8″ + 360) (22 h 6 m 16.2 s/24) = 511˚40′46.08″ = 151˚40′ 46.08″
LHA = GHA – lw = 151˚40′46.08″ – 81˚11′36″ = 70˚ 29′10.08″
Decl = Decl 0 h + (Decl 24 h – Decl 0 h) (UT1/24) + (0.0000395) (Decl 0 h) sin(7.5 UT1) = 10˚15′55″ + (10˚ 37′03.5″ – 10˚15′55″) (22 h 6 m 16.2 s/24) + (0.0000395) × (10˚15′ 55″) × sin(7.5 × 22 h 6 m 16.2 s) = 10˚35′23.67″
AZsun =  = –89˚44′46.98″
= –89˚44′46.98″
Since LHA is between 0˚ to 180˚, and AZsun is negative, the normalized correction equals 360˚.
AZsun = 270˚15′13.02″
D Ang Rt = 295˚58′40″ – 0˚00′00.0″ = 295′58′40″
h = sin–1(sin28˚36′42″sin10˚35′23.67″cos28˚36′42″cos 10˚35′23.67″cos70˚29′10.08″) = 22˚06′7.03″
dH = (sun’s semi-diameter)/cosh = (0˚15′56.1″)/22˚06′ 7.03″ = 0˚17′11.93″
Left edge pointed D & R; therefore the correction dH is positive.
Ang Rt = R Ang Rt + dH= 295˚58′40″ + 0˚17′11.93″ = 296˚15′51.93″
AZL = AZsun + 360 – Ang Rt = 270˚15′13.02″ + 360 –
296˚15′51.93″ = 333˚59′21.09″.
3.2. Second Pointing with Telescope Direct
UT1 = 19 m 30.21 s + 21 h 48 m 9.9 s = 22 h 7 m 40.11 s GHA = GHA 0 h + (GHA 24 h – GHA 0 h + 360) (UT1/24) = 180˚03′32.8″ + (180˚06′59.4″ – 180˚03′32.8″ + 360) (22 h 6 m 16.2 s/24) = 512˚01′44.93″ = 152˚01′ 44.93″
LHA = GHA – lw = 152˚01′44.93″ – 81˚11′36″ = 70˚ 50′8.93″
Decl = Decl 0 h + (Decl 24 h – Decl 0 h) (UT1/24) + (0.0000395) (Decl 0 h) sin(7.5 UT1) = 10˚15′55″ + (10˚ 37′03.5″ – 10˚15′55″) (22 h 7 m 40.11 s/24) + (0.0000395) × (10˚15′55″) × sin(7.5 × 22 h 7 m 40.11 s) = 10˚35′24.9″
AZsun = 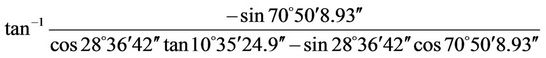 = –89˚34′45.59″
= –89˚34′45.59″

Table 3. Summary of the computed values of the sun’s local hour angle (y), declination (d), and azimuth (L) at the observation latitude (f) for the field data in Table 1.
Since LHA is between 0˚ to 180˚, and AZsun is negative, the normalized correction equals 360˚.
AZsun = 270˚25′14.41″
D Ang Rt = 296˚10′50″ – 0˚00′00.0″ = 296˚10′50″
h = sin–1(sin28˚36′42″sin10˚35′24.9″cos28˚36′42″cos 10˚35′24.9″cos70˚50′8.93″) = 21˚47′42.48″
dH = (sun’s semi-diameter)/cosh = (0˚15′56.1″)/21˚47′ 42.48″ = 0˚17′9.71″
Left edge pointed D & R; therefore the correction dH is positive.
Ang Rt = R Ang Rt + dH= 296˚10′50″ + 0˚17′9.71″ = 296˚27′59.71″
AZL= AZsun + 360 – Ang Rt = 270˚25′14.41″ + 360 –
296˚27′59.71″ = 333˚57′14.7″.
3.3. Third Pointing with Telescope Direct
UT1 = 21 m 1.5 s + 21 h 48 m 9.9 s = 22 h 9 m 11.4 s GHA = GHA 0 h + (GHA 24 h – GHA 0 h + 360) (UT1/24) = 180˚03′32.8″ + (180˚06′59.4″ – 180˚03′32.8″ + 360) (22 h 9 m 11.4 s/24) = 512˚24′34.5″ = 152˚24′34.5″
LHA = GHA – lw = 152˚24′34.5″ – 81˚11′36″ = 71˚ 12′58.5″
Decl = Decl 0 h + (Decl 24 h – Decl 0 h) (UT1/24) + (0.0000395) (Decl 0 h) sin(7.5 UT1) = 10˚15′55″ + (10˚ 37′03.5″ – 10˚15′55″) (22 h 9 m 11.4 s/24) + (0.0000395) × (10˚15′55″) × sin(7.5 × 22 h 9 m 11.4 s) = 10˚35′26.24″
AZsun = 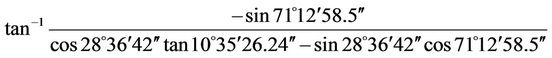 = –89˚23′52.71″
= –89˚23′52.71″
Since LHA is between 0˚ to 180˚, and AZsun is negative, the normalized correction equals 360˚.
AZsun = 270˚36′7.33″
D Ang Rt = 296˚22′20″– 0˚00′00.0″ = 296˚22′20″
h = sin–1(sin28˚36′42″sin10˚35′24.9″cos8˚36′42″cos10˚ 35′24.9″cos70˚50′8.93″) = 21˚27′40.8″
dH = (sun’s semi-diameter)/cosh = (0˚15′56.1″)/21˚27′ 40.8″ = 0˚17′7.33″
Left edge pointed D & R; therefore the correction dH is positive.
Ang Rt = R Ang Rt + dH = 296˚22′20″ + 0˚17′7.33″ = 296˚39′27.33″
AZL = AZsun + 360 – Ang Rt = 270˚25′14.41″ + 360 – 296˚39′27.33″ = 333˚56′21.09″
3.4. First Pointing with Telescope Reverse
UT1 = 23 m 38.05 s +21 h 48 m 9.9 s= 22 h 11 m 49.95 s GHA = GHA 0 h + (GHA 24 h – GHA 0 h + 360) (UT1/24) = 180˚03′32.8″ + (180˚06′59.4″ – 180˚03′32.8″ + 360) (22 h 11 m 49.95 s/24) = 513˚03′43.13″ = 153˚03′ 43.13″
LHA = GHA – lw = 153˚03′43.13″ – 81˚11′36″ = 71˚ 52′7.13″
Decl = Decl 0 h + (Decl 24 h – Decl 0 h) (UT1/24) + (0.0000395) (Decl 0 h) sin(7.5 UT1) = 10˚15′55″ + (10˚ 37′03.5″ – 10˚15′55″) (22 h 11 m 49.95 s/24) + (0.0000395) × (10˚15′55″) × sin(7.5 × 22 h 11 m 49.95 s) = 10˚35′ 28.53″
AZsun = 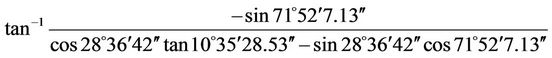 = –89˚5′16.37″
= –89˚5′16.37″
Since LHA is between 0˚ to 180˚, and AZsun is negative, the normalized correction equals 360˚.
AZsun = 270˚54′43.63″
R Ang Rt = 116˚42′40″ – 179˚59′58″ = –63˚17′18″ = 296˚42′42″
h = sin–1(sin28˚36′42″sin10˚35′28.53″ + cos28˚36′42″ cos10˚35′28.53″cos71˚52′7.13″) = 20˚53′20.1″
dH = (sun’s semi-diameter)/cosh = (0˚15′56.1″)/cos20˚ 53′20.1″ = 0˚17′3.36″
Left edge pointed D & R; therefore the correction dH is positive.
Ang Rt = R Ang Rt + dH = 296˚42′42″+ 0˚17′3.36″ = 296˚59′45.36″
AZL = AZsun + 360 – Ang Rt = 270˚54′43.63″ + 360 – 296˚59′45.36″ = 333˚54′58.27″
3.5. Second Pointing with Telescope Reverse
UT1 = 24 m 42.43 s +21 h 48 m 9.9 s = 22 h 12 m 52.33 s GHA = GHA 0 h + (GHA 24 h – GHA 0 h + 360) (UT1/24) = 180˚03′32.8″ + (180˚06′59.4″ – 180˚03′32.8″ + 360) (22 h 12 m 52.33 s/24) = 513˚19′48.02″ = 153˚ 19′48.98″
LHA = GHA – lw = 153˚19′48.98″ – 81˚11′36″ = 72˚ 08′12.98″
Decl = Decl 0 h + (Decl 24 h – Decl 0 h) (UT1/24) + (0.0000395) (Decl 0 h) sin(7.5 UT1) = 10˚15′55″ + (10˚ 37′03.5″ – 10˚15′55″) (22 h 12 m 52.33 s/24) + (0.0000395) × (10˚15′55″) × sin(7.5 × 22 h 12 m 52.33 s) = 10˚35′ 29.47″
AZsun = 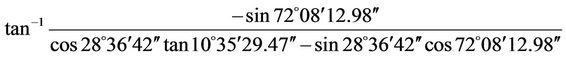 = –88˚57′38.43″
= –88˚57′38.43″
Since LHA is between 0˚ to 180˚, and AZsun is negative, the normalized correction equals 360˚.
AZsun = 271˚2′21.57″
R Ang Rt = 116˚51′1″ – 179˚59′58″ = –63˚08′48″ = 296˚05′12″
h = sin–1(sin28˚36′42″sin10˚35′29.47″ + cos28˚36′42″ cos10˚35′29.47″cos72˚08′12.98″) = 20˚39′12.83″
dH = (sun’s semi-diameter)/cosh = (0˚15′56.1″)/20˚39′ 12.83″ = 0˚17′1.77″
Left edge pointed D & R; therefore the correction dH is positive.
Ang Rt = R Ang Rt + dH = 296˚51′12″ + 0˚17′1.77″ = 297˚08′13.77″
AZL = AZsun + 360 – Ang Rt = 271˚2′21.57″ + 360 – 297˚08′13.77″ = 333˚54′7.8″.
3.6. Third Pointing with Telescope Reverse
UT1 = 25 m 14.93 s +21 h 48 m 9.9 s = 22 h 13 m 24.83 s GHA = GHA 0 h + (GHA 24 h – GHA 0 h + 360) (UT1/24) = 180˚03′32.8″ + (180˚06′59.4″ – 180˚03′32.8″ + 360) (22 h 13 m 24.83 s/24) = 513˚27′56.56″ = 153˚27′ 56.56″
LHA = GHA – lw = 153˚27′56.56″ – 81˚11′36″ = 72˚ 16′20.56″
Decl = Decl 0 h + (Decl 24 h – Decl 0 h) (UT1/24) + (0.0000395) (Decl 0 h) sin(7.5 UT1) = 10˚15′55″ + (10˚ 37′03.5″ – 10˚15′55″) (22 h 13 m 24.83 s/24) + (0.0000395) × (10˚15′55″) × sin(7.5 × 22 h 13 m 24.83 s) = 10˚35′ 29.95″.
AZsun = 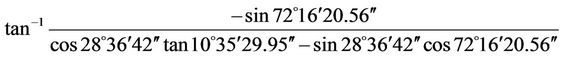 = –88˚53′47.49″
= –88˚53′47.49″
Since LHA is between 0˚ to 180˚, and AZsun is negative, the normalized correction equals 360˚.
Zsun = 271˚6′12.51″
R Ang Rt = 116˚55′40″ – 179˚59′58″ = –63˚04′18″ = 296˚55′42″
h = sin–1(sin28˚36′42″sin10˚35′9.95″cos 28˚36′42″cos 10˚35′9.95″cos 72˚16′20.56″) = 20˚32′5.08″
dH = (sun’s semi-diameter)/cosh = (0˚15′56.1″)/20˚32′ 5.08″ = 0˚17′0.97″
Left edge pointed D & R; therefore the correction dH is positive.
Ang Rt = R Ang Rt + dH= 296˚55′42″ + 0˚17′0.97″ = 297˚12′42.97″
AZL = AZsun + 360 – Ang Rt = 271˚6′12.51″ + 360 – 297˚12′42.97″ = 333˚53′29.54″.
4. Adjustment of Measurements and Error Modeling
Like all types of measurements in surveying, errors in azimuth determination are three types: systematic, mistakes, and random errors. In this study, only random errors in azimuth determination using the hour angle are addressed through modeling. Suppose we have a nonlinear function written as:
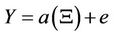 ,
,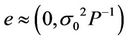 (2)
(2)
Before we continue to solve this problem, we need to transform Equation (2) into linear form. A common way to perform this transformation is a linearization using Taylor’s series expansion. Equation (2) can be linearized in the form shown below:
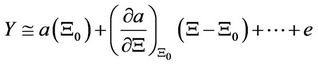 (3)
(3)
where:
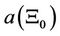 : Value of the function
: Value of the function 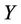 evaluated from parameter approximations
evaluated from parameter approximations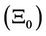 ,
,
 : vector of the differences between value of unknowns parameters and there approximation.
: vector of the differences between value of unknowns parameters and there approximation.
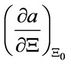 : Jacobian matrix, which represents the partial derivatives of all functions in
: Jacobian matrix, which represents the partial derivatives of all functions in 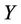 with respect to each of the unknown variables.
with respect to each of the unknown variables.
The higher-order term in Equation (3) can be dropped because it is very small and, therefore, the Equation (3) can be re-written as:
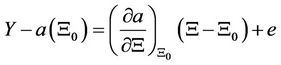 (4)
(4)
Equation (4) can be rewritten in the form of GaussMarkov model, which is:
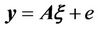 ,
,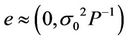 (5)
(5)
where:
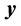 :
: , which is s vectorA:
, which is s vectorA: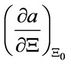 , which is a matrix,
, which is a matrix,
 :
: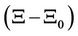 , which is a vector.
, which is a vector.
The least-squares solution for Equation (5), whose the number of observations is larger than that of the unknown parameters, can be obtained by forming an 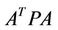 matrix and calculating its inverse and multiplying by
matrix and calculating its inverse and multiplying by 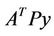 vector. This solution can be written as:
vector. This solution can be written as:
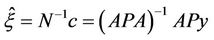 (6)
(6)
However, this solution requires that the inverse; 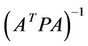 exists, which means that the rank of
exists, which means that the rank of 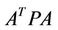 matrix must be greater or equal to the number of unknown parameters. The variance of each unknown parameter is given in the form of variance/covariance matrix, which can be computed as:
matrix must be greater or equal to the number of unknown parameters. The variance of each unknown parameter is given in the form of variance/covariance matrix, which can be computed as:
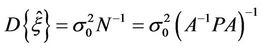 (7)
(7)
where 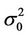 is the variance of unit weight, and its residual vector is:
is the variance of unit weight, and its residual vector is:
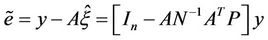 (8)
(8)
where:
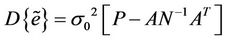 (9)
(9)
The variance of the whole adjustment is an unbiased estimate of the error of the fit can be written as follows; given that the observations are statistically independent:
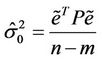 (10)
(10)
Since the Sun azimuth shown in Equation (1) is nonlinear, it needs to be linearlized using Taylor’s series expansion as shown below [7]:
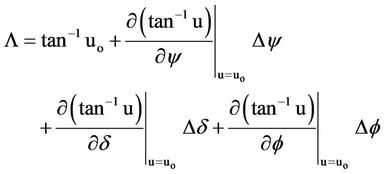 (11)
(11)
where 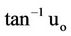 is value of
is value of 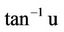 at
at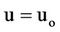 ,
, 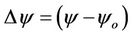 ,
, 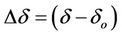 and
and 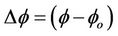
Since , then:
, then:
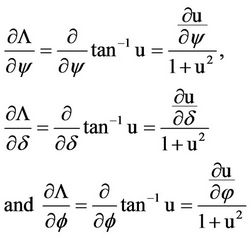 (12)
(12)
The partial derivatives of the function  with respect to the variables y, d and f are shown below:
with respect to the variables y, d and f are shown below:
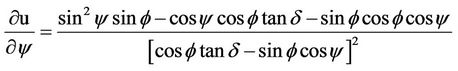 (13a)
(13a)
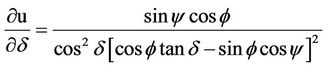 (13b)
(13b)
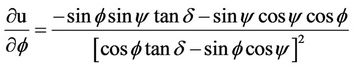 (13c)
(13c)
Accordingly, we can obtain the following partial derivatives of 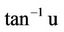 with respect to y, d and f respectively:
with respect to y, d and f respectively:
 (14a)
(14a)
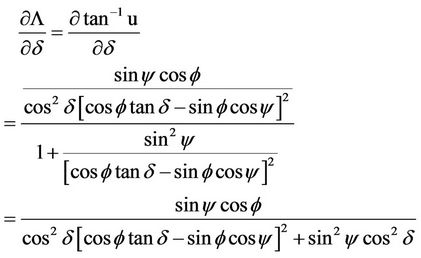 (14b)
(14b)
 (14c)
(14c)
The values of and the partial derivatives in Equation (14) at are as follows:
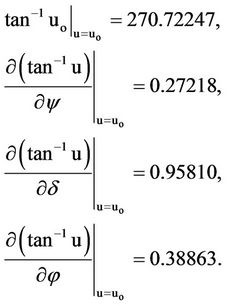 (15)
(15)
Table 4 shows the values of the d(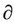 tan–1u)/dy, d(
tan–1u)/dy, d(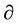 tan–1u)/dd, and d(
tan–1u)/dd, and d(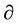 tan–1u)/df for the data shown in Table 2.
tan–1u)/df for the data shown in Table 2.
Re-writing Equation (11) after substituting the values obtained in (15) yields:
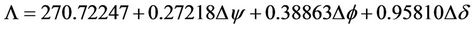 (16)
(16)
Note that Equation (16) resembles the Gauss-Markov model presented earlier in Equation (4). Therefore it presents a linear model of the sun azimuth (Λ) derived from the data collected in the study area. The data model introduces the sun azimuth as a function of the random errors in the longitude and latitude of the geographic location along with that in the sun declination angle. Those errors are essential and need to be determined anyway before using them. Then the corrected value of the sun azimuth (Λ) that can be obtained using Equation (16) should be used to get the azimuth of the line in question using the procedure outlined and adopted in the calculations of the line azimuth in our study area (refer to the Field Data Manipulation and Azimuth Computation section). The errors in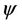 ,
,  and
and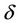 , which are
, which are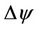 ,
,  and
and 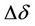 can then be substituted in Equation (16) to compute an adjusted value of the sun azimuth (Λ) and further use that to compute corrected value of the astronomic/true azimuth of the line in question as shown in the study area.
can then be substituted in Equation (16) to compute an adjusted value of the sun azimuth (Λ) and further use that to compute corrected value of the astronomic/true azimuth of the line in question as shown in the study area.
In this study, the values of  and
and 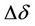 have been obtained as: ±0.73241 and ±0.00071 degrees respectively using the values of the three sets of field measurements (three Direct and three Reverse) acquired in this study. The value of
have been obtained as: ±0.73241 and ±0.00071 degrees respectively using the values of the three sets of field measurements (three Direct and three Reverse) acquired in this study. The value of  was obtained independently as ±0.00344 degrees using a Topcon Hiperlite + GPS unit. Applying these values into Equation (16) above will yield the following most probable sun azimuth (Λ):
was obtained independently as ±0.00344 degrees using a Topcon Hiperlite + GPS unit. Applying these values into Equation (16) above will yield the following most probable sun azimuth (Λ):
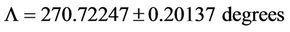 (17)
(17)
This is translated into the following most probable value of the true/astronomic azimuth of the Rogers-P line in the study area: AZ = 333.93206 ± 0.20137 degrees.
5. Conclusion
The linear error model derived in this study can be used to assess the quality of the computed sun azimuth, which is needed to determine the astronomic/true azimuth of a line at a location using the hour angle method. The error modeling framework presented in this article is essential for those seeking an improved accuracy of the estimated value of the sun azimuth. Although the model is data driven and that a little data analysis and manipulation is required to arrive at the model, the robustness of the outcome worth the efforts put forth in the process.
Appendix A
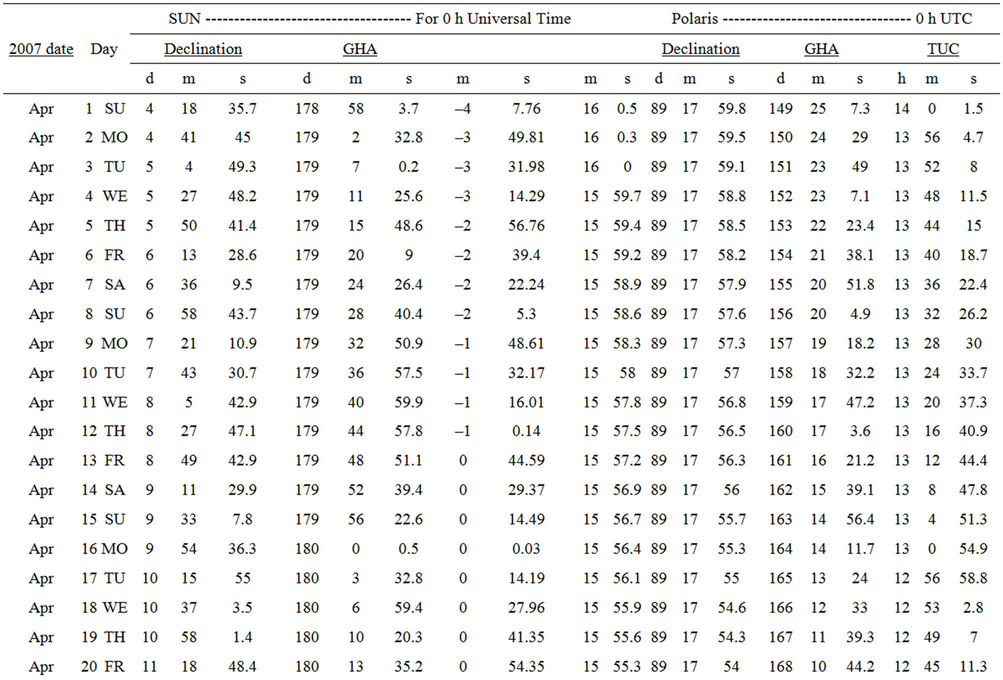
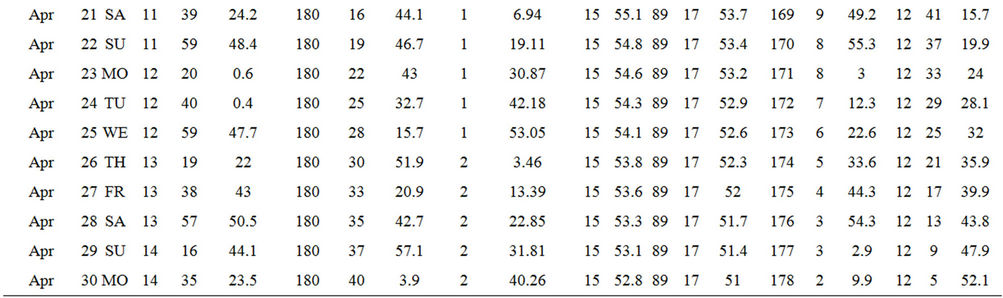
Table 5. April 2007 sun and Polaris ephemeris (Source: http://www.cadastral.com).