Nonconforming Mixed Finite Element Method for Nonlinear Hyperbolic Equations ()
1. Introduction
In this paper, we discuss a nonconforming mixed finite element method for the following nonlinear hyperbolic initial and boundary value problem.
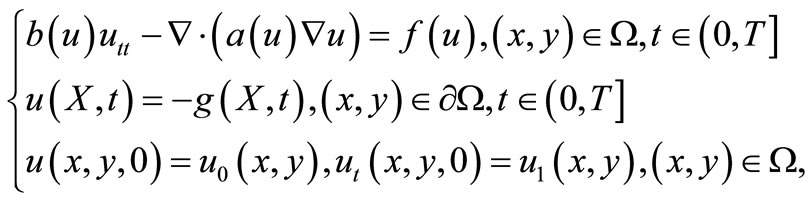(1)
In order to describe the results briefly, we suppose that Equation (1) satisfy following assumptions on the data:
1) 
and 
are smooth and there exist constants 
and 
satisfying
.
2) 
and 
are sufficiently smooth functions with bounded derivatives.
There have been many very extensive studies about this kind of hyperbolic equations. For example, [1-3] studied the linear situations and gave error estimates under semi-discrete and fully-discrete schemes by standard Galerkin methods. [4,5] considered the mixed finite element methods for linear hyperbolic equations and obtained L2 prior estimates about continuous time. In addition, [6] analyzed the mixed finite element methods for second order nonlinear hyperbolic equations. But all the above investigations are mainly about conforming situations and projections are indispensable. As we know, the nonconforming finite element methods arise because of the demands for reducing the calculation cost. [7] has pointed that the nonconforming finite element methods with degree of freedom defined on the element edges or element itself are appropriate for each degree of freedom belong to at most elements.
In the present work, we focus on the nonconforming mixed finite element approximation scheme for nonlinear hyperbolic equations. Firstly, we introduce the corresponding space and the interpolation operators. Secondly, Existence and uniqueness of the solutions to the discrete problem are proved. Finally, Priori estimates of optimal order are derived for both the displacement and the stress.
Throughout this paper, C denotes a general positive constant which is independent of
, and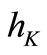 
is the diameter of the finite element K.
2. Construction of the Elements
Let 
be the a rectangular subdivision of
and 
satisfy the regular condition. For every K, let 
be the barycenter, the length of edges parallel to x-axis and y-axis by 
and
. Then there exists an affine mapping 
where 
is the reference element in 
plane and 
is the edges. We define the finite element 
on 
as
,
,
.
The interpolation functions defined above are properly and can be expressed as:
,
,
. And For every
, the associated finite element spaces as
,
where 
denotes the jump of w across the boundary F, and
, if
. 
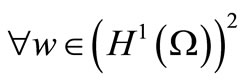, the interpolation operators:
.
.
3. Main Results in Semi-Discrete Scheme
In this section, we will give the main results in this paper, including the existence and uniqueness of the solution to the discrete problem and priori estimates of optimal order.
Firstly, we introduce
and rewrite the Equation (1) as a system:
Secondly, for our subsequent use, we employ the classical Sobolev space 
with norm
. When
, we simply write 
as
. Furthermore, we denote the natural inner production in 
by 
and the norm by
, and let
,
.
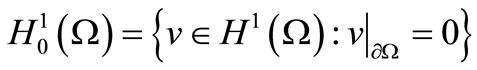Thus the corresponding weak formulation of Equation (1) is to find a pair of
, such that
satisfying
(2)
where
.
The semi-discrete mixed finite element procedure is determined:
, such that
(3)
where
. We define that
,
.
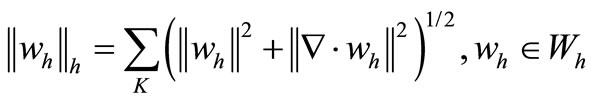It can be seen that 
and 
are the norms for 
and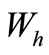
, respectively.
Theorem 1. The above problem (3) has a unique solution.
Proof: Let 
and 
are bases of 
and
, which satisfy
Then semi-discrete scheme can be rewritten as: Find 
and
, such that for every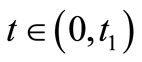 
satisfy
here
, 
,
, 
By the definition of the approximation spaces, we know that E is reversible and
. Thus there holds that
.
Since 
and 
are Lipschitz continuous, it has a unique solution according to the theory of differential equations [8].
Lemma 1. For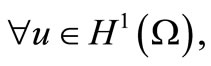 
there hold that
Proof: Firstly, by the interpolation condition and definition, it is easy to see that 
and
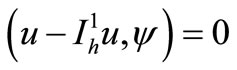. Secondly, for every
, v is a constant, by application of Green’s formula and the interpolation definition yields that
Thus, we complete the proof of Lemma 1.
Lemma 2. [9] For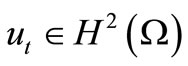
, there hold that
, 
Now we give the main result of this paper.
Theorem 2. Let 
and 
be the solutions of Equations (2) and (3), respectively. For 
, there hold that
Proof: Let
,
.
It is easy to see that 
and 
satisfy the following error equations
(4)
and
(5)
Using derivation about time t of Equation (4), combining Equation (5), we obtain
(6)
Choosing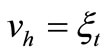 
and 
in Equation (6), and integrating from 0 to t, we have
(7)
We will give the analysis result of Equation (7) in detail. Firstly, by the initial condition, it is followed that
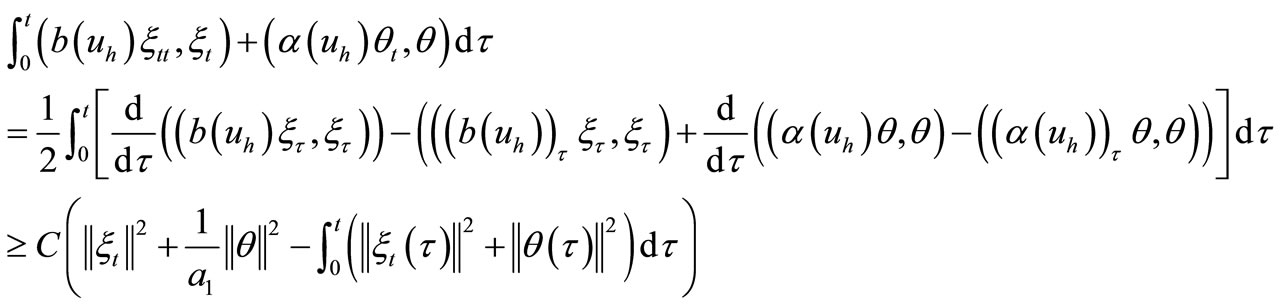(8)
Secondly, by Cauchy-Schwartz’s inequality and Young’s inequality, we obtain
(9)
Similarly, by the initial condition of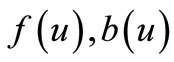 
and
, we use Young’s inequality to get
(10)
Substituting the above estimates, and applying Lemma 2, we get
(11)
Then adding 
at both sides Equation (11), and noticing that
, we obtain that
Further, using Gronwall’s inequality to yield
By the interpolation theory (see [10]), we have
.
Finally, by the triangle inequality, we complete the proof.