Three-Dimensional Free Vibration Analysis of a Viscothermoelastic Hollow Sphere ()
1. Introduction
The exact three-dimensional analysis of free vibrations of elastic spherical structures is well established in [1-4]. The coupled theory of thermoelasticity proposed by Lord and Shulman [5] incorporates a flux-rate term into the Fourier Law of heat conduction and involves a hyperbolic-type heat transport equation admitting wave type thermal signals. Green and Lindsay [6] formulated temperature-rate-dependent thermoelasticity by introducing relaxation time that reckons a finite speed of heat propagation. Hetnarski and Ignaczac [7] studied the response of a semi-space due to a short laser pulse in context of generalized thermoelasticity. Buchanan and Ramirez [8] computed the free vibration frequencies for solid ellipsoids by using Ritz method. Sharma and Sharma [9] studied vibrations of a transradially isotropic coupled thermoelastic solid sphere by using matrix Fröbenius method. Neuringer [10] developed the procedure of Fröbenius method when the roots of indicial equation are complex. Several mathematical models [11,12] have been used to accommodate the energy dissipation is due to internal friction in vibrating viscoelastic solids. Moreover the Kelvin-Voigt model is one of the macroscopic mechanical models which is also used to describe the viscoelastic behavior of a material. Mukhopadhyay [13] studied the effect of thermal relaxation time on viscothermoelastic interactions in an unbounded body with a spherical cavity subjected to periodical loading. Sharma [14] investigated the propagation of waves in an infinite Kelvin-Voigt type viscoelastic plate in the context of coupled thermoelasticity.
This paper is devoted to the exact three-dimensional vibration analysis of homogenous isotropic, viscothermoelastic hollow sphere subjected to 1) stress free thermally insulated and 2) stress free isothermal conditions. The potential function technique has been employed to decouple purely shear motion which remains independent of thermal variations. Upon using separation of variable technique, the problem is reduced to a system of four ordinary differential equations. In order to obtain frequency equation as second class (spheroidal) vibrations the coupled system have been solved by using Matrix FRÖBENIUS series method. The fixed point iteration numerical technique with the help of MATLAB software tools is used to compute frequency and damping of the vibrations. The computer simulated results in respect of lowest frequency, dissipation factor, stresses, displacements and temperature change have been presented graphically of the hollow sphere.
2. Mathematical Model
We consider a homogenous isotropic thermally conducting, viscothermoelastic hollow sphere of outer radius a and inner radius b initially at uniform temperature 
in the undisturbed state. The basic governing equations of motion and heat conduction for displacement 
and temperature change 
in spherical polar coordinates
, in the absence of body forces and heat sources, are given by [15]
(1)
(2)
where 
(3)
(4)
(5)
Here 
and
, 
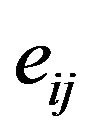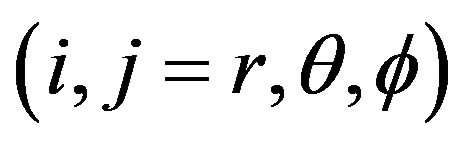are the stress and strain components, respectively; 
is the thermoelastic coupling constant, 
are the viscothermoelastic relaxation times; 
are Lame’s parameters; 
is the coefficient of linear thermal expansion; 
is mass density; 
is the specific heat at constant strain; K is the thermal conductivity; 
and 
are the thermal relaxation times. The quantity
, 
is Kronecker’s delta in which 
corresponds to Lord-Shulman (LS) and 
refers to Green-Lindsay (GL) theory of thermoelasticity. The superposed dots represent time differentiation and comma notion is used for spatial derivatives.
Boundary Conditions
We consider the free vibrations of the sphere which is subjected to stress free, thermally insulated and isothermal conditions and 
(outer radius) and 
(inner radius) of the hollow sphere. Mathematically this provides us
(6)
In order to simplify the model, we define following quantities
(7)
where 
are longitudinal, shear wave velocities and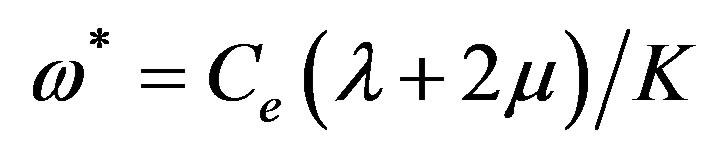 
is characteristic frequency of the medium, 
is thermo-mechanical coupling constant. The primes have been suppressed for convenience.
3. Solution of the Model
In order to solve the model we introduce the potential functions 
and w defined by [1]
(8)
Upon using the Equation (8) in Equations (1) to (2), we find that 
and T satisfy the non-dimensional equations
(9)
(10)
(11)
(12)
where 
We take wave solution for the displacement functions and temperature change as under
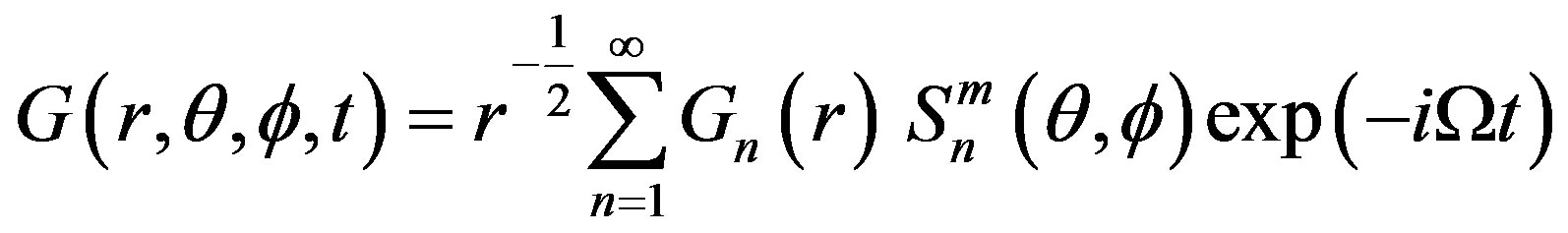(13)
where 
are the spherecal harmonics, 
be the associated Legendre polynomials; n and m are integers, 
, 
is the circular frequency. The substitution of Equations (13) into Equations (9)-(12) provides us
(14)
(15)
(16)
(17)
where
(18)
The uncoupling of Equation (17) for 
from those of 
and 
indicates the existence of two distinct classes of vibrations in the considered hollow sphere. The solution of Equation (17) for 
corresponds to the toroidal modes of vibrations which remains unaffected due to thermal variations and can be discussed in the same manner as was done by Cohen [3] if viscous effects are neglected. The solution of the spherical Bessel Equation (17) is given by
(19)
where
, 
and 
are arbitrary constants determined from arbitrary conditions.
3.1. Extended Power Series Method
In order to solve the coupled Equations (14)-(16), we apply Matrix Fröbenius method for the domain of consideration is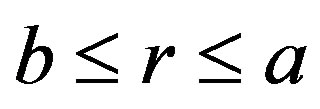
. We take power series of the type
(20)
where 
p is the eigen value and 
are unknowns to be determined.
Substituting the solution (20) in Equations (14)-(16) we get the following matrix equations
(21)
where
(22)
The non zero elements of the matrices 
and 
are given by
,
(23)
where
.
Equating to zero the coefficients of lowest powers of 
(i.e.
) in Equation (21), we obtain
(24)
where
The requirement for the existence of non-trivial solution of Equation (24) leads to the following two indicial equations
(26)
where 
The roots of Equation (26) are of the type p = ± p (i = 1, 2, 3)
where
(27)
We designate the roots of Equation (26) as 
with
. Obviously 
are real and 
may be complex in general. If 
are complex, then leading terms in the complex series solution (24) are of the type
(28)
In order to obtain two independent real solutions, it is sufficient to use any one of the complex root and taking its real and imaginary parts see Neuringer [10]. Moreover, the treatment of complex case is unlike that of the real roots with the advantage that the differential equation is required to be solved only once in the former case rather than twice in the later one. For the choice of roots of the indicial equations, the system of Equations (24) leads to following eigen vectors
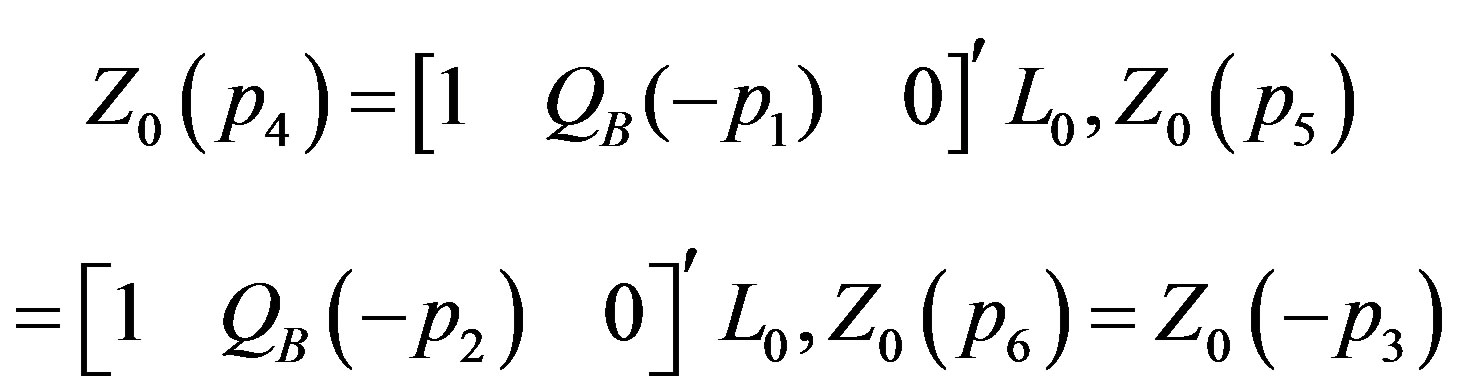(29)
where
(30)
and 
is a constant. This suggest us to have
(31)
where 
can be written from (31) by replacing 
with 
and again equating to zero the coefficients of next lowest degree term
, which corresponds to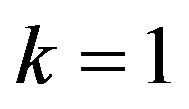
, and using Equations (21) we obtain:
(32)
Clearly 
is non-singular for each
, therefore we have:
(33)
where
(34)
are given by Equations (A.1) to (A.4) of Appendix. Here the matrices 
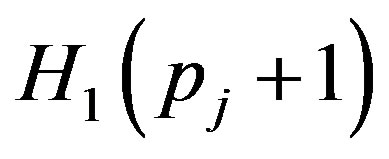and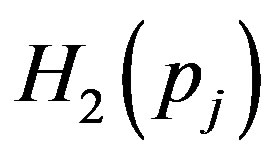 
can be written from the Equations (22) and (23) by setting 
Now equating the coefficients of powers of 
equal to zero, we obtain following recurrence relation:
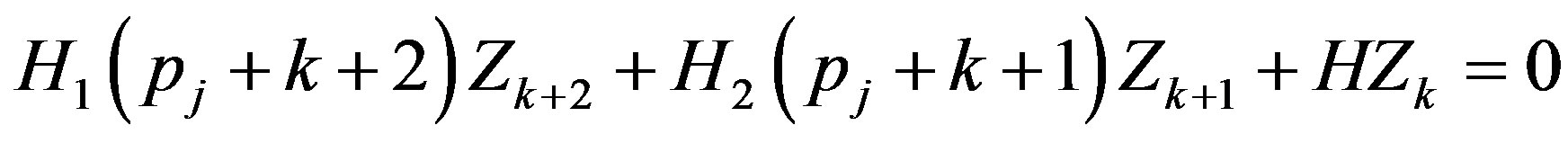(35)
where the matrices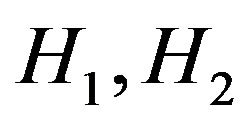 
and H are defined in Equation (22) and (23). This implies that
(36)
Now putting 
successively, and simplifying we get
where
It can be easily shown that the matrix 
has similar form to that of 
for even values of k and it is alike 
for odd values of k. Thus we have
where 
(37)
3.2. Convergence Analysis
According to Cullen [16], in case of a matrix sequence 
in
, we have 
if each of the 
component sequence converges.
After straight forward calculations and simplifications of Equation (37) we have
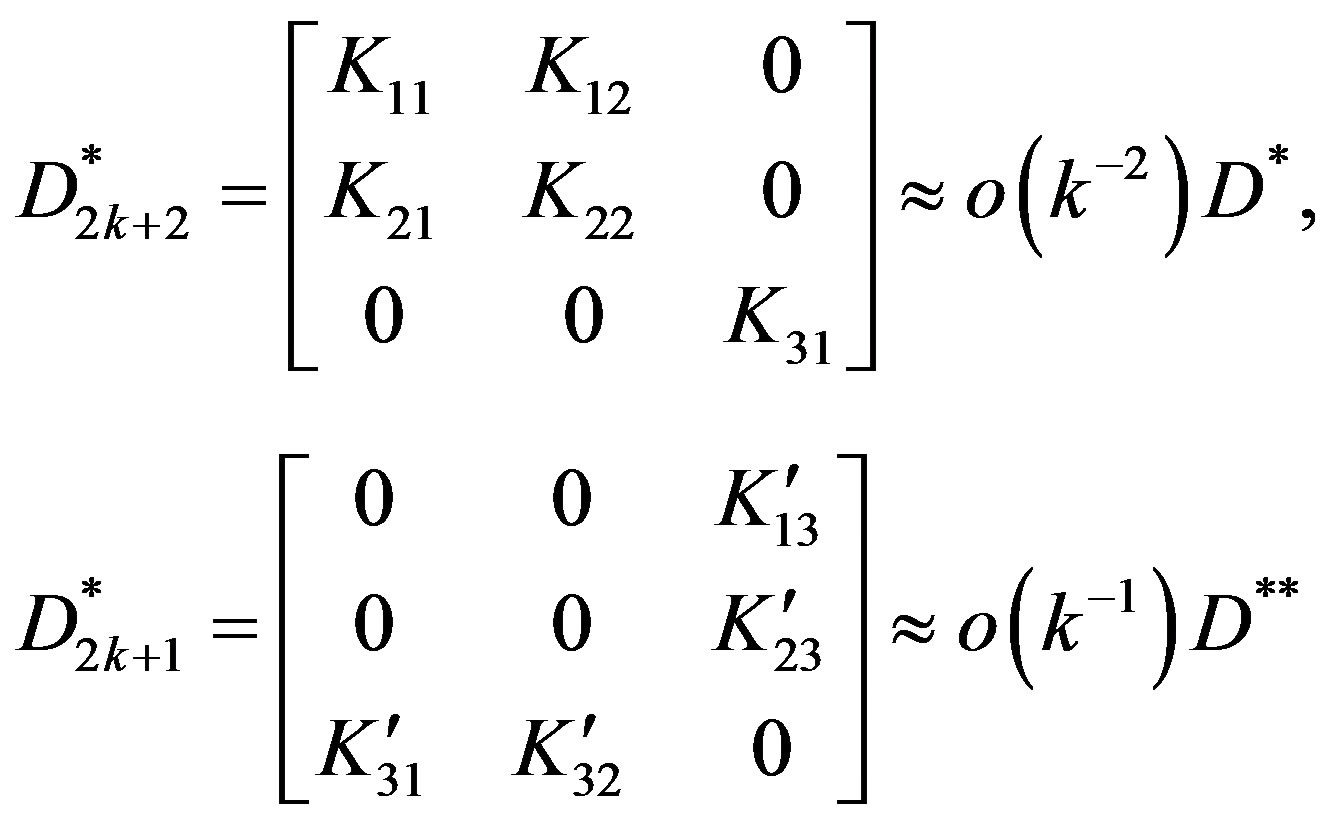
are defined by Equations (A.5)- (A.14) in Appendix.
where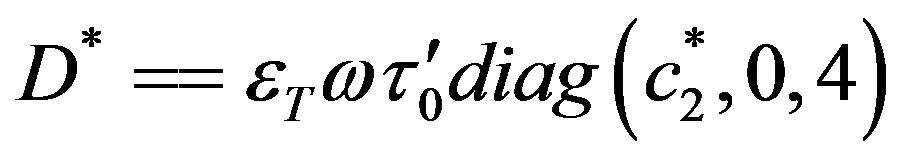 
and 
is 
null matrix. Upon using above facts, we see that both the matrices
, 
, as
. Thus the series (20) is absolutely and uniformly convergent and hence can be differentiated term by term being analytic. Moreover, the derived series are also analytic functions. Therefore, the potential functions 
and 
are given by
(38.1)
(38.2)
where 
are eigenvectors shown in Appendix (A.15) corresponding to the eigenvalues
. The unknowns 
and 
can be evaluated by four boundary conditions of hollow sphere. Displacements and stresses are obtained as
(39.1)
(39.2)
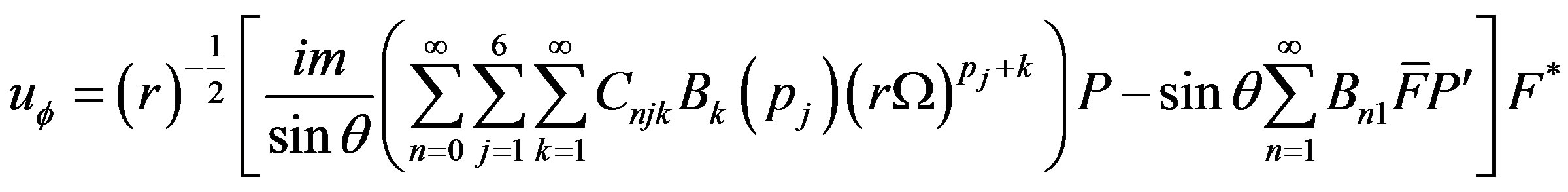(39.3)
(39.4)
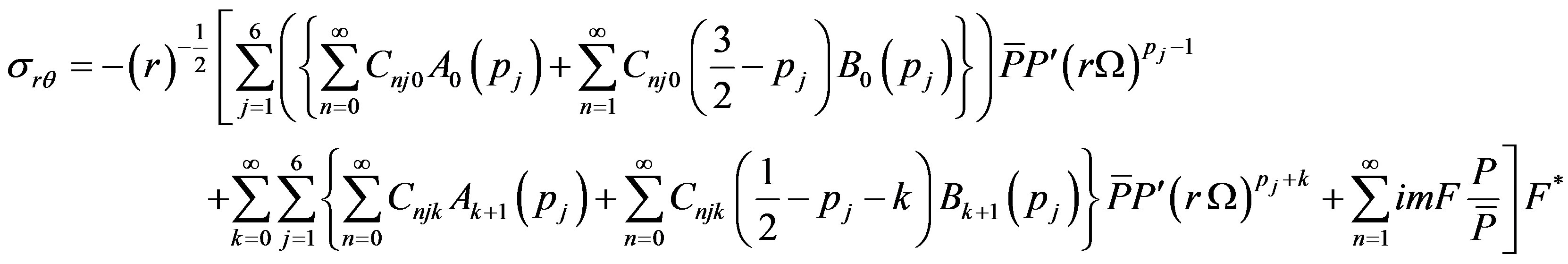(39.5)
(39.6)
where 
(39.7)
4. Secular Dispersion Relations
On applying the boundary conditions on Equations (6) we obtain a system of eight homogeneous linear algebraic equations which will have a nontrivial solution if and only if the determinant of the coefficients Bn1, Bn2 and
vanishes. This requirement of nontrivial solution leads to a determinant equations for thermally insulated hollow sphere which further splits into two different classes of vibrations discussed below.
4.1. For Stress Free Boundary Conditions
Case 1: For 
the secular equations are given as
(40)
(41)
, (42)
where 
(43.1)
(43.3)
(43.4)
Here the elements 
of Equation (40) can be written from 
by replacing 
with 
and the elements 
are obtained by replacing a with b. The element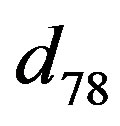 
can be obtained by replacing Bessel’s function of first kind 
with that of second kind 
in Equation (43.4) and the elements 
and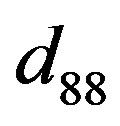 
can be obtained from 
and 
by replacing a with b, respectively.
Case 2: For 
the secular equations are obtained as
(44)
where
The elements 
of Equation (44) can be obtained by just replacing 
in 
with 
while
are obtained by replacing a in
with b.
Case 3: For 
the secular equations are given as
(45)
where
The elements of 
of determinant equation (45) can be obtained by just replacing 
in
with
while 
are obtained by replacing a in 
with b.
4.2. First Class Vibrations
The vibrations of first class correspond to the solution of Equation (38.2) of potential function 
and hence are given by Equation (41). After lengthy but straight forward calculations, the characteristic Equation (41) can be simplified by using asymptotic Expansion [3] for functions as
(46)
where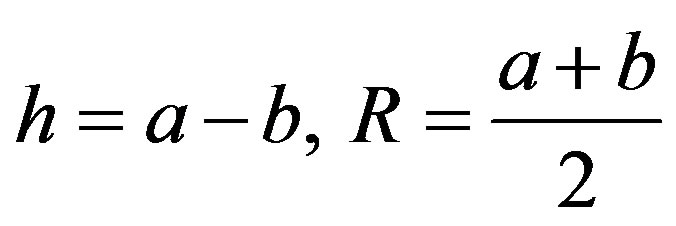 
is the mean radius.
In the limiting case of the thickness h tending to zero, we obtain from Equation (46)
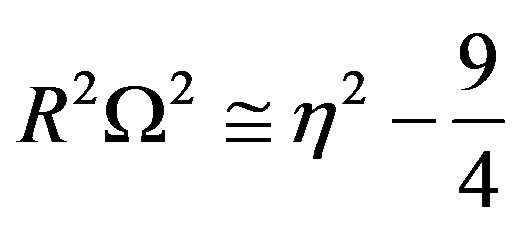(47)
These Equations (46) and (47) are of similar type reported by Cohen et al. [3] but completely agreement in the absence of viscous effect.
If we take 
the Equation (46) reduces to
(48)
(49)
(50)
4.3. Second Class Vibrations
The secular Equations (40), (44) and (45) govern the second class vibrations called spheroidal vibrations (S-modes) for Case 1. 
Case 2.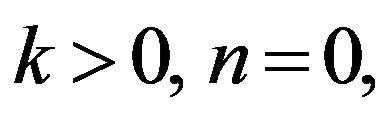 
and Case 3. 
Stress free conditions respectively.
4.4. Thermo-Elastic Hollow Sphere
If we ignore the viscous effect
, then the present analysis reduces to that of generalized thermoelastic hollow sphere. In case the thermal relaxation time is zero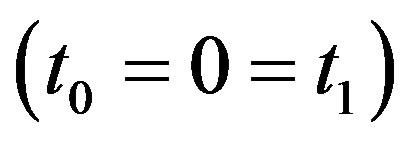
, the above results reduce to those which govern the vibrations of coupled thermoelastic hollow sphere.
4.5. Viscoelastic Hollow Sphere
If thermal equilibrium is assumed to be established then
, and present analysis reduces to one which governs the spheroidal and toroidal vibrations of a viscoelastic hollow sphere.
4.6. Elastic Sphere
When in addition to establishment of thermal equilibrium, the viscous effect in solid is ignored so that 
, then the above analysis completely reduces to one that governs the spheroidal and toroidal vibrations of an elastic hollow sphere. In this case the results are observed to be in agreement with Cohen [3].
5. Numerical Results and Discussion
In order to illustrate the analytical developments, we propose some numerical calculations to compute lowest frequency of S-modes in the hollow sphere made of copper material. The numerical computations have been carried out for spheroidal modes of vibrations for 
by using fixed point iteration numerical technique with the help of MATLAB software tools for thickness to mean radius ratio. Due to the presence of dissipation term in heat conduction Equation (2), the secular equations are, in general, complex transcendental equations and hence provide us complex values of the frequency 
and hence of
. If we write
, then the lowest frequency and dissipation factor are given by 
and
, for fixed values of n and k. The numerical computations have been done by taking sufficient number of values of the Fröbenius parameter k in order to obtain the converged values of lowest frequency 
and dissipation factor 
of S-modes. The computer simulated lowest frequency, dissipation factor, displacements; stresses and temperature change have been presented in Figures 1 to 10 for viscothermoelastic (VTE), thermoelastic (TE), viscoelastic (VE), elastic (E) materials of hollow sphere. The material copper has been taken for the computation purpose as whose physical data [14] is given as
The variations of lowest frequency 
and dissipation factor in a stress-free and thermally insulated hollow sphere of copper material versus degree of harmonics (n) have been plotted in Figures 1 and 2 at different values of thickness to mean radial ratio
, where
. It is concluded from Figure 1" target="_self"> Figure 1 that the lowest frequency increases with increase in degree of harmonics. It can be inferred from Figure 2 that with increase in degree of harmonics the dissipation of vibration modes go on increasing. Figure 3 the lowest frequency of toroidal vibrations has been plotted versus degree of harmonics (n) for different values of thickness to mean radial ratio
. From Figure 3 it is revealed that with increase in the degree of harmonics the frequency of vibrations go on increasing. The trends of the profiles in Figure 3 are similar to that as reported in Ding and Chen [17]. But the dissipation factor of toroidal vibrations is very low (of the order 10–10)
Figure 1. Lowest frequency 
of spheroidal vibrations versus degree of harmonics 
in (VTE) hollow sphere.
Figure 2. Dissipation 
of spheroidal vibrations versus degree of harmonics 
in (VTE) hollow sphere.
Figure 3. Lowest frequency 
of Toroidal vibrations versus degree of harmonics 
in (VTE) hollow sphere
in the instant case which is negligible. From the trends of variations of lowest frequency and dissipation factor of S-mode, it is noticed that the thermal variations, thermal relaxation time and viscous nature of the material significantly affect the characteristics and behavior of spherecal vibrations and their magnitudes in contrast to that of toroidal modes which are only affected due to viscosity but not by the temperature variations as expected.
In Figures 4 to 7, the variations of temperature change (T) and stresses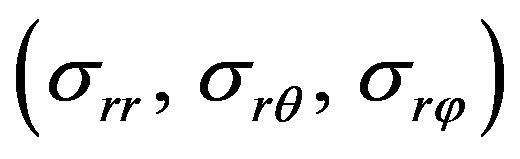 
versus 
i.e. 
(difference in radius and inner radius to thickness) for the modes 
and 
have been plotted in case of stress free and thermally insulated surface of the (VTE) sphere. Figure 4 revealed that the magnitude of temperature change is though meager, but decreases with increasing values of 
from its maximum value at 
to become steady and stable at the 
in case of all the modes. It is inferred from Figure 5 that the variations of radial stress 
for modes 
initially increases and die out with increase in values of
. But for the mode
, the stress is of compressive nature and its magnitude vanishes with increasing values of
. It is noticed from Figure 6 that the meridian stress 
of vibration modes 
has compressive nature and it dies out with increasing values of
. However the mode 
has maximum variations of this quantity and its vibrations die out with increasing values of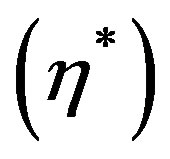
. Figure 7 shows that the variation of stress for mode
. It has maximum value and its magnitude goes on decreasing with increasing values of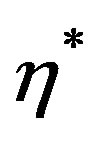 
to ultimately die out.
Figures 8 to 10 represent the variations of displacements 
versus 
for the modes
and
. Figure 8 revealed that the variations of radial displacement decrease from their maximum variation 
with increasing values of 
to become stable at 
for all modes of vibrations. Figures 9 and 10 show the variations of displacements 
and 
versus
. The profiles of
Figure 4. Variation of temperature change 
versus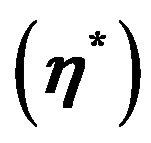 
of hollow sphere.
Figure 5. Variation of stress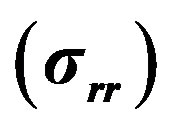 
verses 
of hollow sphere.
Figure 6. Variation of stress 
verses 
of hollow sphere.
Figure 7. Variation of stress 
verses 
of hollow sphere.
Figure 8. Variation of displacement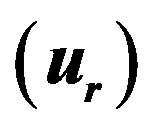 
verses 
of hollow sphere.
Figure 9. Variation of displacement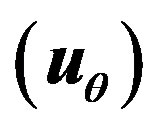 
verses 
of hollow sphere.
Figure 10. Variation between displacement 
verses 
of hollow sphere.
variations of these quantities show increasing trends with respect to increasing values of 
for all the modes of vibrations.
5.1. Numerical Data/Information
Here in Table 1 shows the Comparison of lowest frequency 
and Dissipation factor (D) in different media i.e. viscothermoelastic (VTE), thermoelastic (TE), viscoelastic (VE) and elastic (E) materials of hollow sphere and Table 2 has been given to check the validity of the numerical data by using t-test at fixed degree of harmonics 
for different values of thickness to mean radius ratio i.e.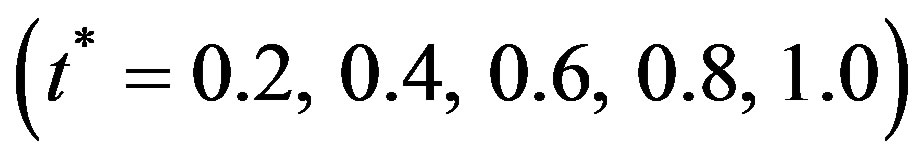 
of hollow sphere.
5.2. Statistical Analysis
In this sub section we have performed some statistical analysis of computed data for lowest frequency and dissipation factor of three harmonics
of spheroidal vibrations in hollow sphere of different thickness to mean radius ratio 
made from VTE, TE, VE and E materials. The t-test has been used during the analysis in order to examine the influence of thermal, viscous and both thermal and viscous, effects on the vibrations.
5.2.1. Lowest Frequency
We take two samples X and Y, where X denotes lowest frequency 
of each mode of vibrations in viscothermoelastic (VTE), thermoelastic (TE) or viscoelastic (VE) hollow spheres and Y represents lowest frequency 
of elastic (E) hollow sphere. The size of the sample in each case is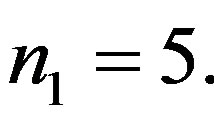 
Let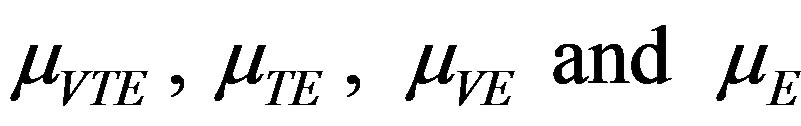 
denote
Table 1. Comparison of lowest frequency 
and dissipation factor (D) of vibrations of a hollow sphere with thickness to mean radius ratio at
.
mean values of the data representing to (VTE), (TE), (VE) and (E) hollow spheres, so that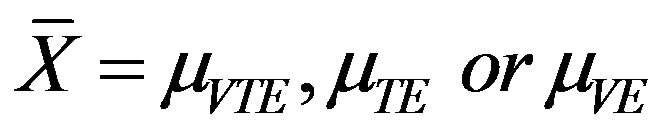 
and
. The standard deviation 
is calculated by using formula
in each case for all the considered modes. In order to test the null hypothesis 
for the consistency of means of the data pertaining to each case, we use t-test in which the t-statistic is computed from the relation
The computed values if t are given below
: 
: 
Table 2. Numerical data of lowest frequency and dissipation factor of first three harmonics 
for different values of thickness to mean radius ratio
.
: 
The tabulated values of t for 8 degree of freedom at 
level of significance is given by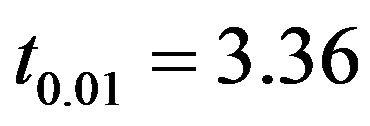
, clearly 
in each case and hence the null hypothesis 
is accepted at 
level of significance. Thus the numerical data is consistent.
5.2.2. Dissipation Factor
Here X denotes the dissipation factor (D) of (VTE) or (TE) hollow spheres and Y represents the dissipation factor (D) of (VE) hollow spheres. The size of the sample is again 
and the null hypothesis 
is tested for (VTE) and (TE) spheres versus (VE) one by adopting above procedure. The computed values of the t-statistic are given below:
: 
: 
: 
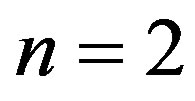Since 
in each case and hence the null hypothesis 
for is accepted at 
level of significance. Thus the numerical data is consistent.
6. Conclusion
After simplifying the system of equations of motion and heat conduction of linear coupled viscothermoelasticity for a homogenous isotropic hollow sphere with the help of Helmholtz decomposition theorem, the extended power series (Matrix Fröbenius) method is successfully used to solve the resulting system of equations. It is noticed that toroidal vibrations (T-modes) get decoupled from rest of the motion and remain free of temperature variations as expected. The lowest frequency of spherical vibrations is noticed to be significantly affected due to temperature variations in a hollow sphere of copper material. This study may be useful in tribology and geophysical industrial applications.
Appendix
The non-zero elements 
of the matrix 
in Equation (34) are given by
(A.1)
(A.2)
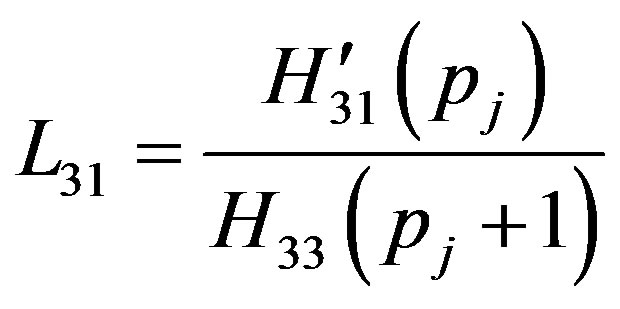(A.3)
(A.4)
The non-zero elements 
and 
of the matrix 
and 
defined in Equation (37) are obtained as
(A.5)
(A.6)
(A.7)
(A.8)
(A.9)
(A.10)
(A.11)
(A.12)
(A.13)
(A.14)
(A.15)
where
