Determination of One Unknown Thermal Coefficient through the One-Phase Fractional Lamé-Clapeyron-Stefan Problem ()
Received 1 October 2015; accepted 27 November 2015; published 30 November 2015


1. Introduction
Heat transfer problems with a phase-change such as melting and freezing have been studied in the last century due to their wide scientific and technological applications, see [1] -[8] .
A review of a long bibliography on moving and free boundary problems for phase-change materials (PCM) for the heat equation is given in [9] . The importance of obtaining explicit solutions to some free boundary problems is given in the work [10] .
We consider a semi-infinite material, with constant thermal coefficients, which is initially solid at its melting temperature Tm. At time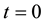 , we impose a constant temperature
, we impose a constant temperature 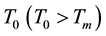 at the fixed face
at the fixed face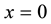 , and a solidification process begins.
, and a solidification process begins.
We consider that one of the four thermal coefficients is unknown and that it will be determined by a fractional phase-change problem by imposing an over-specified heat flux condition of the type described in [2] [11] [12] .
Fractional differential equations have been developed in the last decades, see for example the books [13] -[15] and the articles [16] -[19] , and some papers on the fractional Lamé-Clapeyron-Stefan problem are published in the last few years, see [20] -[26] .
In this paper, the differential equation and a governing condition for the free boundary include a fractional time derivative of order 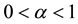 in the Caputo sense, which is defined as [27] :
in the Caputo sense, which is defined as [27] :
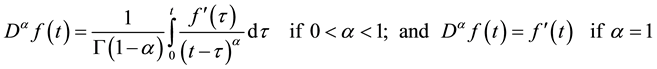 (1)
(1)
where 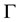 is the Gamma Function defined by:
is the Gamma Function defined by:
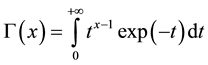 . (2)
. (2)
We also define two very important functions, which will be useful in the next section:
1) Wright Function [28] :
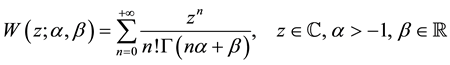 . (3)
. (3)
2) Mainardi Function [16] :
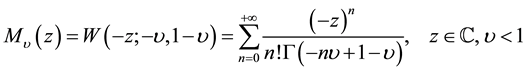 . (4)
. (4)
We note that the Mainardi Function is a particular case of the Wright Function.
Some basic properties for the Caputo fractional derivative and for the Wright Function are the following:
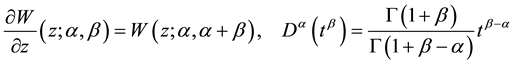 , (5)
, (5)
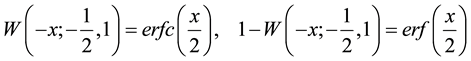 , (6)
, (6)
where the classical error and the complementary error functions are defined by:
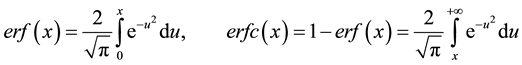 (7)
(7)
The method for the determination of unknown thermal coefficients through a one-phase fractional Lamé- Clapeyron-Stefan problem with an over-specified boundary condition at the fixed face ![]() is a new and original problem, which is defined as: Finding the free boundary
is a new and original problem, which is defined as: Finding the free boundary![]() , and the temperature
, and the temperature![]() , and one thermal coefficient such that the following equation and conditions are satisfied:
, and one thermal coefficient such that the following equation and conditions are satisfied:
![]() , (8)
, (8)
![]() , (9)
, (9)
![]() , (10)
, (10)
![]() , (11)
, (11)
![]() , (12)
, (12)
![]() , (13)
, (13)
![]() , (14)
, (14)
where ![]() is the density of mass, k is the thermal conductivity, c is the specific heat by unit of mass,
is the density of mass, k is the thermal conductivity, c is the specific heat by unit of mass, ![]() is the
is the
latent heat of fusion by unit of mass, ![]() is the diffusion coefficient,
is the diffusion coefficient, ![]() is the temperature at
is the temperature at
the fixed face ![]() and
and ![]() is the coefficient which characterizes the heat flux at the fixed face
is the coefficient which characterizes the heat flux at the fixed face![]() . We assume that data
. We assume that data ![]() and
and ![]() are determined experimentally.
are determined experimentally.
The unknown thermal coefficient can be chosen among the four following ones: ![]() and
and![]() .
.
The goal of the present work is to obtain in Section II:
1) The solution of the one-phase time fractional Lamé-Clapeyron-Stefan of order ![]() (8)-(13) with an over-specified boundary condition of the heat flux type (14) by giving the explicit expression of the temperature
(8)-(13) with an over-specified boundary condition of the heat flux type (14) by giving the explicit expression of the temperature![]() , the free boundary
, the free boundary ![]() and the unknown thermal coefficient for the four different cases (see Table 1);
and the unknown thermal coefficient for the four different cases (see Table 1);
2) The restrictions on the data of the corresponding problem for the four different cases in order to have a unique explicit solution (see Table 1).
We remark that the results and explicit formulae obtained in [12] for the determination of one unknown thermal coefficient through the classical one-phase Lamé-Clapeyron-Stefan problem are generalized for the fractional case![]() , and they can be recovered when
, and they can be recovered when ![]() (see Table 2).
(see Table 2).
2. Determination of One Unknown Thermal Coefficient
First, we obtain a preliminary property in order to have a solution to problem (8)-(14).
Lemma 1. The solution of the problem (8)-(14) with ![]() and one unknown thermal coefficient is given by:
and one unknown thermal coefficient is given by:
![]() , (15)
, (15)
![]() , (16)
, (16)
where the dimensionaless coefficient ![]() and the unknown thermal coefficient must satisfy the following system of equations:
and the unknown thermal coefficient must satisfy the following system of equations:
![]() , (17)
, (17)
![]() . (18)
. (18)
Proof. Following [23] and [24] , by using properties (5) and (7) we have that the expressions (16) and (15) for the temperature and the free boundary satisfy Equation (8) and conditions (9)-(11) and (13). Exploiting conditions (12) and (14), we obtain that the dimensionaless coefficient ![]() and the unknown thermal coefficient must satisfy conditions (17) and (18).
and the unknown thermal coefficient must satisfy conditions (17) and (18).
Now, we will study the four following cases:
Case 1: Determination of![]() ;
;
Case 2: Determination of![]() ;
;
Case 3: Determination of![]() ;
;
Case 4: Determination of![]() ,
,
whose results are summarized in Table 1.
Remark 1.
In a analogous manner, we can compute the explicit formulae for the four thermal coefficients of the solid phase of the semi-infinite material by using a solidification process instead of a fusion process.
Theorem 2 (Case 1: Determination of the thermal coefficient c).
If data verify the condition:
![]() , (19)
, (19)
then the solution of the Case 1 (problem (8)-(14) with ![]() and the unknown thermal coefficient c) is given by:
and the unknown thermal coefficient c) is given by:
![]() , (20)
, (20)
where the coefficient ![]() is the unique solution of the equation:
is the unique solution of the equation:
![]() . (21)
. (21)
Moreover, the temperature ![]() and the free boundary
and the free boundary ![]() are given by the following expressions
are given by the following expressions![]() :
:
![]() , (22)
, (22)
![]() , (23)
, (23)
the dimensionless coefficient ![]() is the unique solution of the Equation (21) and the diffusion coefficient
is the unique solution of the Equation (21) and the diffusion coefficient ![]() is given by the following expression:
is given by the following expression:
![]() . (24)
. (24)
Proof. From condition (18) we obtain expression (20), taking into account the definition of the diffusion coefficient and the expression (20) from condition (17), we obtain the Equation (21) for the dimensionless coefficient![]() . The Equation (21) has a unique positive solution if and only if data verify condition (19). In order to prove this fact we can see that the following real functions:
. The Equation (21) has a unique positive solution if and only if data verify condition (19). In order to prove this fact we can see that the following real functions:
![]() , (25)
, (25)
have the following properties [23] :
![]() , (26)
, (26)
![]() , (27)
, (27)
![]() , (28)
, (28)
and the real function
![]() , (29)
, (29)
is a positive strictly decreasing function because
![]() , (30)
, (30)
and
![]() , (31)
, (31)
owning to the fact
![]() (32)
(32)
Then, we get the expression (24) for the diffusion coefficient.
Theorem 3. If the parameter ![]() then, under the hypothesis (19), the solution of the Case 1, given by (22), (23), (20) and (21) coincides with the one given in [12] :
then, under the hypothesis (19), the solution of the Case 1, given by (22), (23), (20) and (21) coincides with the one given in [12] :
![]() , (33)
, (33)
![]() , (34)
, (34)
![]() , (35)
, (35)
where the dimensionless coefficient ![]() is the unique solution of the equation:
is the unique solution of the equation:
![]() . (36)
. (36)
In particular, the inequality (19) is transformed in the following one:
![]() . (37)
. (37)
Proof. It follows from (6) and properties of functions ![]() and
and![]() .
.
Theorem 4 (Case 2: Determination of the thermal coefficient l).
If data verify the condition:
![]() , (38)
, (38)
then the solution of the Case 2 (problem (8)-(14) with ![]() and the unknown thermal coefficient
and the unknown thermal coefficient![]() ) is given by:
) is given by:
![]() , (39)
, (39)
where the coefficient ![]() is the unique solution of the equation:
is the unique solution of the equation:
![]() , (40)
, (40)
and the real function ![]() is defined by:
is defined by:
![]() . (41)
. (41)
Moreover, the temperature ![]() and the free boundary
and the free boundary ![]() are given by the following expressions
are given by the following expressions![]() :
:
![]() , (42)
, (42)
![]() , (43)
, (43)
the dimensionless coefficient ![]() is the unique solution of the Equation (40) and the diffusion coefficient
is the unique solution of the Equation (40) and the diffusion coefficient ![]() is given by the following expression:
is given by the following expression:
![]() . (44)
. (44)
Proof. From (17), we obtain the Equation (40) for the coefficient![]() , which has a unique solution if and only if data verify the condition (38) because function
, which has a unique solution if and only if data verify the condition (38) because function ![]() (see (28)) is a positive increasing function that satisfies the properties given in (28). From (18), we obtain the expression (39), and then we have that (43) holds.
(see (28)) is a positive increasing function that satisfies the properties given in (28). From (18), we obtain the expression (39), and then we have that (43) holds.
Theorem 5. If the parameter ![]() then, under the hypothesis (38), the solution of the Case 2 given by (42), (43), (39) and (40) coincides with the one given in [12] :
then, under the hypothesis (38), the solution of the Case 2 given by (42), (43), (39) and (40) coincides with the one given in [12] :
![]() , (45)
, (45)
![]() , (46)
, (46)
![]() , (47)
, (47)
where the dimensionless coefficient ![]() is the unique solution of the equation:
is the unique solution of the equation:
![]() . (48)
. (48)
In particular, the inequality (38) is transformed in the following one:
![]() . (49)
. (49)
Proof. It follows from (6) and properties of functions ![]() and
and![]() .
.
Theorem 6 (Case 3: Determination of the thermal coefficient k).
For any data, the solution of the Case 3 (problem (8)-(14) with ![]() and the unknown thermal coefficient k) is given by:
and the unknown thermal coefficient k) is given by:
![]() , (50)
, (50)
where the coefficient ![]() is the unique solution of the equation:
is the unique solution of the equation:
![]() . (51)
. (51)
Moreover, the temperature ![]() and the free boundary
and the free boundary ![]() are given by the following expressions
are given by the following expressions![]() :
:
![]() , (52)
, (52)
![]() , (53)
, (53)
the dimensionless coefficient ![]() is the unique solution of the Equation (50) and the diffusion coefficient
is the unique solution of the Equation (50) and the diffusion coefficient ![]() is given by the following expression:
is given by the following expression:
![]() . (54)
. (54)
Proof. From (18) we have that the coefficient ![]() satisfies the Equation (51), which has a unique solution for any data since the real function
satisfies the Equation (51), which has a unique solution for any data since the real function ![]() is a positive increasing function since the following properties hold [23] [24] :
is a positive increasing function since the following properties hold [23] [24] :
![]() , (55)
, (55)
Therefore, from (17) we obtain the expressions (50) and (54) for the conductivity ![]() and the diffusion coefficient
and the diffusion coefficient![]() , respectively.
, respectively.
Theorem 7. For any data, if the parameter ![]() then the solution of the Case 3 given by (52), (53), (50) and (51) coincides with the one given in [12] :
then the solution of the Case 3 given by (52), (53), (50) and (51) coincides with the one given in [12] :
![]() , (56)
, (56)
![]() , (57)
, (57)
![]() , (58)
, (58)
where the dimensionless coefficient ![]() is the unique solution of the equation:
is the unique solution of the equation:
![]() . (59)
. (59)
Proof. It follows from (6) and properties of functions ![]() and
and![]() .
.
Theorem 8 (Case 4: Determination of the thermal coefficient r).
For any data, the solution of the Case 4 (problem (8)-(14) with ![]() and the unknown thermal coefficient
and the unknown thermal coefficient![]() ) is given by:
) is given by:
![]() , (60)
, (60)
where the coefficient ![]() is the unique solution of the Equation (51). Moreover, the temperature
is the unique solution of the Equation (51). Moreover, the temperature ![]() and the free boundary
and the free boundary ![]() are given by the following expressions
are given by the following expressions![]() :
:
![]() , (61)
, (61)
![]() , (62)
, (62)
the dimensionless coefficient ![]() is the unique solution of the Equation (51) and the diffusion coefficient
is the unique solution of the Equation (51) and the diffusion coefficient ![]() is given by the following expression:
is given by the following expression:
![]() (63)
(63)
Proof. It is similar to the proof of the Case 3 (see Theorem 6).
Theorem 9. For any data, if the parameter ![]() then the solution of the Case 4 given by (61), (62), (60) and (51) coincides with the one given in [12] :
then the solution of the Case 4 given by (61), (62), (60) and (51) coincides with the one given in [12] :
![]() , (64)
, (64)
![]() , (65)
, (65)
![]() , (66)
, (66)
where the dimensionless coefficient ![]() is the unique solution of the equation:
is the unique solution of the equation:
![]() . (67)
. (67)
Proof. It is similar to the proof of the Case 3 (see Theorem 7).
Now, in order to summarize our results on the determination of one unknown thermal coefficient through a fractional Lamé-Clapeyron-Stefan problem with an over-specified heat flux boundary condition on the fixed face, we show the formula and restrictions for data for the four cases for the fractional Lamé-Clapeyron-Stefan problem with ![]() (see Table 1) and for the classical Lamé-Clapeyron-Stefan problem with
(see Table 1) and for the classical Lamé-Clapeyron-Stefan problem with ![]() (see Table 2).
(see Table 2).
![]()
Table 1. Summary of the results corresponding to the determination of one unknown thermal coefficient through a fractional Lamé-Clapeyron-Stefan problem with an over-specified heat flux boundary condition on the fixed face (4 cases).
![]()
Table 2. Summary of the results corresponding to the determination of one unknown thermal coefficient through a classical Lamé-Clapeyron-Stefan problem ![]() with an over-specified heat flux boundary condition on the fixed face (4 cases). These results were obtained by taking
with an over-specified heat flux boundary condition on the fixed face (4 cases). These results were obtained by taking ![]() in the results given in Table 1 [12] .
in the results given in Table 1 [12] .
Acknowledgements
The present work has been sponsored by the Projects PIP N˚ 0534 from CONICET―Univ. Austral, and by AFOSR-SOARD Grant FA9550-14-1-0122.