1. Introduction
The problem of eigenvalue assignment is well established in control theory where numerous methods have been proposed―each with certain advantages and disadvantages. However, a need still arises for methods which are simple in concept and can be easily implemented. A fulfillment to such need is contributed by this paper.
As compared with some previous methods for eigenvalue assignment, this method doesn’t require specific transformations, knowledge of the open loop eigenvalues or the determination of the closed loop eigenvectors. The method utilizes submatrices stemming from a particular state transformation. The transformation is only needed in the development of the method and not the actual assignment of the eigenvalues.
The proposed method tackles eigenvalue assignment by manipulating lower order matrices, hence enjoying some numerical advantages. Furthermore, 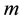 eigenvalues are assigned independently of the remaining
eigenvalues are assigned independently of the remaining  eigenvalues. The method is simplified when
eigenvalues. The method is simplified when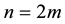 , where
, where 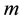 is the rank of
is the rank of , resulting in a systematic feedback law requiring only the specification of two
, resulting in a systematic feedback law requiring only the specification of two 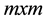 matrices. It can be further simplified in cases where the columns of
matrices. It can be further simplified in cases where the columns of  and
and 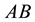 constitute an invertible matrix.
constitute an invertible matrix.
The method is also shown to apply to uncontrollable systems where certain features of some submatrices are pointed out, thus providing additional degrees of freedom in the control law. Furthermore, in the case of maximum number of uncontrollable eigenvalues, the controller is shown to exhibit its simplest form and offer arbitrariness which may be utilized in fulfilling a myriad of design objectives.
Finally, the systematic and straightforward nature of the method is demonstrated by two examples.
2. The Nonrecursive Feedback Law
The assignment law considered is a state feedback law of the form 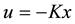 applied to the system
applied to the system
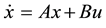 (2.1)
(2.1)
where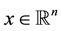 ,
, 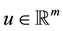 , the rank of
, the rank of 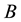 is
is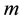 ,
, ![]() and
and ![]() refer to the range and null spaces of
refer to the range and null spaces of![]() .
.
For the development of the simplified methods, a state transformation T is used where![]() , leading to system and input matrices of the form conformal with those in [1] .
, leading to system and input matrices of the form conformal with those in [1] .
![]() (2.2)
(2.2)
where
![]() (2.3)
(2.3)
Such requirement on ![]() necessitates
necessitates ![]() where
where ![]() is an
is an ![]() matrix chosen to ensure the nonsingularity of
matrix chosen to ensure the nonsingularity of![]() . The inverse of
. The inverse of ![]() is represented by
is represented by
![]() (2.4)
(2.4)
where![]() , and
, and ![]() can be looked upon as a name for that partition of
can be looked upon as a name for that partition of ![]() related to
related to ![]() and
and ![]() or as unique generalized inverses of matrices [2] [3] . The generalized inverses are unique in our case since they satisfy the additional conditions
or as unique generalized inverses of matrices [2] [3] . The generalized inverses are unique in our case since they satisfy the additional conditions
![]() (2.5)
(2.5)
Using the terminology above, the submatrices become
![]() (2.6)
(2.6)
In addition
![]() (2.7)
(2.7)
With reference to the recursive method of Hassan et al. [1] , ![]() eigenvalues are assigned through an
eigenvalues are assigned through an ![]() matrix
matrix ![]() while the remaining
while the remaining ![]() eigenvalues are assigned through the reduced order matrix pair
eigenvalues are assigned through the reduced order matrix pair ![]() i.e. the
i.e. the ![]() eigenvalues to be assigned are eigenvalues of
eigenvalues to be assigned are eigenvalues of![]() . The reduced order matrix
. The reduced order matrix ![]() is determined independently of
is determined independently of ![]()
The recursive method [1] is now manipulated to result in a non-recursive feedback law.
According to [1] ; having undergone all recursive steps the final feedback matrix is given by
![]() (2.8)
(2.8)
where
![]() (2.9)
(2.9)
i.e.
![]()
substituting ![]() as given in (2.3) and
as given in (2.3) and ![]() as given in (2.4) yields
as given in (2.4) yields
![]()
![]()
Substituting the values of ![]() as in (2.6), gives
as in (2.6), gives
![]()
![]()
Using the fact that ![]() as in (2.7), the equation can be finally put in the form
as in (2.7), the equation can be finally put in the form
![]() (2.10)
(2.10)
The advantage of this feedback law as given in (2.10) is that assignment of n eigenvalues is split into independent assignment of ![]() eigenvalues through
eigenvalues through ![]() and assignment of
and assignment of ![]() eigenvalues of
eigenvalues of ![]() through a suitable
through a suitable![]() . Existing non-recursive methods not requiring state transformation like [4] and [5] or any other eigenvalue assignment method can be used to determine
. Existing non-recursive methods not requiring state transformation like [4] and [5] or any other eigenvalue assignment method can be used to determine![]() . In addition, since
. In addition, since ![]() has dimension
has dimension![]() , the matrix
, the matrix ![]() has a reduced dimension
has a reduced dimension![]() . Further utilization of (2.10) is to be followed in Section 6 when it comes to assignment of uncontrollable eigenvalues, where it is shown that a further reduction in the order of
. Further utilization of (2.10) is to be followed in Section 6 when it comes to assignment of uncontrollable eigenvalues, where it is shown that a further reduction in the order of ![]() is possible to the extent that
is possible to the extent that ![]() can be taken as zero in certain cases.
can be taken as zero in certain cases.
3. A Simplified Method When ![]() and the System Is Controllable
and the System Is Controllable
Although the previous development resulted in a controller which manipulates lower order matrices; the selection of ![]() remains an eigenvalue problem to be solved. Known methods of eigenvalue assignment can be used with the benefit of dealing with reduced order matrices, see [6] . However, further simplification can be made in the case where
remains an eigenvalue problem to be solved. Known methods of eigenvalue assignment can be used with the benefit of dealing with reduced order matrices, see [6] . However, further simplification can be made in the case where![]() and
and ![]() is invertible as developed below.
is invertible as developed below.
Due to the presence of identical terms within the parenthesis’s, we simplify one term in the state feedback matrix
![]() (2.10)
(2.10)
where ![]() assigns
assigns ![]() eigenvalues and
eigenvalues and ![]() assigns the remaining
assigns the remaining ![]() eigenvalues through
eigenvalues through
![]() (3.1)
(3.1)
Assuming the nonsingularity of ![]() and that the remaining
and that the remaining ![]() eigenvalues are eigenvalues of the matrix
eigenvalues are eigenvalues of the matrix![]() , then
, then
![]() (3.2)
(3.2)
Substituting the value of ![]() in any term within a parenthesis of (2.10) gives
in any term within a parenthesis of (2.10) gives
![]()
Using (2.7), and recalling ![]()
![]() (3.3)
(3.3)
substituting this value for the two terms in the parenthesis’s in Equation (2.10) gives
![]() (3.4)
(3.4)
Some remarks regarding the control law are stated below.
A necessary condition for the invertibility of ![]() is the controllability of the system.
is the controllability of the system.
To see this, suppose ![]() is nonsingular and the system is uncontrollable, then according to (3.4) it is possible to change all
is nonsingular and the system is uncontrollable, then according to (3.4) it is possible to change all ![]() eigenvalues of
eigenvalues of![]() , contradicting the established fact that uncontrollable eigenvalues cannot be changed by state feedback. Hence, only if the system is controllable will
, contradicting the established fact that uncontrollable eigenvalues cannot be changed by state feedback. Hence, only if the system is controllable will ![]() be nonsingular.
be nonsingular.
No need to do the state transformation. The determination of (2.4) is only needed to extract ![]() and to subsequently evaluate the inverse of
and to subsequently evaluate the inverse of ![]() (equals
(equals![]() ).
).
Assignment of ![]() eigenvalues is achieved through
eigenvalues is achieved through ![]() lower order
lower order ![]() and
and ![]() matrices, which can be diagonal, Jordan forms, or skew?symmetric when it comes assignment of complex eigenvalues.
matrices, which can be diagonal, Jordan forms, or skew?symmetric when it comes assignment of complex eigenvalues.
As compared with other assignments laws the highest power of ![]() involved is two while it’s
involved is two while it’s ![]() for certain celebrated methods like Ackermann’s method [7] . This gives numerical advantages in terms of reducing matrix multiplication rounding errors, as demonstrated by Petkov [8] , who showed that matrix multiplications is ill conditioned.
for certain celebrated methods like Ackermann’s method [7] . This gives numerical advantages in terms of reducing matrix multiplication rounding errors, as demonstrated by Petkov [8] , who showed that matrix multiplications is ill conditioned.
4. Further Simplification
Additional simplification can be done to the form of (3.4). By replacing ![]() by
by ![]() where
where![]() ,
, ![]() , and
, and ![]() have the same set of eigenvalues,
have the same set of eigenvalues,
![]()
![]()
Ending up with a compact form for K as
![]() (4.1)
(4.1)
If ![]() is chosen as a matrix representation of
is chosen as a matrix representation of![]() , then
, then ![]() can be obtained independently of
can be obtained independently of![]() , see Lancaster [9] and Schott [10] . Also, theorem 6.4.5 pp 115 of Graybill’s book [2] states, if
, see Lancaster [9] and Schott [10] . Also, theorem 6.4.5 pp 115 of Graybill’s book [2] states, if ![]() and
and![]() , then
, then ![]() can be determined independently as
can be determined independently as ![]() and
and ![]() respectively. The left inverse of
respectively. The left inverse of ![]() now involves an inverse of an
now involves an inverse of an ![]() symmetric
symmetric ![]() matrix instead of the inverse of the generally non-symmetric
matrix instead of the inverse of the generally non-symmetric ![]()
![]() matrix needed to extract
matrix needed to extract![]() .
.
The choice of ![]() has many advantages.
has many advantages.
§ The selection of N is systematic.
§ Such choice gives the advantage of inverting an ![]() matrix through inversion of symmetric
matrix through inversion of symmetric ![]() matrices; thus providing numerical advantages.
matrices; thus providing numerical advantages.
§ Further computational advantages are gained if the Gram-Schmidt ortho-normalization procedure is used (can be easily programmed on a digital computer and is already within the MATLAB function library). In this case, if ![]() is orthonormal, then
is orthonormal, then![]() .
.
A further simplification to (4.1) is possible in the case where ![]() is taken as
is taken as![]() . In which case,
. In which case, ![]() becomes the unity matrix offering a more simplified form given by.
becomes the unity matrix offering a more simplified form given by.
![]() (4.2)
(4.2)
So, the design process now reduces to the selection of ![]() which specify the desired eigenvalues and the calculation of
which specify the desired eigenvalues and the calculation of ![]() according to (2.5).
according to (2.5).
5. The Uncontrollable Case
The non-recursive feedback law can still be applied when the system is uncontrollable. In our case, and as has been shown by [11] , the pair ![]() and
and ![]() is the uncontrollable pair, i.e. the uncontrollable eigenvalues are eigenvalues of
is the uncontrollable pair, i.e. the uncontrollable eigenvalues are eigenvalues of![]() .
.
For the case![]() , the uncontrollability of the system implies the following:
, the uncontrollability of the system implies the following:
a) The matrix ![]() has to be a singular matrix, otherwise an
has to be a singular matrix, otherwise an ![]() exists which can reassign arbitrarily all eigenvalues of
exists which can reassign arbitrarily all eigenvalues of![]() . This, together with the
. This, together with the ![]() arbitrary eigenvalues assigned by
arbitrary eigenvalues assigned by ![]() makes the total number of arbitrarily assigned eigenvalues
makes the total number of arbitrarily assigned eigenvalues![]() , an impossibility for an uncontrollable system as proved in the control literature.
, an impossibility for an uncontrollable system as proved in the control literature.
b) Since ![]() has to be singular, then it has columns which are scalar multiple of each other, or linear combi-
has to be singular, then it has columns which are scalar multiple of each other, or linear combi-
nations of each other. To see this, due to uncontrollability, the matrix ![]() is an
is an ![]() square matrix which can never have the full rank
square matrix which can never have the full rank![]() . Since
. Since ![]() has necessarily rank
has necessarily rank![]() , this leaves
, this leaves ![]() with a rank less than
with a rank less than![]() , indicating a dependence of
, indicating a dependence of ![]() and since
and since ![]() then
then ![]() may annihi-
may annihi-
late![]() . In the case of annihilation,
. In the case of annihilation, ![]() will have at least a zero column, say the
will have at least a zero column, say the ![]() column. Such fact renders the
column. Such fact renders the ![]() row of
row of ![]() immaterial since the product
immaterial since the product ![]() will not depend on that row. This provides arbitrariness in the
will not depend on that row. This provides arbitrariness in the ![]() row of
row of ![]() which can be utilized further in the design of the controller. It can lead to manipulating lower order matrices within
which can be utilized further in the design of the controller. It can lead to manipulating lower order matrices within![]() , gaining calculation efficiency.
, gaining calculation efficiency.
In the light of the above facts since a nonsingular ![]() doesn’t exists, the formula given in (3.4) cannot be used. Instead, any eigenvalue assignment method available in the control literature (see [12] - [14] ) can be used to calculate
doesn’t exists, the formula given in (3.4) cannot be used. Instead, any eigenvalue assignment method available in the control literature (see [12] - [14] ) can be used to calculate ![]() with the advantage of dealing with matrices of reduced order.
with the advantage of dealing with matrices of reduced order.
6. Justification of ![]() for the Case of Maximum Number of Uncontrollable Eigenvalues
for the Case of Maximum Number of Uncontrollable Eigenvalues
If the system has the maximum number of ![]() uncontrollable eigenvalues, then
uncontrollable eigenvalues, then ![]() is identically the zero matrix. This has to be the case, otherwise, a nonzero
is identically the zero matrix. This has to be the case, otherwise, a nonzero ![]() is capable of changing some of these eigenvalues, an impossibility since the total number of uncontrollable is assumed to be
is capable of changing some of these eigenvalues, an impossibility since the total number of uncontrollable is assumed to be![]() .
.
However, although (3.4) cannot be used to get the final feedback matrix![]() , a most simple form of (2.10) is now considered. The simplicity hinges on letting
, a most simple form of (2.10) is now considered. The simplicity hinges on letting![]() . That is.
. That is.
![]() (6.1)
(6.1)
The justification for this form stems from the fact that in our case all uncontrollable eigenvalues are those of![]() , and can be specified by
, and can be specified by ![]() which can be
which can be ![]() itself, in which case, and according to (3.1),
itself, in which case, and according to (3.1), ![]() will be zero, in which case
will be zero, in which case ![]() can be taken as zero. Substituting
can be taken as zero. Substituting ![]() in (2.10) results in (6.1).
in (2.10) results in (6.1).
Seeing it differently, since in our case ![]() is identically zero, this makes the product of
is identically zero, this makes the product of ![]() zero. This renders the value of
zero. This renders the value of ![]() immaterial, so any
immaterial, so any ![]() can be taken including the case
can be taken including the case![]() .
.
Note that ![]() in (6.1) doesn’t depend on
in (6.1) doesn’t depend on![]() , so we can relax the uniqueness of
, so we can relax the uniqueness of![]() ; just requiring
; just requiring![]() . This is because there always exists a nonunique
. This is because there always exists a nonunique ![]() with a corresponding
with a corresponding ![]() such that
such that ![]() as required by conditions (2.4). A systematic choice for
as required by conditions (2.4). A systematic choice for ![]() is
is![]() .
.
Note that ![]() can still be totally arbitrary. Such choice can be used to satisfy certain design requirements like controller matrix norm, sensitivity studies, eigenvector specifications, etc. For such cases, one has to resort to (2.10).
can still be totally arbitrary. Such choice can be used to satisfy certain design requirements like controller matrix norm, sensitivity studies, eigenvector specifications, etc. For such cases, one has to resort to (2.10).
7. Examples
Example 1: Consider the controllable system given by
![]()
It is required to assign the eigenvalues −2, −3 and −5 ± j4.
To extract F3, MATLAB was used with ![]() taken as an orthonormal representation of
taken as an orthonormal representation of![]() , resulting in
, resulting in
![]()
Hence, to five significant digits
![]()
The matrices ![]() may be chosen as
may be chosen as
![]()
Using the control law given by (3.4) results in the following state feedback matrix
![]()
To check, the system closed loop matrix ![]() is
is
![]()
Which has the eigenvalues −2, −3, −5 + j4 and −5 − j4.
Example 2: Consider the following system [15] where
![]()
This system is uncontrollable with −1 and −4 being the uncontrollable eigenvalues. It is desired to assign the two eigenvalues −4 and −5.
So let
![]()
To expose the controllable and uncontrollable eigenvalues, we may take ![]()
Yielding
![]()
Which shows that![]() , −2 and −3 are the controllable eigenvalues and that the uncontrollable eigenvalues are those of
, −2 and −3 are the controllable eigenvalues and that the uncontrollable eigenvalues are those of![]() ; i.e. −1 and −4. In fact, we need not bother finding them as they aren’t needed in the calculation of K.
; i.e. −1 and −4. In fact, we need not bother finding them as they aren’t needed in the calculation of K.
Besides, the inverse of T isn’t needed to extract![]() . Instead,
. Instead, ![]() can be taken as
can be taken as ![]() giving
giving
![]()
Using K as in (6.1) yields a state feedback K matrix, say ![]()
![]()
Another![]() , just satisfying
, just satisfying ![]() with no regard to any N may be
with no regard to any N may be
![]()
Which results in a different state feedback K matrix, say ![]()
![]()
Both ![]() and
and ![]() result in the assignment of two eigenvalues −4 and −5 and the uncontrollable eigenvalues −1 and −4.
result in the assignment of two eigenvalues −4 and −5 and the uncontrollable eigenvalues −1 and −4.
8. Conclusion
The paper has considered a method for eigenvalue assignment based on a scheme of recursive nature. The method involves algebraic manipulation of lower order matrices with an advantage of not requiring state transformation or eigenvectors determination. The method is further simplified in the case where![]() . The method is extended to deal with uncontrollable systems where it is shown that
. The method is extended to deal with uncontrollable systems where it is shown that ![]() exhibits a certain degree of arbitrariness, to the extent of resulting in the simplest form for the state feedback law. The examples considered demonstrate the ease of use of the method.
exhibits a certain degree of arbitrariness, to the extent of resulting in the simplest form for the state feedback law. The examples considered demonstrate the ease of use of the method.