Theories and Analyses Thick Hyperbolic Paraboloidal Composite Shells ()
1. Introduction
The main objective of shell theory is to predict the stress and the displacement arising in an elastic shell in response to given forces. Such a prediction is made either by solving a system of partial differential equations or by minimizing a functional, which may be defined either over a three-dimensional set or over a two-dimensional set, depending on whether the shell is viewed in its reference configuration as a three-dimensional or as a two- dimensional body. The three-dimensional theory of shells is obtained simply by replacing the reference configuration of a general body with that of a shell [2] -[4] .
Tow formulations are used to show equations of motion with required boundary conditions for doubly curved deep thick composite [5] -[7] . The first is based upon the formulation that is presented initially by Reddy [8] . The second formulation is based upon that of Qatu [1] [9] . Qatu considers the radius of twist in his formulation.
The vibration of thick shells has been solved using the first order shear deformation shell theory [5] -[10] . Three dimensional theory of elasticity is used for solving theories of shell structures. Thus three dimensional analyses of shells are considered to be the most accurate.
This paper presents Stress resultants becuase hyperbolic paraboloidal shells are determined by deriving the dynamic stiffness matrix from the equilibrium equations of motion using Hamilton’s minimum energy principle for a simply supported cross-ply structure by Zannon (TSDTZ) [2] -[4] . The results are calculated for orthotropic. The extensional, bending and coupling stiffness parameters are calculated using a commercial software package (ANSYS). In this formulation, the stiffness parameters are calculated using exact integration (and/or terms truncated to a specific order) of stress resultant equations. In addition, Zannon [2] -[4] considers the radius of twist in formulation. The third order polynomials for in-plane displacements in the z-direction are utilized allowing for the inclusion of shear deformation and rotary inertia effects (Third order shear deformation theory or (TSDTZ) [2] -[4] .
Exact static and free vibration solutions for isotropic and symmetric and anti-symmetric cross-ply hyperbolic shells for different length-to-thickness and length-to-radius ratios are obtained using the above theories. Results of both theories are compared with those obtained using a three-dimensional (3D) analysis to test the accuracy of the shell theories presented here. Early treatment of composite thick shells (e.g. [5] [11] ) includes both shear deformation and rotary inertia rotary but fails to include accurate representation of curvature (the z/R terms in the stress resultants).
2. TSDTZ Shell Theory
The approximation of displacement components using the third-order shear deformation shell theory can be written as Zannon (TSDTZ) [2] .

where 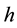 is the shell thickness and
is the shell thickness and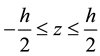 ,
, 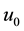 ,
,  ,
, 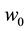 are mid-surface displacements of the shell and
are mid-surface displacements of the shell and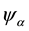 ,
, 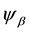 ,
, 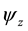 are mid-surface rotations and
are mid-surface rotations and ,
, 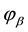 are higher order terms rotation of transverse normal.
are higher order terms rotation of transverse normal.
Equation (1) constitutes the only assumption needed to reduce 3D elasticity equations in curvilinear coordinates to the shell theory by Zannon [2] [3] . The strain-displacement Relationships in the principal coordinates of a doubly-curved shell are given in [2] [3] . The stress resultant or the stiffness matrices are given in [2] [3] . Substituting these stiffness parameters in the Hamilton equation [1] -[5] and simplifying the resulting equations, we get the equations of motion and boundary conditions for S2 are given in [2] [3] .
3. Equation of Motion
Let us consider the Hyperbolic laminated shell as shown in Figure 1 with length 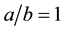 under load per unit area,
under load per unit area, 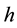 is the thickness of the shell. If the load is orthogonal to the surface, then Lame’ parameters (elastic and
is the thickness of the shell. If the load is orthogonal to the surface, then Lame’ parameters (elastic and
shear modulus) of middle surface 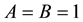 and
and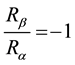 ,
, 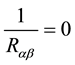 are substituted in moment and force re-
are substituted in moment and force re-
sultants [1] -[3] [9] to formulate the Hyperbolic shell equations for TSDTZ. The moment and force resultant equations are given in [2] [3] . The stress resultant terms are shown in Figure 1.
Therefore, the displacement mid surface for hyperbolic paraboloidal thick shells is rewritten as [2] [3] :
![]()
![]()
Figure 1. Surface to force and moment resultants of shell form composite structures [1] -[3] .
![]()
Thus, the Equations of motion (2) for hyperbolic paraboloidal thick shells reduces to [2] [3]
![]()
4. Numerical Results and Discussion
To validate the third order shear deformation theory, the values of extensional![]() , bending
, bending ![]() and coupling
and coupling ![]() stiffness parameters [2] [3] are given in Tables 1-3 using the MATLAB algorithm for lami-
stiffness parameters [2] [3] are given in Tables 1-3 using the MATLAB algorithm for lami-
nated composite Hyperbolic thick shells. Thenit is compared with the first order shear deformation theory from the literature. There are small discrepancies are seen in the Tables 1-3, which is due to the third order shear deformation and the tolerance limitations. Tables 1-3 show the extensional stress, coupling, and bending stiffness parameters for [0/90] laminated hyperbolic thick shells. While comparing the various stiffness parameters with the existing literature and the present theory [1] -[5] [8] -[11] , we see that the TSDTZ approximation is more accurate in in comparison with first order shear deformation theory.
5. Summary and Conclusion
TSDTZ offers a more accurate representation of the stiffness parameters and the stress resultant equations. Most analyses performed here show that there is an improvement obtained when TSDTZ is used. Also, TSDTZ offers
many other advantages in the accurate representations such as extensional, coupling, and stress stiffness para- meters, as shown in Tables 1-3 and is mainly due to the inclusion of the term ![]() in the mathematical formulation of third order shear deformation theory.
in the mathematical formulation of third order shear deformation theory.
Nomenclature
![]()
NOTES
*Corresponding author.