An Integral Collocation Approach Based on Legendre Polynomials for Solving Riccati, Logistic and Delay Differential Equations ()
1. Introduction
It is well known that the ordinary differential equations (ODEs) have been the focus of many studies due to their frequent appearance in various applications, such as in fluid mechanics, viscoelasticity, biology, physics and engineering applications, for more details, for example [1] -[5] . Consequently, considerable attention has been given to the efficient numerical solutions of ODEs of physical interest, because it is difficult to find exact solutions. Different numerical methods have been proposed in the literature for solving ODEs [6] -[13] .
The Riccati differential equation (RDE) is named after the Italian Nobleman Count Jacopo Francesco Riccati (1676-1754). The book of Reid [14] contains the fundamental theories of Riccati equation, with applications to random processes, optimal control, and diffusion problems. Besides important engineering science applications that today are considered classical, such as stochastic realization theory, optimal control, robust stabilization, and network synthesis, the newer applications include such areas as financial mathematics [15] . The solution of this equation can be reached using classical numerical methods such as, the forward Euler method and RungeKutta method. Bahnasawi et al. [16] presented the usage of Adomian decomposition method to solve the nonlinear RDE in an analytic form. Tan and Abbasbandy [17] employed the analytic technique called homotopy analysis method to solve the quadratic RDE.
The Logistic model can be obtained by applying the derivative operator on the Logistic equation. The model is initially published by Pierre Verhulst in 1838 [18] . The continuous Logistic model is described by first order ODE. The discrete Logistic model is simple iterative equation that reveals the chaotic property in certain regions [19] . There are many variations of the population modeling [20] . The Verhulst model is the classic example to illustrate the periodic doubling and chaotic behavior in dynamical system [19] . The model describs the population growth may be limited by certain factors like population density [20] .
2. Applications of Logistic Equation
A typical application of the Logistic equation is a common model of population growth. Let  represents the population size and
represents the population size and  represents the time where the constant
represents the time where the constant  defines the growth rate.
defines the growth rate.
Another application of Logistic curve is in medicine, where the Logistic differential equation is used to model the growth of tumors. This application can be considered an extension of the above mentioned use in the framework of ecology.
The solution of Logistic equation is explained the constant population growth rate which not includes the limitation on food supply or spread of diseases [21] . The solution curve of the model is increase exponentially from the multiplication factor up to saturation limit which is maximum carrying capacity [21] ,
 where
where  is the population with respect to time,
is the population with respect to time,  is the rate of maximum population growth and
is the rate of maximum population growth and  is the carrying capacity. The solution of continuous Logistic equation is in the form of constant growth rate as in formula
is the carrying capacity. The solution of continuous Logistic equation is in the form of constant growth rate as in formula  where
where  is the initial population [22] .
is the initial population [22] .
A delay differential equation (DDE) is a differential equation in which the derivative of the function at any time depends on the solution at previous time. Introduction of delay in the model enriches its dynamics and allows a precise description of the real life phenomena. DDEs are proved useful in control systems [23] , lasers, traffic models [24] , metal cutting, epidemiology, neuroscience, population dynamics [25] , chemical kinetics [26] , etc. In DDE, one has to provide history of the system over the delay interval  as the initial condition. Due to this reason delay systems are infinite dimensional in nature. Because of in infinite dimensionality the DDEs are difficult to analyze analytically and hence the numerical solutions play an important role.
as the initial condition. Due to this reason delay systems are infinite dimensional in nature. Because of in infinite dimensionality the DDEs are difficult to analyze analytically and hence the numerical solutions play an important role.
In [27] , Mai-Duy, et al. derived an integral collocation approach based on Chebyshev polynomials and used it for solving numerically the bi-harmonic equations. In [28] , Bhrawy and Alofi introduced a new shifted Chebyshev operational matrix of fractional integration of arbitrary order and applied together with spectral tau method for solving linear fractional differential equations. Khader et al. [29] introduced a new approximate formula of the fractional derivative using Legendre series expansion and used it to solve numerically the fractional diffusion equation.
In this article, we extend the previous work and derive some schemes of the integral collocation formulation based on Legendre polynomials. We implement these formulae to solve numerically Riccati, Logistic and delay differential equations.
Our paper is organized as follows: In Section 2, we derive some integration collocation formulations using Legendre series expansion. In Section 3, we give the integral collocation approach for solving Riccati, Logistic and delay differential equations. In Section 4, the paper ends with a brief conclusion and some remarks.
3. Integration Collocation Formulations
The well known Legendre polynomials are defined on the interval 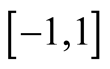 and can be determined with the aid of the following recurrence formula [30]
and can be determined with the aid of the following recurrence formula [30]

where  and
and . In order to use these polynomials on the interval
. In order to use these polynomials on the interval , we define the so called shifted Legendre polynomials by introducing the change of variable
, we define the so called shifted Legendre polynomials by introducing the change of variable . Let the shifted Legendre polynomials
. Let the shifted Legendre polynomials  be denoted by
be denoted by . Then
. Then  can be obtained as follows
can be obtained as follows

where  and
and . The analytic form of the shifted Legendre polynomials
. The analytic form of the shifted Legendre polynomials  of degree
of degree  is given by
is given by
 (1)
(1)
Note that  and
and . The orthogonality condition is
. The orthogonality condition is

In this work, to consider the differential equation of  -th order, we build the integration collocation method using the truncated Legendre series of degree
-th order, we build the integration collocation method using the truncated Legendre series of degree  to represent the
to represent the  -th derivative of the unknown function
-th derivative of the unknown function  in the following manner
in the following manner
 (2)
(2)
Using the integration we can obtain the lower-order derivatives and the function itself as follows
 (3)
(3)
 (4)
(4)
 (5)
(5)
 (6)
(6)
from (1) and (2) we have
 (7)
(7)
We now collocate Equatuions (2)-(6) at  points
points ,
,  as
as
 (8)
(8)
where , and
, and  are integrated matrices.
are integrated matrices.
4. Integral Collocation Approach for Solving Riccati, Logistic and Delay Differential Equations
In this section, we introduce the integral collocation approach using Legendre expansion for solving the Riccati, Logistic and delay differential equations.
4.1. Model 1: Riccati Differential Equation
 (9)
(9)
we also assume an initial condition
 (10)
(10)
The exact solution to this problem at  is
is

The procedure of the implementation is given by the following steps:
1) Approximate the function  using Formula (6) and its relevant derivatives with
using Formula (6) and its relevant derivatives with , as follows
, as follows
 (11)
(11)
where  is defined in (7) as
is defined in (7) as
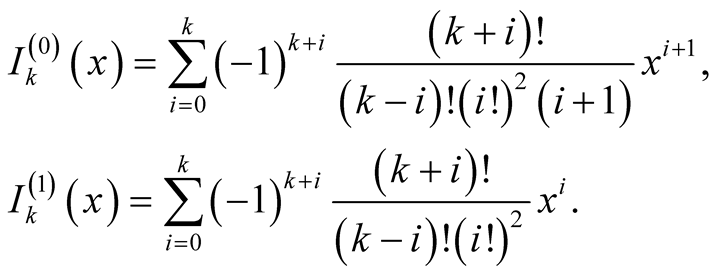
Then the Riccati differential Equation (9) is transformed to the following approximated form
 (12)
(12)
We now collocate Equation (12) at  points
points ,
,  as
as
 (13)
(13)
For suitable collocation points we use the roots of shifted Legendre polynomial .
.
2) Also, by substituting from the initial condition (10) in (11) we can obtain  an equation which gives the value of the constant
an equation which gives the value of the constant  as follows
as follows
 (14)
(14)
Equations (13) and (14) represent a system of non-linear algebraic equations which contains seven equations for the unknowns  and
and .
.
3) Solve the resulting system using the Newton iteration method to obtain the unknowns  as follows
as follows
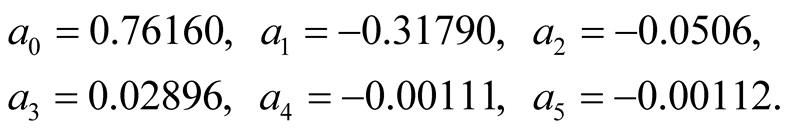
Therefore, from Formula (12) we can obtain the approximate solution in the form

The numerical results of the proposed problem (9) are given in Figure 1 with different values of 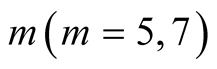 in the interval
in the interval  at
at . From this figure, since the obtained numerical solutions are in excellent agreement with the exact solution, so, we can conclude that the proposed technique is well for solving such class of ODEs.
. From this figure, since the obtained numerical solutions are in excellent agreement with the exact solution, so, we can conclude that the proposed technique is well for solving such class of ODEs.
4.2. Model 2: Logistic Differential Equation
 (15)
(15)
We also assume an initial condition
 (16)
(16)
The exact solution to this problem is given by


Figure 1. A comparison between the exact solution and the approximate solution at  and
and .
.
The existence and the uniqueness of the proposed problem (15) are introduced in details in [31] .
The procedure of the implementation is given by the following steps:
1) Approximate the function  and its relevant derivatives with
and its relevant derivatives with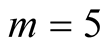 , using Formula (11). Then the Logistic differential Equation (15) is transformed to the following approximated form
, using Formula (11). Then the Logistic differential Equation (15) is transformed to the following approximated form
 (17)
(17)
We now collocate Equation (17) at  points
points ,
,  as
as
 (18)
(18)
For suitable collocation points we use roots of shifted Legendre polynomial  which are
which are
 (19)
(19)
2) Also, by substituting from the initial condition (16) in (11) with  we can obtain
we can obtain  an equation which gives the value of the constant
an equation which gives the value of the constant .
.
Equation (18) represents a system of non-linear algebraic equations which contains six equations for the unknowns .
.
3) Solve the resulting system using the Newton iteration method to obtain the unknowns  as follows
as follows
 (20)
(20)
Therefore, from Formula (11) we can obtain the approximate solution in the form

The numerical results of the proposed problem (15) are given in Figure 2 with different values of  in the interval
in the interval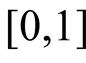 . From this figure, since the obtained numerical solutions are in excellent agreement with the exact solution, so, we can conclude that the proposed technique is well for solving such class of ODEs.
. From this figure, since the obtained numerical solutions are in excellent agreement with the exact solution, so, we can conclude that the proposed technique is well for solving such class of ODEs.
4.3. Model 3: Delay Differential Equation
Consider the linear delay differential equation of third-order
 (21)
(21)
with the initial conditions
 (22)
(22)
The exact solution of this model is .
.
The procedure of the implementation is given by the following steps:
1) Approximate the function  using Formula (6) and its relevant derivatives with
using Formula (6) and its relevant derivatives with , as follows
, as follows

Figure 2. A comparison between the exact solution and the approximate solution at m = 3 and m = 5.
 (23)
(23)
where ,
,  and
and  are defined in (7) as follows
are defined in (7) as follows

Then the delay differential Equation (21) is transformed to the following approximated form
 (24)
(24)
We now collocate Equation (24) at  points
points ,
,  as
as
 (25)
(25)
For suitable collocation points we use the roots of shifted Legendre polynomial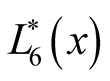 .
.
2) Also, by substituting from the initial conditions (22) in (23) we can obtain  equations which give the values of the constants
equations which give the values of the constants
 (26)
(26)
Equations (25) and (26) represent a system of linear algebraic equations which contains nine equations for the unknowns  and
and .
.
3) Solve the resulting system using the conjugate gradient method to obtain the unknowns 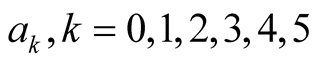 as follows
as follows
 (27)
(27)
Therefore, from Formula (23) we can obtain the approximate solution in the form

The numerical results of the proposed problem (21) are given in Figure 3 with different values of  in the interval
in the interval . From this figure, since the obtained numerical solutions are in excellent agreement with the exact solution, so, we can conclude that the proposed technique is well for solving such class of ODEs.
. From this figure, since the obtained numerical solutions are in excellent agreement with the exact solution, so, we can conclude that the proposed technique is well for solving such class of ODEs.
5. Conclusion and Remarks
In this article, an integral collocation approach based on Legendre polynomials is introduced for solving

Figure 3. A comparison between the exact solution and the approximate solution at m = 5 and m = 7.
numerically the Riccati, Logistic and delay differential equations. The properties of the Legendre polynomials are used to reduce the proposed problems to system of algebraic equations which are solved by a suitable numerical method. From the obtained numerical results, we can conclude that this method gives results with an excellent agreement with the exact solution. All numerical results are obtained using Matlab program 8.