Nikodým-Type Theorems for Lattice Group-Valued Measures with Respect to Filter Convergence ()
1. Introduction
In the literature there have been several recent studies about limit theorems with respect to filter/ideal convergence for measures, taking values in abstract spaces, whose a particular case is the statistical convergence, related with asymptotic density of subsets of the set of natural numbers. Though in general it is not possible to give versions of limit theorems completely analogous to the corresponding classical ones in the filter/ideal setting (see also [1] , Example 3.4), there are different kinds of results on these topics, whose an overview can be found in [2] and its bibliography, together with a historical survey on several types of kinds of such theorems and related topics since the beginning of the last century. Some classical like limit theorems for measures and integrals in the context of lattice groups or similar structures can be found, for instance, in [3] -[7] . In particular, in [1] [8] , some versions of basic matrix theorems are given, extending results of [9] [10] , which were proved in the normed space context and with respect to statistical convergence. In [11] -[17] , some Schur, boundedness, decomposition and/or convergence theorems are given in the lattice group setting with respect to a suitable class of filters, extending some results of [18] , while for positive measures it is possible to have some similar results even for a larger class of filters (see also [19] ). Analogous results have been established also in the setting of topological group-valued measures in [20] [21] . In [22] -[24] , some limit theorems are proved by means of the tool of uniform filter/ideal exhaustiveness. Moreover, in [25] , some results about equivalence between BrooksJewett, Vitali-Hahn-Saks, Nikodým convergence and Dieudonné-type theorems are presented, using the Stone Isomorphism technique and extending results of [26] , given in the classical case for topological group-valued measures. In this paper we use sliding hump-type techniques, similar to those used in the topological group-setting first by D. Candeloro and G. Letta in 1985 in [27] -[30] for proving limit and boundedness theorems for families of finitely additive group-valued measures defined on suitable Boolean algebras, and successively in [4] [5] [16] [17] [20] [21] in the setting of lattice groups and filter convergence. We prove some new further versions of Nikodým convergence, boundedness and Brooks-Jewett-type theorems for lattice group-valued measures, defined on a σ-algebra of an abstract nonempty set. The results and the proofs are direct and, differently than in [14] [16] , without using Schur-type theorems proved for measures defined on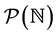 . Finally, we pose some open problems.
. Finally, we pose some open problems.
2. Preliminaries
A lattice group (shortly,  -group)
-group) 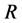 is said to be Dedekind complete iff every nonempty subset of
is said to be Dedekind complete iff every nonempty subset of , bounded from above, admits supremum in
, bounded from above, admits supremum in . A Dedekind complete lattice group is said to be super Dedekind complete iff for every nonempty set
. A Dedekind complete lattice group is said to be super Dedekind complete iff for every nonempty set , bounded from above, there exists a countable subset
, bounded from above, there exists a countable subset , such that
, such that .
.
Let 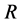 be a Dedekind complete
be a Dedekind complete  -group. A sequence
-group. A sequence  of positive elements of
of positive elements of  is called an
is called an  -sequence iff it is decreasing and
-sequence iff it is decreasing and . A sequence
. A sequence 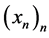 in
in  is said to be order convergent (or
is said to be order convergent (or  -convergent) to
-convergent) to  iff there exists an
iff there exists an 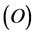 -sequence
-sequence  in
in 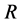 such that for every
such that for every  there is a positive integer
there is a positive integer  with
with  for all
for all , and in this case we will write
, and in this case we will write . A bounded double sequence
. A bounded double sequence  in
in  is called a
is called a  -sequence or a regulator iff for each
-sequence or a regulator iff for each  the sequence
the sequence  is an
is an  -sequence. A sequence
-sequence. A sequence  in
in 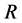 is said to be
is said to be  -convergent to
-convergent to  (and we write
(and we write ) iff there exists a
) iff there exists a  -sequence
-sequence  in
in , such that to every
, such that to every 
there is  with
with  for every
for every . An
. An 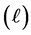 -group
-group  is weakly
is weakly  -distributive iff
-distributive iff  for every
for every  -sequence
-sequence .
.
Observe that, if  is the
is the  -algebra of all Borel subsets of
-algebra of all Borel subsets of  and
and 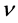 is the Lebesgue measure, then the space
is the Lebesgue measure, then the space  of all
of all  -measurable real-valued functions on
-measurable real-valued functions on  (with identification up to
(with identification up to  -null sets) is super Dedekind complete and weakly
-null sets) is super Dedekind complete and weakly 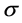 -distributive (see also [5] , [6] , Example 2.17).
-distributive (see also [5] , [6] , Example 2.17).
We now recall the following theorem, which links  - and
- and  -sequences in lattice groups.
-sequences in lattice groups.
Theorem 2.1 ([25] , Theorem 2.3, see also [31] , Theorems 3.1 and 3.4) Given any Dedekind complete  -group
-group  and any
and any 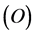 -sequence
-sequence  in
in , the double sequence defined by
, the double sequence defined by ,
,  , is a regulator, such that for every
, is a regulator, such that for every , if
, if , then
, then  Conversely, if
Conversely, if  is super Dedekind complete and weakly
is super Dedekind complete and weakly  -distributive, then for every
-distributive, then for every  -sequence
-sequence  in
in  there are an
there are an  -sequence
-sequence  in
in 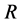 and a sequence
and a sequence  in
in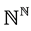 , with
, with  for each k.
for each k.
Let 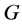 be any nonempty set and
be any nonempty set and  be a
be a  -algebra. A bounded finitely additive measure
-algebra. A bounded finitely additive measure
 is said to be
is said to be  -additive (resp.
-additive (resp.  -bounded) on
-bounded) on  iff
iff  (resp.
(resp. ) for each disjoint sequence
) for each disjoint sequence  in
in , where
, where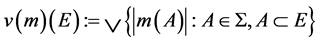 ,
,
 , is the semivariation of
, is the semivariation of  on
on . Moreover, if
. Moreover, if 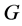 is a compact Hausdorff topological space and
is a compact Hausdorff topological space and  is its Borel
is its Borel  -algebra, we say that a positive finitely additive measure
-algebra, we say that a positive finitely additive measure  is regular iff for every
is regular iff for every 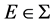 there exists a
there exists a  -sequence
-sequence , depending on
, depending on , such that for all
, such that for all  there are a compact set
there are a compact set  and an open set
and an open set  with
with  and
and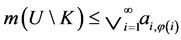 . We recall the following.
. We recall the following.
Theorem 2.2 ([32] , Theorem 2.2) Assume that  is a Dedekind complete and weakly
is a Dedekind complete and weakly 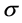 -distributive Riesz space, and let
-distributive Riesz space, and let 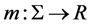 be a regular measure defined on the Borel
be a regular measure defined on the Borel  -algebra of a compact Hausdorff space
-algebra of a compact Hausdorff space . Then
. Then  is
is  -additive.
-additive.
The following result (Fremlin lemma, see [33] , Lemma 1C) allows us to replace a countable family or a “series” of  -sequences with a single regulator.
-sequences with a single regulator.
Lemma 2.3 Let  be any Dedekind complete
be any Dedekind complete  -group and
-group and ,
,  , be a sequence of regulators in
, be a sequence of regulators in . Then for every
. Then for every ,
,  there exists a
there exists a  -sequence
-sequence  in
in  with
with
 for every
for every  and
and .
.
We now give some basic properties of filters, which will be useful in the sequel. Let  be a countable set and
be a countable set and  be a filter of
be a filter of . A subset of
. A subset of 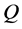 is
is 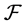 -stationary iff it has nonempty intersection with every element of
-stationary iff it has nonempty intersection with every element of . We denote by
. We denote by  the family of all
the family of all 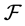 -stationary subsets of
-stationary subsets of .
.
A filter  of
of 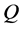 is said to be diagonal iff for every sequence
is said to be diagonal iff for every sequence  in
in 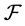 and for each
and for each  there exists a set
there exists a set ,
, 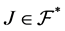 such that the set
such that the set  is finite for all
is finite for all  (see also [15] [16] [18] ). Given an infinite set
(see also [15] [16] [18] ). Given an infinite set , a blocking of
, a blocking of  is a countable partition
is a countable partition 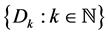 of
of  into nonempty finite subsets. A filter
into nonempty finite subsets. A filter 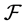 of
of  is said to be block-respecting iff for every
is said to be block-respecting iff for every  and for each blocking
and for each blocking 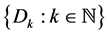 of
of  there is a set
there is a set ,
,  with
with  for all
for all , where
, where 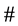 denotes the number of elements of the set into brackets. A particular class of filters, which are block-respecting and diagonal at the same time, is that of the category respecting filters. A filter
denotes the number of elements of the set into brackets. A particular class of filters, which are block-respecting and diagonal at the same time, is that of the category respecting filters. A filter 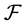 of
of  is said to be category respecting iff for every compact metric space
is said to be category respecting iff for every compact metric space  and for every family of closed subsets
and for every family of closed subsets  of
of , if
, if  whenever
whenever  in
in  and
and , then there is a set
, then there is a set  such that the interior of
such that the interior of  is nonempty (see also [18] , Theorem 4.3).
is nonempty (see also [18] , Theorem 4.3).
Let  be a disjoint partition of
be a disjoint partition of 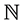 into infinite subsets. For each sequence
into infinite subsets. For each sequence 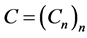 of finite subsets
of finite subsets 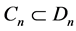 and every
and every , set
, set  The filter
The filter  generated by the sets of type
generated by the sets of type  is a non-diagonal and block-respecting filter. Furthermore, note that the filter of all subsets of
is a non-diagonal and block-respecting filter. Furthermore, note that the filter of all subsets of  having asymptotic density one is a diagonal and not block-respecting filter (see also [18] ).
having asymptotic density one is a diagonal and not block-respecting filter (see also [18] ).
If , then the trace
, then the trace  of
of  on
on  is the family
is the family . It is not difficult to see that
. It is not difficult to see that  is a filter of
is a filter of  (see also [21] ).
(see also [21] ).
Remark 2.4 Observe that, if  is a block-respecting filter of
is a block-respecting filter of , then
, then  is a block-respecting filter of
is a block-respecting filter of  for every
for every  (see also [20] , Proposition 2.1, [21] , Proposition 2.3).
(see also [20] , Proposition 2.1, [21] , Proposition 2.3).
We now recall some main properties of filter convergence in the lattice group setting (see also [1] [16] ).
Let  be a filter of
be a filter of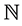 . A sequence
. A sequence  in
in 
 -converges to
-converges to 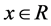 iff there is a
iff there is a  -sequence
-sequence  with the property that
with the property that  for each
for each .
.
Let  be any arbitrary nonempty set. A family
be any arbitrary nonempty set. A family  is said to be
is said to be  -convergent to a family
-convergent to a family 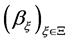 with respect to
with respect to  iff there is a regulator
iff there is a regulator  such that for each
such that for each  and
and  we get
we get 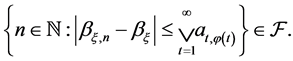
Given , set
, set . For
. For ,
,  , put
, put ,
,  (
( times). Let
times). Let ,
,  , be such that
, be such that  for every
for every . A set
. A set  is said to be
is said to be  -
- -bounded by
-bounded by , iff
, iff , and
, and  -eventually bounded by
-eventually bounded by  iff it is
iff it is  -
- -bounded by
-bounded by  (see also [1] [15] [16] [34] ).
(see also [1] [15] [16] [34] ).
3. The Main Results
We begin with recalling the following Lemma 3.1 ([16] , Lemma 2.3) Let  be a diagonal filter of
be a diagonal filter of ,
,  be a sequence in
be a sequence in  with
with  with respect to an
with respect to an  -sequence
-sequence . Then for every
. Then for every  there exists
there exists  such that
such that  and
and  with respect to the same
with respect to the same  -sequence
-sequence .
.
We now prove the main result, by means of sliding hump-type techniques.
Theorem 3.2 Let  be a Dedekind complete
be a Dedekind complete  -group,
-group, 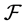 be a block-respecting filter of
be a block-respecting filter of ,
,  ,
,  , be a sequence of equibounded
, be a sequence of equibounded  -additive measures,
-additive measures,  be a disjoint sequence in
be a disjoint sequence in , with
, with
(i)  for any
for any , and
, and
(ii)  with respect to
with respect to . Then3.2.1) for every strictly increasing sequence
. Then3.2.1) for every strictly increasing sequence  in
in  we get
we get
 (1)
(1)
3.2.2) if 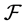 is also diagonal and
is also diagonal and  is super Dedekind complete and weakly
is super Dedekind complete and weakly  -distributive, then the only condition (ii) is sufficient to get (1).
-distributive, then the only condition (ii) is sufficient to get (1).
Proof: For each , set
, set . Let
. Let : such an element does exist in
: such an element does exist in , thanks to equiboundedness of the
, thanks to equiboundedness of the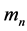 ’s. For each
’s. For each  let
let  be a
be a  -sequence related with
-sequence related with  -additivity of
-additivity of 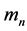 and the sequence
and the sequence . For every
. For every 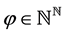 and
and  there is
there is , with
, with
 (2)
(2)
By the Fremlin Lemma 2.3 there is a  -sequence
-sequence  with
with
 (3)
(3)
for each  and
and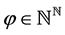 . From (2) and (3) it follows that for every
. From (2) and (3) it follows that for every  and
and  there exists
there exists
 with
with 
Let  be a regulator, satisfying the condition of
be a regulator, satisfying the condition of  -convergence as in (ii).
-convergence as in (ii).
Since  for every
for every , then for each
, then for each  there exists a regulator
there exists a regulator  such that for every
such that for every  there is
there is  with
with  for all
for all . Since the
. Since the ’s are equibounded, arguing analogously as above, by the Fremlin Lemma 2.3 we find a regulator
’s are equibounded, arguing analogously as above, by the Fremlin Lemma 2.3 we find a regulator 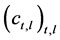 such that for each
such that for each  and
and  there exists
there exists  with
with .
.
Again by Lemma 2.3, there are two  -sequences
-sequences ,
,  , with
, with
 (4)
(4)
 (5)
(5)
for every  and
and . For every
. For every , set
, set
 (6)
(6)
We prove that the  -sequence
-sequence  satisfies the condition of
satisfies the condition of  -convergence in 3.21). Otherwise there is
-convergence in 3.21). Otherwise there is  with the property that
with the property that 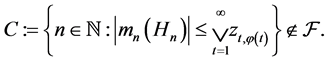
We get that : otherwise, there would be
: otherwise, there would be  with
with
 , that is
, that is  and hence
and hence , a contradiction.
, a contradiction.
Let . By
. By  -additivity of
-additivity of , there is a cofinite subset
, there is a cofinite subset , with
, with
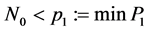 , and
, and 
where . By (i) there is an integer
. By (i) there is an integer 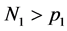 with
with  whenever
whenever  and
and
 . By
. By  -additivity of
-additivity of ,
,  , there is a cofinite subset
, there is a cofinite subset , with
, with and
and  for every
for every , where
, where . Proceeding analogously as abovewe find an integer
. Proceeding analogously as abovewe find an integer  with
with 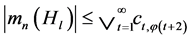 whenever
whenever  and
and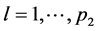 .
.
By induction, it is possible to find: a strictly decreasing sequence  of cofinite subsets of
of cofinite subsets of , a strictly decreasing sequence
, a strictly decreasing sequence  in
in 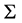 and two strictly increasing sequences
and two strictly increasing sequences ,
,  in
in  such that, for every
such that, for every ,
, 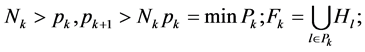
 (7)
(7)
 (8)
(8)
Since  is block-respecting, there is
is block-respecting, there is ,
,  , with
, with  for every
for every . As
. As , then either
, then either  or
or . Without loss of generality, let
. Without loss of generality, let  (see also [15] [16] [18] ). Put
(see also [15] [16] [18] ). Put . We have:
. We have:
 (9)
(9)
Since  and
and
 (10)
(10)
from (7) and (10) we get
 (11)
(11)
Moreover, since  for every
for every , from (8) we obtain
, from (8) we obtain
 (12)
(12)
If , then from (9), (11) and (12) we have
, then from (9), (11) and (12) we have 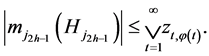
But we know that  and so we get a contradiction.
and so we get a contradiction.
Thus  for all
for all , and hence
, and hence 
Since, by (ii),  , we obtain that
, we obtain that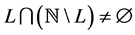 , which is absurd. This proves 3.2.1).
, which is absurd. This proves 3.2.1).
3.2.2) Put ,
, 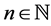 , and let
, and let  be a
be a  -sequence, satisfying
-sequence, satisfying  -convergence in condition (ii). Of course, for every
-convergence in condition (ii). Of course, for every  -stationary set
-stationary set , the regulator
, the regulator  satisfies (ii) also with respect to
satisfies (ii) also with respect to  -convergence. Since
-convergence. Since  is super Dedekind complete and weakly
is super Dedekind complete and weakly  -distributive, by Theorem 2.1 there is an
-distributive, by Theorem 2.1 there is an  -sequence
-sequence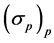 , satisfying condition (ii), when
, satisfying condition (ii), when  -convergence is replaced with
-convergence is replaced with  -or
-or  -convergence. For every
-convergence. For every  there is
there is ,
,  , with
, with ,
,  , with respect to
, with respect to  (see also Lemma 3.1). From this and Theorem 2.1 it follows that there is a regulator
(see also Lemma 3.1). From this and Theorem 2.1 it follows that there is a regulator , such that for every
, such that for every  there exists
there exists ,
,  , with
, with
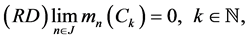 (13)
(13)
with respect to . Let now
. Let now ,
,  , be regulators associated to
, be regulators associated to  -additivity of the
-additivity of the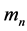 ’s,
’s,  be as in the proof of 3.2.1),
be as in the proof of 3.2.1),  be as in (3) and
be as in (3) and ,
,  ,
,  be as in (4), (5), (6) respectively. We prove that the regulator
be as in (4), (5), (6) respectively. We prove that the regulator 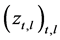 satisfies 3.2.2). Otherwise, by proceeding analogously as in the proof of 3.2.1), we find
satisfies 3.2.2). Otherwise, by proceeding analogously as in the proof of 3.2.1), we find  and
and  with
with 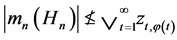 for each
for each . In correspondence with
. In correspondence with , there is
, there is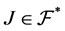 ,
, 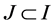 , satisfying (13). Note that the sequence
, satisfying (13). Note that the sequence ,
,  , does not
, does not 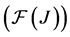 converge to 0 (see also [18] ). Since
converge to 0 (see also [18] ). Since 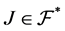 and
and  is block-respecting, then, by Remark 2.4,
is block-respecting, then, by Remark 2.4,  is block-respecting too. By 3.2.1) used with
is block-respecting too. By 3.2.1) used with ,
,  , and
, and , it follows that
, it follows that , getting a contradiction. This proves 3.2.2). A result analogous to Theorem 3.2 holds in the setting of finitely additive measures.
, getting a contradiction. This proves 3.2.2). A result analogous to Theorem 3.2 holds in the setting of finitely additive measures.
Theorem 3.3 Let  be a Dedekind complete
be a Dedekind complete  -group,
-group,  be as in Theorem 3.2,
be as in Theorem 3.2,  be a blockrespecting filter of
be a blockrespecting filter of ,
, 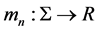 ,
,  , be an equibounded sequence of finitely additive measures, and assume that
, be an equibounded sequence of finitely additive measures, and assume that
(i)  for any
for any ;
;
(ii) 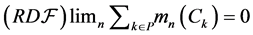 with respect to
with respect to .
.
Then for every strictly increasing sequence  in
in  we get
we get
 (14)
(14)
If  is also diagonal and
is also diagonal and  is super Dedekind complete and weakly
is super Dedekind complete and weakly  -distributive, then the only condition (ii) is enough to get (14).
-distributive, then the only condition (ii) is enough to get (14).
Indeed, it will be enough to apply Theorem 3.2 to the measures , defined by
, defined by
 Analogously as Theorem 3.2 it is possible to prove a Nikodým boundedness-type theorem in the context of
Analogously as Theorem 3.2 it is possible to prove a Nikodým boundedness-type theorem in the context of  -groups and filter convergence, extending [34] , Theorem 4.6 (see also [15] Lemma 3.4).
-groups and filter convergence, extending [34] , Theorem 4.6 (see also [15] Lemma 3.4).
Theorem 3.4 Let  be any Dedekind complete
be any Dedekind complete  -group,
-group,  ,
,  ,
,  ,
,  be a block-respecting filter of
be a block-respecting filter of ,
,  ,
,  , be a sequence of finitely additive measures, and assume that
, be a sequence of finitely additive measures, and assume that
3.4.1) for every disjoint sequence  in
in 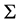 and
and  there is a cofinite set
there is a cofinite set  with
with for each
for each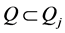 .
.
Let  be a disjoint sequence in
be a disjoint sequence in 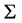 and
and  be an increasing sequence of positive elements of
be an increasing sequence of positive elements of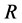 . For each
. For each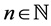 , set
, set  and
and . Moreover suppose that:
. Moreover suppose that:
(i) the set  is
is 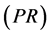 -eventually bounded by
-eventually bounded by  for each
for each ;
;
(ii) the set  is
is  -
-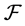 -bounded by
-bounded by  for each
for each .
.
Then we get:
3.4.2) for every strictly increasing sequence 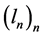 in
in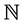 , the set
, the set  is
is  -
- - bounded by
- bounded by ;
;
3.4.3) if 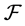 is also diagonal, then the only condition (ii) is enough in order that
is also diagonal, then the only condition (ii) is enough in order that  is
is  -
-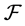 -bounded by
-bounded by .
.
Proof: For every , let
, let . If the thesis of the theorem is not true, then
. If the thesis of the theorem is not true, then  . Set
. Set . By 3.4.1) there is a cofinite set
. By 3.4.1) there is a cofinite set , with
, with  and
and  for each
for each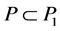 . By (i) there is
. By (i) there is  with
with  for each
for each  and
and . By induction, there are a strictly decreasing sequence
. By induction, there are a strictly decreasing sequence 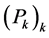 of subsets of
of subsets of  and two strictly increasing sequences
and two strictly increasing sequences ,
,  of positive integers such that, for each
of positive integers such that, for each ,
,
• ,
, ;
; 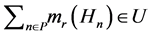 for every
for every  and
and ;
;
•  for any
for any  and
and .
.
As  is block-respecting, proceeding analogously as in the proof of Theorem 3.2, we find a set
is block-respecting, proceeding analogously as in the proof of Theorem 3.2, we find a set  ,
,  , with
, with  for every
for every . For any
. For any  we have:
we have:
 (15)
(15)
 ,
,  ,
,  , and
, and
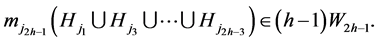 (16)
(16)
Put . If
. If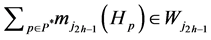 , then from (15) and (16) we get
, then from (15) and (16) we get
 and
and . This contradicts
. This contradicts . Thus
. Thus  for every
for every , and hence
, and hence .
.
From this, arguing as in 3.2.1), we obtain a contradiction, and this proves 3.4.2). From 3.4.2), proceeding analogously as in the proof of Theorem 3.2, we get 3.4.3). Open problems: (a) Find some versions of limit theorems with respect to some other classes of filters and/or algebras satisfying suitable properties.
(b) Find some convergence and boundedness theorems with respect to other kinds of  -boundedness,
-boundedness,  - additivity or boundedness.
- additivity or boundedness.
3. Conclusions
The Schur, Nikodým convergence and boundedness, Vitali-Hahn-Saks and Dieudonné limits have been widely investigated in the literature since the beginning of the last century, and there are several extensions of them along different directions, concerning for example the set of definition of the involved set functions, their properties and the structure of their range. The novelties in our context are both the structure of the space in which the considered measures ta ke values and the types of convergence: indeed we deal with filter/ideal convergence in lattice groups introduced in [1] . Moreover we use a new technique in the filter setting, inspired by those in [27] -[30] and [18] , and similar to that in [20] , which allows us to prove some Nikodým-type convergence and boundedness theorems with respect to filter convergence for measures defined on a  -algebra of parts of an abstract nonempty set
-algebra of parts of an abstract nonempty set 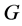 with a direct approach, without using earlier Schur-type results for measures defined on
with a direct approach, without using earlier Schur-type results for measures defined on . In this context the main properties of diagonal and block-respecting filters are used, which allow us to apply the sliding hump argument to filter convergence. So it is possible to pose the question of finding some other classes of filters for which similar theorems hold or for which they are not valid, for measures, or even not necessarily finitely additive set functions, taking values in different types of abstract structures, like topological or lattice groups or metric semigroups, and defined in algebras, which satisfy similar properties and are not necessarily
. In this context the main properties of diagonal and block-respecting filters are used, which allow us to apply the sliding hump argument to filter convergence. So it is possible to pose the question of finding some other classes of filters for which similar theorems hold or for which they are not valid, for measures, or even not necessarily finitely additive set functions, taking values in different types of abstract structures, like topological or lattice groups or metric semigroups, and defined in algebras, which satisfy similar properties and are not necessarily  -algebras. Note that, in general, filter convergence is not inherited by subsequences. In this context, another problem that one can pose is to find similar results on convergence and boundedness theorems for non-additive set functions, or results like some kinds of uniform
-algebras. Note that, in general, filter convergence is not inherited by subsequences. In this context, another problem that one can pose is to find similar results on convergence and boundedness theorems for non-additive set functions, or results like some kinds of uniform  -boundedness of
-boundedness of  -additivity when the limits of the involved sequences are intended in the filter sense, and/or with respect some other kinds of convergence in the lattice group-context, like order convergence (see also [2] ).
-additivity when the limits of the involved sequences are intended in the filter sense, and/or with respect some other kinds of convergence in the lattice group-context, like order convergence (see also [2] ).
Acknowledgements
Our thanks to the anonymous referee for his/her remarks which improved the exposition of the paper.
2010 A. M. S. Classifications
Primary: 26E50, 28A12, 28B10, 40A35, 54A20. Secondary: 06E15, 46G12, 54H11, 54H12.
NOTES
*Corresponding author.