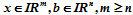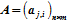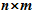In this paper, we find two formulas for the solutions of the following linear equation , where is a real matrix. This system has been well studied since the 1970s. It is known and simple proven that there is a solution for all 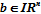if, and only if, the rows of A are linearly independent, and the minimum norm solution is given by the Moore-Penrose inverse formula, which is often denoted by 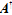; in this case, this solution is given by 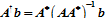. Using this formula, Cramer’s Rule and Burgstahler’s Theorem (Theorem 2), we prove the following representation for this solution

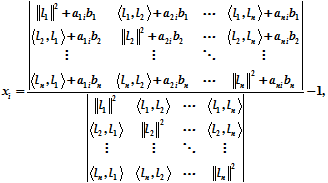
, where 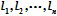are the row vectors of the matrix A. To the best of our knowledge and looking in to many Linear Algebra books, there is not formula for this solution depending on determinants. Of course, this formula coincides with the one given by Cramer’s Rule when .