1. Introduction
Published in 1859, Reimann Hypothesis attempts to predict the occurrence of prime numbers using a mathematical function. Prime numbers do not follow a pattern of occurrence. After you find one, it is impossible to predict the occurrence of the next prime number. Mathematical greats like Euclid, Euler, and Gauss are among many who attempted to address this problem. Bernhard Riemann, a student of Gauss, found a pattern in the frequency of prime numbers. He found them to follow a pattern that could be explained with a function, which he called Riemann zeta function. The Riemann’s function formulation is defined as [1] [2] :
(1)
● The zeta function plays an important role in mathematical research. It constitutes a first link between arithmetic and analysis. It was used by Euler, Dirichlet, Tchebychev and Riemann to study the distribution of prime numbers.
● The Riemann zeta function and the Dirichlet L-functions are powerful analytical tools for studying the distribution of prime numbers. It seems that these functions are also revealing of the most hidden properties of number theory. They are far from being well understood!
● In 1737, Leonhard Euler (1707-1783) studies the zeta function and discovers Euler’s identity between prime numbers and integers.
● In 1900, David Hilbert (1862-1943) places the Riemann hypothesis among the great mathematical challenges of the 20th century
● Since 1920, Number theory and algebraic geometry tend to be unified. These functions are perhaps only the fragmentary elements of a more general theory to be discovered. Dedekind generalized these functions and relations to integer ideals and prime ideals.
● The German Edmund Landau assumed the Riemann conjecture to be true, and showed that a large number of conclusions would be drawn from it.
● The study of the complex zeta function of Riemann shows that it passes through the value zero. It exists:
- trivial (uninteresting) zeros like −2, −4, 6 ... and
- Particular zeros which seem to line up on the line of the real
.
● The Riemann hypothesis or conjecture consists in asserting that all non-trivial zeros are on this line
[3] . Novely, Mercedes Orús-Lacort and al has been performed a detailed analysis of Riemann’s hypothesis, dealing with the zeros of the analytically-extended zeta function [4] .
● The complex issue of the Riemann’s Hypothesis and ultimately its elementary proof was explained by Jan Feliksiak [5] , the numerically and computationally provable was been provided by Suhaas Pediredla [6] . We are going to proove that all zeros are on the line
with the first is in
. And all imaginary values are irrational [7] .
The gap in all these researches is found in their accurates, that we are going to perform in this work.
Firstly we are going to take a sample of particular zeros that the are going to increase gradually it number and establishing a function that accurate the whole and finally prooving that this function is always irrational.
2. Methods
Let variable
in which each element
the index of Riemann zeta function non trivial zero
element of variable
the Riemann zeta function non trivial zero, as
[8] [9] [10] . We wish to fit the model for
(2)
where
,
, and
is uncorrelated across measurements. The sum of squares for n known data points is given by:
(3)
As you can see we have n + 1 coefficients
in the equation. Partials derivate of a is given by:
(4)
Partial derivate of
is given as:
(5)
Now to find the minima, we will set the partial derivatives to 0.
(6)
we get a generalized matrix [11] [12] [13] :
An overdetermined system is solved by first creating a residual function, summing the square of the residual which forms a parabola/paraboloid, and then finding the coefficients by finding the minimum of the parabola/paraboloid using partial derivatives. It give that since the set
is the infinity countable numbers, every n th non-trivial zero of the Riemann zeta function exists, which means that all non-trivial zeros lie on the line of real
of the riemann zeta. We are going now to proove that for nth no-trivial zero existing, this zero is
.
For this we are going to calculate all polynomial coefficients in prooved that at least these one of all is real [14] [15] .
3. Results and Discussion
Frisly we are going to compute a Riemann Zéta Non trivial zeros number data with
by using this code:
import numpy as np
import matplotlib.pyplot as plt
#The next library contains the zeta(), zetazero(),and siegelz() functions from mpmath import *
mp.dps = 25; mp.pretty = True
D=[]
def graph_zeta(real, image_name):
A,B,C = [], [], []
for i in np.arange(0.1, , 0.1):
function = zeta(real + 1j*i)
function1 = siegelz(i)
A.append(abs(function))
B.append(function1)
C.append(i)
return A,B,C
A,B,C=graph_zeta(0.5, "Z(t)_Plot.png")
fig = plt.figure()
ax = fig.add_subplot(111)
ax.grid(True)
ax.plot(C,A,label='modulus of Riemann zeta function along critical line, s = 1/2 + it', lw=0.8)
ax.plot(C,B, label='Riemann-Siegel Z-function, Z(t)', lw=0.8)
ax.set_title("Riemann Zeta function - re(s)=1/2")
ax.set_ylabel("Z(t)")
ax.set_xlabel("t")
D.append(zero.imag)
#Include legend
leg = ax.legend(shadow=True)
#Edit font size of legend to make it fit into chart
for t in leg.get_texts():
t.set_fontsize('small')
#Edit the line width in the legend
for l in leg.get_lines():
l.set_linewidth(2.0)
#Plot the zeroes of zeta
for i in range(1, \tau):
zero = zetazero(i)
ax.plot(zero.imag, [0.0], "ro")
#save plot and print that it was saved
ax.set_ylim(-7, 7)
plt.savefig("Z(t)_Plot.png")
print("Successfully plotted %s !" % "Z(t)_Plot.png")
show
Let’s begining with
, Figure 1.
Here is a code to save data:
D=[] a=np.linspace(1, 100, 2000, endpoint=True)
for i in a:
zero = zetazero(i)
D.append(zero.imag)
plt.plot(a,D,'b*',label='Mark')
plt.xlabel('n')
plt.ylabel('zero image')
plt.legend(loc='upper left')
plt.show(
An exemple of 2000 data points are been plotted in Figure 2.
Evidently, our study is based on much data as
with
. Accordingly, evaluating those data points with polynomial regression, we obtain a
residual for polynomial degree
for Riemann Zéta Non trivial zeros data
(Figure 3).
However, we are going to generalize for all existing zeros. Firstly let’s define mathematical expression of each coefficient:
![]()
Figure 1. For tau = 2000 data points (non trivial zero in red).
![]()
Figure 2. An exemple of 2000 data points plotted.
Using Cramer methods we get:
 (7)
(7)
Let’s
(8)
(9)
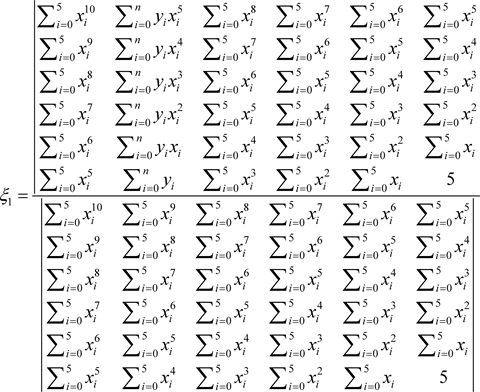 (10)
(10)
Let’s
(11)
(12)
 (13)
(13)
Let’s
(14)
(15)
 (16)
(16)
Let’s
(17)
(18)
 (19)
(19)
Let’s
(20)
(21)
 (22)
(22)
Let’s
(23)
(24)
Evidently each
varies everytime the
increase. We must then establish for each
a function describing it evolution. By increasing
value, consequently,
coefficient is described as for
:
(25)
coefficient is described as:
(26)
coefficient is described as for
:
(27)
coefficient is described:
(28)
coefficient is described as for
:
(29)
For any coefficient, It is define as:
. Necessarily therefor the best accurate polynomial function for non trivial Riemann zeta function zeros is defined as:
(30)
Direct computational analysis verifies that for
, the residual value increase and the polynomial degree must been decreased for
, with an existing
maintaining residual value constant for
, x being Riemann zeta function non trivial zero index. Consequently
such as
the polynomial function is generalized as:
(31)
This polynomial function is defined from
to
for any Rieman zeta non trivial zero. That proove that all non trivial zeros are in
and the each zero is only in
.
4. Conclusion
In this work, we have presented a method for solving the Riemann hypothesis conjecture. We began our study by computing and saving Riemann’s zeta function non trivial zeros, then we fit each data points and studied the polynomial coefficients variations maintaining the best accurate constant and finished by solving the Riemann hypothesis conjecture. This work is one best demonstration of the validity of Riemman’s conjecture. As such, we have proven here that the conjecture is true, up to the best of our numerical analysis. The demonstration of the work is important as it allows Riemann’s zeta function to be a model function in the Dirichlet series theory and be at the crossroads of many other theories.
Scope of Future Work
The present investigation will be very helpful to the researchers who are engaged for those area research works in earth and in Universe [16] [17] [18] [19] .
1) Mathematics. This is so because any number, when broken down into its factors at the end, can be defined as the multiplication of prime numbers.
2) Music, all the structures of tonal music like chords, scales, harmony, modality, it is possible to represent them all mathematically as rational structures of prime numbers.
3) Nature, many interesting examples of prime numbers exist because some insects like cicadas only emerge from the underground habitat after the prime number of years, such as 17 years. Often flowers have an odd number of petals, and most often these are prime numbers. For example, five is a commonly found number of petals in flowers.
List of Symbols
: Polynomial function;
: Riemann’s Zéta function;
z: Complex number;
RSS: Squares sum of n known data points;
: Integers;
: Rational Numbers;
: Real Numbers;
: Uncorrelated across measurements;
: Riemann Zéta Non trivial zeros number;
X: The index of Riemann zeta function non trivial zero numbers;
Y: The Riemann zeta function non trivial zero numbers;
var: The Variance;
: Infinity;
E: Exponent;
: Polynomial coefficients;
: Sum;
: Parameter;
: Parameter;
: Parameter;
: Parameter;
: Real part;
: Partials derivate;
: expectation probability.