1. Introduction
Some of the unsolved problems of number theory are related to the equidistance to a central point. Such are the twin primes equidistantly placed around the series of multiples of six and the union of primes equidistantly placed around an even number. Reflecting the union of the series of multiples of primes around a point creates symmetric assembly. This allows analyzing and solving these unsolved problems.
Two equidistant primes around a prime at the point of reflection compose a triad. The evaluation of the number of triads follows the same procedure, as the evaluation of the number of primes in the case of the simple density of occupation (see Ref. [1] ). This procedure is the summation of all sections of the number of primes within each section. The length of the sections is equal to the square root of the distance of the point of reflection to the origin. The number of primes within each section is evaluated with the local density. The simplification in the case of the double density of occupation results in the triads-number-formula, similarly to the simplification resulting in the prime-number-formula in the case of the simple density of occupation (see Ref. [1] ).
As the prime-number-formula represents the low limit of the number of primes, the triads-number-formula gives the low limit of the number of triads. If the point of reflection is not a prime, the equidistant primes compose diads. The low limit of the number of diads is growing to infinity as well, proving Goldbach’s conjecture.
The number of diads with any given difference between the primes composing the diads grows to infinity, allowing proving the infinity of the number of twin primes.
Because of the symmetry condition, the number of primes within the first section of the summation procedure is equal to the number of primes within the last section. Therefore, the number of primes within the last section is given by the number of primes up to the square root of the distance of the point of reflection. Since this number—given by the triads-number-formula—is certainly smaller than the corresponding number within the same section in the case of the simple density of occupation, this proves that there are primes in the last section, and therefore, the set of the number of primes represents a continuum.
The present paper starts with the analysis of the symmetric, double density of occupation of integer positions by the union of the straight and the reflected series of multiples of primes. The evaluation of the low limit of the integer positions left free by this arrangement proves Goldbach’s conjecture. The analysis of other effects of the double density arrangement follows: the presence of primes within the section of the size equal to the square root of the distance to the origin, the infinitely growing prime gaps, the infinity of the number of primes with a given size of the distance between them, proving the infinity of the number of twin primes.
The most important results are checked in the annexes with numeric results. First in Annex 1, general data and vectors are given, together with definitions of functions used in the following annexes.
2. The Number of Free Positions Left in Case of the Double Density of Occupation
The series of multiples (arithmetic progression) of any prime (
) in union with the same series reflected over a point at the distance (c,
) from the origin, cover a subset of all integer positions. The positions covered by the straight and by the reflected series of multiples of any prime are mutually exclusive: They cover (
)-th part of all integer positions. The union of the first (
) series of multiples of primes and of their reflected series results the double density of occupation. Each of the pairs of free positions—left by the double density ofoccupation—compose with the prime at the point of reflection the set of central triads. This method may be regardedas a special case of the sieve proposed in Ref. [2] [3] .
The share of the integer positions left free by the double density of occupation of each of the series of multiples of primes is: (
). The density of free positions left by the series of multiples of the first prime is (1/2). The density of free positions is multiplicative, therefore the density of free positions left by the double density of occupation of the first (
) primes, at the distance (c) from the origin is:
(1.1)
This under the condition, that the distance of the point of reflection to the origin is relative prime to all (
) primes.
If the distance of the point of reflection (c) is divisible by any of the primes (
) with (
), then the reflected series of multiples of this prime do not cover any additional positions over the number already covered by the single, straight series of multiples of the same prime. The local density of free positions is in this case:
(1.2)
Lemma 1.1:
The density of free positions left by the double density of occupation has a minimum in case the distance of the point of reflection to the origin is equal to a prime (
).
Proof: The series of multiples of any prime (
) together with its reflected series, occupy the (
)-th part of all integer positions, if the distance of the point of reflection is relative prime to all primes up to: (
). Otherwise, the series of multiples of the prime dividends of the distance (c) occupy only a share of the (
)-th part of all integer positions. Therefore, the share of the remaining free positions left by the double density of occupation has a minimum, if the point of reflection is relative prime to all primes, meaning it is a prime itself, as stated in the lemma and concluding the proof.
Definition of triads and diads
If the point of reflection is a prime, then the positions left free by the double density of occupation represent primes, which are equidistantly placed around the prime at the point of reflection. Two primes equidistantly placed around the prime at the point of reflection compose a central triad. The distance to the origin of the prime at the center of the triads is the mean value of the distances to the origin of the two other primes. In case the point of reflection is not a prime, then the equidistant primes compose diads.
The local density of free positions left at the distance (d,
) from the point of reflection by the straight series of multiples is (
), by the reflected series is (
). The combined local density of free positions is:
(1.3)
Here the factor of correction (
) had to be introduced. It accounts for the replacement of the double density (1.2) by the product of the local single densities:
(1.4)
The factor quickly converges to a constant value, because of the following convergencies:
with
.
The factor (
) is evaluated in Annex 2. Replacing the distance of the point of the local density to the center of reflection in (1.3) by the distance to the origin (
), respectively to the point at the double distance to the origin of the center of the reflection (
) gives the density of diads, respectively—with the density of the prime at the point of reflection—the density of triads:
(1.5)
Similarly, to the evaluation of the number of primes as the integral of the local logarithmic density of primes by Riemann (see Ref. [1] ), the total number of triads results of the integral of the density (1.5). The approximation of the integral by the sum of the logarithmic densities over all integers gives the best estimate number of triads, evaluated in Annex 3:
(1.6)
This above sum may be written as summing up first over all integers within the sections of the length (
) and then summing up over all the (
) sections of the length (
). Taking the average value over each section and—as a first simplification—summing up over the sections gives:
(1.7)
With Lemma 1.1 the number of triads corresponds to the minimum of the number of diads. Therefore, in the following the minimum, the number of triads will be evaluated. The lower limit of the best estimate number of triads results with the density taken for all sections of the length (
) with (
) at the upper limit of the distance, the triads-number-formula (TNF):
(1.8)
The method replacing the local density in the formula by the density at the upper limit of the distance in the formula of the best estimate number of triads is the same, which results the prime-number-formula as low limit of the best estimate number of primes. The fact, that the PNF is well proven, proves the TNF as well since it is evaluated the same way.
The best estimate number of central triads with one component within the first section (
) is equal to the number of free positions within the last section to (2c). It is evaluated with (1.7) as:
(1.9)
The lower limit of the approximating function results—similarly the lower limit of the approximating function results with the density taken at its upper limit (
) for all sections of the length (
), yielding the triads-number-formula-last (TNFL):
(1.10)
The effective number of triads (
) and (
) is evaluated in Annex 3. The dispersion of the effective values of the number of triads around the approximating function (1.9) divided by the number of primes at the square root of the distance (
) results the relative dispersion, evaluated in Annex 4. It is found, that for large distances the standard deviation of the relative dispersion asymptotically approaches a constant value:
;
(1.11)
The constancy of the standard deviation of the relative dispersion of the primes over the distance results from the fact, that the dispersion increases proportionally to the number of primes (
), see Ref. [1] . The same is valid for the reflected union of series of multiples of primes and therefore for the double density of occupation as well. Therefore the relation of the relative dispersion between adjacent primes approaches unity for large distances:
(1.12)
The density of primes decreases with the inverse of the logarithmic of the distance. Herewith the distance between the primes—on the average—increases with the logarithm of the distance. With the standard deviation of the dispersion increasing monotonously to infinity, the largest difference between consecutive primes—the gap—is increases similarly (see Ref. [4] and [5] ).
From the constancy of the standard deviation of the relative dispersion follows the congruency of the relative dispersion of the effective number of primes around their best estimate value (1.7), over the distance.
The above constant factor (1.11) represents an inherent property of the double density of occupation, respectively of the prime numbers.
It is known that at the distance (c) the difference between the effective number of primes (
) and the value resulting from the PNF is proportional to the square of the number of the series of multiples of primes:
;
, which are covering positions at this distance (see Ref. [1] ).
(1.13)
This identity allows to state, that the difference between the effective number of triads and the TNF is greater than the distance divided by the fourth power of the logarithm of the distance, multiplied by a constant (
):
(1.14)
3. The Low Limit of the Number of Triads
Up to now basically the phenomenology of the double density of occupation by the union of series of multiples of primes was analyzed. Now the consequences will be summarized. First the following lemmas may be stated:
Lemma 2.1:
The difference between the best estimate value of the number of triads and the value of the TNF—over large distances—is always greater than the local value of the dispersion of the effective number of triads around the best estimate value: The TNF represents the low limit of the effective number of triads.
Proof: The difference between the value of the best estimate of the effective number of triads and the value of the (TNF) is with (1.14) proportional to (
). The standard deviation and therefore the width of the dispersion of the number of triads is growing proportionally to the number of the series of multiples (
), which are covering integer positions at the distance (c).
For any sufficiently large distance (
) the difference between the value of the effective number of triads and the value of the (TNF) outgrows the width of the dispersion (
), for any value of the constant (
):
;
From this follows, that the effective number of central triads has a lower limit function, the TNF, which is growing to infinity, as stated in the lemma, and concluding the proof.
Lemma 2.2:
The number of free positions left by the double density of occupation by the series of multiples of primes, within each of the first and last sections of the length (
) up to (2c), is proportional to the number of the series of multiples of primes (
), which cover positions at (c), multiplied by the square of the density of primes at the distance (c). It is growing without limit with the distance.
Proof: The triads-number-formula-last TNFL (1.10)gives the low limit of the approximation of the number of triads at (c), evaluated up to (
) and equal to the same number evaluated over the last section up to (2c):
The prime-number-formula PNF for the distance (
) from the origin is (
). It gives the number of primes within the last section up to (2c) and is growing without limit. Divided by (
) and multiplied by (
), has a low limit function (
), which is growing without limit as stated in the lemma and concluding the proof.
The congruency of the relative dispersion—the dispersion of the number of central triads around its best estimate value, divided by (
)—over the distance is an important property of the set of primes.
The number of central triads up to the distance (c) grows with the distance to infinity, since it has a monotonously and continuously to infinity growing lower limit function.
In case the central element of a triad is not a prime, but any positive even number, then with Lemma 1.1 the number of equidistant primes around it is greater, then with a prime as central element. Thus, the low limit of the number of triads is the low limit of the diads as well and grows to infinity (see Ref. [6] ). With Lemma 2.1 the monotonously growing low limit of the number of the triads proves Goldbach’s conjecture.
Replacing (c) by (c/2) in the triads-number-formula (1.8) allows formulating the following lemma:
Lemma 2.3:
In case of the union of the series of multiples of the first (
) primes the low limit of free positions left within the section of the length (
) following any distance (c) is greater than zero.
Proof: With Lemma 2.1 and 2.2, in case of the double density of occupation, at the distance (c/2), within the last section of the length (
), the low limit of the number of free positions left is equal to:
But this is certainly the case within the section of the same length, at the same distance, in case of the single density of occupation by the straight series of multiples alone, because of the lower density of occupation:
Further, this is certainly the case within the next larger section just below (c) of the size (
) as well.
And the same low limit is valid for the section of the size (
) above (c) as well, because the first free position covered by the series of multiples of the smallest possible prime greater then (
)—equal to (
)—is already greater then (
):
The smallest prime, the series of multiples of which is covering positions following (c) is:
(
), in case (
) and (
) are twin primes.
In this case is:
(
) and the square of this smallest prime is already over (
):
Herewith the low limit of free positions left within the section of the size (
) following (c) is the same, as within the last section of the size (
) just below (c), as stated in the lemma and concluding the proof.
4. Consequences of the Double Density of Occupation
Based on Lemma 2.3 the following law may be formulated:
Prime distance law:
Taking the arbitrary integer (
), then the series of multiples of all primes up to (
) are not able to occupy all integer positions within the interval (
): there remains at least one position free, representing a prime number.
Proof: Within the interval of the length (
) there is one section of the length (
), because:
With Lemma 2.3 within this section there is at least a position left free by the union of the series of multiples of the first (
) primes, with (
, which are covering positions up to (
), as stated in the prime distance law and concluding the proof.
With Lemma 2.3 within each of the sections of the length (
) up to (
) there is a position free from the double coverage of the union of the series of multiples of the first (
) primes. This is valid for the larger distance of the length (
) above (n) too. This fact has far reaching consequences: The set of the series of multiples of all primes up to (
), represents—as limit—a continuum, since the difference between any two consecutive primes within the set, divided by the prime is approaching zero:
;
(3.1)
5. Density of Occupation of Subsets of All Integers by the Union of the Series of Multiples of Primes
There is another interesting consequence of the double density of occupation by the reflected series of multiples of primes: it is the proof of the infinity of the number of diads with any given even number as the distance between their components (see Ref. [6] ).
Definition of subsets of all integers:
With the infinite number of integers (
,
) all integers are part of one of the following infinite subsets:
(4.1)
All integers in the subsets for (
) are divisible either by (
), and/or by (
). Therefore, all primes (
) are members of one of the infinite subsets (
) or (
), having the distance (
) to the members of the set (
).
Members of the subset (
) with one of their neighboring positions equal to a prime compose the infinite subsets of the following subsets:
(4.2)
The section of the above sets defines an infinite subset of the subset (
), with primes at both neighboring positions having the distance between them equal to (
), meaning they are twin primes:
The distance (c) of the center point of the diads to the origin is named in the following the distance of the diads. The distance between the primes composing the diads (N) is named in the following the distance of the components. The distance of the components may be any even number. The only condition is, that the half-distance of the components must be smaller than the distance of the diad (
). In the following it is listed, which values may take the distances of the components (N) of the diads to any given distances of the diads, belonging to one of the subsets (
) corresponding to (4.1).
In case of the point of reflection at the distance (c), corresponding to the subsets (a), the following distances of the diads and of the components of the diads are possible, with the parameters (
):
The union of all half distances of the components (N/2) as well as the distances of the diads (c) listed in (4.3) through (4.10) include all integers. Therefore, the set of distances (N) between the primes composing diads includes all even numbers and grows to infinity with the distance of the center point of the diads (c). Differently expressed the number of diads—at a growing distance, with the corresponding even number as the distance of their components—is unlimited. With (1.8), the low limit of the number of diads—including twin primes—is given by the diads-number-formula and grows to infinity.
;
(4.11)
As an example, for the diads the twin primes are evaluated as a special case of (4.3), with (
). For (
) the density of occupation of twins is:
(4.12)
The approximating function of the total number of twins up to (c), with density in case of the double occupation by the union of the series of multiples of the primes and with the factor of correction gives the twin-number-formula as well:
(4.13)
The evaluation and the comparison with the effective number twins are carried out in Annex 5.
Annexes
All above formula are checked in the following annexes with numeric results. The annexes together compose executable files in MATHCAD from PTC. The syntax corresponds to the MATHCAD syntax. Especially the symbols (
) and (
) stand for rounding up or down a real value to the next larger respectively to the next smaller integer.
General Data, Vectors, and Functions used in the following annexes are given in Annex 1. The set of primes and the listed known formula given in Annex 1 as well. They are used for the checking. Some vectors of the results of the known formula, which are often used, are evaluated and the results are saved. All above formula are checked with numeric examples in the following annexes.
Annex 1: General Data, Vectors, and Functions
The set of primes is read from a file: (
). The number of primes in the set and their numbering are: (
,
)
The number of primes up to (c) is approximated with the prime-number-formula (
). At (c) only the multiples of the primes up to (
) are covering free integral positions (
). The numbers of these primes are given as well:
;
(A0.1)
;
For the evaluation of the number of primes and twins up to the distance (c) the routines (
) resulting the index (n) of the prime, respectively of the twin next to any integer is needed (
) respectively (
). The evaluation starts either at the last evaluated index (
), or at the index resulting from the prime-number-formula, respectively twin-number-formula (4.15).
This, to shorten some of the evaluation processes. Further functions are the formula evaluating the index of the next smaller prime to any distance and to the square of any distance:
 ;
;
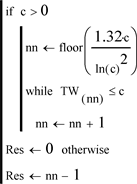 (A0.2)
(A0.2)
;
In the following all functions, which are listed above, are evaluated at sparse distances for illustration, equal to multiples of the square root of the largest distance considered (
), resp. at the next smaller prime
(
):
;
;
(A0.3)
;
;
;
The values are evaluated once at sparse distances and written to a file. They are read from this file:
;
Annex 2: Evaluation of the Constant of the Density of Diads
The constant in (1.4) is evaluated the following way: The factor (
) respectively (
) expressed from (1.4), the relation below quickly converges to a constant value as limit, resulting the factor of correction. Its graphical representation for the sparse values (
) is shown below:
(A1.1)
;
;
;
Annex 3: The Effective Number of Central Triads and of Their Approximation
The approximate values of the number of triads (1.8) are evaluated once for sparse distances:
(
), with the center of the triads being equal to the next smaller prime to the sparse distances. The results are written to a file and are read from this file:
;
(A2.1)
;
Similarly, the total number of triads around the center point at (c) with components within the last section before (2c) is given by (1.9). It is evaluated for sparse distances with the center of the triads being equal to the next smaller prime to the sparse distances. The results are written to a file and are read from this file:
;
; (A2.2)
The routine evaluating the effective number of triads at (c) checks for each prime (
), if the integer at de distance (
) were a prime too. If it is a prime, then the sum of the diads around the central point is risen by one. The checking is made by control of the equality of the prime next to the distance (d) to the distance itself. Similarly, the diads within the last section are evaluated. For the evaluation of the index of the next smaller prime to a given distance the routine (A0.2) is used. The results are written to files and are read from these files:
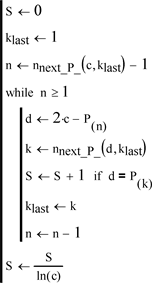 ;
;

;
(A2.3)
;
;
Annex 4: Evaluation of the Properties of the Dispersion of Triads and of Diads
The dispersion of both, of the free positions left by both, by the straight and by the reflected series of multiples of primes are proportional to the number of primes (
), the series of multiples of primes, which are covering free positions at (c). Therefore, this is the case by the dispersion of the number of triads around its best estimate approximation as well. In case of the number of diads within the last section the width of the dispersion is proportional to the number of primes up to the square root of the distance.
The relative dispersion with reference to (
) respectively (
) are evaluated and shown in the figure below. The dispersion is equal in both cases, as expected Figure A1:
;
(A3.1)
;
The estimated width of the dispersion of the triads is:
;
(A3.2)
The standard deviation SD of the dispersion (A3.1)—relative to (
)—must be constant for large distances. It is evaluated below:
;
(A3.3)
The factor of proportionality for the standard deviation of the triads and of the triads in the last section are constants and about equal:
(A3.4)
Annex 5: The Effective Number of Twin Primes and Its Dispersion
The evaluation procedure of the best estimate number of twin primes corresponding to (4.13) is given below. The routine returns the best estimate value:
![]()
Figure A1. The relative dispersion of the number of triads and of triads with their above components in the last section, around their best estimate approximation.
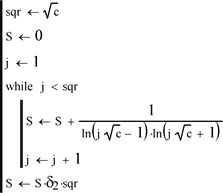 (A4.1)
(A4.1)
The approximate number of twins at sparse distances is evaluated once and the results are written to a file, and they are read from it. The vector of the effective number of twin primes (
) is evaluated elsewhere and the results are written to a file. They are read from this file:
; (A4.2)
;
To evaluate the variables at sparse distances, first the indexes of the next smaller twins to the sparse distances are evaluated and written to a file for later reading:
(A4.3)
;
The difference between the effective number of twins and their approximating function is:
;
(A4.4)
;
The relative dispersion around the approximating function (
) and around the number of primes (
)—starting with (
)—are illustrated in the figures below. The start value is chosen, in order to avoid the larger initial deviation in case of smaller distances.
(A4.5)
The standard deviation of the relative dispersion of the number of twin primes around its approximating function and around the number of primes (
) are in the range (
):
(A4.6)
The factor of proportionality for the standard deviation of the twin primes is approaching a constant value Figure A2:
(A4.7)
The standard deviation of the dispersion of the number of twins is therefore about 0.02% of the value of the approximating function.
Conjecture:
Reflecting the union of the series of multiples of the primes additionally over the ails of triads define five equidistant primes, pentides. Continuing this way, septides and so on may be defined. The number of all these multiple equidistant primes is unlimited.
![]()
Figure A2. Dispersion of the effective number of twins around its approximating function and its limiting boundaries at sparse distances and its standard deviation.