Hyperbolic Monge-Ampère Equation

Fang Gao

School of Mathematical Sciences, Liaocheng University, Liaocheng, China.

DOI: 10.4236/jamp.2020.812220                

Abstract

In this paper, based on the Lie symmetry method, the symmetry group of a hyperbolic Monge-Ampère equation is obtained first, then the one-dimensional optimal system of the obtained symmetries is given, and finally the group-invariant solutions are investigated.

Keywords

Hyperbolic Monge-Ampère Equation, Lie Symmetry, One-Dimensional Optimal System, Group-Invariant Solutions

1. Introduction

In the 19th century, in order to study differential equations, Lie proposed Lie group theory. Due to the relatively abstract Lie group theory, it was not widely used until the 1970s. It was not until Bluman Cole wrote an intuitive and understandable book [1] in 1974 that Lie group theory became widely used to study and solve nonlinear partial differential equations [2] [3] [4] [5]. The basic idea of Lie group method is to simplify or solve partial differential equations by constructing group invariances as the basis of function transformation.

Gao and Zhang studied a new class of dissipative hyperbolic geometric flows. By applying the Lie group method, the optimal system is obtained, and then the equation is similarly reduced and the exact solution is obtained [6]. They primarily study Lie symmetry analysis and exact solutions for the coupled integrable no dispersion equations, and gave the exact solution in the form of power series [7]. Then, by applying the classical symmetry method, Gao obtained the group invariant solution, the optimal system and the exact solution of the evolution equation of a hyperbolic curve flow [8]. Gao also discussed the normal hyperbolic mean curvature flow with dissipation, and obtained the symmetric optimal system and exact solutions by applying Lie symmetry method [9]. Ding and Wang considered symmetry group and invariant solutions of one dimensional hyperbolic inverse mean curvature flow [10].

In [11], during studying the life-span of classical solutions of hyperbolic inverse mean curvature flow, Wang deduced a hyperbolic equation with Riemann invariance that can be reduced to a hyperbolic Monge-Ampè re equation, namely

$u_{tt}{u}_{xx}-u_{xt}^2=-{\left(1+u_x^2\right)}^2.$ (1.1)

In this paper, firstly, we investigate the symmetry group of Equation (1.1). Secondly, we discuss a one-dimensional optimal system of the obtained symmetries. Thirdly, we obtain group-invariant solutions. Finally, we draw conclusions.

2. Symmetry Group

Suppose the one-parameter group of infinitesimal transformations $\left(x,t,u\right)$ is given by

$\begin{array}{l}{x}^{*}=x+\epsilon \xi \left(x,t,u\right)+o\left({\epsilon }^2\right),\\ t^{*}=t+\epsilon \eta \left(x,t,u\right)+o\left({\epsilon }^2\right),\\ u^{*}=u+\epsilon \tau \left(x,t,u\right)+o\left({\epsilon }^2\right),\end{array}$ (2.1)

in which $\epsilon$ is a group parameter.

Let the symmetric group of Equation (1.1) be generated by the vector field in the following form:

$V=\xi \left(x,t,u\right)\frac{\partial }{\partial x}+\eta \left(x,t,u\right)\frac{\partial }{\partial t}+\tau \left(x,t,u\right)\frac{\partial }{\partial u}.$ (2.2)

The first and second-order prolongation of V are respectively:

$p{r}^{\left(1\right)}\left(V\right)=\xi \frac{\partial }{\partial x}+\eta \frac{\partial }{\partial t}+\tau \frac{\partial }{\partial u}+{\tau }^x\frac{\partial }{\partial u_x}+{\tau }^t\frac{\partial }{\partial u_t},$

$p{r}^{\left(2\right)}\left(V\right)=p{r}^{\left(1\right)}\left(V\right)+{\tau }^{xx}\frac{\partial }{\partial u_{xx}}+{\tau }^{xt}\frac{\partial }{\partial u_{xt}}+{\tau }^{tt}\frac{\partial }{\partial u_{tt}}.$

The necessary and sufficient condition for Equation (1.1) to remain unchanged under an infinitesimal transformation is that the vector field should satisfy the invariance conditions of Lie symmetry:

${p{r}^{\left(2\right)}\left(V\right)\left(\Delta \right)|}_{\Delta =0}=0,$ (2.3)

in which $\Delta =u_{tt}{u}_{xx}-u_{xt}^2+{\left(1+u_x^2\right)}^2$, namely

$4{\tau }^x\left(1+u_x^2\right)u_x+{\tau }^{xx}{u}_{tt}+{\tau }^{tt}{u}_{xx}-2{\tau }^{xt}{u}_{xt}=0,$ (2.4)

in which

$\{\begin{array}{l}{\tau }^x=D_x\left(\tau -\xi u_x-\eta u_t\right)+\xi u_{xx}+\eta u_{xt},\\ {\tau }^{xx}=D_{xx}\left(\tau -\xi u_x-\eta u_t\right)+\xi u_{xxx}+\eta u_{xxt},\\ {\tau }^{tt}=D_{tt}\left(\tau -\xi u_x-\eta u_t\right)+\xi u_{xtt}+\eta u_{ttt},\\ {\tau }^{xt}=D_{xt}\left(\tau -\xi u_x-\eta u_t\right)+\xi u_{xxt}+\eta u_{xtt},\end{array}$ (2.5)

in which $D_x$ is total differential tox, $D_{xx},D_{tt}$ are respectively double total differential to x, t, $D_{xt}$ is the total differential with respect tot and then with respect tox. By substituting (2.5) into Equation (2.4), the decision equations of the original equation can be solved as follows

$\{\begin{array}{l}{\eta }_t={\eta }_u={\eta }_x=0,\\ {\xi }_{tt}={\xi }_{uu}={\xi }_{xx}=0,\\ {\tau }_u={\xi }_x,{\tau }_x=-{\xi }_u,{\tau }_{tt}=0,\\ {\xi }_{ut}={\xi }_{xt}={\xi }_{xu}=0,\end{array}$

the above equations can be solved as follows:

$\{\begin{array}{l}\xi =c_{1}x+c_{2}t+c_{3}u+c_4,\\ \eta =c_5,\\ \tau =c_{1}u-c_{3}x+c_{6}t+c_7,\end{array}$ (2.6)

in which $c_1,c_2,c_3,c_4,c_5,c_6,c_7$ are all real constants. Substitute (2.6) into (2.2). Hence the associated seven generators for the one-parameter Lie group of infinitesimal transformations are

$\{\begin{array}{l}{V}_1=\frac{\partial }{\partial x},\hfill \\ V_2=\frac{\partial }{\partial t},\hfill \\ V_3=\frac{\partial }{\partial u},\hfill \\ V_4=t\frac{\partial }{\partial x},\hfill \\ V_5=t\frac{\partial }{\partial u},\hfill \\ V_6=x\frac{\partial }{\partial x}+u\frac{\partial }{\partial u},\hfill \\ V_7=u\frac{\partial }{\partial x}-x\frac{\partial }{\partial u}.\hfill \end{array}$ (2.7)

The corresponding one-parameter transformation groups are:

$\{\begin{array}{l}{G}_1:\left(x,t,u\right)\to \left(x+\epsilon ,t,u\right),\hfill \\ G_2:\left(x,t,u\right)\to \left(x,t+\epsilon ,u\right),\hfill \\ G_3:\left(x,t,u\right)\to \left(x,t,u+\epsilon \right),\hfill \\ G_4:\left(x,t,u\right)\to \left(t\epsilon +x,t,u\right),\hfill \\ G_5:\left(x,t,u\right)\to \left(x,t,t\epsilon +u\right),\hfill \\ G_6:\left(x,t,u\right)\to \left(x{\text{e}}^{\epsilon },t,u{\text{e}}^{\epsilon }\right),\hfill \\ G_7:\left(x,t,u\right)\to \left(u\sin \epsilon +x\cos \epsilon ,t,-x\sin \epsilon +u\cos \epsilon \right).\hfill \end{array}$

3. Optimal System

Definition 3.1: If a set ${\left\{v_{\alpha }\right\}}_{\alpha \in n}$ of r-dimensional subalgebras satisfies the following conditions:

1) Any r-dimensional subalgebras are equivalent to some element in set $\left\{v_{\alpha }\right\}$ ;

2) If ${\beta }_1\ne {\beta }_2$, then $v_{{\beta }_1}$ is not equivalent to $v_{{\beta }_2}$, then set ${\left\{v_{\alpha }\right\}}_{\alpha \in n}$ is known as ther-dimensional optimal system.

Theorem 3.1: Generators in (2.7) generate an optimal system S:

$\begin{array}{l}\{V_1,V_2,V_3,V_4,V_5,V_6,V_7,V_7\pm V_2,V_7\pm V_6,V_7\pm V_2\pm V_6,\\ V_6\pm V_2,V_2\pm V_4,V_2\pm V_5,V_5\pm V_1,V_4\pm V_3\}.\end{array}$

Proof: By formula $\left[V_i,V_j\right]=V_i{V}_j-V_j{V}_i$, we get table of Lie brackets (Table 1).

Let’s say any vector

$V=l_1{V}_1+l_2{V}_2+l_3{V}_3+l_4{V}_4+l_5{V}_5+l_6{V}_6+l_7{V}_7,$ (3.1)

in order to set up the linear transformation of $\tilde{l}=\left(l_1,l_2,l_3,l_4,l_5,l_6,l_7\right)$, let’s say

$E_i=c_{ij}^k{l}_j{\partial }_{l_k},i=1,2,3,4,5,6,7,$ (3.2)

in which $c_{ij}^k$ comes from $\left[V_i,V_j\right]=c_{ij}^k{X}_k$. According to (3.2) and table of Lie brackets, $E_1,E_2,E_3,E_4,E_5,E_6,E_7$ can be written in

$\{\begin{array}{l}{E}_1=l_6{\partial }_{l_1}-l_7{\partial }_{l_3},\hfill \\ E_2=l_4{\partial }_{l_1}+l_5{\partial }_{l_3},\hfill \\ E_3=l_7{\partial }_{l_1}+l_6{\partial }_{l_3},\hfill \\ E_4=-l_2{\partial }_{l_1}+l_6{\partial }_{l_4}-l_7{\partial }_{l_5},\hfill \\ E_5=-l_2{\partial }_{l_3}+l_7{\partial }_{l_4}+l_6{\partial }_{l_5},\hfill \\ E_6=-l_1{\partial }_{l_1}-l_3{\partial }_{l_3}-l_4{\partial }_{l_4}-l_5{\partial }_{l_5},\hfill \\ E_7=-l_3{\partial }_{l_1}+l_1{\partial }_{l_3}-l_5{\partial }_{l_4}+l_4{\partial }_{l_5},\hfill \end{array}$

for $E_1,E_2,E_3,E_4,E_5,E_6,E_7$, Lie equation with parameter $a_1,a_2,a_3,a_4,a_5,a_6,a_7$ and initial conditions ${\tilde{l}|}_{a_i=0}=l,i=1,2,3,4,5,6,7$ are as follows

The solution of the above equation consists of the following transformation

Table 1. Lie brackets.

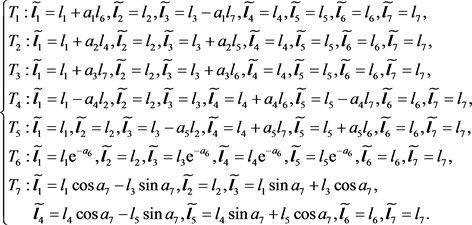
To set up an optimal system, we need to simplify the vector

$\tilde{l}=\left(l_1,l_2,l_3,l_4,l_5,l_6,l_7\right),$ (3.3)

construct the simplest representation of (3.3) by using transforms $T_1~T_7$.

Case 1. When $l_7\ne 0$, Let $a_1=\frac{l_3}{l_7}\left(T_1\right),a_3=-\frac{l_1}{l_7}\left(T_3\right),a_4=\frac{l_5}{l_7}\left(T_4\right),a_5=-\frac{l_4}{l_7}\left(T_5\right)$, make $\widetilde{l_3}=0,\widetilde{l_1}=0,\widetilde{l_5}=0,\widetilde{l_4}=0$, so (3.3) $\iff \left(0,l_2,0,0,0,l_6,l_7\right)$, as a result, we obtain the following representative:

$V~V_7,V_7\pm V_2,V_7\pm V_6,V_7\pm V_2\pm V_6.$

Case 2. When $l_7=0,l_6\ne 0$, Let $a_1=-\frac{l_1}{l_6}\left(T_1\right),a_4=-\frac{l_4}{l_6}\left(T_4\right),a_3=-\frac{l_3}{l_6}\left(T_3\right)$, $a_5=-\frac{l_5}{l_6}\left(T_5\right)$, make $\widetilde{l_1}=0,\widetilde{l_3}=0,\widetilde{l_4}=0,\widetilde{l_5}=0$, so (3.3) $\iff \left(0,l_2,0,0,0,l_6,0\right)$, as a result, we obtain the following representative:

$V~V_6,V_6\pm V_2.$

Case 3. When $l_7=l_6=0,l_2\ne 0$, Let $a_4=\frac{l_1}{l_2}\left(T_4\right),a_5=\frac{l_3}{l_2}\left(T_5\right)$, make $\widetilde{l_1}=0,\widetilde{l_3}=0$, so (3.3) $\iff \left(0,l_2,0,l_4,l_5,0,0\right)$, as a result, we obtain the following representative:

$V~V_2,V_2\pm V_4,V_2\pm V_5.$

Case 4. When $l_7=l_6=l_2=0,l_5\ne 0$, Let $a_2=-\frac{l_3}{l_5}\left(T_2\right)$, make $\widetilde{l_3}=0$, so (3.3) $\iff \left(l_1,0,0,l_4,l_5,0,0\right)$.

(4.1) When $l_4\ne 0$, Let $a_2=-\frac{l_1}{l_4}\left(T_2\right),a_7=\arctan \left(-\frac{l_5}{l_4}\right)\left(T_7\right)$, make $\widetilde{l_1}=0,\widetilde{l_5}=0$, so (3.3) $\iff \left(0,0,0,l_4,0,0,0\right)$ ; as a result, we obtain the following representative:

$V~V_4$.

(4.2) When $l_4=0$, (3.3) $\iff \left(l_1,0,0,0,l_5,0,0\right)$, we obtain the following representative:

$V~V_5,V_5\pm V_1.$

Case 5. When $l_7=l_6=l_2=l_5=0,l_4\ne 0$, (3.3) $\iff \left(l_1,0,l_3,l_4,0,0,0\right)$, Let $a_2=-\frac{l_1}{l_4}\left(T_2\right)$, make $\widetilde{l_1}=0$, so (3.3) $\iff \left(0,0,l_3,l_4,0,0,0\right)$, as a result, we obtain the following representative:

$V~V_4,V_4\pm V_3.$

Case 6. When $l_7=l_6=l_2=l_5=l_4=0,l_3\ne 0$, (3.3) $\iff \left(l_1,0,l_3,0,0,0,0\right)$, Let $a_7=\arctan \left(\frac{l_1}{l_3}\right)(T_7)$, make $\widetilde{l_1}=0$, so (3.3) $\iff \left(0,0,l_3,0,0,0,0\right)$, as a result, we obtain the following representative:

$V~V_3.$

Case 7. When $l_7=l_6=l_2=l_5=l_4=l_3=0,l_1\ne 0$, (3.3) $\iff \left(l_1,0,0,0,0,0,0\right)$, we obtain the following representative:

$V~V_1$.

To sum up, optimal system is

$\begin{array}{l}\{V_1,V_2,V_3,V_4,V_5,V_6,V_7,V_7\pm V_2,V_7\pm V_6,V_7\pm V_2\pm V_6,\\ V_6\pm V_2,V_2\pm V_4,V_2\pm V_5,V_5\pm V_1,V_4\pm V_3\}.\end{array}$

4. Group-Invariant Solutions

In this section, using the optimal system, the reduced equations and exact solutions are analyzed for Equation (1.1).

4.1. $V=V_6=x\frac{\partial }{\partial x}+u\frac{\partial }{\partial u}$

The corresponding characteristic equations are

$\frac{\text{d}x}{x}=\frac{\text{d}t}{0}=\frac{\text{d}u}{u},$

the invariances are

$t,\frac{u}{x}$

the invariant solutions is

$u=xf\left(t\right),$

then Equation (1.1) can be reduced as

${f^{\prime }}^2-2f^2-f^4-1=0.$ (4.1)

By solving the above equation, we can get:

$f\left(t\right)=\frac{f\left(0\right)\pm \tan t}{1\mp f\left(0\right)\tan t},u=x\frac{f\left(0\right)\pm \tan t}{1\mp f\left(0\right)\tan t}.$

When we take $f\left(0\right)=1$, we have

$u_1=x\frac{1+\tan t}{1-\tan t},$

and

$u_2=x\frac{1-\tan t}{1+\tan t}\text{.}$

Figure 1 and Figure 2 depict solutions $u_1$ and $u_2$.

Figure 1. $u_1=x\frac{1+\tan t}{1-\tan t}$.

Figure 2. $u_2=x\frac{1-\tan t}{1+\tan t}$.

4.2. $V=V_7=u\frac{\partial }{\partial x}-x\frac{\partial }{\partial u}$

The corresponding characteristic equations are

$\frac{\text{d}x}{u}=\frac{\text{d}t}{0}=\frac{\text{d}u}{-x},$

the invariances are

$t,x^2+u^2,$

the invariant solution is given by

$u=\sqrt{f\left(t\right)-x^2},$

then Equation (1.1) can be reduced as

$\left(f-3x^2\right){f^{\prime }}^2+\left(6x^{2}f-2f^2-6x^4\right)f^{″}+8x^2+4x^6-12x^{4}f+4=0.$ (4.2)

4.3. $V=V_7+V_2=u\frac{\partial }{\partial x}+\frac{\partial }{\partial t}-x\frac{\partial }{\partial u}$

The corresponding characteristic equations are

$\frac{\text{d}x}{u}=\frac{\text{d}t}{1}=\frac{\text{d}u}{-x},$

the invariance is

$z=x^2+u^2,$

the invariant solution is given by

$u=-xt+f\left(z\right),$

then Equation (1.1) can be reduced as

${\left(2x{f}^{\prime }-t\right)}^4+2{\left(2x{f}^{\prime }-t\right)}^2=0.$ (4.3)

4.4. $V=V_6+V_2=x\frac{\partial }{\partial x}+\frac{\partial }{\partial t}+u\frac{\partial }{\partial u}$

The corresponding characteristic equations are

$\frac{\text{d}x}{x}=\frac{\text{d}t}{1}=\frac{\text{d}u}{u},$

the invariance is

$z=x{\text{e}}^{-t},$

the invariant solution is given by

$u=xf\left(z\right),$

then Equation (1.1) can be reduced as

$-x^3{f}^{\prime }{f}^{″}+6x^2{f^{\prime }}^2{f}^2{\text{e}}^t+4x{f}^3{f}^{\prime }{\text{e}}^{2t}+4x^{3}f{f^{\prime }}^3+4xf{f}^{\prime }{\text{e}}^{2t}+\left(f^4+2f^2\right)=0.$ (4.4)

4.5. $V=V_2+V_4=t\frac{\partial }{\partial x}+\frac{\partial }{\partial t}$

The corresponding characteristic equations are

$\frac{\text{d}x}{t}=\frac{\text{d}t}{1}=\frac{\text{d}u}{0},$

the invariance is

$z=2x-t^2,$

the invariant solution is given by

$u=g\left(z\right),$

then Equation (1.1) can be reduced as

$8{g^{\prime }}^2+16{g^{\prime }}^4-8g^{\prime }{g}^{″}+1=0.$ (4.5)

5. Conclusion

This paper includes four parts: the first part is the introduction, which introduces the background knowledge of the hyperbolic Monge-Ampè re equation and Lie symmetry; in the second part, symmetry group is given; in the third part, optimal system of the symmetry is discussed; in the fourth part, we obtain group-invariant solutions.

Acknowledgements

I would like to thank Z G Wang teacher for drawing my attention to hyperbolic Monge-Ampè re equation. I am grateful to my tutor for her suggestions, which have greatly improved this paper.

Conflicts of Interest

The author declares no conflicts of interest regarding the publication of this paper.

References

[1]	Bluman, G.W. and Cole, J.D. (1974) Similarity Methods for Differential Equations, Springer, Berlin. https://doi.org/10.1007/978-1-4612-6394-4
[2]	Li, Y., Liu, X.Q. and Xin, X.P. (2016) Explicit Solutions, Conservation Laws of the Extended (2 + 1)-Dimensional Jaulent-Miodek Equation. Journal of Hebei Normal University (Natural Science Edition), 40, 376-384.
[3]	Wen, S.Q., Li, C.H. and Mo, D.L. (2014) Lie Symmetries and Exact Solutions for the Vakhnenko-Parkes Equation. Journal of Sichuan Normal University (Natural Science), 37, 320-324.
[4]	Zhang, L.H., Liu, X.Q. and Cheng, L. (2007) BAI. Symmetry Groups and New Exact Solutions to (2+1)-Dimensional Variable Coefficient Canonical Generalized KP Equation. Communications in Theoretical Physics, 48, 405. https://doi.org/10.1088/0253-6102/48/3/004
[5]	Kumar, S., Singh, K. and Gupta, R.K. (2012) Painleve Analysis, Lie Symmetries and Exact Solutions for (2+1)-Dimensional Variable Coefficients Broer-Kaup Equations. Communications in Nonlinear Science and Numerical Simulation, 17, 1529-1541. https://doi.org/10.1016/j.cnsns.2011.09.003
[6]	Zhang, Y. and Gao, B. (2019) Lie Symmetry Analysis for the Coupled Integrable Dispersionless Equations. Pramana, 93, 1-11. https://doi.org/10.1007/s12043-019-1857-5
[7]	Gao, B. and Zhang, Y. (2019) Symmetry Analysis, Exact Solution and Power Series Solutions of Dissipative Hyperbolic Geometry Flow. Advances in Differential Equations and Control Processes, 20, 17-36. https://doi.org/10.17654/DE020010017
[8]	Gao, B. and Zhang, S. (2020) Analysis of the Evolution Equation of a Hyperbolic Curve Flow via Lie Symmetry Method. Pramana, 94, 55. https://doi.org/10.1007/s12043-020-1920-2
[9]	Gao, B. and Zhang, S. (2020) Invariant Solutions of the Normal Hyperbolic Mean Curvature Flow with Dissipation. Archiv der Mathematik, 114, 227-239. https://doi.org/10.1007/s00013-019-01397-4
[10]	Ding, R. and Wang, Z.G. (2019) Symmetry Group and Invariant Solutions of One Dimensional Hyperbolic Inverse Mean Curvature Flow. Journal of Liaocheng University (Natural Science Edition), 32, 6-11.
[11]	Wang, Z.G. (2020) Life-Span of Classical Solutions to Hyperbolic Inverse Mean Curvature Flow. Discrete Dynamics in Nature and Society, 2012, 1-12. https://doi.org/10.1155/2020/6905269