Alternative Approach to Time Evaluation of Schrodinger Wave Functions ()
1. Introduction
Quantum theory is undoubtedly one of the most successful theories within the scientific history and opens the doors to countless technological applications through describing, correlating and predicting the behavior of vast range of physical systems made up of molecules, atoms, nuclei and other elementary particles. No experimental evidence has been detected so far opposing the predictions of the theory flashing the extraordinary success [1-6]. In spite of its exceptional success, the theory surprisingly faces certain paradoxes and conceptual difficulties such as Einstein, Podolsky, Rosen (EPR) Paradox/Quantum Entanglement [7-9], Quantum Decoherence/Quantum Measurement Problem [10-12] and Quantum Zeno Effect [13,14], all question the theory philosophically from the spine and could not be fully resolved yet. In spite of nearly 100 years, famous discussions, primarily between Einstein and Bohr, have unfortunately not been completely terminated and some numerable quantum physicists still feel some disturbances about the probabilistic nature of the atomic world and of course about the conceptual composition of quantum theory [15-17]. A number of conceptual and philosophical interpretations, of the quantum theory, have been developed in the past and most of them experience some support but more noticeably intelligent criticism. Philosophically speaking, quantum theory barely probes its tools to explore the atomic order/world however believing it as the only possible theory/method would surely be dumpy mentality and alternative approaches/means could unquestionably be developed by other civilizations.
The complicatedness of the theory mostly originates from the unsatisfactory definition of the most fundamental concepts, namely “time” and “position”. Consequently, the paradoxes and conceptual difficulties of the quantum theory, to our view, regularly initiate from the lack of a clear scientific definition between “time” and “position”. Quantum theory basically assumes that “time” and “space” are continuous and completely independent variables and no scientific relation between time and position can be defined no matter if the particle is free or confined. The theory also assumes that quantum particles are solid spheres, however the particles are also somehow accompanied by physical waves. Hence, the existence of spatially and temporally distributed matter waves prevents to define position and time dependence of dynamical variables such as velocity, momentum and energy, in unfriendliness to classical physics. Instead, well defined time and position dependant waves and depending on the wave functions particle’s the existence probability is smartly defined. Quantum theory, in general, describes two distinct physical concepts, namely dynamical variables/operators and wave functions/state vectors. Concerning the time evolution of a quantum system, two distinct approaches simply exist, namely Schrödinger and Heisenberg models/pictures [18,19]. Schrödinger Model (SM) assumes that the wave functions/state vectors are time dependant but the dynamical variables/operators are constant and the model offers an equation, TDSWE, for the time evolution of the state vectors/wave functions. Heisenberg Model (HM) is, on the other hand, based on that the wave functions/state vectors are steady whereas the operators/dynamical variables are time dependant and the model offers the well known Heisenberg Equation (HE). Independently from those pictures, ontological and epistemological approaches to the subject forwards that “a quantum particle must exist at a specific point at a particular time” therefore the position of the particle could, somehow, be definable. Additionally, the very existence of any quantum particle in space and time must ontologically be conserved; hence both state vectors and operators should be, in the most general case, considered time dependant. In order to obtain a full picture of the atomic world, means precise position at a given time, both time and position dependence of the operators ought to be defined. To our view, Einstein was/is right and it is the insufficiency of the theory and the theory ought to be progressed substantially to resolve the certain paradoxes and conceptual difficulties [15]. Philosophic and scientific discussion of this most fundamental theme is not the scope of the present paper, however will be handled in an upcoming paper.
In addition to those paradoxes and conceptual difficulties, there are some other fundamental insufficiencies and difficulties within the quantum theory. Quantum systems may have obviously been both “closed” and “open” in character and the problem must be treated accordingly [20,21]. The physical properties of any quantum particle for open quantum systems are much more complicated due to having strongly time and position dependant energies; potential, kinetic or overall mechanical energy. The exchange energy, of the most quantum systems, seems to be negligible compared to the actual system energy maintaining the assumption of being a closed system is highly fulfilled. Open quantum systems, however, demonstrate very high exchange energies between the actual system and the environment leading to very important applications in quantum optics, measurement in quantum mechanics, quantum statistical mechanics, quantum information, quantum cosmology, some semi classical applications and recently the ultimate concept of human consciousness [22-27]. Bohr emphasized that the border between classical and quantum worlds must be mobile so that even the ultimate apparatus the “Human Consciousness” could in principle be measured and analyzed as a quantum object, provided that a suitable classical device could be found to carry out the task. The problem of open quantum systems is traditionally resolved by means of non-Markovian Stochastic Schrödinger Equation. The approach basically considers that the present state of any quantum system is random but linked with the past and future states but can be predicted. Specific applications of the stochastic equation are known as von Neumann Equation (NE) or Quantum Liouville Equation (QLE) and Lindblad Equation (LE). Both NE/QLE and LE describe the time evolution of a mixed quantum state by means of the density matrix/density operator that is defined as the sum of all the possible probability densities [28-30].
The approaches above are all based on the position dependant Hamiltonian operator which is defined in terms of position/space dependant momentum operator leading to a kinetic energy operator purely depends on position/space. However, open quantum systems surely consist of time dependant kinetic and potential energies. Therefore especially as in Hamilton picture, time dependant momentum operator seems to be quite important and missing. Definition of time dependant momentum operator is only possible if time dependant position is actually definable. Description of time position relation could undoubtedly influence the quantum theory and philosophy in the sense that as deterministic view strengthens, probabilistic view would weaken. The approach is scientifically very simple but philosophically complicated and would possibly be bridging the existing gap between the theory and certain paradoxes and conceptual difficulties of the quantum theory. The present work is based on this simple however vital estimation of realistic time dependant momentum operator. The work initially defines a realistic time dependant alternative momentum operator and Hamiltonian operator, leading to an ATDSWE which can be employed from the simplest to the most complicated quantum systems.
The other motivation of the present work arises from the fact that no counterpart of the time dependant classical wave equation exists within the quantum theory. Waves accompanying the quantum particles are, naturally, matching with the classical counterparts. It is very well known that, space dependant classical wave equation is matched by the time independent and position dependant Schrödinger wave equation. The other part of the classical wave equation, namely time dependant part, is simply missing. Nevertheless, time dependant classical wave equation should naturally exist within the quantum world. The present work is also based on this most obvious mental picture.
2. Method
Schrödinger/matter waves, in quantum theory, are characteristically very similar to the classical waves and space or time dependence of the waves could in fact be described by the equations identical to the classical waves [3-5]. Evidently, the position dependant SWE is just identical to the space dependent classical wave equation. Time dependant classical wave equation, on the other hand, has no counterpart in quantum mechanics; instead a first order differential equation, known as TDSWE, exists in the theory. Hence, the present work proposes to link this fundamental gap and offers the quantum mechanical counterpart of the time dependant classical wave equation. The work is in fact an extension of the “existing quantum theory” and following some philosophical arguments the ATDSWE is derived in a simplest possible form [19].
3. Theory
The physical concepts/dynamical variables, in quantum theory, are represented by mathematical operators therefore any quantum physical concept/dynamical variable transforms the wave function to a different one. The quantum operators are typically defined in terms of time or position derivatives and must be linear and hermitical to represent the physical world. Quantum theory purely relies on the two distinct concepts namely state vectors/ wave functions and physical concepts/dynamical variables [6,19]. Therefore, spatial and temporal variation of the wave functions and dynamical variables are very crucial to be determined. Time evaluation of quantum mechanical concepts, namely dynamical variables and wave functions, is a fundamental issue and being handled differently in the sense that Heisenberg Picture considers that operators are time dependant whereas the wave functions/state vectors are constant; alternatively Schrödinger Picture assumes wave functions/state vectors being time dependant but the operators are stationary [6,19]. Heisenberg Equation (HE) simply gives the time evaluation of a quantum mechanical operator and the approach is simply based on the instantaneous operator terms which means that time dependence of any quantum mechanical operator could be defined. QLE or NE, on the other hand, describes time evaluation of the density matrix/operator which is the overall wave function of the quantum system. The equations are very similar in the sense that both derived from the TDSWE and both depend on the commutation relation between the density matrix and the Hamiltonian operator.
In order to derive the ATDSWE, we start with the simplest text book expression of momentum operator that can simply be extracted by taking the derivative of classical plane waves with respect to the position r, that is
 (1)
(1)
where i denotes the imaginary unit of the complex numbers,  is the reduced Planck’s constant and r denotes the three dimensional position of the particle. It is very crucial to note that the position here is considered as a “continuous variable” and the momentum operator is purely defined as a function of position, means that momentum of the quantum particle is solely position dependant. Quantum theory also assumes that “time” is as continuous and independent variable. Ontological and epistemological approach to the problem clearly indicates that a mathematical relation between position and time could be described since the quantum particle continuously exists in space and time. This philosophical approach straight forwardly leads to a definition of the momentum operator depend purely on “time”. Employing the textbook chain rule for the partial derivations gives the realistic time dependant momentum operator stated as
is the reduced Planck’s constant and r denotes the three dimensional position of the particle. It is very crucial to note that the position here is considered as a “continuous variable” and the momentum operator is purely defined as a function of position, means that momentum of the quantum particle is solely position dependant. Quantum theory also assumes that “time” is as continuous and independent variable. Ontological and epistemological approach to the problem clearly indicates that a mathematical relation between position and time could be described since the quantum particle continuously exists in space and time. This philosophical approach straight forwardly leads to a definition of the momentum operator depend purely on “time”. Employing the textbook chain rule for the partial derivations gives the realistic time dependant momentum operator stated as
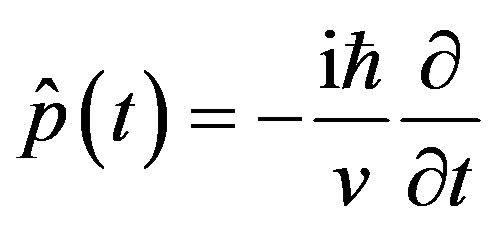 (2)
(2)
where v denotes the time dependant instant velocity of the quantum particle defined by the HE of the quantum theory. In the expression above commutation property of the operators of ¶/¶t and ¶/¶x is simply used. The definition above assumes that the “ontological approach” must be satisfied since the particle, as a physical object, is bound to be existing somewhere at an instant time. The approach above also expresses that time depended position, r(t), is definable and can be defined in principle. The existing quantum theory offers no time dependant position and this would certainly be leading to a more deterministic theory of the atomic world. This is not covered in the present work but will be tried to address in the future studies. The quantum particle, at this point, can be treated as a continuously moving object with well defined potential and kinetic energies that are directly time dependant. One can now reach to an alternative time dependant Hamiltonian operator, significantly different from the traditional time dependant Hamiltonian that can be affirmed as
 (3)
(3)
where v(t) denotes time dependant instantaneous velocity, m denotes the particle mass, and V(t) is the time dependant potential energy of the particle. The expression above can commonly be employed for the quantum particles having a potential energy or overall mechanical energy directly and heavily depending on time which is generally the case for the open quantum systems. Time dependant Hamiltonian operator can now be employed in any involving equation, namely QLE/NE or LE to obtain time evaluation of any quantum system. The purpose of this paper is, however, to derive an alternative and simplest feasible wave equation to analyze the motion of a quantum particle. In order to do so, the well known eigen value-eigen function equation is employed as usual to acquire the ATDSWE for the most general case, that is
 (4)
(4)
where E(t) denotes total mechanical energy which is considered to be time dependant and corresponding wave function is of course only time dependant. The most general case is the open quantum systems and substitution of the Hamiltonian expression above and also using the classical definition of kinetic energy simply gives the ATDSWE as,
 (5)
(5)
This basic equation is identical to the time dependant part of the classical wave equation by simply considering the angular frequency as,
 (6)
(6)
The angular frequency in this case is defined in general for the open quantum systems and can easily be reduced to the closed quantum systems. The main problem at this stage is of course to determine the time dependant kinetic energy of the quantum particle or alternatively time dependant potential and total mechanical energies of the quantum particle. Using the basic expression of kinetic energy,  , leads to the result that, the quantum particles are accompanied by the Schrö- dinger/matter waves with frequencies totally depends only on the kinetic energy. This is evident that the wave frequency instantaneously varies as the kinetic energy changes as being the common property of the open quantum systems.
, leads to the result that, the quantum particles are accompanied by the Schrö- dinger/matter waves with frequencies totally depends only on the kinetic energy. This is evident that the wave frequency instantaneously varies as the kinetic energy changes as being the common property of the open quantum systems.
4. Results and Discussion
The present work primarily based on the view that both Schrödinger wave functions/state vectors and dynamical variables/operators may simultaneously vary for any quantum mechanical particle. The view in a way combines the views of Schrödinger and Heisenberg and offers an alternative momentum operator, the Equation (2), depends on time not position. The momentum expression is simply offered by considering ontologically and epistemologically realistic approach, rather than conventional Heisenberg or Schrödinger approaches, assuming that the quantum particle should be present at a definable position at a definite instant. The approach partially considers the Heisenberg model but further the state vectors/wave functions are considered to be varying. The approach is significant and should lead to a new pathway for the interpretation of the quantum mechanics in the sense that the probabilistic view would, to some extent, switch to a deterministic view.
The ATDSWE, the Equation (5), is valid for the most general cases only excluding the relativistic region however including open quantum systems in which all the energies and state vectors/wave functions are highly time dependant. The equation is important in the sense that it is the quantum mechanical counterpart of the time dependant classical wave equation. The equation basically expresses that the frequency of the waves accompanying the quantum particle varies continuously and instantly as a function of the kinetic energy. Quantum particles, basically having stationary kinetic energies, are accompanied by stationary Schrödinger waves with constant frequencies. The simplest possible case, to apply the Equation (5), is the free particle case having no potential energies and also having uniform overall mechanical energies. First of all, the relation between overall mechanical energy and the angular frequency, concerning the free particle case, is given by
 (7)
(7)
where E denotes the total mechanical energy and also equals to the kinetic energy for the free particles, w denotes the angular frequency. This energy expression is well known as the “zero point energy” and just equal to the minimum energy given by the position dependant SWE solved for the harmonic oscillator problem. This is very interesting in many ways but especially ought to be expected in the sense that “zero point energy” is jut universal and independent of both time and space. Therefore, derived ATDSWE passes a solid test by this simple but very important comparison [4,19].
General solution, concerning the free particle case, of the offered ATDSWE is straightforward and is simply given by
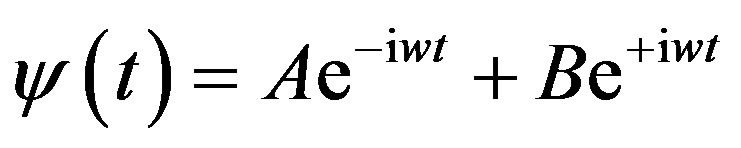 (8)
(8)
where w denotes the angular frequency, A and B are constants determined by the physical boundary conditions in the backward and forward propagations, respectively. It is very interesting to note that the general term has both forward and backward time propagation terms, exclusively different from the free particle solution of the ordinary TDSWE that is given by. Quantum systems/ particles are, in most cases, naturally confined in space but confinement in terms of time does not normally exist. Therefore boundary conditions for the time evolution of the quantum systems/particles is quite different compared to the spatial conditions. Spatial confinement of any quantum particle is just fulfilled naturally however temporal confinement is not possible. Philosophically speaking creation of any quantum system or particle is not possible to set the initial conditions. Instead, the quantum systems and particles just exist independently from time and no temporal initial conditions can normally be set. Physical boundaries in terms of time propagation in the forward and backwards then A and B can be considered to be equal then the solution can be given by
 (9)
(9)
where y0 denotes the initial wave function at the time beginning. Immediate comparison indicates missing imaginary part for the ATDSWE solution. The existence of forward and backward time propagation and the superposition of those terms lead to a real time dependant waves. This, in many ways, may lead to very important impacts on the interpretation of the quantum theory. The most widely accepted Copenhagen Interpretation of the wave functions/state vectors is employed to compare the finding probability of the quantum particles. The free particle wave functions/state vectors simply give the probability density, that is finding the quantum particle in a unit time at the instant t, as
 (10)
(10)
which is obviously time dependant. Ordinary TDSWE, on the other hand, gives a probability density,  , that is of course time independent. ATDSWE offers a probability density being time dependant however as time goes to zero, the probability density equals to the result of the ordinary TDSWE. The result would seem to be conflicting with the present quantum theory and experiments; however this view would not be so satisfying since how fast the probability density varies with time is not known.
, that is of course time independent. ATDSWE offers a probability density being time dependant however as time goes to zero, the probability density equals to the result of the ordinary TDSWE. The result would seem to be conflicting with the present quantum theory and experiments; however this view would not be so satisfying since how fast the probability density varies with time is not known.
The ATDSWE includes the velocity term, finally converted to the kinetic energy term, which to our opinion is very important aspect. The existing quantum theory cannot possibly estimate the exact position or velocity at a certain time, instead it gives the finding probabilities for the quantum particle. Having classical definition of velocity in the ATDSWE might be important and might lead to open the door to a further and even better theory of atomic world.
5. Conclusions
The first conclusion is the definition of a “momentum operator” in terms of time derivative; hence the operator simply describes time evolution of momentum for a quantum particle. The pictures of Schrödinger and Heisenberg, without any doubt, analyze the counterintuitive structure of the quantum theory, however more realistic picture ought to consider simultaneous variation of both dynamical variables/operators and state vectors/ wave functions with respect to time and space. Restricting the variation of them, as in the HM and SM, is not philosophically realistic. The momentum operator is simply based on the “time dependant position operator” for the quantum particles and in fact would initiate deep philosophic discussions. Time dependant momentum operator specifically expresses that instantaneous position of any quantum particle could, in fact, be predictable. This conclusion, on its own, is revolutionary and supports Einstein in the sense that a novel approaches could be developed to move the quantum theory from purely probabilistic to more deterministic.
The second important conclusion is the suggested so called ATDSWE. Fundamental equation of ATDSWE, which is the counterpart of the classical time dependant wave equation of classical mechanics, is simply derived by means of the time dependant momentum operator. The equation only requires time dependant kinetic energy or alternatively time dependant both potential and overall mechanical energies to estimate the time evolution of the Schrödinger wave functions. The equation can further be expanded to obtain the time evolution of the density matrix, as in the cases of NE/QLE or LE.
The ATDSWE also expresses the basic relation between kinetic energy, or alternatively potential and total mechanical energies, and angular frequency of the Schrödinger waves accompanying the quantum particles. The time evolution of the frequency is directly proportional to the actual time dependant kinetic energy of the quantum particles. It is also important to note that time dependant kinetic energy for the quantum particles is simply equal to the “zero point energy”, would surely ignite many philosophical and scientific discussions in the future.
6. Acknowledgements
The author wishes to thank to Abdullah VERCIN from Ankara University and to Altuğ OZPINECI from Middle East Technical University for some beneficial discussions.