A With-In Host Dengue Infection Model with Immune Response and Beddington-DeAngelis Incidence Rate ()
1. Introduction
Dengue is an infections mosquito-borne viral disease. It is estimated that about 50 million infections occur annually in over 100 countries [1]. There is no specific treatment for curingdengue patients. Hospital treatment in general is given as supportive care which includes bed rest, antipyretics, and analgesics. Most dengue infections are asymptomatic. Few of them suffer dengue fever and dengue haemorrhagic fever, which may end up in fatality.
Dengue virus is one of the most difficult arboviruses to isolate. There are four serotypes of the dengue virus and each of the serotype has numerous virus strains. Infection with one dengue serotype may provide lifelong immunity to that serotype, but there is no cross-protective immunity to other serotype, [2]. Identification of the primary target cells of dengue virus replication in infected human body has proven to be extremely difficult. It is generally believed that the target cells of dengue virus are monocytes or its differentiated cells the macrophages [3].
It is usually believed that dengue virus is quickly cleared in human body within approximately 7 days after the day of sudden onset of fever [1]. Naturally this clearing process is done by the immune system which is a result of complex dynamic reactions. Following [4], in this paper we try to understand the process using a mathematical model.
Mathematical modeling of dengue disease transmission in human and mosquito populations has been done since the beginning of last century. Some of the recent models could be seen in [2-5]. Several studies on infection model within human body have been done for various cases [2,3] and [5-11]. Meanwhile, mathematical modeling for with-in host dengue viral disease is quite new.
The model for with-in host dengue viral infection with Beddington-DeAngelis incidence rate and immune response is as following.
 (1)
(1)
where,  and
and .
.
The constant , is the rate constant characterizing infection of the cells. The constants
, is the rate constant characterizing infection of the cells. The constants  are positive.
are positive.
In the above  and
and  represent the density of susceptible monocytes, infected monocytes, free virus particles and immune cells in 1 μl blood at time t, respective. The production of susceptible monocytes by bone marrow is assumed at a constant rate μ and the life span of susceptible monocytes is
represent the density of susceptible monocytes, infected monocytes, free virus particles and immune cells in 1 μl blood at time t, respective. The production of susceptible monocytes by bone marrow is assumed at a constant rate μ and the life span of susceptible monocytes is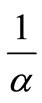 . The flow from susceptible monocytes to the infected monocytes depends on the incidence rate of susceptible monocytes and free virus particle. This rate is shown by
. The flow from susceptible monocytes to the infected monocytes depends on the incidence rate of susceptible monocytes and free virus particle. This rate is shown by  where
where  is the incidence response of susceptible monocytes to free virus particles. The period of infected monocytes is assumed constant as
is the incidence response of susceptible monocytes to free virus particles. The period of infected monocytes is assumed constant as  . We assumed virus multiplication is at constant rate k and the virus clearance rate is at constant rate
. We assumed virus multiplication is at constant rate k and the virus clearance rate is at constant rate . We also assumed the immune cells are produced at constant rate
. We also assumed the immune cells are produced at constant rate 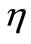 and their life span is
and their life span is . Moreover we assumed there is stimulation of immune cells production due to the increase of infected cell which is proportional to the density infected monocytes at a constant rate c as well as from the contacts with infected cells at the rate d and the immune cells will eliminate the infected monocytes at a constant rate v. Finally, the positive constants
. Moreover we assumed there is stimulation of immune cells production due to the increase of infected cell which is proportional to the density infected monocytes at a constant rate c as well as from the contacts with infected cells at the rate d and the immune cells will eliminate the infected monocytes at a constant rate v. Finally, the positive constants  and
and  have some biological meanings.
have some biological meanings.
The above model is valid for only one serotype of dengue virus circulate in an infected host and dengue infects monocytes in blood stream.
For more detail the reader is referred to [4] and references therein.
The local stability of the equilibrium points of the system (1) for Lotka-Voltera functional response i.e.  , has been discussed in [4]. The model (1) is a generalization of the self-regulating cytotoxic T lymphocytes (CTL) response model. The predator-prey like CTL response model and the linear immune response model in chapter 6 of [5].
, has been discussed in [4]. The model (1) is a generalization of the self-regulating cytotoxic T lymphocytes (CTL) response model. The predator-prey like CTL response model and the linear immune response model in chapter 6 of [5].
In this paper, we will analyze the global of stability of the viral free equilibrium for Beddington-DeAngelis incidence response,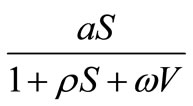 . In fact we will show that if this equilibrium is the only rest point of the system (1), then it is globally asymptotically stable. If there are some other equilibria, then the local stability of them depends on the values of the parameters.
. In fact we will show that if this equilibrium is the only rest point of the system (1), then it is globally asymptotically stable. If there are some other equilibria, then the local stability of them depends on the values of the parameters.
2. Global Stability of the Uninfected Equilibrium
In this section, at first we will find the equilibrium points of the system (1) and the eigenvalues of this system at these points. This information leads us to prove the locally asymptotical stability of the equilibrium points.
At an equilibrium point of the system (1) we must have
 (2)
(2)
From the first equation we obtain, .
.
Substituting this value of V into the third equation yields,  . From the fourth equation we obtain
. From the fourth equation we obtain  . Substituting these values of
. Substituting these values of  and
and  into the second equation yields,
into the second equation yields,

If,  , then from this, we have
, then from this, we have . Thus
. Thus  is one of the equilibrium points of the system (1). If,
is one of the equilibrium points of the system (1). If,

then
 (3)
(3)
where,
 ,
,
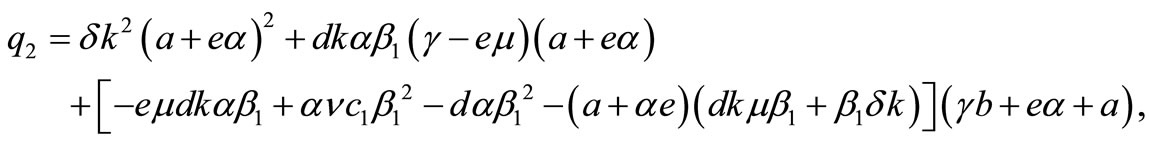
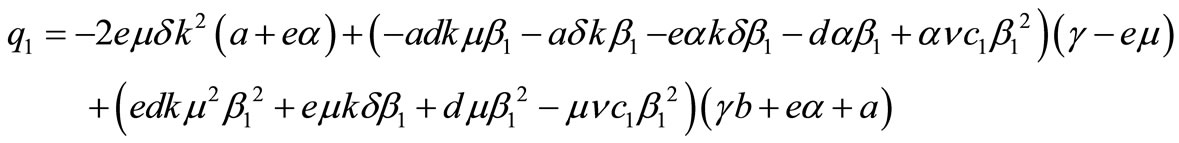
and
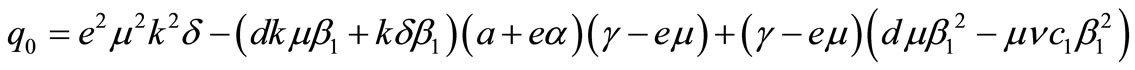
In the following we consider the stability property of the equilibrium point . In order to do this we check the sign of the eigenvalues of Jacobi matrix of (1) at
. In order to do this we check the sign of the eigenvalues of Jacobi matrix of (1) at . The Jacobi matrix is
. The Jacobi matrix is
 (4)
(4)
So the value of  at
at  is
is

The eigenvalues of  are the roots of the characteristic polynomial
are the roots of the characteristic polynomial
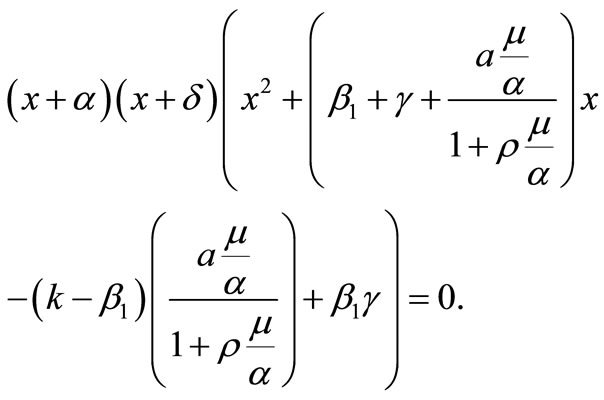
Thus,  and
and  are two of the eigenvalues and the other two are the roots of
are two of the eigenvalues and the other two are the roots of
 .
.
These roots are


where, .
.
Clearly, 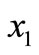 ,
,  and
and have negative real part. If
have negative real part. If  has negative real part, then the equilibrium
has negative real part, then the equilibrium  is locally asymptotically stable. But
is locally asymptotically stable. But 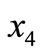 is negative if and only if,
is negative if and only if, . This condition equals to,
. This condition equals to, .
.
Set, . This number is called the basic reproduction ratio [7].
. This number is called the basic reproduction ratio [7].
Therefore we have the following theorem.
Theorem 2.1. If,  , the equilibrium point
, the equilibrium point  is locally asymptotically stable and if
is locally asymptotically stable and if , the equilibrium
, the equilibrium  is unstable.
is unstable.
Now we will show that if,  , then the equilibrium,
, then the equilibrium,  is globally asymptotically stable. In order to see this, first of all consider the following domain in the
is globally asymptotically stable. In order to see this, first of all consider the following domain in the  space.
space.

It follows that the flow generated by that system (1.1) gets into 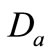 on the boundary of
on the boundary of . Let
. Let  for,
for,
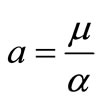 . Thus
. Thus  is a global attractor. Now in
is a global attractor. Now in  consider the following set for
consider the following set for :
:
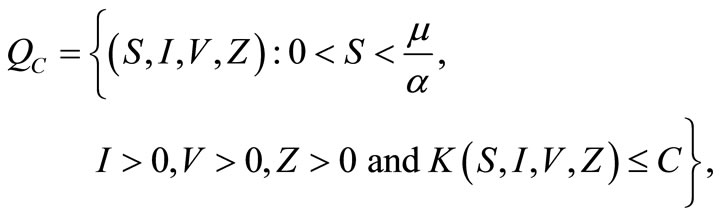
where, 
and



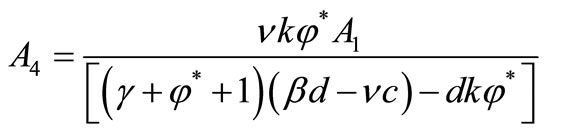
and .
.
If we differentiate  along the orbits of the system (1), we obtain:
along the orbits of the system (1), we obtain:
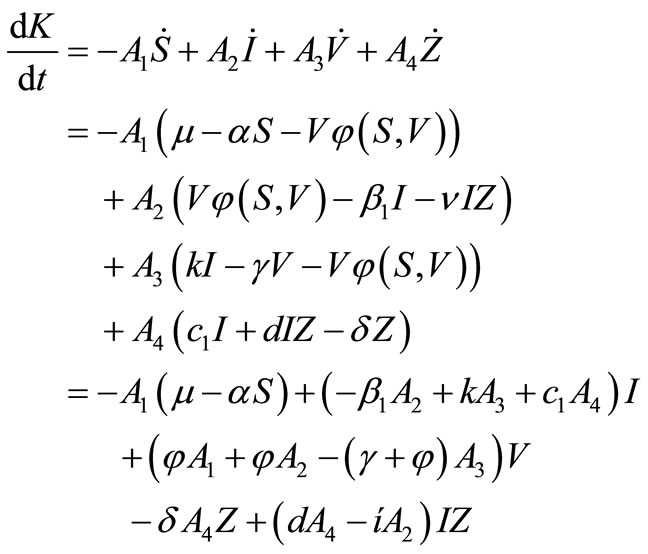
Here, . Since on the surface
. Since on the surface  of the boundary of
of the boundary of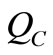 , we have
, we have  and
and 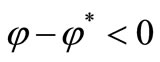 and
and 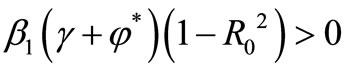 , therefore
, therefore . Thus the flow gets into
. Thus the flow gets into  on,
on, . Hence the flow gets into
. Hence the flow gets into  from its boundary. Therefore
from its boundary. Therefore  is an attractor in D for all
is an attractor in D for all . But
. But . Thus
. Thus  is a global attractor. Thus we have proved the following theorem.
is a global attractor. Thus we have proved the following theorem.
Theorem 2.2. If,  , then
, then  ,the uninfected equilibrium is the only equilibrium of the system (1). Moreover this equilibrium is globally asymptotically stable.
,the uninfected equilibrium is the only equilibrium of the system (1). Moreover this equilibrium is globally asymptotically stable.
Since 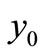 is globally asymptotically stable for
is globally asymptotically stable for , any other equilibrium points of the system (1) cannot exist for
, any other equilibrium points of the system (1) cannot exist for . Therefore,
. Therefore, 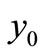 is the unique equilibrium point for
is the unique equilibrium point for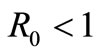 .
.
3. Stability of the Other Equilibrium Points
In this section, we consider the stability of the other rest point of the system (1). In order to this, we consider the Equation (3). First, we consider this equation for  and then for
and then for .
.
There are two cases for  as follows.
as follows.
Case 1. 
In this case, the system (1) has two equilibrium points,  and another one. To see this, from the first equation of (2) we obtain,
and another one. To see this, from the first equation of (2) we obtain, . Since
. Since 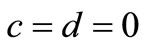
from the fourth equation we get . Substituting these values of
. Substituting these values of 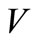 and
and  into the second equation yields,
into the second equation yields, . By using the value of
. By using the value of  and the third equation we get
and the third equation we get . Using these values into the equation (3), we obtain,
. Using these values into the equation (3), we obtain,
 .
.
Therefore, we obtain
 where
where
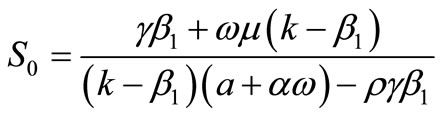 as the second rest point of the system (1).
as the second rest point of the system (1).
Notice that this rest point exists if , or
, or . If
. If , this rest point is the same as
, this rest point is the same as
 . If
. If , then
, then
 or
or
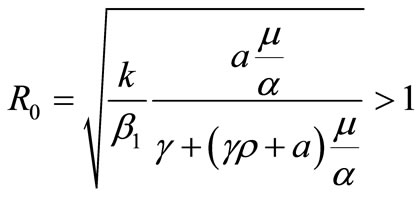 .
.
Now, we consider the local stability of the equilibrium . By using the formula (4), the value of Jacobi matrix at
. By using the formula (4), the value of Jacobi matrix at  is
is

where ,
,  ,
,
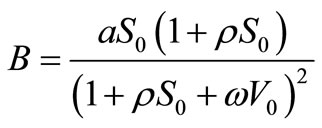 and
and .
.
We calculate the eigenvalues of  as follow:
as follow:
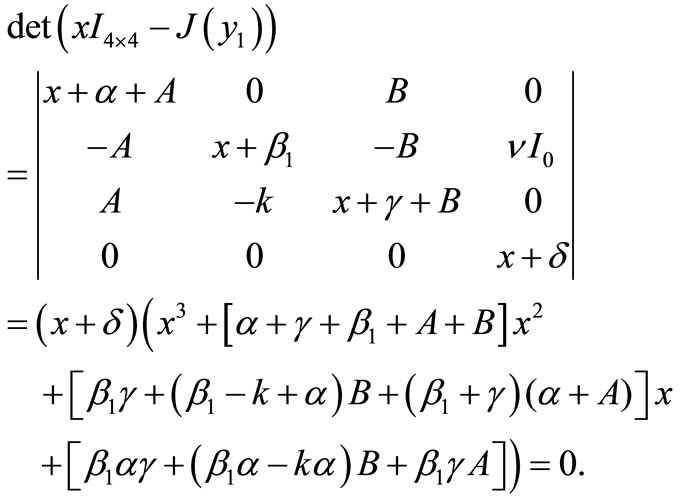
Thus one of the roots is . The other roots are given by
. The other roots are given by

Here ,
,  and
and  .
.
By substituting the value of  and
and  in
in  and
and  we see that
we see that  and
and  are positive. Moreover, it is easy to check that,
are positive. Moreover, it is easy to check that, . By the Rouths Hurwitz Criteria, all roots of the cubic polynomial have negative real part. Therefore we have the following theorem.
. By the Rouths Hurwitz Criteria, all roots of the cubic polynomial have negative real part. Therefore we have the following theorem.
Theorem 3.1. If,  , then the equilibrium point
, then the equilibrium point 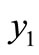 exists and is locally asymptotically stable. Moreover the equilibrium
exists and is locally asymptotically stable. Moreover the equilibrium  exists and is unstable.
exists and is unstable.
Remark 3.1. Since, the rest points and the eigenvalues depend continuously on the parameters, thus for small values of  and
and ,
,  exists and is locally asymptotically stable.
exists and is locally asymptotically stable.
Case 2. 
In this case, the system (1) has three equilibrium points and  is one of them. Since
is one of them. Since , the fourth equation of the system (1) gives
, the fourth equation of the system (1) gives  Therefore,
Therefore,  or
or  For
For , substituting the value of
, substituting the value of 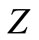 into the second equation yields,
into the second equation yields,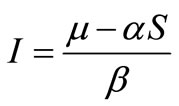 . By using the value of
. By using the value of  and the third equation we get,
and the third equation we get,
 . Substituting values of
. Substituting values of 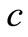 and
and 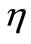
into the equation (3), we obtain,
 . Thus, we get
. Thus, we get
 as another rest point of the system (1).
as another rest point of the system (1).
For , by substituting this value of
, by substituting this value of 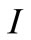 in the second equation of the system (1), we obtain
in the second equation of the system (1), we obtain
 . Then from the third equation we get,
. Then from the third equation we get, . By using this value of
. By using this value of  into the first equation of the system (1), we obtain the following quadratic equation.
into the first equation of the system (1), we obtain the following quadratic equation.

If  and
and then
then 
is another equilibrium point of the system (1) where 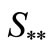 is the positive root of the above quadratic equation.
is the positive root of the above quadratic equation.
In the following, we consider the stability property of these points.
At first consider it for . Here we check the sign of the eigenvalues of Jacobi matrix of the system (1) at
. Here we check the sign of the eigenvalues of Jacobi matrix of the system (1) at . From the formula (4) we have
. From the formula (4) we have

where ,
,  ,
,
 and
and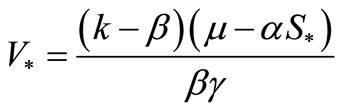 .
.
We calculate the eigenvalues of  as follows:
as follows:

Thus one of the roots is . The other roots are given by
. The other roots are given by

Here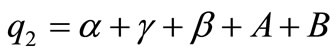 ,
,  and
and 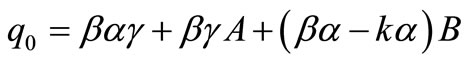 .
.
By substituting the value of  and
and  in
in  and
and  we will see that
we will see that  and
and 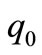 are positive. Moreover, it is easy to see that,
are positive. Moreover, it is easy to see that,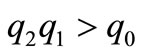 . By the Rouths Hurwitz Criteria, all roots of the cubic polynomial have negative real part. If
. By the Rouths Hurwitz Criteria, all roots of the cubic polynomial have negative real part. If  or
or
 , then real part of all of the eigenvalues are negative. Therefore the point
, then real part of all of the eigenvalues are negative. Therefore the point 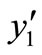 is locally asymptotically stable.
is locally asymptotically stable.
Now we consider the stability property of the other equilibrium point,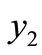 . From the formula (4) we have
. From the formula (4) we have
 where
where ,
,  ,
,  ,
,  and
and  .
.
The eigenvalues of the matrix  are given by the algebraic equation,
are given by the algebraic equation,
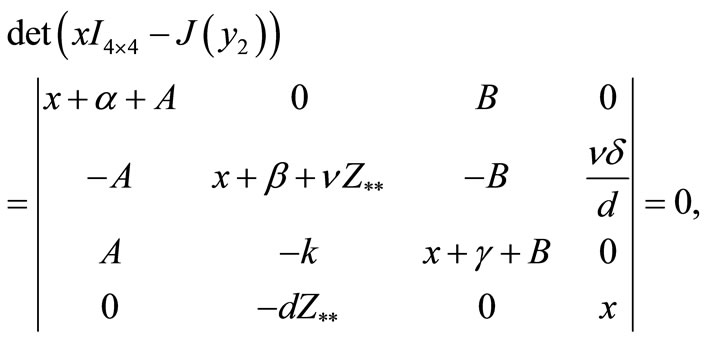
or
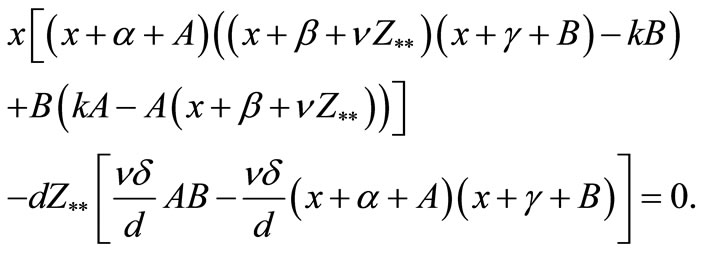
Then from the above equation we get

By considering the value of  it follows that all of the coefficients of the above equation are positive, then from Routh Hourwitz Criteria we see that all of the roots have negative real parts. Therefore we have the following theorem.
it follows that all of the coefficients of the above equation are positive, then from Routh Hourwitz Criteria we see that all of the roots have negative real parts. Therefore we have the following theorem.
Theorem 3.2. For  and
and , we have the following results.
, we have the following results.
1) If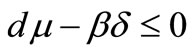 , the equilibrium points
, the equilibrium points  and
and  are the only rest points of the system (1), then
are the only rest points of the system (1), then  is unstable and
is unstable and  is locally asymptotically stable.
is locally asymptotically stable.
2) If , then for
, then for , the equilibrium
, the equilibrium , is locally asymptotically stable and for
, is locally asymptotically stable and for
 , it becomes unstable. If
, it becomes unstable. If ,
,
 and
and  are the only two rest points of the system (1) and
are the only two rest points of the system (1) and  does not exist.
does not exist.
3) If 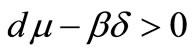 and
and then the equilibrium
then the equilibrium  exists and is locally asymptotically stable. Moreover the equilibrium points
exists and is locally asymptotically stable. Moreover the equilibrium points  and
and  are unstable.
are unstable.
Remark 3.2. If , the point
, the point  does not exist, therefore the point
does not exist, therefore the point  is the only endemic equilibrium point of the system (1). Also, for
is the only endemic equilibrium point of the system (1). Also, for 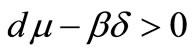
and , the point
, the point  is the only endemic equilibrium point.
is the only endemic equilibrium point.
Remark 3.3. 1) From continuous dependent of the equilibrium points and eigenvalues to the parameters, Theorem 3.1 and 3.2 must be valued for  and small.
and small.
2) For the case,  and large, if
and large, if , the point
, the point
 is the unique equilibrium of the system
is the unique equilibrium of the system
(1) which is globally asymptotically stable. If , the system (1) has a unique endemic equilibrium point,
, the system (1) has a unique endemic equilibrium point, 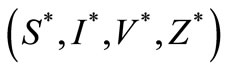 satisfying in the equations
satisfying in the equations
 ,
,
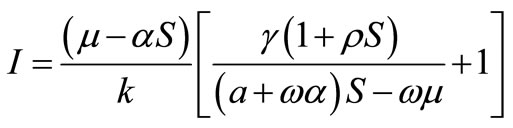 ,
,  and the equation (3). Here stability property of this point is not shown.
and the equation (3). Here stability property of this point is not shown.
4. Numerical Simulation
For the following numerical simulations, we use parameters of T-cells as the parameters of immune cells, those are ,
,  days. The estimated value of
days. The estimated value of 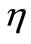 is obtained by assuming that the equilibrium value of the density of immune cells in the absence of infection is 2000 cells.
is obtained by assuming that the equilibrium value of the density of immune cells in the absence of infection is 2000 cells.
In this model the endemic status of the disease depends on the individual response toward incoming viruses. The larger the invasion rate , the chance is higher to catch the disease. On the contrary the increase of the elimination rate
, the chance is higher to catch the disease. On the contrary the increase of the elimination rate  of infected cell, the risk of infection is lower.
of infected cell, the risk of infection is lower.
For ,
,
 ,
,
 , we have For
, we have For  we obtain the same result in the above table.
we obtain the same result in the above table.
If  then for the same value of parameters we have the following table.
then for the same value of parameters we have the following table.
5. Conclusions
In order to understand the main characteristic of Dengue mystery, the author in [4] assumed that this virus can be eliminated by immune response which is described by the last equation of the system (1).
By using linear incidence rate of susceptible and free virus particle, they analyzed the existence of the endemic virus equilibria.
In this paper, from the analysis of the endemic equilibria it is found that, for Beddington DeAngelis incidence rate of susceptible and free virus particle, the same results are valid.
The reson for this correspondence is that in both models, the feature of the immune response is described by the term . However, the parameter
. However, the parameter  in Beddington DeAngles makes the elimination of dengue virus by immune response in a shorter time. This fact can be seen by comparing Tables 1 and 2.
in Beddington DeAngles makes the elimination of dengue virus by immune response in a shorter time. This fact can be seen by comparing Tables 1 and 2.
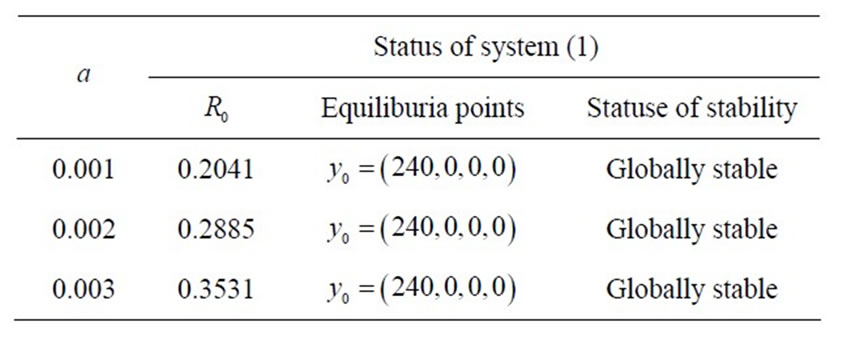
Table 1. status of equilibrium points of system (1) in the case .
.
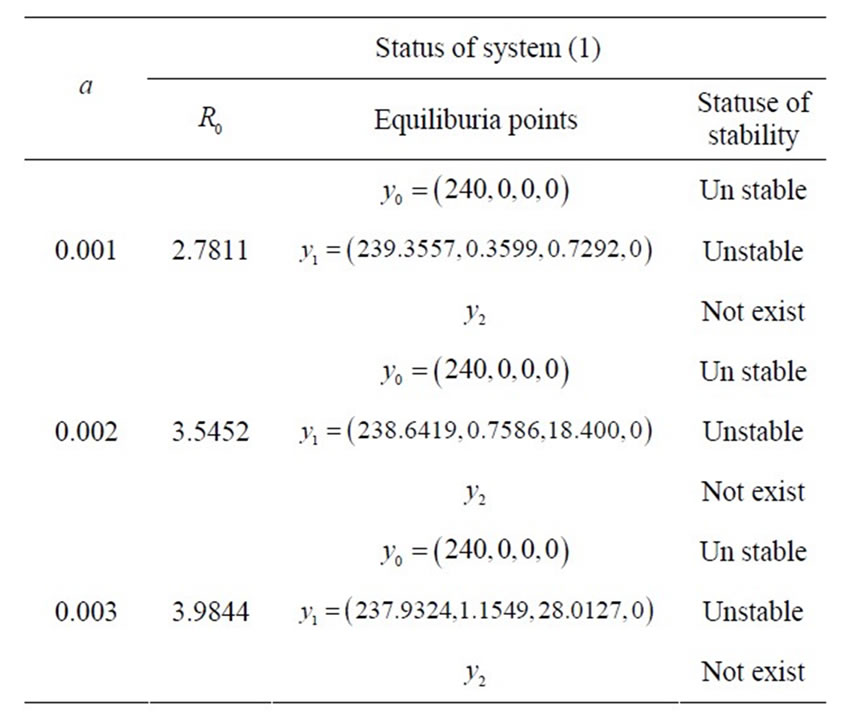
Table 2. status of equilibrium points of system (1) in the case ,
, .
.
By Theorem 2.2, if the basic reproduction number,  is less than one, then uninfected equilibrium point,
is less than one, then uninfected equilibrium point,  is the only steady state point of system (1) and it is globally asymptotically state. This means that the virus is eliminated by immune response. For larger values of
is the only steady state point of system (1) and it is globally asymptotically state. This means that the virus is eliminated by immune response. For larger values of 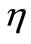 and
and ,
,  is more attractor and the virus is cleared much faster.
is more attractor and the virus is cleared much faster.
If the basic reproduction number,  is more than one; for
is more than one; for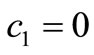 , besides of the uninfected steady state
, besides of the uninfected steady state 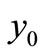 which is uninfected, there are some infected steady state. Here we consider two cases of endemic virus.
which is uninfected, there are some infected steady state. Here we consider two cases of endemic virus.
Fist, for , we have only one infected endemic
, we have only one infected endemic . If
. If , there is no immune response, so the density of susceptible moncytes equal zero. In
, there is no immune response, so the density of susceptible moncytes equal zero. In , this density equals
, this density equals , so it does not depend on the other parameters for virus load of infected cell. For lager values of
, so it does not depend on the other parameters for virus load of infected cell. For lager values of  and
and , the infected endemic
, the infected endemic  is closer to the uninfected endemic
is closer to the uninfected endemic  and it is more controllable.
and it is more controllable.
Second, for  and
and , from Theorem 3.2 we see that if
, from Theorem 3.2 we see that if  is negative or positive small, then there is only one infected endemic equilibrium
is negative or positive small, then there is only one infected endemic equilibrium  which is stable. However if
which is stable. However if  is positive and large, then the endemic virus equilibrium
is positive and large, then the endemic virus equilibrium  exists and is stable. This means that we found
exists and is stable. This means that we found 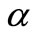 new threshold for
new threshold for . For condition
. For condition 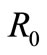 is less than this threshold the dynamic of the model is qualitatively same as the case
is less than this threshold the dynamic of the model is qualitatively same as the case . When
. When  is greater than this threshold, we have a new endemic virus equilibrium,
is greater than this threshold, we have a new endemic virus equilibrium, 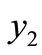 which is stable and the equilibrium points
which is stable and the equilibrium points  and
and  are unstable. From the components of the endemic equilibrium
are unstable. From the components of the endemic equilibrium  we see that after the onset of the symptom, if
we see that after the onset of the symptom, if 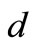 increases, the
increases, the  and
and  components of equilibria decrease and the
components of equilibria decrease and the  and Z-components of equilibria will increase. Conversely, if
and Z-components of equilibria will increase. Conversely, if  and
and  increase, the
increase, the 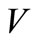 and I-components of equilibria will decrease but the virus load increases at the initial viral infection.
and I-components of equilibria will decrease but the virus load increases at the initial viral infection.
For case  and large and
and large and , the model has a unique endemic virus. The
, the model has a unique endemic virus. The  and
and  components of this equilibrium point decrease as
components of this equilibrium point decrease as  increases and the
increases and the  and Z-components of it increase as
and Z-components of it increase as  increases.
increases.
Therefore, 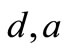 and
and  are the important parameters to capture the phenomena that dengue virus is quickly cleared in
are the important parameters to capture the phenomena that dengue virus is quickly cleared in  shorter time.
shorter time.
6. Acknowledgements
The authors would like to thank the anonymous reviewers for their valuable comments and suggestions to improve the manuscript.