The Dynamic Properties of a Deterministic SIR Epidemic Model in Discrete-Time ()
1. Introduction
Since Kermack and McKendrick [1] proposed the Susceptible-Infective-Recovered model (or SIR for short) in 1927, a lot of glorious studies on the dynamics of the epidemic models have been presented (see [2] -[9] ). The basic and important research subjects for these systems are local and global stability of the disease-free equilibrium and the endemic equilibrium, existence of periodic solutions, persistence and extinction of the disease, etc. According to the dependence on variable (i.e., time), these systems were classified into two types: continuous- time system and discrete-time system.
For the epidemic models, there have been a lot of researches focusing on the case of continuous-time (see [2] -[6] and that cited therein). However, discrete-time models (or called difference equations) are also useful for modeling situations of epidemic. They can not only have the basic features of the corresponding continuous- time models but also provide a substantial reduction of computer time (see [10] ). What is more, a lot of discrete- time models are not trivial analogues of their continuous ones and simple models can even exhibit complex behavior (see [5] [10] ).
In 1989, Hethcote [7] considered a class of continuous epidemic model with vertical and horizontal transmission.
 (1)
(1)
where S represents the proportion of individuals susceptible to the disease, who are born (with b) and die (with d) at the same rate b (b = d), and have mean life expectancy 1/b. The susceptible becomes infectious at a bilinear rate βI, where I is the proportion of infectious individuals and β is the contact rate. The infectious recover (i.e., acquire lifelong immunity) at a rate r, so that 1/r is the mean infectious period. The constant p, q,  ,
, 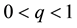 , and
, and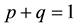 , where p is the proportion of the offspring of infective parents that are susceptible individuals, and q is the proportion of the offspring of infective parents that are infective individuals. Because of biological meanings, a natural constraint is
, where p is the proportion of the offspring of infective parents that are susceptible individuals, and q is the proportion of the offspring of infective parents that are infective individuals. Because of biological meanings, a natural constraint is . A similarly detailed description of the model and its dynamics may be found in [7] . In recent, Meng and Chen [8] have also studied the epidemic system (1). In their work, the basic reproductive rate determining the stability of disease-free equilibrium point and endemic equilibrium point was found out and the local and global stability of the equilibrium points have been researched by using Lyapunov function and Dulac function.
. A similarly detailed description of the model and its dynamics may be found in [7] . In recent, Meng and Chen [8] have also studied the epidemic system (1). In their work, the basic reproductive rate determining the stability of disease-free equilibrium point and endemic equilibrium point was found out and the local and global stability of the equilibrium points have been researched by using Lyapunov function and Dulac function.
In this paper, we pay attention to the discrete situation of (1). From discussing the influence of the coefficient parameters effected on the eigenvalues, we give the hyperbolicity of equilibrium points and determine which point is saddle, node or focus as well as their stability. Further, by deriving equations describing flows on the center manifolds, we discuss the transcritical bifurcation at the non-hyperbolic equilibrium point and research how does small perturbation of coefficient parameters affect the number and stability of equilibrium points. Moreover, we give some numerical simulation examples for illustrating the theoretical analysis and explain the biological meaning of our theorem.
2. Discrete SIR Epidemic Model with Vertical and Horizontal Transmission
In this section, we consider the discrete SIR epidemic model with vertical and horizontal transmission:
 (2)
(2)
where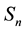 ,
, 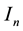 and
and 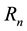 represent susceptible, infective and removed (or isolated) subgroups respectively, n represents a fixed time,
represent susceptible, infective and removed (or isolated) subgroups respectively, n represents a fixed time,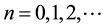 . It is assumed that
. It is assumed that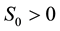 ,
, 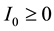 ,
, 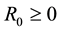 and
and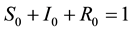 . In view of assumption that population is a constant size in [3] , i.e.,
. In view of assumption that population is a constant size in [3] , i.e.,
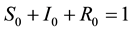 (3)
(3)
system (2) can be changed into
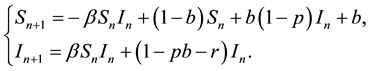 (4)
(4)
Rewrite (4) as a planar map F:
![]() (5)
(5)
It is obvious that this map has a disease-free equilibrium point ![]() and an endemic equilibrium point
and an endemic equilibrium point
![]() , where
, where![]() ,
, ![]() and
and![]() .
.
The organization of this paper is as follows. In next section, we identify all cases of non- and hyperbolic equilibria, which is a fundament for all succeeding studies. In Section 4, we discuss the transcritical bifurcation at the disease-free equilibrium of (1), the direction and stability of the transcritical bifurcation is investigated by computing a center manifold. In Section 5, some simulations are made to demonstrate our results and the biologic explanation of the theorem is also given.
3. Hyperbolic and Non-Hyperbolic Cases
In this section, we will discuss the hyperbolic and non-hyperbolic cases in a two parameters space parameter.
Theorem 1. The equilibrium point ![]() is non-hyperbolic if and only if
is non-hyperbolic if and only if ![]() lies on the lines:
lies on the lines:
![]()
and
![]()
Otherwise, the equilibrium point ![]() is in one of the following types (see Table 1).
is in one of the following types (see Table 1).
Remark 1. By Theorem 3.1 the domain ![]() is divided by line
is divided by line ![]() and
and ![]() into three districts
into three districts![]() ,
, ![]() and
and ![]() for equilibrium point P (see Figure 1).
for equilibrium point P (see Figure 1).
Proof. The Jacobian matrix of (5) at ![]() is
is
![]()
and its eigenvalues are
![]()
![]()
Table 1. Types of hyperbolic equilibrium point![]() .
.
![]()
Figure 1. Districts for equilibrium point P.
From the assumption![]() , we see that
, we see that![]() . Then non-hyperbolicity will be happened in the case
. Then non-hyperbolicity will be happened in the case![]() . From
. From![]() , we know
, we know ![]() which means
which means ![]() lies on
lies on![]() ; Also, From
; Also, From![]() , we get that
, we get that ![]() and
and ![]() lies on
lies on![]() . When
. When ![]() (referred to the case
(referred to the case![]() ), the eigenvalue
), the eigenvalue ![]() satisfies
satisfies![]() , then the equilibrium point P is a stable node. When
, then the equilibrium point P is a stable node. When ![]() (referred to the case
(referred to the case![]() ), the equilibrium point P is a node since
), the equilibrium point P is a node since ![]() and meanwhile when
and meanwhile when![]() , the equilibrium P is a saddle since
, the equilibrium P is a saddle since![]() . The proof is complete. □
. The proof is complete. □
Theorem 2. There does not exist non-hyperbolic case for equilibrium point![]() . But the hyperbolicity can be divided into the following cases:
. But the hyperbolicity can be divided into the following cases:
(I) When![]() , there exist six types for hyperbolic equilibrium point Q (see Table 2).
, there exist six types for hyperbolic equilibrium point Q (see Table 2).
Where ![]() and
and ![]() satisfy
satisfy![]() :
:
![]()
and![]() :
:![]() , respectively.
, respectively.
(II) When![]() , there exist four types for hyperbolic equilibrium point Q (see Table 3).
, there exist four types for hyperbolic equilibrium point Q (see Table 3).
Where ![]() satisfies
satisfies![]() :
:![]() .
.
Remark 2. By Theorem 3.2, when![]() , the domain
, the domain ![]() is divided by line
is divided by line![]() ,
, ![]() and
and ![]() into three districts
into three districts![]() ,
, ![]() ,
, ![]() and
and ![]() for equilibrium point Q (see Figure 2).
for equilibrium point Q (see Figure 2).
When![]() , the domain
, the domain ![]() is divided by line
is divided by line ![]() and
and ![]() into three dis-
into three dis-
tricts![]() ,
, ![]() and
and ![]() for equilibrium point Q (see Figure 3).
for equilibrium point Q (see Figure 3).
Proof. Performing a coordinate shift as follows:
![]()
![]()
Table 2. Types of hyperbolic equilibrium![]() .
.
![]()
Table 3. Types of hyperbolic equilibrium![]() .
.
![]()
Figure 2. Districts for equilibrium point Q when b < β.
![]()
Figure 3. Districts for equilibrium point Q when b1 < β ≤ b.
and letting ![]() denote the transformed f, we translate equilibrium
denote the transformed f, we translate equilibrium ![]() into
into ![]() and discuss equilibrium point
and discuss equilibrium point ![]() of the map
of the map![]() . The matrix of linearization of
. The matrix of linearization of ![]() at
at ![]() is
is
![]()
where ![]() and
and![]() , and its eigenvalues are
, and its eigenvalues are
![]() (6)
(6)
It is known that ![]() is hyperbolic if and only if none of eigenvalues
is hyperbolic if and only if none of eigenvalues![]() ,
, ![]() lies on the unit circle
lies on the unit circle![]() . In the following we discuss the eigenvalues in two cases, i.e.,
. In the following we discuss the eigenvalues in two cases, i.e., ![]() and
and![]() .
.
Case (I). When discriminant![]() , then λ1 and λ2 are both real. Because non- hyperbolicity happens if and only if
, then λ1 and λ2 are both real. Because non- hyperbolicity happens if and only if ![]() or
or ![]() is 1. For whether λ1 = 1 or λ2 = 1, we can get
is 1. For whether λ1 = 1 or λ2 = 1, we can get![]() . However, for positive equilibrium point Q, we have 0 < a < 1 and 0 < r < 1. Therefore, neither λ1 = 1 nor λ2 = 1 is possible. Next, let’s examine
. However, for positive equilibrium point Q, we have 0 < a < 1 and 0 < r < 1. Therefore, neither λ1 = 1 nor λ2 = 1 is possible. Next, let’s examine ![]() and
and![]() . From whether
. From whether ![]() or
or![]() , we can get
, we can get![]() . By condition
. By condition![]() ,
, ![]() , we see that
, we see that![]() . This is a contradiction with
. This is a contradiction with![]() , so
, so ![]() and
and ![]() are impossible.
are impossible.
In the case of![]() ,
, ![]() and
and ![]() are a pair of conjugate complex. Since
are a pair of conjugate complex. Since
![]()
![]() and
and ![]() lie inside of
lie inside of ![]() and the equilibrium point Q is a stable focus referred to the case
and the equilibrium point Q is a stable focus referred to the case![]() .
.
If![]() , i.e.,
, i.e., ![]() , the matrix has a double real eigenvalue
, the matrix has a double real eigenvalue![]() . From the constraint condition
. From the constraint condition ![]() of s, it is obvious that
of s, it is obvious that![]() . Therefore, equilibrium Q is stable node in the cases of
. Therefore, equilibrium Q is stable node in the cases of ![]() and
and![]() .
.
If![]() , i.e.
, i.e. ![]() and
and![]() , the eigenvalues
, the eigenvalues ![]() and
and ![]() are different real numbers. We first discuss the case that
are different real numbers. We first discuss the case that![]() , i.e.
, i.e.![]() . In this case we have
. In this case we have
![]()
Since
![]()
we have ![]() for
for![]() . On the other hand, there also exists
. On the other hand, there also exists ![]() for
for![]() . In fact, since
. In fact, since
![]()
and
![]()
we have![]() . Therefore, the equilibrium Q is a stable node as
. Therefore, the equilibrium Q is a stable node as![]() .
.
For the case![]() , i.e.,
, i.e., ![]() , we have
, we have
![]()
and
![]()
Therefore, the equilibrium Q is a stable node as![]() .
.
Finally, we study the case of![]() , i.e.
, i.e.![]() . We have
. We have
![]()
Then, we have ![]() for
for![]() . Moreover, there also has
. Moreover, there also has ![]() for
for![]() . In fact that,
. In fact that,
![]()
This means that the equilibrium Q is a stable node for![]() .
.
Case (II). When discriminant![]() , then
, then ![]() and
and ![]() are both real. Because non-hyperbolicity happens if and only if
are both real. Because non-hyperbolicity happens if and only if ![]() or
or ![]() is 1. Similar to the proof in case (I), neither
is 1. Similar to the proof in case (I), neither ![]() nor
nor ![]() is possible.
is possible.
When![]() ,
, ![]() and
and ![]() are a pair of conjugate complex. Since
are a pair of conjugate complex. Since
![]()
![]() and
and ![]() lie inside of
lie inside of ![]() and the equilibrium point Q is a stable focus referred to the case
and the equilibrium point Q is a stable focus referred to the case![]() .
.
If![]() , the matrix has a double real eigenvalue
, the matrix has a double real eigenvalue![]() . From the constraint condition
. From the constraint condition ![]() of s, it is obvious that
of s, it is obvious that![]() . Therefore, equilibrium Q is stable node in the cases of
. Therefore, equilibrium Q is stable node in the cases of![]() .
.
If![]() , We first discuss the case that
, We first discuss the case that![]() , i.e.
, i.e.![]() . In this case we have
. In this case we have
![]()
We know ![]() for
for![]() . On the other hand, there exists
. On the other hand, there exists ![]() for
for![]() . In fact, since
. In fact, since
![]()
Therefore, the equilibrium Q is a saddle as![]() .
.
Finally, we study the case of![]() , i.e.
, i.e.![]() . We easily prove
. We easily prove ![]() for
for ![]() by same methods as in case (1). This means that the equilibrium Q is a stable node for
by same methods as in case (1). This means that the equilibrium Q is a stable node for![]() .
.
The proof is complete. □
4. Transcritical Bifurcation
In this section we consider the case that![]() , where the transcritical bifurcation at equilibrium point
, where the transcritical bifurcation at equilibrium point ![]() will happen. The following lemmas were be derived from reference [11] .
will happen. The following lemmas were be derived from reference [11] .
Lemma 1. ([11] , Theorem 2.1.4) The map
![]() (7)
(7)
satisfies that A is ![]() matrix with eigenvalues of modulus one, and B is
matrix with eigenvalues of modulus one, and B is ![]() matrix with eigenvalues of modulus less than one, and
matrix with eigenvalues of modulus less than one, and
![]() (8)
(8)
where f and g are ![]() in some neighborhood of the origin. Then there exists a
in some neighborhood of the origin. Then there exists a ![]() center manifold for (7) which can be locally represented as a graph as follows
center manifold for (7) which can be locally represented as a graph as follows
![]()
for ![]() sufficiently small. Moreover, the dynamics of (7) restricted to the center manifold is, for u sufficiently small, given by the c-dimensional map
sufficiently small. Moreover, the dynamics of (7) restricted to the center manifold is, for u sufficiently small, given by the c-dimensional map
![]()
Lemma 2. ([11] , in page 365) A one-parameter family of ![]() one-dimensional maps
one-dimensional maps
![]() (9)
(9)
having a nonhyperbolic fixed point, i.e.,
![]()
undergoes a transcritical bifurcation at ![]() if
if
![]()
Theorem 3. A transcritical bifurcation occurs at the equilibrium P when w = 1. More concretely, for w < 1 slightly there are two equilibriums: a stable point P and an unstable negative equilibrium which coalesce at w = 1 and for w > 1 slightly there are also two equilibriums: an unstable equilibrium P and a stable positive equilibrium Q. Thus an exchange of stability has occurred at w = 1.
Proof. For![]() , we have
, we have ![]() and
and![]() . Consider w as the bifurcation parameter and write F as
. Consider w as the bifurcation parameter and write F as ![]() to emphasize the dependence on w. One can easily see that the matrix
to emphasize the dependence on w. One can easily see that the matrix ![]() is
is
![]()
and it has eigenvectors
![]() (10)
(10)
corresponding to ![]() and
and ![]() respectively, where T means the transpose of matrices.
respectively, where T means the transpose of matrices.
First, we put the matrix ![]() into a diagonal form. Using the eigenvectors (10), we obtain the transformation
into a diagonal form. Using the eigenvectors (10), we obtain the transformation
![]() (11)
(11)
with inverse
![]() (12)
(12)
which transform system (5) into
![]() (13)
(13)
Rewrite system (13) in the suspended form with assumption![]() ,
,
![]() (14)
(14)
where![]() ,
, ![]() ,
, ![]() ,
,![]() .
.
Thus, from Lemma 4.1, the stability of equilibrium ![]() near
near ![]() can be determined by studying a one-parameter family of map on a center manifold which can be represented as follows,
can be determined by studying a one-parameter family of map on a center manifold which can be represented as follows,
![]()
for sufficiently small v and![]() .
.
We now want to compute the center manifold and derive the mapping on the center manifold. We assume
![]() (15)
(15)
near the origin, where ![]() means terms of order
means terms of order![]() . By Lemma 4.1, those coefficients A, B and C can be determined by the equation
. By Lemma 4.1, those coefficients A, B and C can be determined by the equation
![]() (16)
(16)
Substituting (15) into (16) and comparing coefficients of![]() ,
, ![]() and
and ![]() in (16), we get
in (16), we get
![]()
from which we solve
![]()
Therefore the expression of (15) is approximately determined. Substituting (15) into (14), we obtain a one dimensional map reduced to the center manifold
![]() (17)
(17)
It is easy to check that
![]() (18)
(18)
The condition (18) implies that in the study of the orbit structure near the bifurcation point terms of ![]() do not qualitatively affect the nature of the bifurcation, namely they do not affect the geometry of the curves of equilibriums passing through the bifurcation point. Thus, (18) shows that the orbit structure of (17) near
do not qualitatively affect the nature of the bifurcation, namely they do not affect the geometry of the curves of equilibriums passing through the bifurcation point. Thus, (18) shows that the orbit structure of (17) near ![]() is qualitatively the same as the orbit structure near
is qualitatively the same as the orbit structure near ![]() of the map
of the map
![]() (19)
(19)
Map (19) can be viewed as truncated normal form for the transcritical bifurcation (see Lemma 4.2). The stability of the two branches of equilibriums lying on both sides of ![]() are easily verified. □
are easily verified. □
5. Simulations
In this section, we will give a simulation to illustrate the result obtained in the above section.
Example 1. Let![]() ,
, ![]() ,
, ![]() and choose three groups of initial values for
and choose three groups of initial values for ![]() as follows:
as follows:
![]()
If let![]() , we see that
, we see that ![]() and two equilibrium points of system (2) occurs, where disease-free equilibrium point
and two equilibrium points of system (2) occurs, where disease-free equilibrium point ![]() is stable and other negative equilibrium point (no meaning in biology) is unstable. The simulation result is presented in Figure 4.
is stable and other negative equilibrium point (no meaning in biology) is unstable. The simulation result is presented in Figure 4.
If let![]() , then
, then ![]() and and two equilibrium points of system (2) occurs, where disease-free equilibrium point
and and two equilibrium points of system (2) occurs, where disease-free equilibrium point ![]() is unstable and other positive equilibrium point
is unstable and other positive equilibrium point ![]() is unstable. The simulation result is presented in Figure 5.
is unstable. The simulation result is presented in Figure 5.
6. Biological Explanation
The conclusion in Theorem 4.1 reveals a fact that the topological structure changes at disease-free equilibrium point will take place when system (2.1) encounters small perturbation for coefficient parameters. Concretely, when parameter![]() , system (2.1) has only one equilibrium point
, system (2.1) has only one equilibrium point ![]() which is structural unstable. With the change of the parameter w, the number and the stability of equilibrium point will be changed.
which is structural unstable. With the change of the parameter w, the number and the stability of equilibrium point will be changed.
If let![]() , we see that two equilibrium points of system (2) occur, where disease-free equilibrium point
, we see that two equilibrium points of system (2) occur, where disease-free equilibrium point ![]() is stable and other negative equilibrium points (no meaning in biology) are unstable. No matter how to select the initial value, the system tends to the disease-free equilibrium point after multiple iterations. Therefore, in this case, whether selection of initial value or small perturbation for coefficient parameters, epidemic disease will tend to be eliminated, that is, the population will be in disease-free state finally.
is stable and other negative equilibrium points (no meaning in biology) are unstable. No matter how to select the initial value, the system tends to the disease-free equilibrium point after multiple iterations. Therefore, in this case, whether selection of initial value or small perturbation for coefficient parameters, epidemic disease will tend to be eliminated, that is, the population will be in disease-free state finally.
If let![]() , we also see that two equilibrium points of system (2) occur, where disease-free equilibrium point
, we also see that two equilibrium points of system (2) occur, where disease-free equilibrium point ![]() is unstable and other endemic disease equilibrium points
is unstable and other endemic disease equilibrium points ![]() are stable. No matter how to select the initial value, the system tends to the endemic disease equilibrium point after multiple iterations. Therefore, in this case, whether selection of initial value or small perturbation for coefficient parameters, disease does not tend to be eliminated, that is, the population will be diseased state finally.
are stable. No matter how to select the initial value, the system tends to the endemic disease equilibrium point after multiple iterations. Therefore, in this case, whether selection of initial value or small perturbation for coefficient parameters, disease does not tend to be eliminated, that is, the population will be diseased state finally.
Therefore, in reality we may control the factors of contact rate, birth rate, recovery rate, etc., to achieve the aim of prevention and treatment of disease.
Acknowledgements
We thank the Editor and the referee for their comments. This work has been supported by the Science Innovation Project (Grant 2013KJCX0125) and the Innovation and Developing School Project (Grant 2014KZDXM065) of Department of Education of Guangdong province, the NSF of Guangdong province (Grant S2013010013385) and the NSFP of Lingnan Normal University (Grant ZL1303).
NOTES
*Corresponding author.