On Optimal Ordering of Service Parameters of a Coxian Queueing Model with Three Phases ()
1. Introduction
Phase-type queueing models are one of the essential parts of the stochastic queueing models. There is an urgent need to construct phase-type distributions for complex representations of queueing models. The recent works being done in this field are: D. R. Cox shows how any distribution having a rational Laplace transform can be represented by a sequence of exponential phases [1] . S. Asmussen, O. Nerman and M. Olsson gave a paper on fitting phase-type distributions with the EM Algorithm [2] . Q. -M. He and H. Zhang presented an algorithm for computing minimal ordered Coxian representations of phase-type distributions whose Laplace-Stieltjes transform had only real poles [3] . The optimal ordering of the tandem server with two stages is given by [4] . R. Marie studied on calculating equilibrium probabilities for Coxian queueing systems in [5] . X. A. Papaconstantinou analyzed the stationary Ek/C2/s queueing system in [6] . P. M. Snyder and W. J. Stewart considered two approaches to the numerical solution of single node queueing models with phase-type [7] . In [8] , an exact analysis of a fork/join station in a closed queueing network with inputs from servers with two-phase Coxian service distributions is represented. Q. -M. He and H. Zhang studied the approximation of matrix-exponential distributions by Coxian distributions in [9] . M. Fackrell gave a survey of where the phase-type distributions were used in the healthcare industry and purposed some ideas on how they were further utilized [10] . A. B. Zadeh studied a batch arrival queue system with Coxian-2 server vacations and admissibility restricted in [11] . V. Sağlam et al. give a paper on optimization of a Coxian queueing model with two phases in [12] .
There is not enough work on the studies of optimizing the orders of service parameters for Coxian queueing model so far. Considering this fact in this paper we analyze a Coxian stochastic queueing model with three phases, and the Kolmogorov equations of this model are constructed, limit probabilities and the stationary probabilities of customer numbers in the system are found. The performance measures of this model are obtained and in addition the optimal order of service parameters is given by a theorem by obtaining the loss probabilities of customers in the system. We also give the loss probability in terms of mean waiting time in the system. Finally it is shown with a numeric example that this theorem holds.
2. Stochastic Model
We have obtained stochastic equation systems of a Coxian queueing model with three servers in which the stream is Poisson with λ parameter. The service time of any customer at server i  is exponential with parameter
is exponential with parameter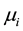 . Two or more customers can not have service in the system at the same time. Let
. Two or more customers can not have service in the system at the same time. Let 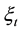 be the state of server 1,
be the state of server 1, 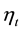 be the state of server 2 and
be the state of server 2 and 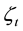 be the state of server 3 at any t time.
be the state of server 3 at any t time. 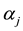 is the transition probability from
is the transition probability from 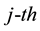 phase to
phase to 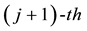 phase
phase 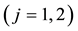 and 1 − αi be the loss probability of the system. This stochastic queueing model is illustrated in Figure 1. Limit probabilities, differential and difference equations of this system given later.
and 1 − αi be the loss probability of the system. This stochastic queueing model is illustrated in Figure 1. Limit probabilities, differential and difference equations of this system given later.
Limit Probabilities
Here ![]() is a three-dimensional Markov chain with continuous parameter and state space is
is a three-dimensional Markov chain with continuous parameter and state space is ![]()
![]() (1)
(1)
![]()
Figure 1. A three phase coxian queueing model.
Kolmogorov differential equation for these probabilities is obtained. The probabilities of the process ![]() will be found for
will be found for![]() , namely
, namely
![]() (2)
(2)
We write Equation (2) as follows as ![]()
![]() (3)
(3)
Furthermore, it is supposed that limiting distribution of ![]() are exist as follow:
are exist as follow:
![]() . (4)
. (4)
Steady-state equations for ![]() are obtained as following:
are obtained as following:
![]() (5)
(5)
![]() (6)
(6)
We define ![]() If we solve Equation (5) under condition (6), we obtain the following three dimension probability function:
If we solve Equation (5) under condition (6), we obtain the following three dimension probability function:
![]() (7)
(7)
3. Obtaining the Measures of Performance
Let be the random variable that describes the number of customers in the system. The mean number of costumers:
![]() (8)
(8)
![]() . (9)
. (9)
3.1. The Coxian Queue Using Laplace Transform
Let W be the random variable that describes waiting time of customers in the system. Laplace transform of W
![]() . (10)
. (10)
Mean waiting time in system of a customer for Cox(3) is found by formula (10)
![]() (11)
(11)
3.2. The optimization of Measures of Performance
Loss probability
Let ![]() be the loss probability of customer in the system. In this regards, since there is no queue in the system, loss probability is calculated as following:
be the loss probability of customer in the system. In this regards, since there is no queue in the system, loss probability is calculated as following:
![]() . (12)
. (12)
3.3. Optimal Order of Servers
We can put three different service parameters to three stages in 3! different position. In this case there are 6 different loss probabilities.
The following theorem is given on minimization of loss probability.
Theorem 1. Putting the greatest service parameter at first phase and the second greatest service parameter at second phase and the smallest service parameter at third phase makes the loss probability minimum. That is,
![]() (13)
(13)
Proof. Let’s suppose![]() . In this case we have,
. In this case we have,
![]()
![]()
![]()
![]()
![]()
![]()
![]()
![]() .
.
Similarly,
![]()
![]()
![]()
![]()
![]()
![]() .
.
Since,
![]()
we obtain
![]()
![]()
![]()
![]() .
.
![]()
![]()
![]()
![]()
![]() .
.
Finally,
![]()
![]()
![]()
![]()
![]()
Corollary. Since,
![]() (14)
(14)
the minimum value which makes ![]() minimum also makes
minimum also makes ![]() mininmum.
mininmum.
4. Numerical Example
In this section the loss probabilities are calculated for some values of system probabilities and ![]() probabilities. The calculated loss probabilities are given in Table 1. For the values
probabilities. The calculated loss probabilities are given in Table 1. For the values![]() ,
,![]() ;
;![]() ,
, ![]() and for various values of
and for various values of ![]() it is seen in Table 1 that
it is seen in Table 1 that ![]() has its minimum value, this shows that Theorem1 holds.
has its minimum value, this shows that Theorem1 holds.
Under condition given in Theorem1, for the values![]() ,
,![]() ;
;![]() ,
, ![]() and for all values of
and for all values of ![]() in domain set, the loss probabilities are calculated in Table 2 and graphically given in 3D Figure 2 in two different view angle.
in domain set, the loss probabilities are calculated in Table 2 and graphically given in 3D Figure 2 in two different view angle. ![]() is indicated by green surface in this figure. As it is seen in this graph,
is indicated by green surface in this figure. As it is seen in this graph, ![]() is minimum for all values of
is minimum for all values of![]() . For a customer to have service at each stage it must be
. For a customer to have service at each stage it must be ![]() or it must be
or it must be ![]() for the customer to leave the system after first stage.
for the customer to leave the system after first stage.
5. Conclusion
By constructing this stochastic queueing model, transient probabilities are obtained. Depending on these probabilities, mean number of customer in the system, the mean waiting time in this system by Laplace transform and the loss probability of any customer are given. It is shown by Theorem 1 that putting the greatest service parameter at first phase and the second greatest service parameter at second phase and the smallest service parameter at third phase makes the loss probability minimum. For the values![]() ,
,![]() ;
;![]() ,
, ![]() and
and
![]()
Table 1. Placing the service parameters to phases and corresponding loss probabilities.
for various values of ![]() it is seen in Table 1 that
it is seen in Table 1 that ![]() has its minimum value, this shows that Theorem1 holds. In the case
has its minimum value, this shows that Theorem1 holds. In the case![]() , the loss probabilities are all equal to each other. This is seen in both Table 1 and Graph 1. While
, the loss probabilities are all equal to each other. This is seen in both Table 1 and Graph 1. While ![]() and
and ![]() this system turns into
this system turns into ![]() queueing model and while
queueing model and while ![]() the system turns into Cox(2) queueing model. For further studies, higher moments of meanwaiting time in the system can be obtained and by using these moments some various statistical measures can be calculated such as variance, skewness, kurtosis and coefficient of variation. Also this model can be expanded to k-phases.
the system turns into Cox(2) queueing model. For further studies, higher moments of meanwaiting time in the system can be obtained and by using these moments some various statistical measures can be calculated such as variance, skewness, kurtosis and coefficient of variation. Also this model can be expanded to k-phases.