An Improved Algorithm for the Solution of Generalized Burger-Fishers Equation ()
1. Introduction
Generalized Burger-Fisher equation, being a nonlinear partial differential equation, is of great importance for describing the interaction between reaction mechanisms, convection effects, and diffusion transports. Since there exists no general technique for finding analytical solution of nonlinear diffusion equations so far, numerical solutions of nonlinear equations are of great importance in physical problems.
Many researchers [1] -[13] have used various numerical methods to solve Generalized Burger-Fisher. Recently Javidi [10] used modified pseudospectral method for generalized Burger’s-Fisher equation. Kaya [2] introduced a numerical simulation of the generalized Burger’s-Fisher equation. Ismail [8] presented a restructive pade approximation for the solution of the generalized Burger’s-Fisher equation. Hassan et al. [3] studied Adomian Decomposition Method (ADM) for generalized Burger’s-Huxley and Burger’s-Fisher equations.
Unlike some previous methods that used various transformations and several iterations, we present a new Modified Variational Iteration Method (MVIM) for the numerical solutions of generalized Burger-Fisher equation.
In Table 1, the results of MVIM were compared with those of ADM and VIM when
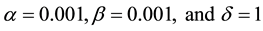 .
.
In Table 2, we compared MVIM, ADM and VIM results for 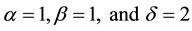 while in Table 3 the results are compared for
while in Table 3 the results are compared for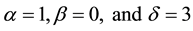 .
.
Figure 1 and Figure 2 show the graphical representation of gBF for various values of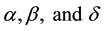 .
.
Figure 3 shows the graph of exact solution and MVIM solution. Figure 4 also represents graph of gBF when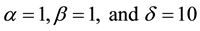 .
.
2. Modified Variational Iteration Method (MVIM)
The idea of variational iteration can be traced to Inokuti [9] . The variational iteration method was proposed by J.-H. He [4] - [7] , In this paper, a Modified Variational Iteration Method proposed by Olayiwola [11] - [14] is
presented for the solution of the generalized Burger-Fisher equation.
To illustrate the basic concept of the MVIM, we consider the following general nonlinear partial differential equation:
![]() (1.0)
(1.0)
where L is a linear time derivative operator, R is a linear operator which has partial derivative with respect to![]() , N is a nonlinear operator and g is an inhomogeneous term. According to MVIM, we can construct a correct functional as follows:
, N is a nonlinear operator and g is an inhomogeneous term. According to MVIM, we can construct a correct functional as follows:
![]() (1.1)
(1.1)
![]() (1.2)
(1.2)
where ![]() can be evaluated by substituting
can be evaluated by substituting ![]() in (2.1) and at
in (2.1) and at![]() .
.
![]() is a Lagrange multiplier which can be identified optimally via Variational Iteration Method. The subscript
is a Lagrange multiplier which can be identified optimally via Variational Iteration Method. The subscript ![]() denote the nth approximation,
denote the nth approximation, ![]() is considered as a restricted variation i.e.,
is considered as a restricted variation i.e.,![]() .
.
3. MVIM for the Solution of Generalized Burger-Fisher Equation
The following generalized Burger-Fisher (gBF) equation problems arising in various field of science is considered.
![]() (1.3)
(1.3)
with the initial condition
![]() (1.4)
(1.4)
And the boundary conditions
![]() (1.5)
(1.5)
![]() (1.6)
(1.6)
![]() are parameters such that
are parameters such that![]() . When
. When![]() , equation (1.3) reduces to Burger’s-Fisher (BF) equation
, equation (1.3) reduces to Burger’s-Fisher (BF) equation
We used Maple to code (1.1 - 1.2) for the solution of (1.3 - 1.6) and the following results were obtained after one iteration:
When ![]() (1.3) is reduced to the generalized Burger’s equation. The comparison between the absolute error for the exact solution and approximate solution is presented in Table 3.
(1.3) is reduced to the generalized Burger’s equation. The comparison between the absolute error for the exact solution and approximate solution is presented in Table 3.
4. Results and Discussion
Tables 1-3 shows that the MVIM is the best approximant when compared with VIM and ADM. Figure 2 is the graph of Exact solution for the generalized Burger-fisher when![]() .
.
Figure 3 compares the graph of Exact with the MVIM. It is also to be noted that both graphs of Burger-Fisher and generalized Burger-Fisher as shown in Figure 1 and Figure 3, respectively, justify the conclusion that the two equations approaches the same steady state. However, as ![]() grows
grows ![]() becomes independent of
becomes independent of ![]() as shown in Figure 4.
as shown in Figure 4.
5. Conclusions
There are some important points to note here. First, the MVIM provides the solutions in terms of convergent series with easily computable components. Second, it is clear and remarkable that approximate solutions using MVIM are in good agreement. Third, the MVIM technique requires less computational work than many existing approaches. The MVIM was used in a direct way without using linearization, perturbation or restrictive assumptions.
The MVIM provides more realistic series solutions, very high accuracy, fast transformation and possibility of implementation of algorithm. The Algorithm makes it easier for the system to predict the next series.