Numerical Study of Fractional Differential Equations of Lane-Emden Type by Method of Collocation ()
1. Introduction
Lane-Emden Differential Equation has the following form:
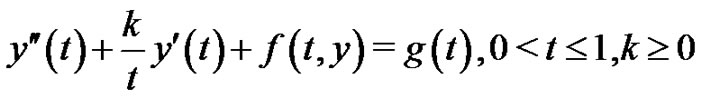 (1)
(1)
with the initial condition

where 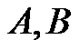 are constants,
are constants,  is a continuous real valued function and
is a continuous real valued function and  (see [1]).
(see [1]).
Lane-Emden differential equations are singular initial value problems relating to second order differential equations (ODEs) which have been used to model several phenomena in mathematical physics and astrophysics.
In this paper we generalize the definition of LaneEmden equations up to fractional order as following:
 (2)
(2)
with the initial condition

where  are constants,
are constants,  is a continuous real-valued function and
is a continuous real-valued function and  The theory of singular boundary value problems has become an important area of investigation in the past three decades [2-5]. One of the equations describing this type is the Lane-Emden equation. Lane-Emden type equations, first published by Jonathan Homer Lane in 1870 (see [6]), and further explored in detail by Emden [7], represents such phenomena and having significant applications, is a second-order ordinary differential equation with an arbitrary index, known as the polytropic index, involved in one of its terms. The Lane-Emden equation describes a variety of phenomena in physics and astrophysics, including aspects of stellar structure, the thermal history of a spherical cloud of gas, isothermal gas spheres,and thermionic currents [8].
The theory of singular boundary value problems has become an important area of investigation in the past three decades [2-5]. One of the equations describing this type is the Lane-Emden equation. Lane-Emden type equations, first published by Jonathan Homer Lane in 1870 (see [6]), and further explored in detail by Emden [7], represents such phenomena and having significant applications, is a second-order ordinary differential equation with an arbitrary index, known as the polytropic index, involved in one of its terms. The Lane-Emden equation describes a variety of phenomena in physics and astrophysics, including aspects of stellar structure, the thermal history of a spherical cloud of gas, isothermal gas spheres,and thermionic currents [8].
The solution of the Lane-Emden problem, as well as other various linear and nonlinear singular initial value problems in quantum mechanics and astrophysics, is numerically challenging because of the singularity behavior at the origin. The approximate solutions to the Lane-Emden equation were given by homotopy perturbation method [9], variational iteration method [10], and Sinc-Collocation method [11], an implicit series solution [12]. Recently, Parand et al. [13] proposed an approximation algorithm for the solution of the nonlinear LaneEmden type equation using Hermite functions collocation method. Moreover, Adibi and Rismani [14] introduced a modified Legendre-spectral method. While, Bhrawy and Alofi [15,16] imposed a Jacobi-Gauss collocation method for solving nonlinear Lane-Emden type equations. Finally, Yigider [1] introduced numerical study of Lane-Emaden Type using Pade Approximation.
2. Fractional Calculus
Fractional calculus and its applications (that is the theory of derivatives and integrals of any arbitrary real or complex order) has importance in several widely diverse areas of mathematical physical and engineering sciences. It generalized the ideas of integer order differentiation and n-fold integration. Fractional derivatives introduce an excellent instrument for the description of general properties of various materials and processes. This is the main advantage of fractional derivatives in comparison with classical integer-order models, in which such effects are in fact neglected. The advantages of fractional derivatives become apparent in modeling mechanical and electrical properties of real materials, as well as in the description of properties of gases, liquids and rocks, and in many other fields (see [17]).
The class of fractional differential equations of various types plays important roles and tools not only in mathematics but also in physics, control systems, dynamical systems and engineering to create the mathematical modeling of many physical phenomena. Naturally, such equations required to be solved. Many studies on fractional calculus and fractional differential equations, involving different operators such as Riemann-Liouville operators [18], Erdlyi-Kober operators [19], Weyl-Riesz operators [20], Caputo operators [21] and GrnwaldLetnikov operators [22], have appeared during the past three decades. The existence of positive solution and multipositive solutions for nonlinear fractional differential equation are established and studied [23]. Moreover, by using the concepts of the subordination and superordination of analytic functions, the existence of analytic solutions for fractional differential equations in complex domain are suggested and posed in [24,25].
One of the most frequently used tools in the theory of fractional calculus is furnished by the Riemann-Liouville operators (see [22]). The Riemann-Liouville fractional derivative could hardly pose the physical interpretation of the initial conditions required for the initial value problems involving fractional differential equations. Moreover, this operator possesses advantages of fast convergence, higher stability and higher accuracy to derive different types of numerical algorithms [26].
Definition 2.1. The fractional (arbitrary) order integral of the function  of order
of order  is defined by
is defined by

when  we write
we write 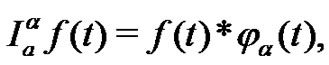 where
where  denoted the convolution product (see [22]),
denoted the convolution product (see [22]),
 and
and  and
and 
as  where
where  is the delta function.
is the delta function.
Definition 2.2. The fractional (arbitrary) order derivative of the function  of order
of order 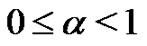 is defined by
is defined by

Remark 2.1. From Definition 2.1 and Definition 2.2, we have

and

In this note, we consider the fractional Lane-Emden equations of the in Equation (2).
3. Analytic Solution
Consider that we are given a power series representing the solution of fractional Lane-Enden differential equations:
 (3)
(3)
hence
 (4)
(4)
Theorem: The analytic solution of the IVP(2) satisfied the following equation:
 (5)
(5)
proof Substitute (3) and (4) into Equation (2), we obtain the desired equation.
The method of power series depends to find the coefficients  as a function of n and
as a function of n and .
.
3.1. Linear Lane-Emden Fractional Differential Equation
Consider  in Equation (2) thus
in Equation (2) thus
 (6)
(6)
with the initial condition

Equation (5) convert to the following equation
 (7)
(7)
In case  = 0, we obtain
= 0, we obtain  and in general
and in general
 (8)
(8)
Examples
Example 3.1.1.1 Let , we pose the linear FDE
, we pose the linear FDE
 (9)
(9)
with the initial condition

Consider the solution of FDE is 
Consequently,we have
 (10)
(10)
Hence
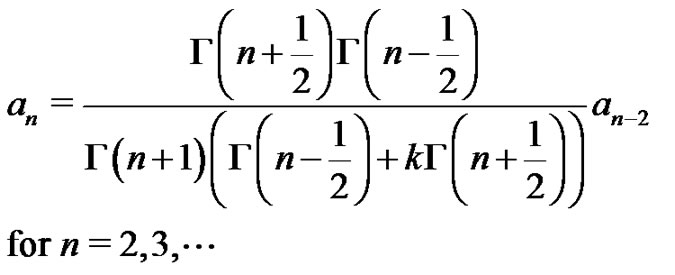 (11)
(11)
Example 3.1.1.2 Let , we get the linear FDE
, we get the linear FDE
 (12)
(12)
with the initial condition
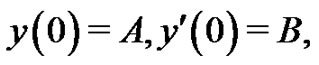
Consider the solution of FDE is 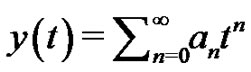
Consequently,we have
 (13)
(13)
with
 (14)
(14)
4. Numerical Collocation Method
Collocation method for solving differential equations is one of the most powerful approximate methods for solving fractional differential equations. This method has its basis upon approximate the solution of FDE by a series of complete sequence of functions, in which we mean by a complete sequence of functions, a sequence of linearly independent functions which has no non zero function perpendicular to this sequence of functions In general, y(t) is approximated by
 (15)
(15)
where  for
for  are an arbitrary constants to be evaluated and
are an arbitrary constants to be evaluated and  for
for  are given set of functions. Therefore, the problem in Equation (6) of evaluating y(t) is approximated by (16) then is reduced to the problem of evaluating the coefficients
are given set of functions. Therefore, the problem in Equation (6) of evaluating y(t) is approximated by (16) then is reduced to the problem of evaluating the coefficients  for
for .
.
Let  is a partition to interval [0,1] and
is a partition to interval [0,1] and
 and
and  and
and 
Define
 (16)
(16)
Hence
 (17)
(17)
Consider the solution of Equation (6) as following
 (18)
(18)
operating by  we obtain
we obtain

hence

put  we get
we get
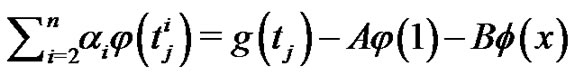
A linear system Ax = b of n – 1 equations in n – 1 variables is obtained and 
 for
for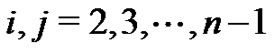 .
.
Hence, from Equation (6) we obtain the linear system Ax = b which could be solved by using any numerical method for solving linear system of algebraic equations.
Numerical Examples
To implement our examples, we used Matlab R2009b on Intel(R)core TM2Duo processor with 3.00 GHZ and 3 GB RAM.
Example 4.1.1
 (19)
(19)
with the initial condition 
Hence

See Table 1 and Figure 1, where the exact solution is
 and
and .
.
Example 4.1.2
 (20)
(20)
with the initial condition 
Hence

See Table 2 and Figure 2, where the exact solution is
 and
and .
.

Table 1. Absolute error of numerical solution of Example 4.1.

Figure 1. Numerical and analytic graph of solution of Example 4.1.

Figure 2. Numerical and analytic graph of solution of Example 4.2.

Table 2. Absolute error of numerical solution of Example 4.2.
5. Conclusion
From above, we imposed the Lane-Emden differential equation of fractional order. The generality of definition of Lane-Emden as a fractional order is more importance in applied mathematics, mathematical physics and astrophysics. The order appeared in two different fractional powers. An approximate solution is obtained by employing the method of power series. Furthermore, a numerical solution is established by Collection method for these equations.