Modifications of the Weyl-Heisenberg algebra
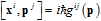
are proposed where the classical limit
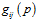
corresponds to a metric in (curved) momentum spaces. In the simplest scenario, the
2D de Sitter metric of constant curvature in momentum space furnishes a hierarchy of modified uncertainty relations leading to a minimum value for the position uncertainty . The first uncertainty relation of this hierarchy has the same functional form as the
stringy modified uncertainty relation with a Planck scale minimum value for
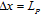
at
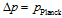
. We proceed with a discussion of the most general curved phase space scenario (cotangent bundle of spacetime) and provide the noncommuting phase space coordinates algebra in terms of the symmetric
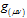
and nonsymmetric

metric components of a Hermitian complex metric
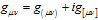
, such
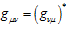
. Yang’s noncommuting phase-space coordinates algebra, combined with the Schrodinger-Robertson inequalities involving angular momentum eigenstates, reveals how a quantized area operator in units of

emerges like it occurs in Loop Quantum Gravity (LQG). Some final comments are made about Fedosov deformation quantization, Noncommutative and Nonassociative gravity.