Journal of Applied Mathematics and Physics
Vol.03 No.07(2015), Article ID:57573,4 pages
10.4236/jamp.2015.37088
On No-Node Solutions of the Lazer-McKenna Suspension Bridge Models
Fanglei Wang, Kangbao Zhou
College of Science, Hohai University, Nanjing, China
Email: wang-fanglei@hotmail.com, kangabaoz@sina.cn


Received 3 March 2015; accepted 23 June 2015; published 30 June 2015

ABSTRACT
In this paper, we are concerned with the existence and multiplicity of no-node solutions of the Lazer-McKenna suspension bridge models by using the fixed point theorem in a cone.
Keywords:
Differential Equations, Periodic Solution, Cone, Fixed Point Theorem

1. Introduction
In [1], the Lazer-McKenna suspension bridge models are proposed as following

If we look for no-node solutions of the form 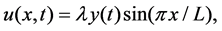
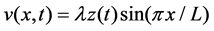 and impose a forcing term of the form
and impose a forcing term of the form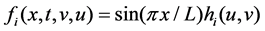 , then via some computation, we can obtain the following system:
, then via some computation, we can obtain the following system:
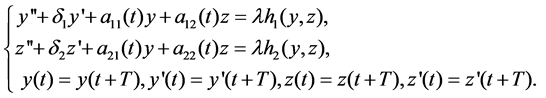 (1)
(1)
In this paper, by combining the analysis of the sign of Green's functions for the linear damped equation, together with a famous fixed point theorem, we will obtain some existence results for (1) if the nonlinearities satisfy the following semipositone condition
(H) The function 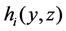 is bounded below, and maybe change sign, namely, there exists a sufficiently large constant M > 0 such that
is bounded below, and maybe change sign, namely, there exists a sufficiently large constant M > 0 such that 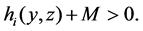
Such case is called as semipositone problems, see [2]. And one of the common techniques is the Krasnoselskii fixed point theorem on compression and expansion of cones.
Lemma 1.1 [3]. Let  be a Banach space,and
be a Banach space,and  be a cone in
be a cone in . Assume
. Assume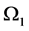 ,
,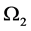 are open subsets of
are open subsets of 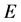 with
with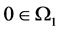 ,
, 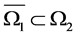 , Let
, Let 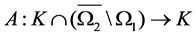 be a completely continuous operator such that either
be a completely continuous operator such that either
(i) ; or
; or
(ii)
Then, 
2. Preliminaries
If the linear damped equation

is nonresonant, namely, its unique T-periodic solution is the trivial one, then as a consequence of Fredholm’s alternative in [4], the nonhomogeneous equation 
which can be written as 
we will assume that the following standing hypothesis is satisfied throughout this paper:
(H1) 

is positive for all
(H2) 
Let E denote the Banach space 





If (H), (H1) and (H2) hold, let

where 
Let 

t is straightforward to verify that the solution of (1) is equivalent to the fixed point Equation
Lemma 2.1 Assume that (H), (H1) and (H2) hold. Then 
For convenience, define

Lemma 2.2 [2] Assume that (H), (H1) and (H2) hold. If




Lemma 2.3 [2] Assume that (H), (H1) and (H2) hold. If




3. Main Results
Theorem 3.1 Assume that (H), (H1) and (H2) hold.
(I) Then there exists a 
(II) If

(III) If

Proof. (I) On one hand, take R > 0 such that
Set 

Then from the above inequalities, it follows that there exists a 
Furthermore, for any
In the similar way, there exists a

So let us choose 
On the other hand, from the condition 





Then, for any
So we have
Therefore, from Lemma 1.1, it follows that the operator B has at least one fixed point 

(II) Since







Then, for each
In the similar way, for any

Therefore, from Lemma 1.1, it follows that B has one fixed point 

(III) Since




Choosing 


Thus from the above inequalities, we can get
Therefore, from Lemma 1.1, it follows that the operator B has at least two fixed points 



Cite this paper
Fanglei Wang,Kangbao Zhou, (2015) On No-Node Solutions of the Lazer-McKenna Suspension Bridge Models. Journal of Applied Mathematics and Physics,03,737-740. doi: 10.4236/jamp.2015.37088
References
- 1. Lazer, A.C. and McKenna, P.J. (1990) Large-Amplitude Periodic Oscillations in Suspension Bridges: Some New Connections with Nonlinear Analysis. Siam Review, 32, 537-578. http://dx.doi.org/10.1137/1032120
- 2. Wang, H. (2009) Periodic Solutions to Non-Autonomous Second-Order Systems. Nonlinear Analysis: Theory, Methods & Applications, 71, 1271-1275. http://dx.doi.org/10.1016/j.na.2008.11.079
- 3. Dajun, G. and Lakshmikantham, V. (1988) Nonlinear Problems in Abstract Cones.
- 4. Chu, J., Fan, N. and Torres, P.J. (2012) Periodic Solutions for Second Order Singular Damped Differential Equations. Journal of Mathematical Analysis and Applications, 388, 665-675. http://dx.doi.org/10.1016/j.jmaa.2011.09.061

































