 Advances in Pure Mathematics, 2013, 3, 381-389 http://dx.doi.org/10.4236/apm.2013.34055 Published Online July 2013 (http://www.scirp.org/journal/apm) The Integrals of Entwining Structure Yuzhuo Yuan1,2, Lihong Dong1, Zhengming Jiao1* 1Department of Mathematics, Henan Normal University, Xinxiang, China 2Department of Mathematics, Nanyang Normal University, Nanyang, China Email: *zmjiao@htu.cn Received January 31, 2013; revised March 18, 2013; accepted April 27, 2013 Copyright © 2013 Yuzhuo Yuan et al. This is an open access article distributed under the Creative Commons Attribution License, which permits unrestricted use, distribution, and reproduction in any medium, provided the original work is properly cited. ABSTRACT ,,ACIn this paper the integrals of entwining structure are discussed, where is a k-algebra, a k-coalgebra and C CA AC: a k-linear map. We prove that there exists a normalized integral :Hom,CCA of ,,AC if and only if any representation of ,,AC is injective in a functorial way as a corepresentation of . We give the dual results as well. C k k k Keywords: Entwining Structure; Integral; Forgetful Functor; Natural Transformation 1. Introduction The integrals for Hopf algebras were introduced by Larson and Sweedler [1,2]. Integrals have proven to be essential instruments in constructing invariants of surgically pre- sented 3-manifolds or 3-dimensional topological quantum field theories [3-5]. The aim of this paper is to show that some results of recent paper [6] concerning integrals and its properties for Doi-Koppinen structure hold for the more general concept known as entwining structure [7,8]. It is a structure of an algebra, a coalgebra and a k-linear map such that several compatibility conditions are satis- fied. Unlike Doi-Koppinen structure, there is no need for a background bialgebra, which is an indispensable part of the Doi-Koppinen construction. The bialgebra-free formu- lation also has a remarkable self-duality property, which essentially implies that for every statement involving co- algebra structure of an entwining structure there is a cor- responding statement involving its algebra structure. This paper is organized as follows. In Section 2, we recall definitions and give examples of entwining struc- tures and entwined modules. In Section 3, we introduce the integrals of entwining structure and analyse its pro- perties generalizing the results of [6]. Finally, in Sec- tion 4 we derive the dual form of the integrals of entwin- ing structure and its properties. 2. Preliminaries Throughout this paper, will be a field. Unless spe- cified otherwise, all modules, algebras, coalgebras, bial- gebras, tensor products and homomorphisms are over . For a -algebra , A (resp. A) will be the category of right(resp. left) -modules and -linear maps. will be a Hopf algebra over k. We omit Sweedler’s sigma-notion [9] extensively. For example, if ,C is a coalgebra, then for all we write cC cc c k 12 Definition 2.1 An entwining structure on consists of a triple . ,,AC , where is a -algebra, a -coalgebra and k C k:CA AC k, a -linear map satisfying the relations ,abca bc 11, AA cc 12 , C acacc CC ca ca ,,abAc C , where for all .ca acac Remark 2.2 Generally, we call the entwining structure in Definition 2.1 a right-right entwining structure. Unless specified otherwise, all the entwining structures men- tioned in this paper are right-right entwining structures. ,,AC ,,AC Definition 2.3 Let and : be two entwining structures, AA : be an algebra map and CC be a coalgebra map. We call *Corresponding author. C opyright © 2013 SciRes. APM  Y. Z. YUAN ET AL. 382 ,:,,fg AC ,,AC . is an entwining map if ggf Example 2.4 Let be a bialgebra, a right - comodule algebra, C a right -module coalgebra. Then Doi-Koppinen structure ,, AC [10] is an ent- wining structure. The entwining structure map is 01 .a ca:,ACcaCA If has a bijective antipode , the S is bijective with 11 10 :.a a C ac cS Example 2.5 [3] Let be a coalgebra, an alge- bra and a right -comodule. Let C , , AA AaAbab a C Bb and assume that the canonical right A-module, right - comodule map :, B AAC aa A a a CA AC can A is bijective. Let : k 11. A n ca be a -linear map given by ca canca ,,AC Then is an entwining structure. The exten- sion is called a coalgebra-Galois extension and denoted by BA C B. ,,AC is the canonical entwin- ing structure associated to C B ,,AC . Lemma 2.6 Let be a right-right entwin- ing structure. where is invertible, its inverse is : CCA , then ,,AC is a left-left ent- wining structure. i.e. ,a bcabc 11, AA cc 12 ,acc , CC ca . c ac C ac ca c C for all , where ,,ab A caa Definition 2.7 Let ,,AC be an entwining struc- ture. An ,,AC -entwined module is a -module k with a right -action and a right -coaction such that for all , C mM,aA 01 .m m Mma a A module morphism of entwining structure ,,AC is a right -module map and a right -comodule map. Generally, we denote the module category of C ,,AC C by A . Modules associatedto the entwining cture in Actor worgets the C-coactio AAMC stru Example 2.4 are Doi-Hopf module. But entwined modules associated to the entwining structure in Example 2.5 do not seem to be of Doi-Hopf type. Let :C CA F be the forgetful fun hich fn and :, C CM its right adjoint, where the structure maps on C are given by ,mcama c 12 , MCmc mcc for any ,,aAcCmM . The unit of the adjoint pair , C C is :1 C A C CF the C-coaction : M MC, therefor is A- ear and can be view linear and C-colined as a natural trans- formation between the functor 1C and C CF . C and CC is a right -module, so are od entwining mule via: bca,ba c 12 , AC bc bcc ,bcdabac d 12 , ACC bcd bcdd for any , , , ab Acd C . :C AA be the other forgetful func- to forgets the A-ac A Let C G r whichtion and :, C C N NA its left adjoint, where for C A N , NA via the structures 01 , NAna nan ,nabnab for any ,, ab An N . The unit anunit of the ad- d co , A joint pair G are :1, A AA :1, CC GGA :, , MM AA mama :, 1. NN NNA nn In particular, ,C A CACAA 3. The Integrals of the Entwining Structure s . In this section, we first present a point of view which i Copyright © 2013 SciRes. APM  Y. Z. YUAN ET AL. 383 essential for the rest of paper: the existence of an integral on a Hopf algebra is a necessary and sufficient criterion for constructing a natural transformation between two functors. Lemma 3.1 [6] Let be a finite dimension Hopf algebra over k, is its dual. There exists a right integral o n such that ,1H hh for all h and ly if :H ifon k is right - comap, where k has l right - odule mthe trivia co s this result as follows. - moule structure. □ Doi [11] generalize Definition 3.2 Let be a -comodule algebra. A map : A is called an integral if is right -linear. is called a total integral if additionally 11 A . rion T he r the existence of a total integral is gi crite fo ven by the theorem following. Theorem 3.3 [11] Let be a right -comodule alqugebra. The following are eivalent 1) There exists a total integral : A ; 2) Any Hopf module M is injective as right H-comodule. i.e. the righton : H-coacti MH splits in the category ; 3) : A AH splits in the category . eorem of Doi s. Thecan be restated as follow th Theorem 3.4 [6] Let be a right -comodule algebra. The following are equivalent 1) There exists a total integral : A rmation 2) There exists a natural transfo :1 H AA FHFF that splits :1 H A AA FHF : ; 3) A AH splits in the category . rk 3.5 1) Tstilllid le Rem he above theorem is vaa aving aside the normalizing condition 11 A . More exactly, there exists an integral : A d only if there exists : if an 1 H A FHF . In particular, if A F k,ight integral : we obtain that there exists a r k on if and only if there exists a natural tron . ansformati :1 H HF Further- more, 11 k if and only if splits :1 H F forgetful functo . This is equivalent tohe fact : HH k F is separable. 2) Let t that the r be a right -comodule ve algebra. The rsion of Theorem 3.4 fore category th A is still true. In this case the H-colinear split of : M MH associated to a right total integral : A is given by the formula :, M HM 01 .Smh We will now give the definition of integral of entwine- in Mmh m g structure arising from Theorem 2.4 and the definition of integral of Doi-Koppinen structure in [6]. Definition 3.6 Let ,,AC be an entwining struc- tu :HC re. A k-linear map om,CA is called an integral of ,,AC if 12 21 ,cdcddc for all ,cdC . An integral :Hom,CCA is rmalized called no if for all cC 1.cc c 21 A Remark 3.7 If Doi-Koppinen structure ,, AC in Example 2.4 takes place of the entwining structure in the above definition and obviously entwining structure map is 01 :, .CA ACcaaca Definition 3.6 is just the definition of integral of Doi- K e that the existence of an integral oppinen structure. We shall now prov :Hom,CCA permits the deformation of k- n two entwined modules until it becomes a C-colinear map. Propositi 3.8 Let linear map betwee on ,,CA be an entwining st C A an . Suppose that there exi ructure. , C MN d :uNM a k- linear mapsts :Hom,CCA . Then 1) For all nN , the map 010 01 unn un is right C-colinear; :,N Muun 2) If Hom ,CCA is a normalized integral an : d : Mism in C N is a morphA which is a k-splresp. a k-split surjehen it injection (ction), t has a C-colinear retraction(esp. a section). oof. 1) For nNr Pr , we have 010 01 010 0 01211 011012 01 0001 001 01 01 . M M N un n un unnunun unnunn unn unn unnuidn un 2) Let :uN M be a k-linear retraction(resp. se ction) of . Then spn) of :uN M is a right C-colinear retraction(re. a sectio . Then, for mM Copyright © 2013 SciRes. APM  Y. Z. YUAN ET AL. 384 01 1 0 021, 0 01 00 1 ufm fm ufm ufmm ufm mmm m hence is a right Cof uf m u -colinear retraction . of On the other handf :uN M is a section , i , then for nN 01 01 021. fu 0 01 0 01 fu nn un funn fun nnn n i.e. is a right C-colinear section of n u . □ le Dinition 3.9 [11] A right C-comodu ef is inj called ective, if for any k-split onomorhism:iUV in C and for any C-colinear map : m UM, there exists C-colinear map : VM sut ch tha if. 1] A right CLemma 3.10 [1-comodule is in- jective, if : M MC splits in C , . there exists a C-colin : M i.e ear map CM such that MM di . □ We will prove now the 3.4 for version of Theorem entwining modules which have inverse entwining struc- ture map. Parts of the following theorem are closely related to the ideas presented in [6]. Theorem 3.11 Let ,,AC be an entwining struc- ture, where is invits inverse is ertible, . The following stateents are equivalent: 1) There exists a normalized integral m :Hom,CCA; nsf 2) The natural traormation :1 C A C AA FCF 3) The right C-co splits; n on actio C, : r AC CACC spl onsef the equivalent co C its in . ,nditions ho CC quently if one o lds, any entwined module is injective as a right C- comodule. Proof. 12 Let :Hom,CCA be a no malized integral. r- construct a naW toe havetural trans- formation that splits . Let C A M and : M uMCM , M umc c inear retraction of m be the k-l : M MC cm , for all c given by M umc ,CmM. We define M u , i.e. for all , cC m M M , :,CM M 01 Mmc mcm It follows from Proposition 3.8 that the map . is a right C-colinear retraction of . It remains to prove that is a naturrans- formation. Let : al t MN beo a mrC phism in A . We have to prove that . MN C fid Since is right -linear, we have 01 01 , fm cm fm cm M fmc 01 01 . NC N idm c cfm fmcfm fm cm i.e. is a natural transformation that splits . 23 C A Assume that for any M C-caction splits in C . In particular, , the o r: AC CACC splits in : AC C. Let CC CA be a right C-colinear retraction of r AC . Using the naturality of AC , we will prove that AC is also left C-coliner, where a C and CC are left C-comodules via: 12 . l ac c acd cacd 12 , la c Let V be a k-module and C A M . Then C VM A he structures a the es of via trising from on as follows , .m vmaid VMV M v Using the naturality of , we shall prove that . VMV M id Let vV and :, . VV MVMgmvm From the naturality of we obtain that . C VM VMV g id Hence . VM VM M VM vmc mcvmc idv m c In particular, let , ACVC, then Copyright © 2013 SciRes. APM 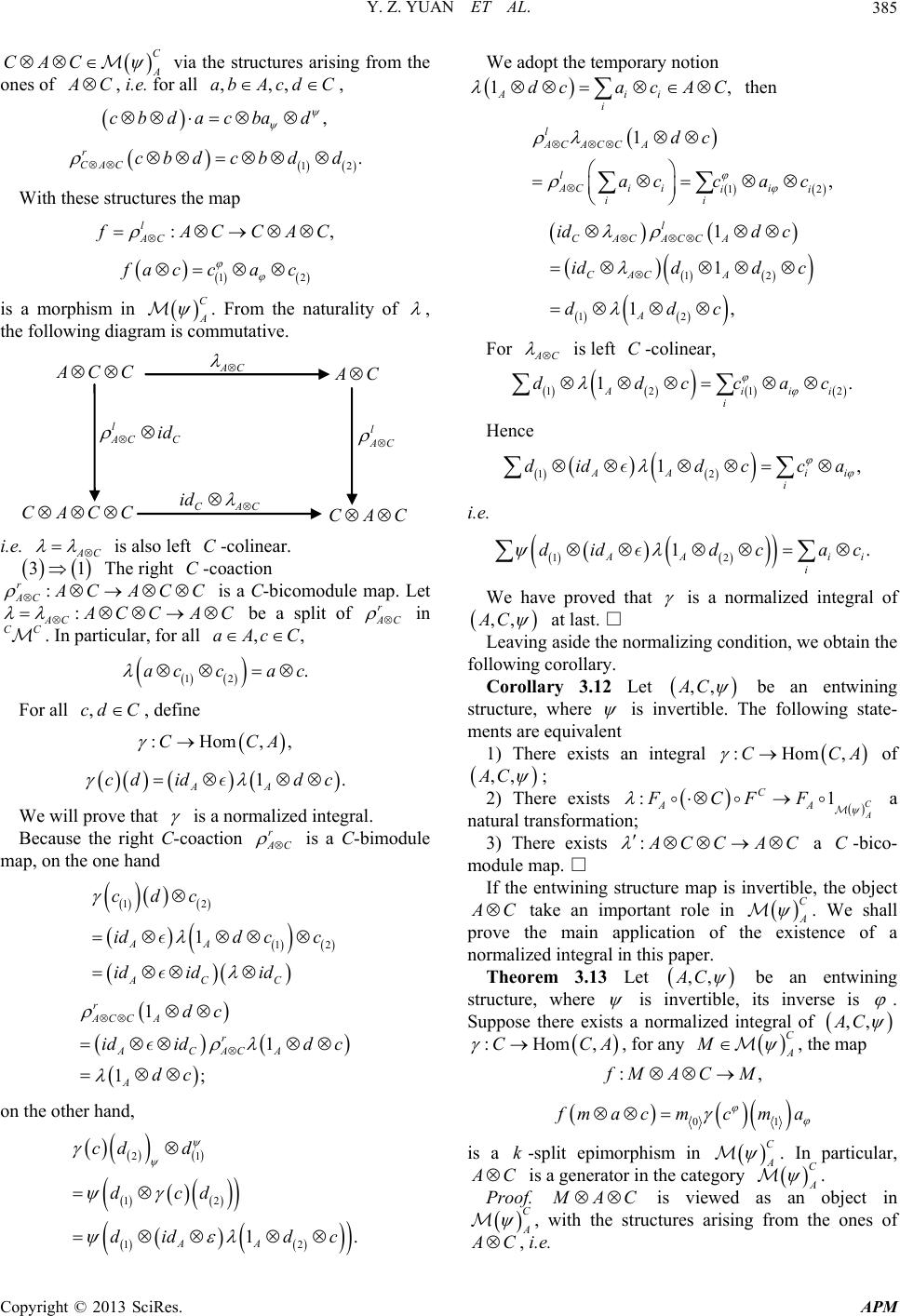 Y. Z. YUAN ET AL. 385 AC C A C es arisin via the structurg from the ones of C, i.e. fo c ,cba d r all , , , abAcd C, bda 12 .bd thes , CA Ccbd e structures the map AC rc d With : l ACC AC 12 ac c rphism in C A a c is a mo . From the naturality of , the following diagramutative. m is com CC AC C l AC C id l AC CACC CAC id CAC i.e. AC is also left C-colinear. -c : r AC 3he right Coaction 1 T CAC is a C-bicoCmodule map. Let AC : CC AC be a split of r AC in ,,AcC CC . In particular, for all a ac , cd C, define 12 .c c m,,CA 1. AA c a For all We will :HoC cd id prove that d is a normalized integral. -odule Because the right Ccoaction r AC is a C-bim ma on the othe p, on the one hand 12 1 AA AC cdc id d id id 1 rdc 12 C c c id 1 ACA d c 12 1. d dc We adopt the temporary notion 1, Aii i dca c AC then 2 1 , l AC ACCA lii dc accac 1; ACC A r AC A id id dc r hand, cd 21 12 AA dc d did 1 AC i ii ii 12 12 1 1 1, l CACACCA CAC A A id iddd c ddc For AC d c is left C-colinear, 1212 1. Ai ii i ddccac Hence 12 1, ii i diddc ca AA i.e. 1. AA ii i diddc ac 12 We have proved that is a normalized integral of ,,AC at last. □ ving aside the normalizing condition, we obtain the ing corollary. Corollary 3.12 Let Lea follow ,,AC be an entwining structure, where is invertible. The following state- m 1) The integral ents are equivalent re exists an :Hom,CCA of ,,AC ; 2) There exists :1 C C AA FCFF a na tural transformation; 3) There exists: CC AC a C-bico- module map. □ If the entwining structure map ect is invertible, the obj take an important role in C A C . We shall prplication of the existence of a is paper. Let ove the main ap normalized integral in th ,,ACTheorem 3.13 be an entwining structure, where is invertible, its inverse is . Suppose there exists a normalized integral of ,,AC Hom,CA, for any C M:C A the map :, , MACM 01 macmam c is a k-split epimorphism in C A . In p, articular is a generatotegory r in the ca C A C Proof. . AC as an C A is viewed object in , with the structures arising from the ones of , i.e. C Copyright © 2013 SciRes. APM  Y. Z. YUAN ET AL. 386 mbca ,mba c 1 c 2 .mbc mbc b fo ,AcCm r all ,,a M . First we shall prove that is a k-split surjection. Let 01 , 1, A : MMACgmm m r all . Thenfo mM is right C-colinear. For mM, weve ha mm m 01 00 1Am m 1 01 021 01 , fgm fm m m mmm i.e. is a right c-colinear section of . For ,c Cm M , weve ,,ab A ha 01 fmbca 01 , mba cmc mcmbafm mba bca i.e. is right -r linear. It remains to ove thatp is ght C-colinearalso ri. In fact, 101 , 12 12 2 CMAC C idm b c idmb cc fm b cc mcmbc 01 00 1 02 02 01 1 01 01 ( () M Mmc m mcm mcmb mcm mc mb mcmb mcmb 01 1 1 2 12 12 , fm b c b bm m bm c c c i.e. is right C-colinear. Hence, is an epimorphism in C A and has a rig C-colinear section. Taking a k-free presentation of ht in the category of k-modules 0, I kM we obtain an epimorphism in C A 0 IIg ACk ACM where AC fII . Hence C is a gene- rator in C A . □ Remark 3.14 An important application of integrals in finite dimension Hopf algebra is Maschke theorem. It finds the condition of finite dimension Hopf algebras to be semisimple. [12-14] have studied the relation between the integral of Doi-Koppinen structure and Maschke theorem. The integrals of entwining structure we study alwith Maschke theorem, the rer. 4. n e has the property of self- duality, we will get some dual results of Section 3. In nition of integrals of the tly, we have the lemma as follows Let here so have a tend relation aders can efer to the reference [3,15] The Coitegrals of Entwining Structure Because the entwining structur order to give the dual defi entwining structure convenien ,,ACLemma 4.1 be an ent The fol: 1) The a nor wining structure. lowing are equivalent re existsmalized integral :Hom,CCA; 2) There exists a k-linear map :CCA such that for all ,.cd C 12 21 ,cddc dc 12 1. A cc c Proof. Let dc cd . The proof of the lemma is obvious. □ Definition 4.2 Let ,,AC be an entwining structure. A k-linear map :CAA is called an cointegral of ,,AC if for any 12 ,cCccc AA , caa 1212 .cc c A cointegral is called normalized if 12 1. A c c have the dualProposition 3.8, .11 and Them 3.13. Proposition 4.3 Let c We shall results of Theorem 3eor ,,AC be a entwing struc- ture. , C A M A Nin , :uN M a k-linear map. Suppose that there exists :CAA, then: Copyright © 2013 SciRes. APM  Y. Z. YUAN ET AL. 387 1) For all nN, the map 11 :uN n is 12 0 , Mu unn right -linear; 2) Let :CAA is lized cointegral and : a norma MN phism in is a m C or A which is a k-split injection (resp. a k-split surection), then j has a -linear retraction. a section). Proof. It is just the dual of Proposition 3.8. □ Let (resp Theorem 4.4 ,,AC be an entwinitruc- ture, where ng s is invertible, its inverse is . The fo e 1) There exists a normalized cointegral :CAA llowing statemnts are equivalent: ; splits; 2) The natural transformation :1 C CC A GAGG A 3) The right -action on CA, in AA . le equivalent ns hontwine is projective as a right C-comodule. :CAA : r CACAA CA splits nconditioConseque one of th lds, any eed modul ty, if Proof. 12 Let be a norma- in to construct a natural trans- lized cotegral. We have formation that splits . Let C A M and for , , :1 any , MA cCu MMm the k-linear section of : M m AuMm be AM . We d - fine e M u , i.e. :, MM MA 12 01 1 Mmm mm w ma here mM. It follows from Proposition 4.3 that the p is a rht ig -linear section of . It remains to prove that is a natural transformation. Let : MN be a C A morphism in . We at . have to prove th MN A id f For any mM, using that is right C-colinear, we have 1 12 1 12 1 1 , A fid m fm m 12 01 01 0 M A m fidmmm fm m m 12 01 1 ,fm m m i.e. 12 01 1 Nfm fm fmfm is a nasformation that splits tural tran . 3 Assu C A 2me that for any M right , -action splits in A. In particular, : CACAA CA splits in . Let :CA CAAA be a right CA -linear se CA ction of . Using the naturality of CA , we will prove that-linear, where CA CA is also left and CAA are left -modules via: ,ac bcab ,acbb c abb where , , , .abbAc C First let V be a k-module and C A M . Then C A VM , via the structures arising from the ones of , i.e. , . VMV M vmavma id Using the naturality of , we prove that .id VMV M vV Let and :, vv . MVMg From e naturality of m m th v we obtain that . VA M gid VM V g Hence VM m VM VVA M MVM v . mgid m vmidm In particular, let , CAVA , then C A A AC a the st viructures arising from the ones of ,bcbabcba 12 r AC Abcbbcb c fo . r all , , , abbAc C . ith these structureWs the map :, l CA AC AA C c bca a b is a morphism in C A . From the naturality of , the following diagram is commutative. CA CAA AC l CA CAACA id CAA l CA A id Copyright © 2013 SciRes. APM  Y. Z. YUAN ET AL. 388 i.e. 1212 .ccaacc Then we obtained that CA is also left -linear. right 31 : The -action A A is a is a normalized cointegral of : r CA CAC -bimodule m p. Let a ,,AC : CCA ACAA be a split of CA r in . all ,,aAcC AA In particular, for c aca. , we define 1. AA A c t id m finally. □ Leaving aside the normalizing condition, we obtain the following corollary Corollary 4.5 Let ,,AC be an entwining struc- ture, where For all cC :, CAAcidi d We will prove tha is 1 11. AA A AA c c ,,cCaA on the one hand A j ca jjj c a b on the other hand, 1 1 A A A a c a we haveed a normalized cointegral. 12 ccmidid is invertible. The following statements are equivalent: 1) There exists a cointegral :CAA ; 2) There exists :1 C A CC A GGAG a natural transformation; 3) There exists :CACAA id idmc For any 12 AA AA A AA A acc mid idca mid ididid id m 1 ca 1, A AA id aididc j j j b , then 12 Let 1A c acc ; AAA j j mi d aidid jj j ca a b 12 1 AA AAAA AAAA AA AA AA A AA jjj j AA jjj j j cca idc a idmididid c id idid idmid ididc a id idca idid ac ididac a b ididcaab ca jj j j ab id m , jj j ca a b us is a -bimodule map. Hence an -bi- module map. □ Theorem 4.6 Let ,,AC be an entwining struc- ture, where is invertible, its inverse is . Suppose there exists a normalized cointegral of ,,AC :CAA, for any C A M , the map :, MMCA 12 m 01 21 .fm mmm for all ,,aAcCmM is a k-split monom in Corphism A . In particular, CA is a cogenerator in the category C A . Proof. just the dual It is of Theorem 3.13. □ ents EFERENCES . E. Sweedler, “An Asve form for Hopf Algebra , Vol. 91, No. 1 0 5. Acknowledgem This work was partially supported by the Natural Science Foundation of Henan Province (102300410049). R [1] M R. G. Larson andsociati Or- thogonal Bilinears,” American Journal of Mathematics, 1969, pp. 75-94. doi:10.2307/237327 1969, pp 323-335. doi:10.2307/1970672 [2] M. E. Sweedler, “Integrals for Hopf Algebra,” Annals of Mathematics Second Series, Vol. 89, No. 2, . [3] T. Kerler, “Bridged Links and Tang Presentations of Cobordism Categories,” Advances inthematics, Vol. 141, No. 2, 1999, pp. 207-281. doi:10.1006/aima.1998.1772 le Ma Kuperberg, “Non-Involuntory Manifo e Mat 96, pp. 83-129. [4] G. Hopf Algebras and 3- ld Invariants,” Dukhematical Journal, Vol. 84, No. 1, 19 doi:10.1215/S0012-7094-96-08403-3 V. Turaev, “Quantum Invariants of Knots and 3-Mani-[5] erlin, 1994. ru, “Integral, Quantum Galois ol. 247, folds,” Walter de Gruyter, B [6] C. Menini and G. Mimita Extensions and the Affineness Criterion for Quantum Yetter-Drinfeld Modules,” Journal of Algebra, V No. 2, 2002, pp. 467-508. doi:10.1006/jabr.2001.8899 [7] T. Brezinski and S. Majid, “Coalgebra Bundles,” Commu- Copyright © 2013 SciRes. APM  Y. Z. YUAN ET AL. Copyright © 2013 SciRes. APM 389 nications in Ml. 191, No. 2, 1998athematical Physics, Vo, pp. 467-492. doi:10.1007/s002200050274 [8] T. Brezinski, “On Modules Associated to Coalgebra Ga- lois Extensions,” Journal of Algebra, Vol. 215, No. 1, 1999, pp. 290-317. doi:10.1006/jabr.1998.7738 [9] M. E. Sweedler, “Hopf Algebras,” Benjamin, New York, 1969. [10] Y. Doi, “Unifying Hopf Modules,” Journal of Algebra, Vol. 153, No. 2, 1992, pp. 373-385. doi:10.1016/0021-8693(92)90160-N [11] Y. Doi, “Algebras with Total Inte in Algebra, Vol. 13, No. 10, 1 grals,” Communications 985, pp. 2137-2159. ican Mathematical Society [12] S. Caenepeel, G. Militaru and S. Zhu, “Doi-Hopf Modules, Yetter-Drinfel’d Modules and Frobenius Type Proper- ties,” Transactions of the Amer, Vol. 349, No. 11, 1997, pp. 4311-4342. doi:10.1090/S0002-9947-97-02004-7 [13] S. Caenepeel, G. Militaru and S. Zhu, “A Maschke Type Theorem for Doi-Hopf Modules and Applications,” Jour- nal of Algebra, Vol. 187, No. 2, 1997, pp. 388-412. doi:10.1006/jabr.1996.6794 [14] S. Caenepeel, G. Militaru and S. Zhu, “Separable Func- tors for the Category of Doi-Hopf Modules, Applica- tions,” Advances in Mathematics, Vol. 145, No. 2, 1999, pp. 239-290. doi:10.1006/aima.1998.1817 [15] T. Brzeziński, S. Caenepeel, G. Militaru and S. Zhu, “Fro- benius and Maschke Type Theorems for Doi-Hopf Mod- ules and Entwined Modules Revisited: A Unified Ap- proach,” In: A. Granja, J. Alonso Hermida and A. Verscho- ren, Eds., Marcel Dekker, New York, 2001.
|