N. A. AL-ZAID ET AL. 163
(a) (b)
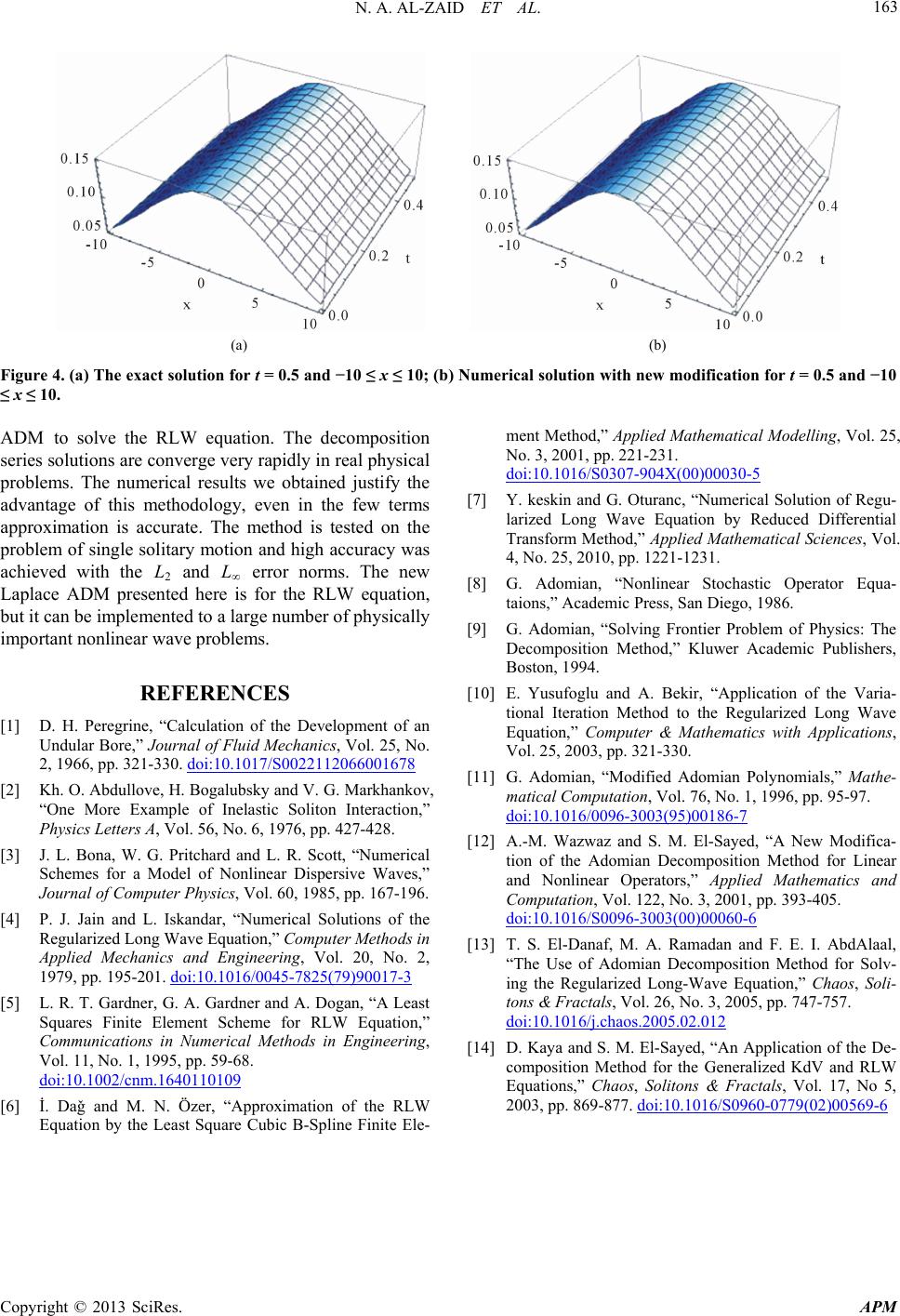
Figure 4. (a) The exact solution for t = 0.5 and −10 ≤ x ≤ 10; (b) Numerical solution with new modification for t = 0.5 and −10
≤ x ≤ 10.
ADM to solve the RLW equation. The decomposition
series solutions are converge very rapidly in real physical
problems. The numerical results we obtained justify the
advantage of this methodology, even in the few terms
approximation is accurate. The method is tested on the
problem of single solitary motion and high accuracy was
achieved with the L2 and L∞ error norms. The new
Laplace ADM presented here is for the RLW equation,
but it can be implemented to a large number of physically
important nonlinear wave problems.
REFERENCES
[1] D. H. Peregrine, “Calculation of the Development of an
Undular Bore,” Journal of Fluid Mechanics, Vol. 25, No.
2, 1966, pp. 321-330. doi:10.1017/S0022112066001678
[2] Kh. O. Abdullove, H. Bogalubsky and V. G. Markhankov,
“One More Example of Inelastic Soliton Interaction,”
Physics Letters A, Vol. 56, No. 6, 1976, pp. 427-428.
[3] J. L. Bona, W. G. Pritchard and L. R. Scott, “Numerical
Schemes for a Model of Nonlinear Dispersive Waves,”
Journal of Computer Physics, Vol. 60, 1985, pp. 167-196.
[4] P. J. Jain and L. Iskandar, “Numerical Solutions of the
Regularized Long Wave Equation,” Computer Methods in
Applied Mechanics and Engineering, Vol. 20, No. 2,
1979, pp. 195-201. doi:10.1016/0045-7825(79)90017-3
[5] L. R. T. Gardner, G. A. Gardner and A. Dogan, “A Least
Squares Finite Element Scheme for RLW Equation,”
Communications in Numerical Methods in Engineering,
Vol. 11, No. 1, 1995, pp. 59-68.
doi:10.1002/cnm.1640110109
[6] İ. Daǧ and M. N. Özer, “Approximation of the RLW
Equation by the Least Square Cubic B-Spline Finite Ele-
ment Method,” Applied Mathematical Modelling, Vol. 25,
No. 3, 2001, pp. 221-231.
doi:10.1016/S0307-904X(00)00030-5
[7] Y. keskin and G. Oturanc, “Numerical Solution of Regu-
larized Long Wave Equation by Reduced Differential
Transform Method,” Applied Mathematical Sciences, Vol.
4, No. 25, 2010, pp. 1221-1231.
[8] G. Adomian, “Nonlinear Stochastic Operator Equa-
taions,” Academic Press, San Diego, 1986.
[9] G. Adomian, “Solving Frontier Problem of Physics: The
Decomposition Method,” Kluwer Academic Publishers,
Boston, 1994.
[10] E. Yusufoglu and A. Bekir, “Application of the Varia-
tional Iteration Method to the Regularized Long Wave
Equation,” Computer & Mathematics with Applications,
Vol. 25, 2003, pp. 321-330.
[11] G. Adomian, “Modified Adomian Polynomials,” Mathe-
matical Computation, Vol. 76, No. 1, 1996, pp. 95-97.
doi:10.1016/0096-3003(95)00186-7
[12] A.-M. Wazwaz and S. M. El-Sayed, “A New Modifica-
tion of the Adomian Decomposition Method for Linear
and Nonlinear Operators,” Applied Mathematics and
Computation, Vol. 122, No. 3, 2001, pp. 393-405.
doi:10.1016/S0096-3003(00)00060-6
[13] T. S. El-Danaf, M. A. Ramadan and F. E. I. AbdAlaal,
“The Use of Adomian Decomposition Method for Solv-
ing the Regularized Long-Wave Equation,” Chaos, Soli-
tons & Fractals, Vol. 26, No. 3, 2005, pp. 747-757.
doi:10.1016/j.chaos.2005.02.012
[14] D. Kaya and S. M. El-Sayed, “An Application of the De-
composition Method for the Generalized KdV and RLW
Equations,” Chaos, Solitons & Fractals, Vol. 17, No 5,
2003, pp. 869-877. doi:10.1016/S0960-0779(02)00569-6
Copyright © 2013 SciRes. APM