Classification of All Single Traveling Wave Solutions to (3 + 1)-Dimensional Breaking Soliton Equation ()

1. Introduction
For the past decades, to deal with nonlinear partial differential equations (PDEs), many methods have been developed. These methods have been widely applied to many PDEs to obtain the exact solutions. Recently, a method named the complete discrimination system for polynomial method has been proposed by Liu [1] -[5] . By Liu’s method, we can obtain the classification of single traveling wave solutions to some PDEs. For the PDE being considered, we take the traveling wave transformation and integrate it. The PDE can be directly reduced to ordinary differential equation (ODE) which can be turned into the integral form as follows:
 (1)
(1)
where  is a n-th order polynomial. By Liu’s method, we can obtain the classification of all solutions to the Equation (1).
is a n-th order polynomial. By Liu’s method, we can obtain the classification of all solutions to the Equation (1).
In this paper, we take into account (3 + 1)-dimensional breaking soliton equation, and it reads as
 (2)
(2)
where a, b, c, d and e are arbitrary constants.
Equation (2) was originally proposed by Lin [6] to study the Virasoro-type symmetry algebra. Li [7] got some solitary wave solutions and periodic wave solutions of Equation (2) by using a simple transformation relation and solving the ordinary differential equation. Shi [8] gave some exact solutions of Equation (2) by turning it into KdV equation though introducing a simple transformation, and so on.
2. Classification
For Equation (2), we take the traveling wave transformation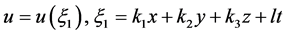 , and can obtain the corresponding reduced ODE as follow
, and can obtain the corresponding reduced ODE as follow
 (3)
(3)
Integrating Equation (3) with respect to  once , we simplify it and yield
once , we simplify it and yield
 (4)
(4)
where  is an integral constant.
is an integral constant.
Let
 (5)
(5)
Then we have
 (6)
(6)
Or equivalently
 (7)
(7)
Integrating the Equation (7) once with respect to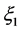 , we get
, we get
 (8)
(8)
where  is an integral constant. For purpose of use the complete discrimination system for the third order polynomial, we have the following solving process.
is an integral constant. For purpose of use the complete discrimination system for the third order polynomial, we have the following solving process.
Let
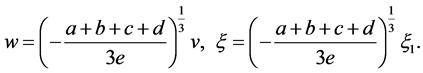 (9)
(9)
Then Equation (8) becomes
 (10)
(10)
where ,
, 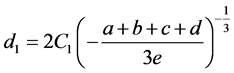 ,
,  and
and  is a function of
is a function of . The integral form of Equation (8) is
. The integral form of Equation (8) is
 (11)
(11)
Denote
 (12)
(12)
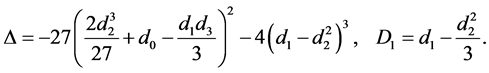 (13)
(13)
According to the complete discrimination system, we give the corresponding single traveling wave solutions to Equation (2).
Case 1.  has a double real root and a simple real root. Then we have
has a double real root and a simple real root. Then we have
 (14)
(14)
When , the solutions to Equation (8) are as follows
, the solutions to Equation (8) are as follows
 (15)
(15)
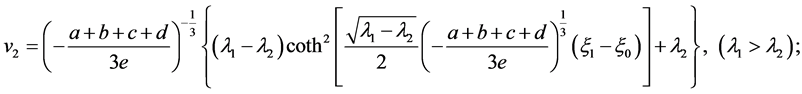 (16)
(16)
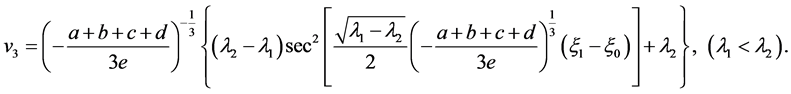 (17)
(17)
The corresponding solutions to Equation (2) are
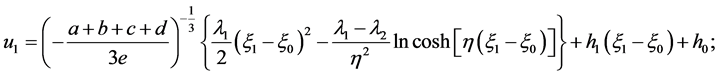 (18)
(18)
 (19)
(19)
 (20)
(20)
Case 2. 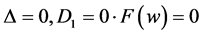 has a triple root. Then we have
has a triple root. Then we have
 (21)
(21)
The corresponding solution to Equation (2) is
 (22)
(22)
Case 3.  has three different real roots. Then we have
has three different real roots. Then we have
 (23)
(23)
When , the corresponding solutions to Equation (2) is
, the corresponding solutions to Equation (2) is
 (24)
(24)
When , the corresponding solutions to Equation (2) is
, the corresponding solutions to Equation (2) is
 (25)
(25)
where .
.
Case 4.  has only a real root. Then we have
has only a real root. Then we have
 (26)
(26)
When , the corresponding solutions to Equation (2) is
, the corresponding solutions to Equation (2) is
 (27)
(27)
where ,
,  are integral constants in Equations (18)-(20), (22), (24), (25) and (27).
are integral constants in Equations (18)-(20), (22), (24), (25) and (27).
In Equations (24) (25) and (27), we give the expression of some signals as follow
 (28)
(28)
 (29)
(29)
The solutions 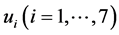 are all possible exact traveling wave solutions to Equation (2). We can see it is easy to write the corresponding solutions to (3 + 1)-dimensional breaking soliton equation.
are all possible exact traveling wave solutions to Equation (2). We can see it is easy to write the corresponding solutions to (3 + 1)-dimensional breaking soliton equation.
3. Conclusion
From the descriptions above, we use the complete discrimination system for polynomial and direct integral method to obtain all possible traveling wave solutions to (3 + 1)-dimensional breaking soliton equation. This method is direct and effective. With the same method, some of other equations can be dealt with.
Acknowledgements
I would like to thank the referees for their valuable suggestions.