1. Introduction
In this paper,  is assumed to be a filtered probability space where
is assumed to be a filtered probability space where 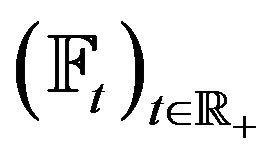 is a filtration satisfying
is a filtration satisfying  for all
for all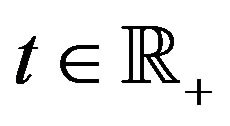 , the usual condition of right continuity and completeness. The random movement of
, the usual condition of right continuity and completeness. The random movement of  risky assets in the market is modeled via cadlag, nonnegative stochastic processes
risky assets in the market is modeled via cadlag, nonnegative stochastic processes , where
, where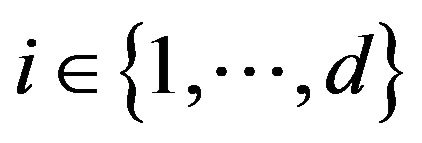 . We assume that all wealth processes are discounted by another special asset which is considered a baseline. In the market described above, economic agents can trade in order to reallocate their wealth.
. We assume that all wealth processes are discounted by another special asset which is considered a baseline. In the market described above, economic agents can trade in order to reallocate their wealth.
Consider a simple predictable process
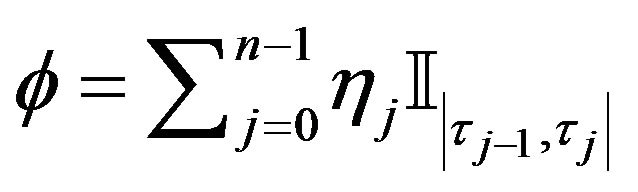 .
.
where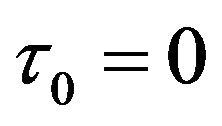 , and for all
, and for all ,
, 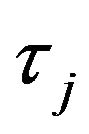 is a finite stopping time and
is a finite stopping time and  is
is  -measurable.
-measurable.
Each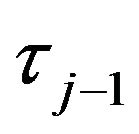 ,
, 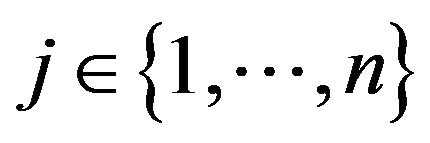 , is an instance when some give economic agent may trade in the market, then,
, is an instance when some give economic agent may trade in the market, then,  is the number of unit from the ith risky assets that the agent will hold in the trading interval
is the number of unit from the ith risky assets that the agent will hold in the trading interval . This form of trading is called simple, as it comprises of finite number of buy-and-hold strategies, in contrast to continuous trading where one is able to change the position of the assets in a continuous fashion. The last form of trading is only theoretical value, since it cannot be implemented in reality, even if one ignores market frictions.
. This form of trading is called simple, as it comprises of finite number of buy-and-hold strategies, in contrast to continuous trading where one is able to change the position of the assets in a continuous fashion. The last form of trading is only theoretical value, since it cannot be implemented in reality, even if one ignores market frictions.
Starting from initial capital  and following the strategy described by the simple predictable process
and following the strategy described by the simple predictable process
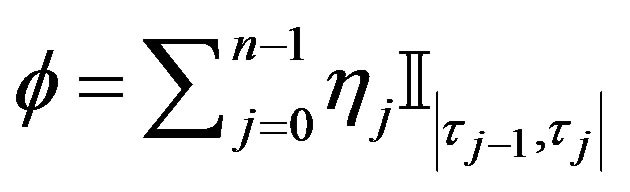 , the agent’s discounted process is given by
, the agent’s discounted process is given by
 .
.
where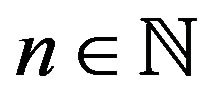 ,
, 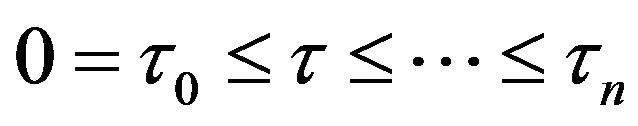 are a.s. finite stoping times with respect to
are a.s. finite stoping times with respect to 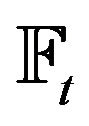 and the
and the  are
are 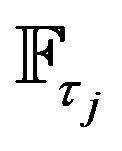 -measurable real random variables. Note that the trader is allowed to trade on an infinite time horizon, because we do not restrict to bounded stoping times for the re-allocation of the capital. Of course trading on a finite time horizon [0, T] is covered by switching to the process
-measurable real random variables. Note that the trader is allowed to trade on an infinite time horizon, because we do not restrict to bounded stoping times for the re-allocation of the capital. Of course trading on a finite time horizon [0, T] is covered by switching to the process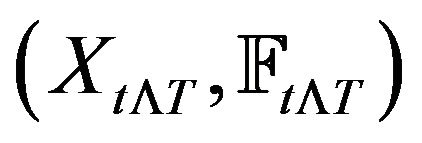 .
.
Theorem 1.1. [1,2] A real valued, cadlag, adapted process  the following are equivalent:
the following are equivalent:
1) X is a good integrator.
2) X may be decomposed as , where
, where  is a local martingale and
is a local martingale and  is an adapted process of finite variation.
is an adapted process of finite variation.
Defination 1.1. [1,3] A real valued, cadlag, adapted process  allows for A Free Lunch With Vanishing Risk for simple integrands if there is a sequence
allows for A Free Lunch With Vanishing Risk for simple integrands if there is a sequence  of simple integrands such that for
of simple integrands such that for ,
,
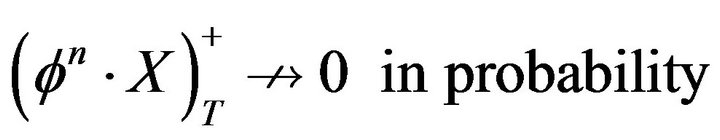 .
.
and

In contrast, X therefore admits No Free Lunch With Vanishing Risk (NFLVR) for simple integrands if for every sequence  satisfying (VR) we have
satisfying (VR) we have
(NFL)  in probability.
in probability.
A free lunch with vanishing risk (FLVR) for simple integrands indicates that S allows for a sequence of trading schemes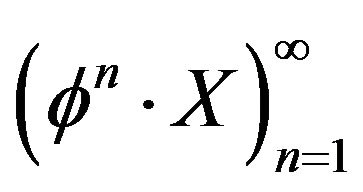 , each
, each  involving only finitely many rebalancing of the portfolio, such that the losses tend to Zero in the sense that of (VR) ,while the terminal gains (FL) remain substantial as n goes to infinity. It is important to note that the condition (VR) of vanishing risk pertains the maximal losses of the trading strategy
involving only finitely many rebalancing of the portfolio, such that the losses tend to Zero in the sense that of (VR) ,while the terminal gains (FL) remain substantial as n goes to infinity. It is important to note that the condition (VR) of vanishing risk pertains the maximal losses of the trading strategy 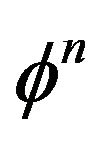 during the entire interval [0,T]: if the left hand side of (VR) equals
during the entire interval [0,T]: if the left hand side of (VR) equals  this implies that, with probability one, the strategy
this implies that, with probability one, the strategy  never, i.e. for not
never, i.e. for not , cause an accumulated loss of more than
, cause an accumulated loss of more than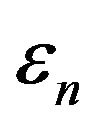 .
.
Resently, it has been argued that existence of an Equivalent Martingale Measure(EMM) is not necessary for viability of the market; to see this effect, see [4-6]. In [7], the concept of strictly positive supermartingale deflator which is weaker than the existence of an EMM, that allows for consistent theory to be developed.In this paper, we investigate the relation between the no free lunch with vanishing risk property for simple integands and the semimartingale property.
Theorem 1.2. [1,8] Let 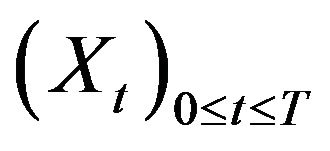 be a real-valued, cadlag, locally bounded process based on and adepted to a filtered probability space
be a real-valued, cadlag, locally bounded process based on and adepted to a filtered probability space . If S satisfies the condition of no free lunch with vanishing risk (NFLVR) for simple integrands then S is a semimartingale.
. If S satisfies the condition of no free lunch with vanishing risk (NFLVR) for simple integrands then S is a semimartingale.
Theorem 1.3. For a locally bounded, adopted, cadlag process X the following are equivalent 1) X satisfies NFLVR + LI(little Investment)
2) X is a classical semimartingale.
Theorem 1.4. For an adapted cadlag process X the following are equivalent.
1) For all sequences  of simple predictable processesa)
of simple predictable processesa) 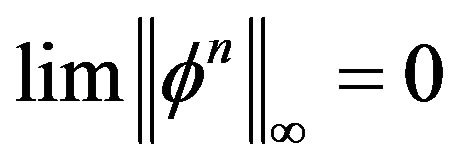
b) 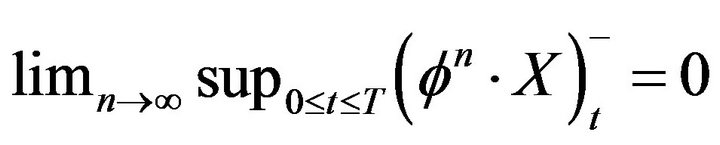
together imply 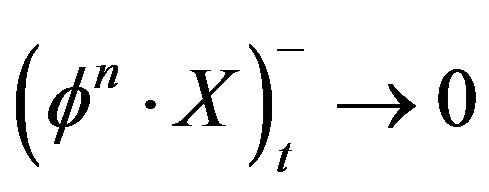 in probability.
in probability.
2) X is a classical semimartingale.
Proposition 1.5. Let  be cadlag and adapted, with X0 and such that
be cadlag and adapted, with X0 and such that 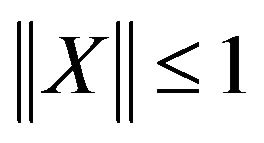 and X satisfies NFLVR + LI For all
and X satisfies NFLVR + LI For all 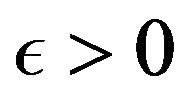 there is
there is  and a sequence of stopping times
and a sequence of stopping times  such that, for all n 1)
such that, for all n 1)  takes values in
takes values in .
.
2) .
.
3) The stopped processes 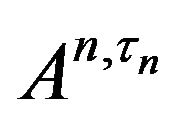 and
and 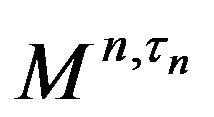 satisfyfor all n,
satisfyfor all n, 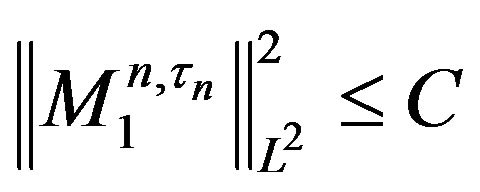 and
and
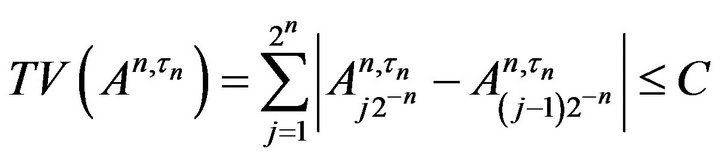
Lemma 1.6. Under the assumptions as in the proposition above with
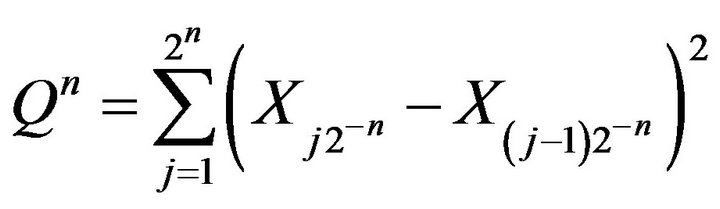 the sequence
the sequence  is bounded in probability.
is bounded in probability.
Proof. For all n, let

a simple predictable process, then 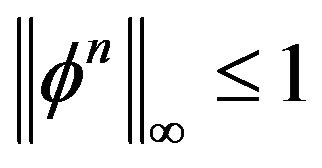 since
since 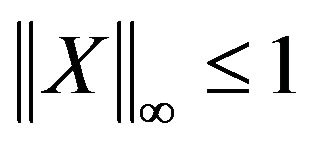
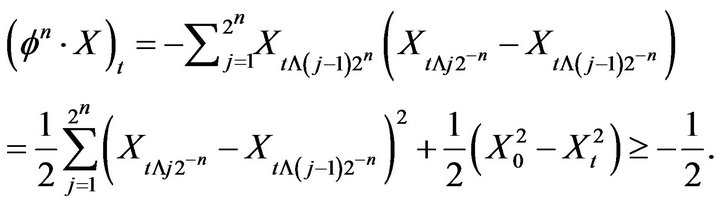
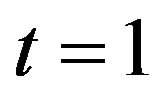
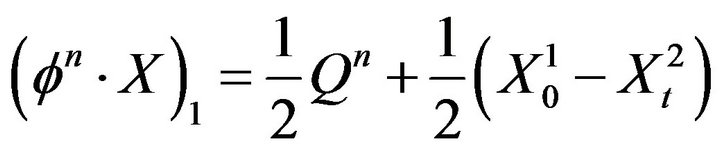
since X satisfies NFLVR + LI,  is bounded in
is bounded in .
. 
For  define a sequence of stopping times
define a sequence of stopping times
 .
.
Given 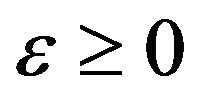 there is
there is 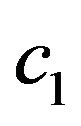 such that
such that
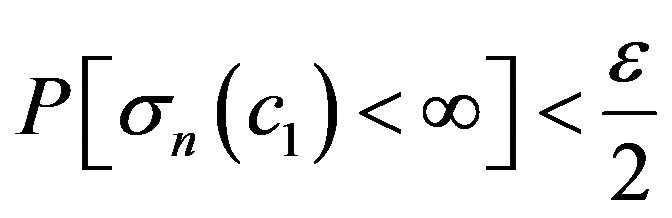
Lemma 1.7. Under the same assumptions as in Proposition 1.5 the stopped martingales 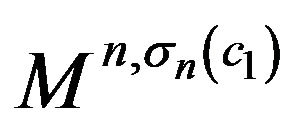 satisfy
satisfy
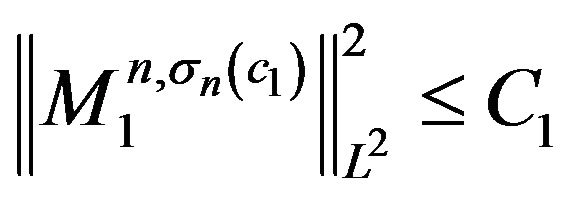 .
.
Proof. For  and
and , since the
, since the 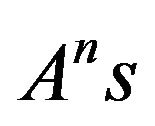 are predictable and the
are predictable and the  are martingales,
are martingales,
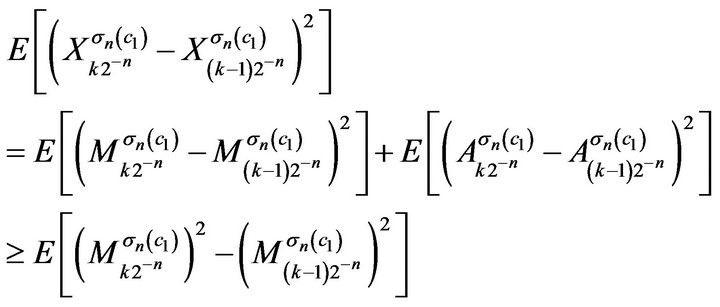
we write 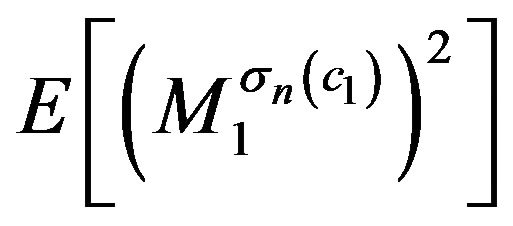 as a telescoping series and simplifying to get
as a telescoping series and simplifying to get
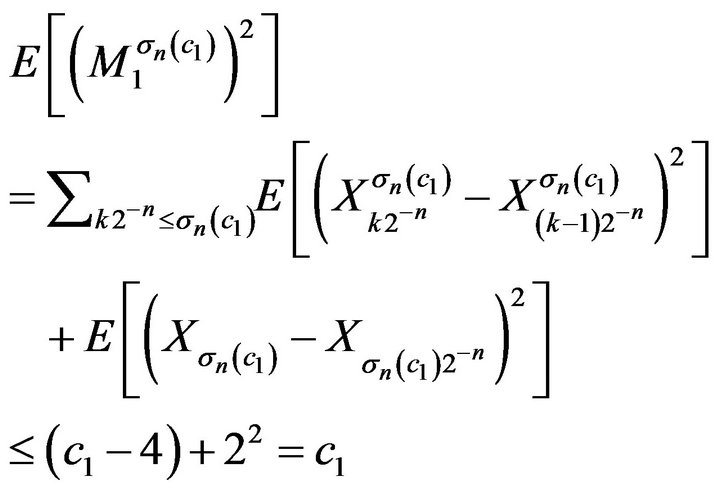

Lemma 1.8. Let
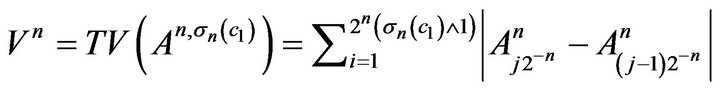 .
.
Under the assumption of Proposition 1.5 the sequence  is bounded in probability.
is bounded in probability.
Proof. Assume for contradiction that  is not bounded in probability. Then there is
is not bounded in probability. Then there is 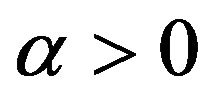 such that for all k there is
such that for all k there is 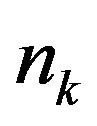 such that
such that  For
For 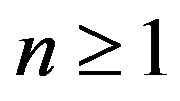 define
define

and
 .
.
Then  and
and
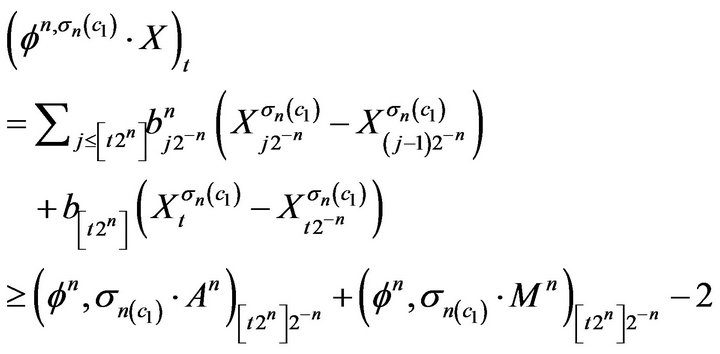
and at time t = 1 we have
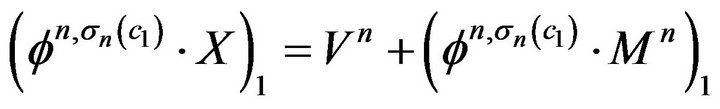 .
.
But the second summand is bounded in L2, so we conclude that  is not bounded in probability.
is not bounded in probability.
We defined a sequence of stopping times
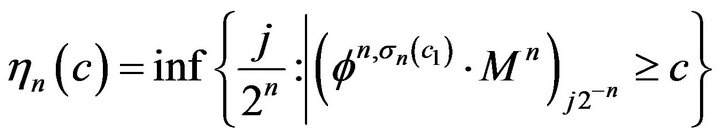 .
.
Because
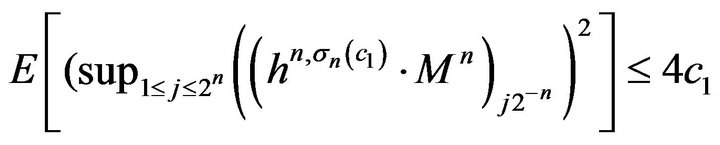
by Doob’s sub-martingale in-equality,(see [9,10])
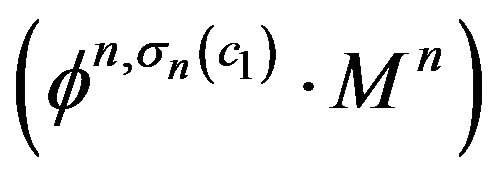 is bounded in probability. Therefore there is
is bounded in probability. Therefore there is  such that
such that . Note that
. Note that 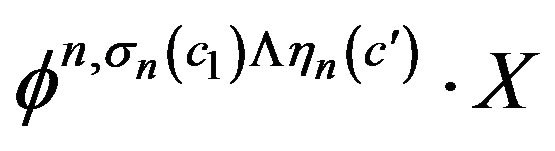 is uniformly bounded below by
is uniformly bounded below by . We claim
. We claim  is not bounded in probability. Indeed, for any n and any k,
is not bounded in probability. Indeed, for any n and any k,

Since , the probability of the other event is at least
, the probability of the other event is at least . This gives the desired contradiction because it is now easy to construct a FLVR + LI.
. This gives the desired contradiction because it is now easy to construct a FLVR + LI.
Proof of Proposition 1.5: Defined a sequence of stopping times
 .
.
By Lemma 1.8 there is c2 such that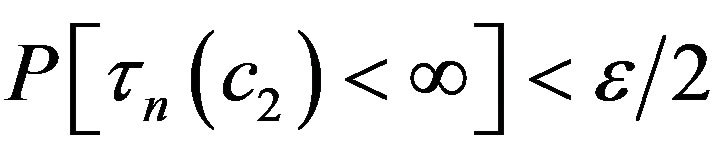 . Take
. Take  and
and 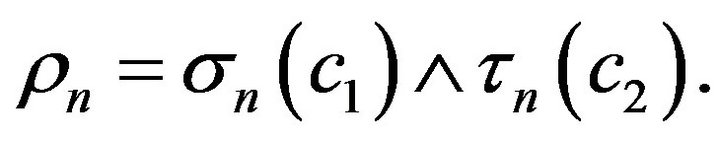
Lemma 1.9. [11]. Let 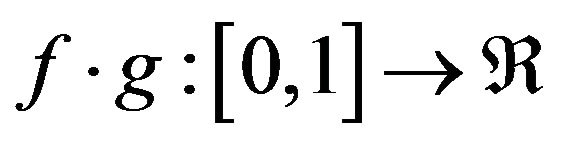 be measurable functions, where f is left continuous and takes finitely many values. Say
be measurable functions, where f is left continuous and takes finitely many values. Say . Define
. Define
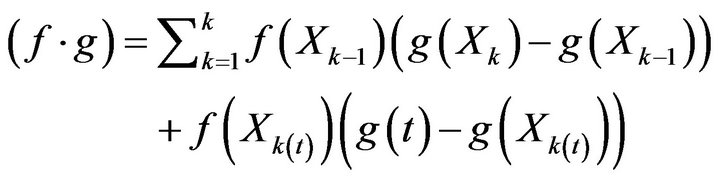
where 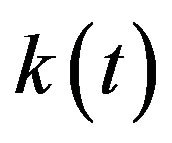 is the biggest of the k such that Xk less than or equal to t. Then for all partition
is the biggest of the k such that Xk less than or equal to t. Then for all partition ,
,
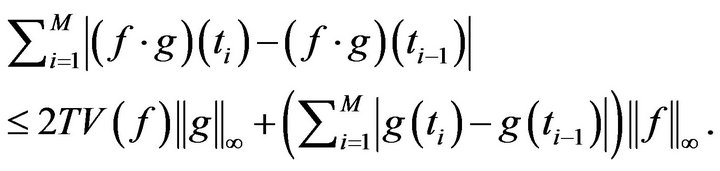
Proposition 2.0. Let  be cadlag and adopted, with
be cadlag and adopted, with 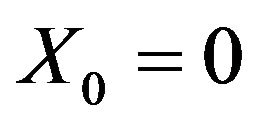 and such that
and such that 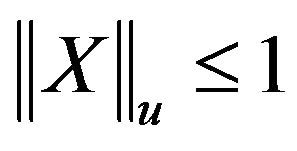 and X satisfies NFLVR + LI. For all
and X satisfies NFLVR + LI. For all  there is C and a
there is C and a 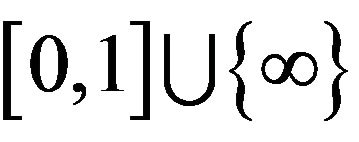 valued stopping time
valued stopping time  such that
such that
 and sequence
and sequence 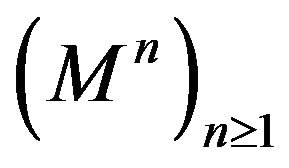 and
and  of continuous time cadlag processes such that for all n1)
of continuous time cadlag processes such that for all n1) 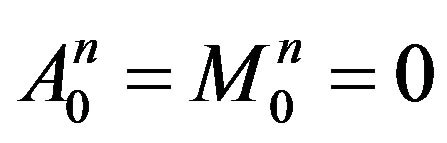
2) 
3)  is a martingale with
is a martingale with 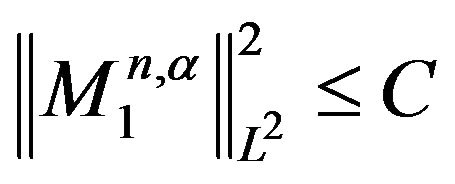
4) 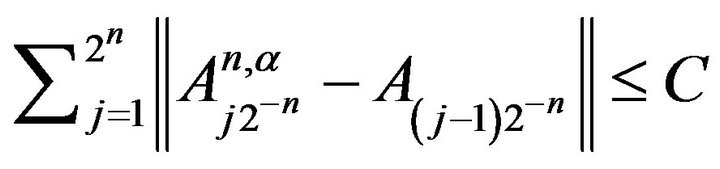
Proof. Let  be given. Let C,
be given. Let C, 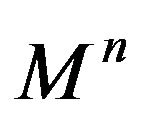 ,
,  , and
, and  be as in proposition 1.5. Extended
be as in proposition 1.5. Extended 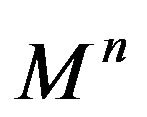 and
and 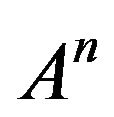 to all
to all 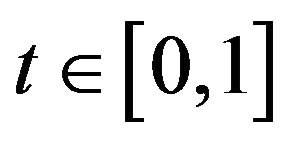 by defining
by defining 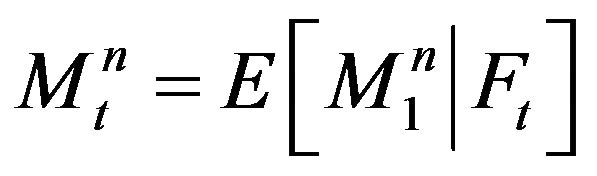 and
and
 . Not that the extended
. Not that the extended  is no longer predictable, and currently we only have control of the total variation of
is no longer predictable, and currently we only have control of the total variation of  over
over , i.e.
, i.e.

Notice that, for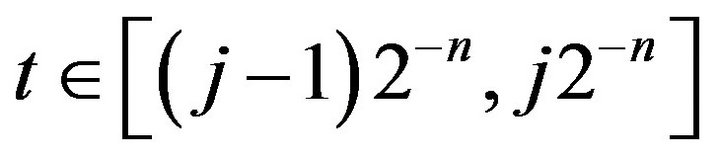 ,
,
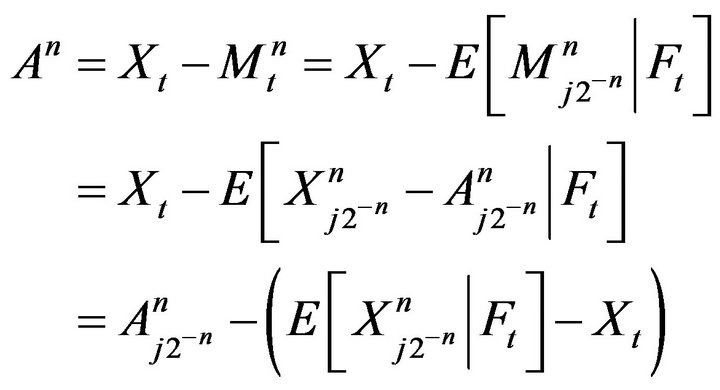
From this and  it follow that
it follow that
 , so
, so . How do we fine the limit of the sequence of stopping times
. How do we fine the limit of the sequence of stopping times ? The trick is to define
? The trick is to define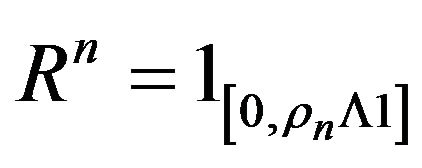 , a simple predicator process, and note that stopping at
, a simple predicator process, and note that stopping at  is like integrating Rn, i.e.
is like integrating Rn, i.e. 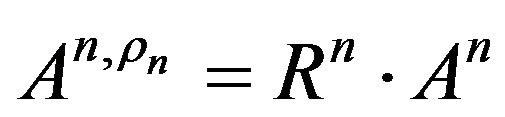 and
and . We have that
. We have that
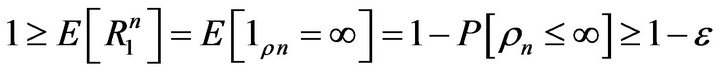 .
.
Apply Komlos’ Lemma to obtain convex weights 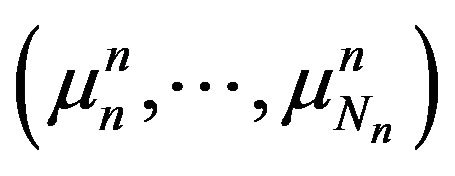 such that
such that
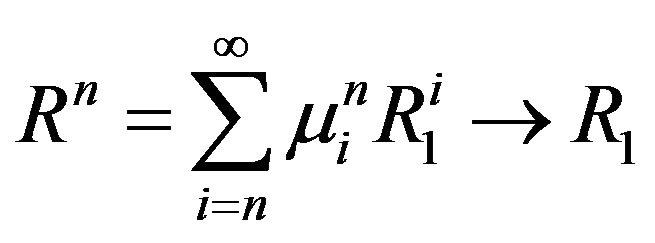
a.s as  By the dominated convergence theorem,
By the dominated convergence theorem,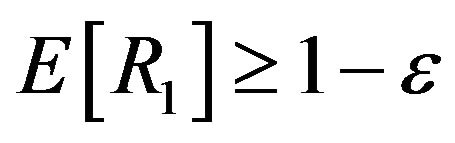 . Observe that
. Observe that
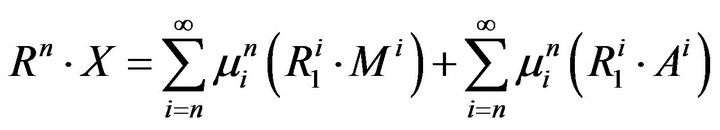
Define . Each
. Each  is left continuous, decreasing process. In particular,
is left continuous, decreasing process. In particular, 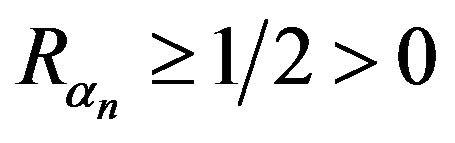 , so we can divide by this quantity. We claim that
, so we can divide by this quantity. We claim that 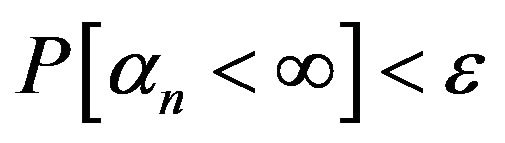 . In deed, on the event
. In deed, on the event ,
, 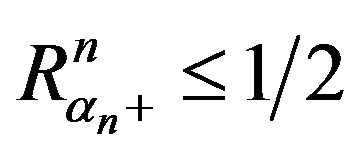
 so
so

Define new processes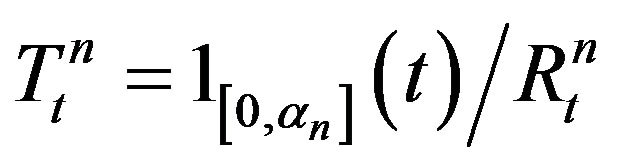 . Then
. Then 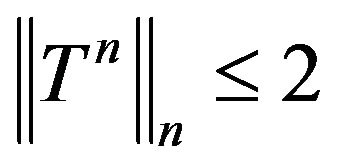 and
and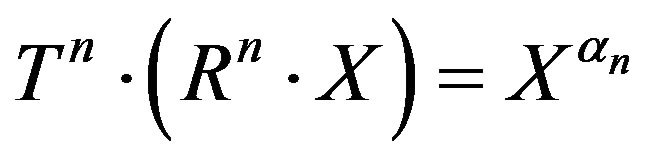 . Thus we define Mn and An by
. Thus we define Mn and An by
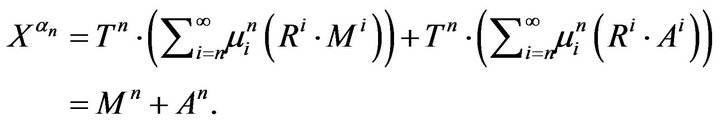
The total variation of 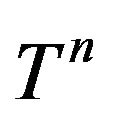 over
over  is bounded by 3. By Lemma 1.9,
is bounded by 3. By Lemma 1.9,

That  follows from the fact that
follows from the fact that
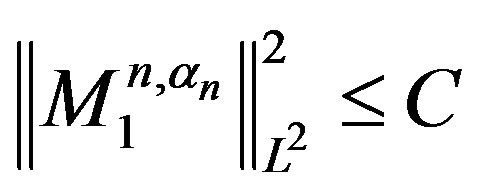 . To finish the proof, we show that there is a subsequence
. To finish the proof, we show that there is a subsequence  such that
such that 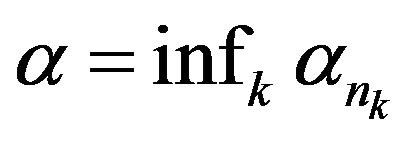 satisfies
satisfies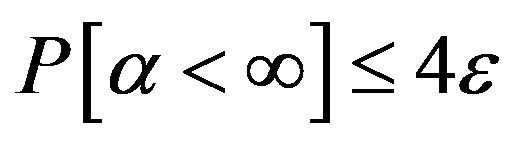 . We know
. We know 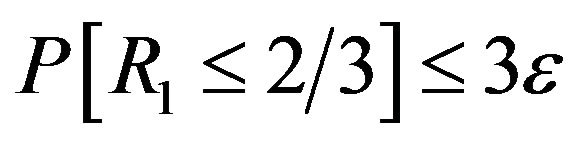 because
because . Since
. Since  a.s there is a subsequence such that
a.s there is a subsequence such that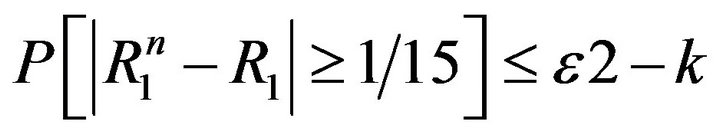 . Finally,
. Finally,
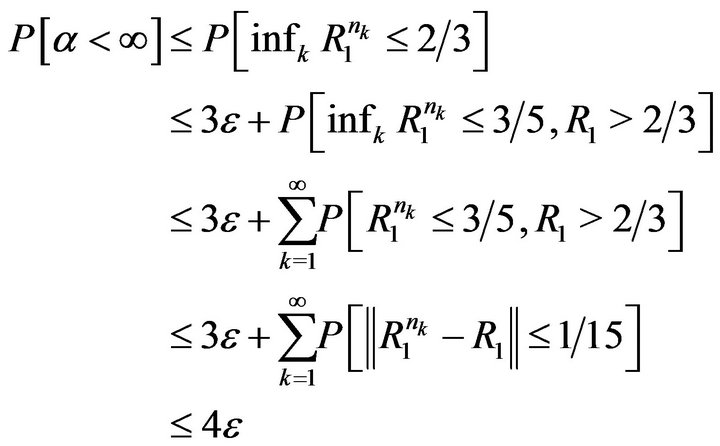
Therefore ,
, 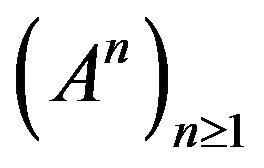 and
and  have the desired properties.
have the desired properties.
Proof of the Main Theorems Proof of Theorem 1.3. We may assume the hypothesis of proposition. Let 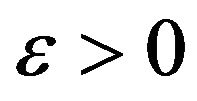 and take C,
and take C,  ,
, 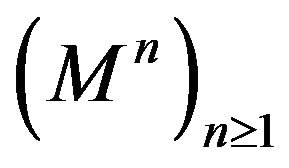 ,
, 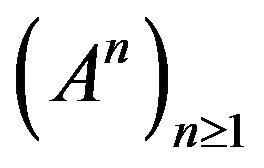 as in proposition. Apply komlos lemma to find convex weights
as in proposition. Apply komlos lemma to find convex weights 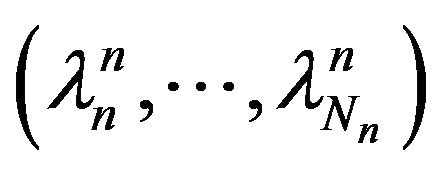 such that
such that


for all , where the convergence is a.s. For all n,
, where the convergence is a.s. For all n,
 so the total variation of A over D is bounded by C. Further, we have
so the total variation of A over D is bounded by C. Further, we have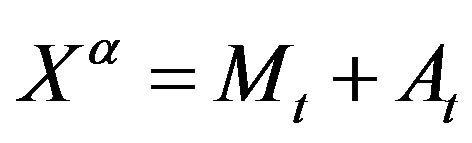 . A is a cadlag on D, so define it on all of [0,1] to make it cadlag. M is
. A is a cadlag on D, so define it on all of [0,1] to make it cadlag. M is  martingale so it has a cadlag modification. Since
martingale so it has a cadlag modification. Since  and
and 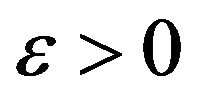 was arbitrary, and the class of classical semimartingales is local, X must be a classical semimartingale.
was arbitrary, and the class of classical semimartingales is local, X must be a classical semimartingale. 
Proof of Theorem 1.4. We no longer assume that X is locally bounded. The trick is to leverage the result for locally bounded processes by subtracting the big jump from X. Assume without loss of generality that  and defined
and defined . Then X = X − J is an adopted, cadlag locally bounded process. We will show that theorem 1.4 for X implies NFLVR + LI for X, so that we may apply theorem 1.3 to X. Then since J is finite variation, this will then imply X is a classical semimartingale .
. Then X = X − J is an adopted, cadlag locally bounded process. We will show that theorem 1.4 for X implies NFLVR + LI for X, so that we may apply theorem 1.3 to X. Then since J is finite variation, this will then imply X is a classical semimartingale .
Suppose 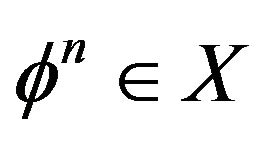 are such that
are such that  and
and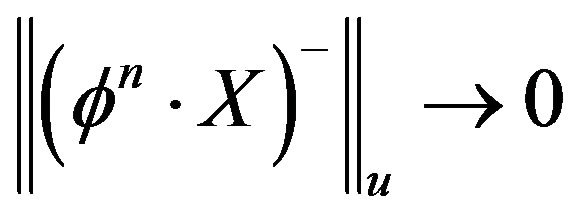 . We need to prove that
. We need to prove that 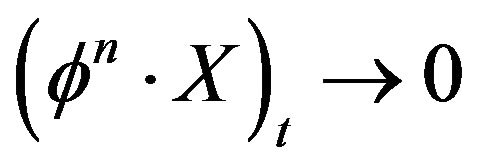 in probability . First we will show that
in probability . First we will show that .
.

by the assumptions on 
By (1), 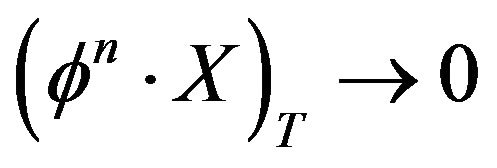 in probability. Since
in probability. Since
 in probability, we conclude that
in probability, we conclude that
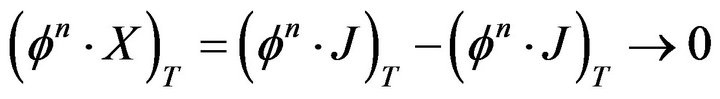
in probability. Therefore X satisfies NFLVR + LI. 
NOTES