Decun Zhang, Liying Wang, Jie Huang, Wenqiang Ji
Institute of Applied Mathematics, Naval Aeronautical and Astronautical University Yantai, China.
DOI: 10.4236/am.2012.32029   PDF    HTML   XML   4,259 Downloads   7,919 Views   Citations

Abstract

In this paper, we investigate the global character of all positive solutions of a population model of systems. Some interesting convergence properties of the solution are given, and lastly, we obtain that the solution is permanent under some conditions.

Keywords

Population Model; Global Attractor; Difference Equations

Share and Cite:

1. Introduction

In the recent monograph [1, p.129], Kulenovic and Glass give an open problem as follows:

Open problem 6.10.16 (A population model).

Assume that and. Investigate the global character of all positive solutions of the systems:

(1)

where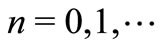, which may be viewed as a population model.

To this end, we consider Equation (1) and obtain some interesting results about the positive solutions of Equation (1).

2. Basic Lemma

Lemma 1 Assume that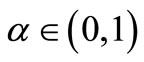,. Then the following statements are true:

1) If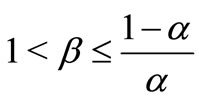, then Equation (1) has a unique nonegative equilibrium solution as follows:

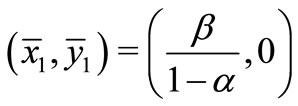
2) If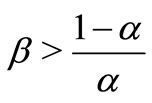, then Equation (1) has two no-negative equilibrium solutions as follows:

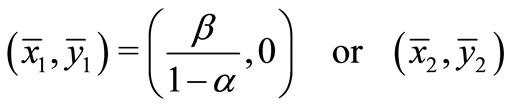
where, 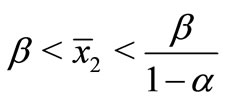such that

(2)

Proof: The equilibrium equations about Equation (1) can be written as follows:

(3)

It is easy to see that, is a group solutions of Equation (3).

By (3) we obtain

(4)

Thus

(5)

Noting that (3) and (4) we get:

Changing (5) to (6)

(6)

Set

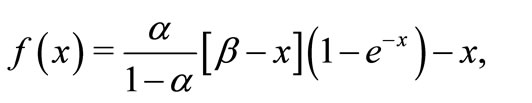
Observing that

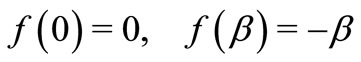
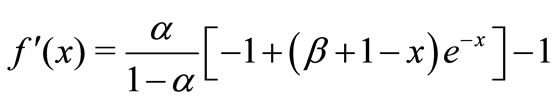
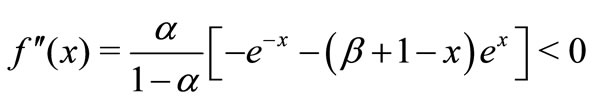
So, by the convex functions properties, if

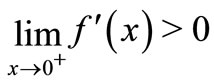, then we can obtain Equation (6) has a unique positive solution.

In fact, by the continuous of, we can get

Hence, we complete the proof.

3. Main Results

Theorem 3.1 Assume that 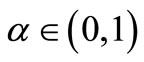and.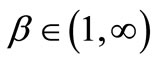

Then every positive solutions and 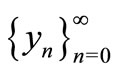of Equation (1) have the following properties:

1);

2).

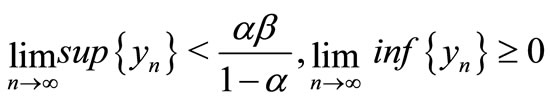Proof: By Equation (1) we have

It is to say that .

By Equation (1) we also get

Thus,.

This completes the proof.

Theorem 3.2 Assume that,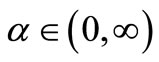

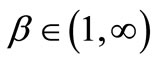
and. Then every positive solutions of Equation (1) convergences to the unique no-negative equilibrium solution.

Proof: By Theorem 3.1, we have that there exists a nature number n₀ such that 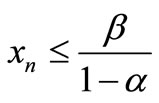for.

Hence, by Equation (1) we get

Thus is decreasing.

Suppose that

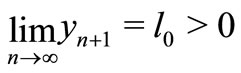(7)

Then by Equation (1) we have

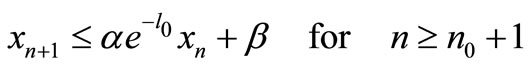
By induction we obtain

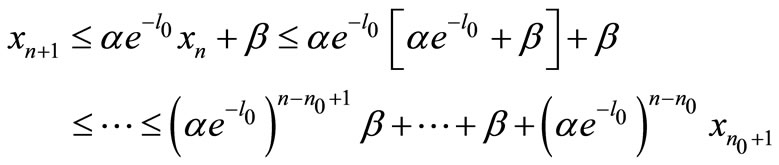
Thus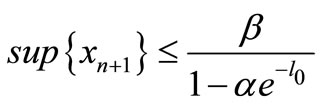. Hence there exists a such that for.

Noting that Equation (1)

By induction,

It is to see that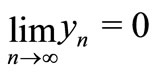. This is a contradiction with (7), then.

Noting that Equation (1) we have

i.e.

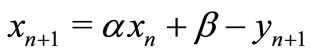
Let,. Then

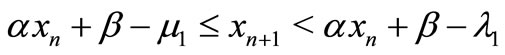
By induction we obtain

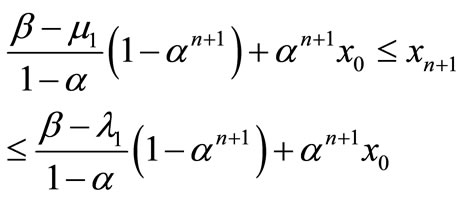
as, then

(8)

Because of, we obtain that.

Hence

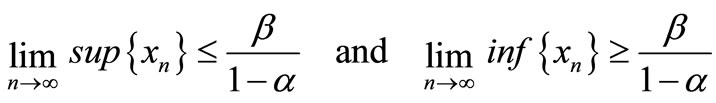(9)

By (9) we get.

We complete the proof.

Theorem 3.3 Assume that, and. Then Equation (1) is permanent.

Proof: By Equation (1) we obtain

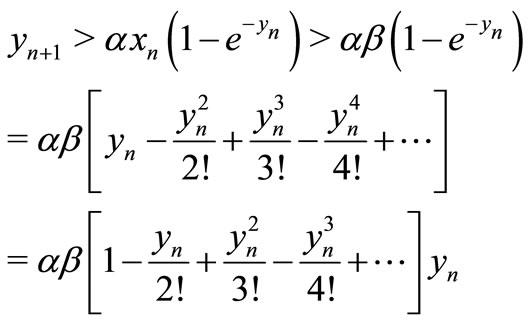
There exists two positive constants and such that

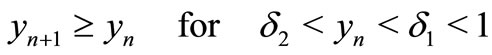
Hence.

Using Theorem 3.1, we complete the proof.

NOTES

Conflicts of Interest

The authors declare no conflicts of interest.

References

[1]	M. R. S. Kulenovic and G. Ladas, “Dynamics of Second Order Rational Difference Equations,” Chapman & Hall/ CRC, Boca Raton, 2002.