Vol.3, No.10, 866-883 (2011) Natural Science
http://dx.doi.org/10.4236/ns.2011.310112
Evidence for pentagonal symmetry in living and model
cellular systems
John J. Wille
Bioderm Technologies, Inc., Chesterfield, NJ, USA;
Received 11 August 2011; revised 15 September 2011; accepted 26 September 2011.
ABSTRACT
Microscope observations of normal human ke-
ratinocytes (NHK) propagated in a serum-free
medium reveal a high frequency (>70%) of pen-
tagonally-shaped colonies over a wide range of
colony sizes that persist over many sequential
cell generations. NHK colonies derived from sin-
gle cell isolates also display pentagonal symme-
try as confirmed by a photographic technique
known as “Markham Rotation”. The generality of
pentagonal cellular morphology was extended
to observations in situ of pentagonally-shaped
basal layer epidermal cells of normal human
epidermis, monolayer cultures of normal and
immortalized keratinocytes, several different ch-
ick embryo cells, and in previously published
photographs. Statistical methods were applied
that differentiate planar close-packing of po-
lygonal configurations observed in living cellu-
lar system from several examples of non-living
cellular aggregates that were produced spon-
taneously in nature or in the laboratory under
defined physico-chemical conditions.
Keywords: Cells; Colonies; In Vitro Tissue
Culture; Mammalian Tissues; Model Tissues;
Pentagonal Symmetry; Rotational Symmetry
Analysis
1. INTRODUCTION
“While pentagonal symmetry is frequent in the or-
ganic world, one does not find it among the most per-
fectly symmetrical creations of inorganic nature, the
crystals.” Hermann Weyl, Symmetry (1952), p. 63.
Very few objects in the physical world display five-
fold symmetry. Yet, living forms manifest a ubiquitous
five-fold rotational symmetry in the plant world [1], and
across many levels of organization in the acellular and
animal kingdoms, e.g., the icosahedral symmetry of viral
capsids of Adenovirus, ΦX174, and the Hepatitus vi-
ruses [2], the five-fold radial symmetry of the medusa
forms of Coelenterates, the asteroidal arms of Echino-
derms [3], and the five gill arch system of Chordates [4].
In addition, there are numerous examples of adult or-
gans/tissue structures [5] as seen in the five-fold sym-
metry of the human heart, hands and feet, and the
five-fold symmetry the distal limb appendages seen in
the Crossopterygian fish, a trait inherited by all its ver-
tebrates descendants and exemplified in the pentadactly
fingers and bones of the human hand and the skeletal
systems across a wide spectrum of both invertebrate and
vertebrate phylogenies.
Reports of pentagonal symmetry at the subcellular,
cellular and tissue level of organization are rare and con-
fined to surface features of icosahedral viruses, surface
features (e.g., cirri) of several genera of Hypotrichs, e.g.,
Oxytrichia flax and Euplotes patella [6] and the beautiful
five-fold radial symmetry of Radiolarians first described
by Ernst Haeckel in his Vo y ag e r studies, and depicted in
Growth and Form [7], and in Symmetry [1]. The geome-
try of cellular structures whose surface pattern is po-
lygonal has been previously investigated [8], and under-
pins knowledge and theoretical modeling made in the
ensuing years bearing on the underlying filamentous
cytoskeleton and regulatory protein networks that gives
shape to cells [9-12]. Unremarked upon examples of
pentagonally-shaped cellular aggregates occur in pub-
lished photomicrographs: 1) a human squamous carci-
noma cell line, DJM-1 [13] (see Figure 1(c)), 2) normal
human keratinocytes [14] (see Figure 1(a)), 3) rat der-
mal papilla cells [15] (see Figure 1(c)), and 4) rat hepa-
tocytes [16] (Figures 2(a) and (c)), 5) luminal surface of
rat uterine endometrium before and after estrogen treat-
ment [17] (see Figures 3(a) and (f)). Nevertheless, there
are no previously published reports of either individual
eukaryotic cells or their colonies displaying a predomi-
nant pentagonal morphology with confirmed five-fold
rotational symmetry.
Here, for the first time, we document the predominant

J. J. Wille / Natural Science 3 (2011) 866-883
867
867
occurrence of pentagonal-shaped epidermal keratinocyte
cells and their colonies in rapidly proliferating serum-
free cultures confirmed by the technique of Markham
Rotation [18]. This report provides further evidence for
the generality of pentagonal colony symmetry seen in
epithelia of other vertebrate tissues. We propose a model
that postulates that pentagonal colonies are result of cel-
lular tactoidal structures composed predominantly of
five-sided cells. Finally, we challenge the hypothesis that
predominant pentagonal symmetry of cells and their
cellular aggregates, which we here document, are solely
representative of living cells by undertaking a compara-
tive statistical analysis of non-living cellular aggregates
formed by nature or under defined laboratory conditions.
2. MATERIALS AND METHODS
2.1. Cell Culture
Secondary passaged normal human keratinocytes were
cultured in complete serum-free (MCDB 153, SFM)
medium. Single-celled clones were first isolated on glass
Figure 1. Histogram plot of the frequency (ordinate) of pen-
tagonal colonies (%) having N total cells per colony. A total
number T of colonies analyzed was 44.
Figure 2. Photograph of a single colony of NHK cells formed
from a single cell cultured in serum-free medium for ten days,
fixed and stained with 0.2% crystal violet. Magnification, 35×.
cloning clips and transferred to 35 mm Petri dishes and
fed SFM. The HaCat immortalized human epidermal
keratinocytes were obtained from the laboratory of Dr.
Mark Pittelkow Mayo Clinic Rochester, MN). They
were cultured in SFM and in DMEM-10% FCS.
2.2. Cytochemical and Immunohistological
Staining
A modification of Mallory’s Trichrome stain [19] was
(a)
Circled
Pentagons
(b)
Figure 3. (a) is a low power phase contrast photomicrograph
of a rapidly dividing NHK cells cultured in serum-free medium
for five days after seeding at 3 × 103 cells/cm2. (Mag., 840×);
(b) is a low power phase contrast photomicrograph of a rapidly
dividing low density secondary culture of HaCat cells cultivated
in serum-containing medium for three days (Mag., 240×).

J. J. Wille / Natural Science 3 (2011) 866-883 868
employed to differentially stain non-keratinized undif-
ferentiated basal keratinocytes a blue color, and differen-
tiated and keratinizing epidermal keratinocytes a red
color, according to previously published methods [20].
Human skin was embedded in paraffin sectioned on a
microtome and 1 - 2 micron thin sections prepared by
technique of indirect immunfluorescense microscopy
using mouse monoclonal antibodies to detect epidermal
basal layer cells with the Hairy Cell Leukemia HCL an-
tibody and a mouse anti-Involukrin monoclonal antibody
to detect differentiated layers of the epidermis. A
Goat-IgG anti-mouse was employed for the secondary
antibody.
2.3. Phase Contrast Photomicrographs
Early passage cultures of normal human keratinocytes
and HaCat cells were photographed using a Cannon 35
mm camera back loaded with 200ASA B & W film set-
up on a Nikon inverted phase contrast microscope using
5×, 10× and 20× phase objectives and 15× oculars.
Black and white prints were made from 35 mm B & W
film and were enlarged by a factor of 6.6×.
2.4. Rotational Symmetry
The symmetry-enhancing photographic technique known
as “Markham Rotation” was employed to analyze pho-
tomicrograhphs of cells and colonies [17]. For this pur-
pose, we made a laminated board (15 inches × 15 inches)
with small metal pivot well in the center for inserting a
metal pin with attached string. We also made a turn table
made from a square sheet (12 inches × 12 inches) clear
plastic with a central hole corresponding to the center
hole metal pivot well. Attached to the underside of the
turn table was affixed a square piece of green cardboard
upon which was drawn with a black felt pen lines radi-
ating out from the center to the edge, which marked out
24 intervals representing the progressive 15 degree in-
crements of a 360˚ circle. The turntable is rotated on the
laminated board by placing the metal pin through the
plastic sheet into the pivot well and turning the square
plastic sheet to the desired angle by counterclockwise
movement relative to the identity image positioned at
zero angle. The image to be analyzed is placed directly
on the turntable and fixed to it with the metal pin
through its determined center of gravity. Rotation of the
image by different angular displacements under a photo-
graphic camera system allows superimposition of the
rotated image on to the photo graph the original
un-displaced image. By displacing the original image
and photographing it at fixed angles that are multiples
underlying the N-order of rotational symmetry the final
superimposed image will either be enhanced or blurred.
Briefly, the center of gravity of the photographed object
to be analyzed is located by geometric construction and
is used as the center of rotation. The N-fold order of ro-
tational symmetry is determined by selecting the best fit
to the original shape of the object, i.e., shape invariance
under rotation.
2.5. Normal and Artificial Cellular Tissues
Photocopies were made of published figures of mono-
layer cultures of normal human skin, NHK cells, MDCK
cells, and three different chick embryo cell types: retinal
pigment cells, lung epithelial cells and chondrocytes [8].
In addition, the cell boundaries of each cell were out-
lined to reveal the position and cell contacts with their
nearest neighbors. For artificial cellular tissues, we pho-
tocopied a published figure reproduced by Thompson [7]
(see page 501 and figure 181 after Leduc). The artificial
cellular tissue formed by the diffusion in gelatin of drops
of a solution of potassium ferrocyanide. Another artifi-
cial cellular tissue formed by crowding deformable clay
spheres into a close packed array from an initially ran-
dom configuration was investigated earlier [8]. Unlike
hard spheres the final configuration arrived at is irregular
with both hexagons and pentagons in close packed space
filling array. The remarkable resemblance of these artifi-
cial tissues to histological preparations of living tissues
has been known and commented upon elsewhere [1,7].
To quote Weyl (page 89), “The regularity leaves some-
thing to be desired; there are even places where a penta-
gon is smuggled in instead of a hexagon”. In both nor-
mal and artificial tissues we found substantial countable
numbers of rectangular, pentagonal, and hexangular cells
and even heptangular polyhedral cells. We subjected
these photocopied figures to further analysis by coloring
in the hexagons, pentagon and other polygonal shaped
cells with different colors and performed various statis-
tical calculations to determine if normal and artificial
tissues differed in their number and types of polygonal
shaped cells and a statistical analysis of nearest neighbor
cell contacts between the different classes of polygons
using the binomial expansion derived from their relative
frequencies, and calculating the probability that such
cellular arrangement could be due to chance alone using
the Student t-test and Chi-Square formulas.
3. RESULTS
3.1. Pentgonal Symmetry of Normal and
Immortalized Human Keratinocyte
Colonies
Figure 3(a) is a typical low power (10× phase objec-
tive close packed space filling array)) phase contrast
photomicrograph of a rapidly dividing NHK cells sev-
eral days after seeding at 3 × 103 cells/cm2 into SFM. It

J. J. Wille / Natural Science 3 (2011) 866-883
869
869
is estimated that greater than 70% (5/7) of the counted
colonies in this one field have an apparent pentagonal
shape. Once again (Figure 3(b)) this low power (4×
phase objective) phase contrast picture shows many pen-
tagonally-shaped colonies (circled). We have observed
routinely that pentagonal-shaped colonies can reassem-
ble in less than one day from component single cells
dissociated by trypsin treatment and replated in DMEM:
10% FCS medium, indicating that formation of pen-
tagonal colonies is not just the result of clonal growth, but
can occur by re-association of cells in different stages of
asynchronous growth.
3.2. Pentagonal NHK Clones
Figures 4(a) and (b) present photomicrographs of ten
clones taken from the same early-passaged secondary
culture seeded at a cell density of 1000 cells/cm2. Under
low density serum-free culture conditions every cell di-
vides on average once a day [20]. The cells in each col-
ony are the result of progressive division starting on
days 2 - 3 with a small 6-cell colony and ranging up to a
large 54-cell colony seen on days 5 - 6. In each case the
overall shape of each colony is roughly pentagonal. The
above photomicrographs reveal an internal cellular
structure in which individual keratinocytes cells some-
how arrange themselves to maintain a roughly pentago-
nal colony shape even under continuous conditions of
cell growth and division. Figure 1 is a histogram giving
the frequency of NHK colonies of different sizes all de-
rived from a single 3 - 4 day old culture seeded into
SFM media at a cell density of 2 × 103 - 3 × 103 cell/cm2.
A total of 44 colonies were assessed for their total cell
count and their colony shape. The results indicate that
the smallest colony size detectable with a pentagonal
shape was four-cell colony. The majority of pentagonal
colonies were composed of eight cells and 86% (6/7) of
all 8-celled colonies were pentagonal. A majority (5/8)
of colonies composed of more than 8-cells were also
pentagonal.
3.3. A Pentagonal Colony Derived from a
Single-Cell Clone
Figure 2 present a black and white photo of a macro-
scopic clone of about 1000 cells. This clone arose from a
single neonatal foreskin keratinocyte cell that was iso-
lated on a glass cloning chip and transferred to a 35 mm
circular plastic Petri dish and fed SFM for 10 days, fixed
and stained with crystal violet stain and photographed.
The shape appears to be roughly pentagonal. This colony
shape is the outcome of a single cell and all its descen-
dants dividing ten times in ten days to form a coherent
pentagonal clone.
3.4. Markham Rotational Analyses of a
Macroscopic Keratinocyte Clone
Figure 5 Markam rotational analysis of NHK clone
generated from a single cell isolate and cultured in low
calcium (0.1 mM) serum-free medium. As same as de-
picted in Figure 2. Inserts: (a) original colony morphol-
ogy; N (0, 4, 5, 6, and 8) the rotational symmetry. Best
fit by eye is (b) (N = 5).
(a) (b)
Figure 4. (a) Series of phase contrast photomicromicraph of live NKC cultures propagated in a serum-free medium for
several consecutive days to generate clones of different sizes A) n = 6, 7, 8, 9, 10, and 12-cells. (Mag., 1250×); (b)
Series of phase contrast photomicromicraph of live NKC cultures propagated in a serum-free medium for several
consecutive days to generate clones of different n = 15, 27, 44, and 55-cells and of different culture ages. (Mag.,
1250×). Clones depicted were all from the same initial plating.

J. J. Wille / Natural Science 3 (2011) 866-883 870
In order to determine if the above single-celled clone
is pentagonal in shape we performed a series of rota-
tional image enhancements on it. Figure 5 is a photo-
graph showing the results of Markham Rotation per-
formed on a 1000-cell clone that was initiated from a
single-cell. The best fit to the colony shape is N = 5 (b)
or image invariance recurs at 72 degree angular rotation.
All other fixed angular rotations show did not enhance
the image, i.e., angular rotation of 90 degree (N = 4),
angular rotations of 60 degrees (N = 6), and angular ro-
tations of 45 degrees (N = 8) only produce blurred circles.
3.5. Rotational Symmetry Analysis of a
Growth Arrested but Undifferentiated
Keratinocyte Colony
Figure 6 presents a photograph of a secondary pas-
sage NHK culture that was seeded at 500 cells in to a
dish and fed high calcium (2 mM cCa++) growth factors
replete SFM for 7 days. The cells were fixed and stained
with Mallory’s Trichrome stain [19]. The entire colony
consisting of 152 cells stained blue indicating that all of
the cells remained undifferentiated. Each cell of the col-
ony had doubled approximately seven times in a 7 day
period of growth as expected for rapidly dividing kera-
tinocytes with a 24 hour doubling time. As shown in
Figures 6(b)-(d) Markham Rotational analysis confirmed
that the initial pentagonal shaped image of the colony (N
= 1) gives a best-fit for image enhancement with a
five-fold order of reflective symmetry, i.e., N = 5.
Figure 5. Markam rotational analysis of NHK clone generated
from a single cell isolate and cultured in low calcium (0.1 mM)
serum-free medium. A same as depicted in Figure 4. Inserts: (a)
original colony morphology; N (0, 4, 5, 6, and 8) the rotational
symmetry. Best fit by eye is (b) (N = 5).
Figure 6. Markham rotational analysis of a 152-cell NHK colony
cultured in high calcium (2 mM) serum-free medium. (a), (b), (c),
and (d) are different rotational symmetries of order (N): 1, 4, 5, and 6.
Best fit by eye is (c) (pentagonal).

J. J. Wille / Natural Science 3 (2011) 866-883
871
871
3.6. Rotational Symmetry Analysis of a
Growth Arrested-Partially
Differentiated Keratinocyte
Colony
A first passage, NHK culture was seeded at 500 cells
per dish into high calcium (2 mM Ca++) growth factor
replete SFM (NF179P1, GF+, 2, d6) and fixed six days
later. The cells were stained with Mallory’s Trichrome
[19], which stains basal epidermal keratinocytes a blue
color and the differentiated and stratifying keratinocytes
a red color [20]. Figures 7 is a photograph showing a
single undifferentiated pentagonally-shaped colony of
greater than 100 cells. The periphery and the interior are
uniformly stained blue to red and a small nest of dark
blue cells is located near the center of the colony. The
overall shape of the colony remains pentagonal as con-
firmed by the best-fit at N = 5 for enhancement of the
peripheral portion of the image, but N = 4 and N = 6 also
seem to fit best for the interior of the colony. The results
suggest that the interior of the colony is composed of
hexagonal and rectangular shaped “congeries, and that
cells at the peripheral the loci of undifferentiated cells
are mainly responsible for the overall pentagonal shape
of the colony.
3.7. Rotational Symmetry Analysis of a Fully
Differentiated Keratinocyte Colony
This first passage NHK colony was seeded at 500
cells per dish and cultured for seven days in high cal-
cium (2 mM Ca++) SFM. The cells were fixed and
stained with a modified Mallory Trichrome stain [19] to
reveal basal cells (blue color) from differentiated kerati-
nizing cells (red color). Figure 8 presents a photograph
showing a pentagonally-shaped colony (N = 1) in which
the entire interior stains red and is stratified and kerati-
nizing. By contrast, the cells lying along the perimeter
stain blue, indicating that they are undifferentiated. Fig-
ures 8(b)-(d) present the results of Markham rotational
analyses. The best-fit enhancement of the initial image
yields a five-fold reflective symmetry, N = 5. The blur-
ring of the initial image for N = 4 and N = 6 is likely the
result of the chaotic arrangement of the stratifying cells
in colony interior.
Figure 7. Markam rotational analysis of a photomicrograph of
a NHK colony cuktured in high calcium (2 mM) serum-free
medium. A, B, C, and D are different rotoational symmetries of
order 1, 4, 5, and 6, respectively. Best fir by eye is C (pen-
tagonal).
Figure 8. Markham rotational analysis of a single NHK colony
cultured in high calcium (2 mM) serum-free medium for 7
days, fixed and stained with crystal violet. (a), (b), (c), and (d),
depict different orders (N) of rotational symmetry: 1, 4, 5 and 6.
Best fit by eye is (c) (pentagonal).

J. J. Wille / Natural Science 3 (2011) 866-883 872
3.8. Evidence That Many Epithelial Type
Cells Close Pack into Cellular Sheet
in Monolayer Culture with a High
Frequency of 5-Sided Polygonal Cells
Case 1. Monolayer of epidermal keratinocytes.
Figure 9 is a previously published photograph of a
monolayer of epidermal keratinocytes stained with a
monoclonal antibody that illustrates cell boundaries and
various cell-cell contacts (Cover: J. Invest. Dermatology,
January, 2006). Of the ten (10) cells that could be as-
sessed for their polygonal shapes, 80% (8/10) were pen-
tagonal or made contact with five neighbors. The re-
maining two cells were either 4-sided or 6-sided poly-
gons. Statistical calculations showed a highly significant
deviation from chance outcome if the expectation was all
hexagons (P < 0.001), or if the frequencies of hexagons
and pentagons were equal (P < 0.001), but the chance of
all polygons being pentagons could be due to chance (P
< 0.3). Nearest neighbor statistical analysis revealed that
cell contacts between 4 - 5, and 6-sided polygons was
due to chance given the relative frequencies of the dif-
ferent polygons (P < 0.95) or even if the relative fre-
quencies of hexagons and pentagons were set equal (P <
0.5).
Case 2. Frequency distribution analysis of a 26-cell
NHK clone: number and position of pentagonal-shaped
cells.
Figure 10(a) is high magnification phase contrast
photomicrograph of NHK colony composed of 26 cells
that was cultured in low calcium (0.1 mM) serum-free
medium that helps to accentuate the cell-cell separation.
Two cells were excluded for later analysis as they are not
in close contact with the colony as a whole. Cell outlines
were drawn for each of the remaining 24 cells. Twelve
cells are located at the perimeter; they were traced in
green. Seventy percent (8/12) have outer curved lines
surfaces and are thus indefinite as to polygonal shape.
The remaining four (25%) have definite pentagonal out-
lines. All nine (9) of the interior located cells of the col-
ony were outlined in yellow color. They all have a defi-
nite (nearly regular) pentagonal outline. Two cells were
left uncolored as they were located partially internal and
partially external. The last cell lying near the periphery
is rounded up into two connected spheres, typical of
NHK cells in the process of cell division. If we let p
equal to the frequency of pentagons (13/24 = 0.542), and
q be equal all other shapes (11/24 = 0.458) statistical
calculations showed a significant deviation from chance
outcome if the expectation was all non-pentagonal (P <
0.001), or if the frequencies of non-pentagonal and pen-
tagons were equal (P < 0.001). The chance that all po-
lygonal outlines are pentagonal ones (13/13) is due to
chance is highly improbable (P  0.001). Nearest
Figure 9. Photomicrograph of a monolayer of NHK
cells fixed and stained for cytoimmunifluorescent la-
beling which highlights the cell borders and the ap-
parent polygonal outlines of cells. (Mag., 2750×).
(a) (b)
Figure 10. (a) A phase contrast photomicrograph of a living NHK clone composed of 26 cells cultured in
low calcium (0.1 mM serum-free medium (Mag, 1600×); (b) Enalrged image of (a). Green shows outlines
of externally-located cells; yellow show outlines of internally-located cells.

J. J. Wille / Natural Science 3 (2011) 866-883
873
873
neighbor Chi2-statistical analysis revealed that the ob-
served cell contacts for the three expected types for a
total of 90 cell-cell contacts: pentagons with a neighbor-
ing pentagon (Np/p = 29), non-pentagons with a neigh-
boring pentagon (Np/n = 41), and non-pentagon with
neighboring non-pentagon (Nn/n = 20) is entirely due to
chance (P > 0.2) based their relative frequencies for the
entire cluster of 24 cells.. When the same analysis of cell
to cell contacts are restricted to the 11 interior cells of
the colony, then 59% (26/44) of total contacts are be-
tween pentagons, and the nearest neighbor frequency
Chi2-analysis of interior cells shows that the observed
frequencies of associations between the three expected
classes of contacts are those expected by chance (P >
0.2). Similarly, when we restrict analysis to only the ex-
terior cells of the colony, then 93.5% of the total cell
associations are between a pentagon and a non-pentagon,
and the Chi2-analysis for exterior cells show that the
observed frequencies of association between the three
expected classes of contacts is again those expected by
chance (P > 0.3). Clearly, pentagons are preferentially
located in the interior of the colony and associated with
either other neighboring pentagons or other non-penta-
gons in the frequencies predicted by their relative fre-
quencies according to the binomial distribution expecta-
tions.
Case 3. Pentagonal shaped basal layer cells in human
epidermis.
It is possible that the presence of pentagonal-shaped
keratinocytes is an artifact of growing epidermal kerati-
nocytes in serum-free culture.
To address this concern, we examined the polygonal
shape of epidermal basal layer cells using a immune
histologically-stained section of normal human skin to
reveal the location of the basal layer cells (Figure 11,
top) and obtained data on the number of polygonal
shaped cells by outlining individual cells of the basal
layer (Figure 11, bottom). The number of pentagons,
hexagons and quadragons tallied were 56% (20/36), 39%
(14/36), and 5% (2/36), respectively. Taking into account
just the frequency of pentagons and hexagons, statistical
calculations showed a significant deviations from chance
if either the expectations were all hexagons (P < 0.001)
or all pentagons (P < 0.001) but not significantly differ-
ent from chance if the expectation were an equal fre-
quency of both (P < 0.2). We next performed a statistical
calculation on the nearest neighbor cell contacts among
the three different polygonally-shaped cells. Given their
relative frequencies, the results showed there was no
statistical deviation from chance (P < 0.7) for any of the
six types of cell contacts between for-, five-, or six-sided
polygons. These results demonstrate that the findings of
predominant pentagonally-shaped cells reported for in
vitro cultured keratinocytes are duplicated for the in situ
epidermal keratinocytes in vivo.
Case 4. MDCK monolayer. There are many published
photomicrographs that clearly show five-sided cells in
clusters with among four- and or six-sided cells.
Figure 12 is a good example published as a recent
journal cover page. The photograph shows many pen-
tagonal shaped cells within a colony of MDCK cells
(a)
(b)
Figure 11. (a) Photomicrograph of basal layer cells of normal
human skin stained by indirect immunofluorescent microscopy
to high light basal cell only (b) Enlargement of A showing
polygonal cell oulines of the basal cells.
Figure 12. A published photograph of a monlyayer of MDCK
cells stained immunocytologically with different flours to dif-
ferentiate the cell boundaries in blue Cover; Nature Biotech-
nology 25(3) (2007).

J. J. Wille / Natural Science 3 (2011) 866-883 874
stained with TRITC antibodies, which stain tight junc-
tions blue to outline the cell boundaries [21]. Cell pro-
files outlined by the cadherin-stained cell boundaries
display several different polygonal cell boundaries. Of
interest, is the number and proportion of 4-, 5- and
6-sided polygons. A simple count of the 14 polygonal
(omitting cells at the edges of the photograph) showed
that a surprising 79% (11/14) were 5-sided, 14% (2/14)
were 4-sided, and the remaining one (1/14) was 6-sided.
This result is clearly not the expectation of close packing
of similar sized units in space filling hexagonal array.
The statistical Chi-square test confirmed a highly sig-
nificant deviation from chance if the expected outcome
was all hexagons (P < 0.001) or an equal frequency of
pentagons and hexagons (P < 0.001), but not signifi-
cantly different from chance if the expectation was all
pentagons (P < 0.3). Clearly other causes for a predomi-
nance of pentagons must be at work. This was approached
in yet another statistical study, where we asked if the
nearest neighbors of the six predicted classes of cell
contact frequencies among 4-, 5- and 6-sided cells based
on their relative frequencies in the binomial expansion
was due to chance. The Chi-square test results showed
that the frequencies for all classes of contacts are due to
chance (P < 0.95) even if the frequency of 5-, and
6-sided cells is made equal (P < 0.5). This suggests that
the predominant pentagonal shape of MDCK cells is
unlikely to be a mere accident.
Case 5: Evidence of pentagonal cells in chick embryo
monolayer cultures.
In Figure 13 we examined published photographs
showing the cellular arrangement in cellular monolayers
from three different chick embryo tissues: a) lung
epithelial cells, b) retinal pigment cells, and c) chondro-
cytes.
Figure 13. (a)-(c). A set of three published photomicrograph showing the cell arrangements and polygonal shapes of chick embryo (a)
lung cells (top left), (b) retinal pigment cells (top right), and (c) chondrocytes (bottom). All three figures after Honda, 1983. Center
points are calculated geometric centers.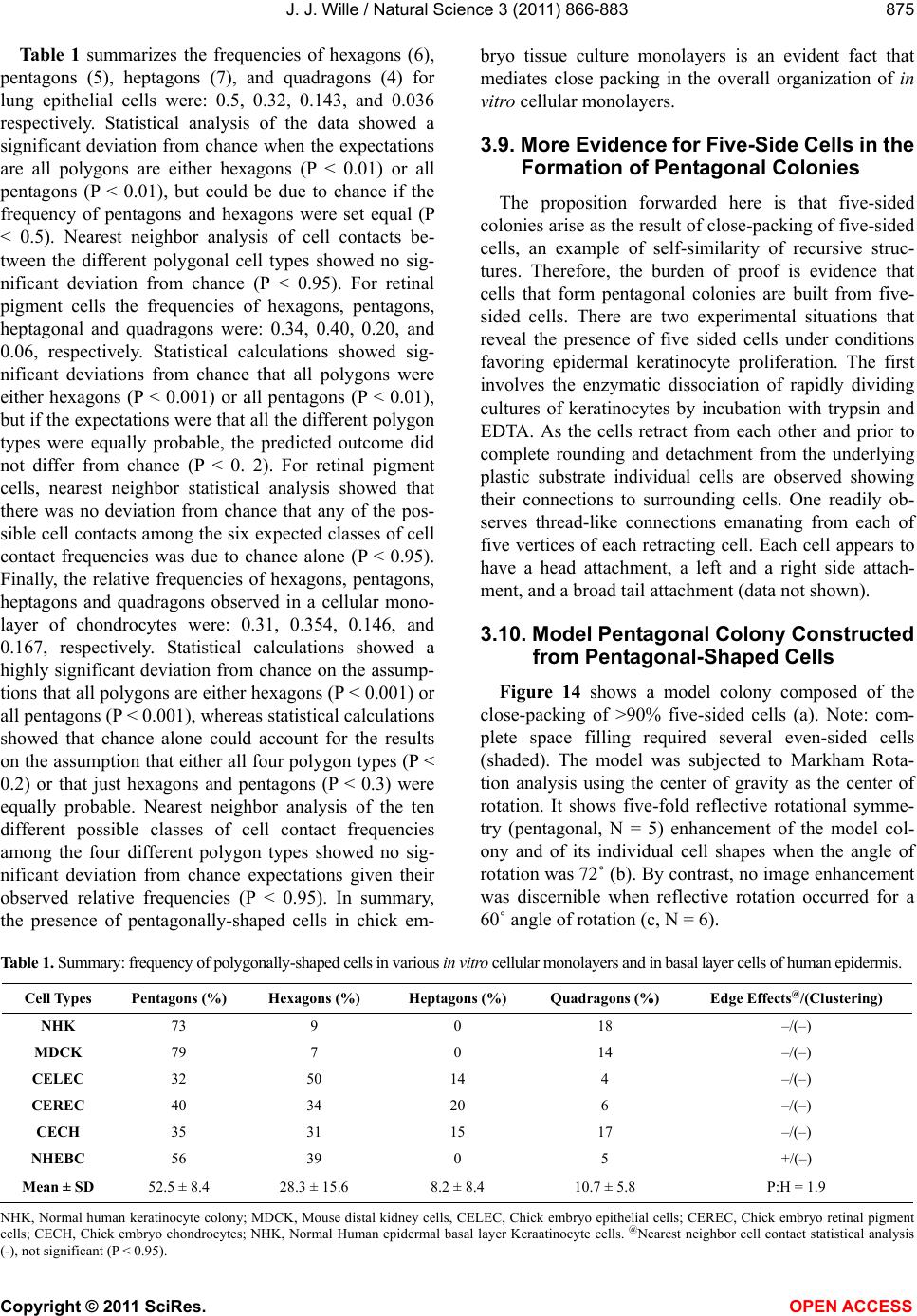

J. J. Wille / Natural Science 3 (2011) 866-883
875
875
Table 1 summarizes the frequencies of hexagons (6),
pentagons (5), heptagons (7), and quadragons (4) for
lung epithelial cells were: 0.5, 0.32, 0.143, and 0.036
respectively. Statistical analysis of the data showed a
significant deviation from chance when the expectations
are all polygons are either hexagons (P < 0.01) or all
pentagons (P < 0.01), but could be due to chance if the
frequency of pentagons and hexagons were set equal (P
< 0.5). Nearest neighbor analysis of cell contacts be-
tween the different polygonal cell types showed no sig-
nificant deviation from chance (P < 0.95). For retinal
pigment cells the frequencies of hexagons, pentagons,
heptagonal and quadragons were: 0.34, 0.40, 0.20, and
0.06, respectively. Statistical calculations showed sig-
nificant deviations from chance that all polygons were
either hexagons (P < 0.001) or all pentagons (P < 0.01),
but if the expectations were that all the different polygon
types were equally probable, the predicted outcome did
not differ from chance (P < 0. 2). For retinal pigment
cells, nearest neighbor statistical analysis showed that
there was no deviation from chance that any of the pos-
sible cell contacts among the six expected classes of cell
contact frequencies was due to chance alone (P < 0.95).
Finally, the relative frequencies of hexagons, pentagons,
heptagons and quadragons observed in a cellular mono-
layer of chondrocytes were: 0.31, 0.354, 0.146, and
0.167, respectively. Statistical calculations showed a
highly significant deviation from chance on the assump-
tions that all polygons are either hexagons (P < 0.001) or
all pentagons (P < 0.001), whereas statistical calculations
showed that chance alone could account for the results
on the assumption that either all four polygon types (P <
0.2) or that just hexagons and pentagons (P < 0.3) were
equally probable. Nearest neighbor analysis of the ten
different possible classes of cell contact frequencies
among the four different polygon types showed no sig-
nificant deviation from chance expectations given their
observed relative frequencies (P < 0.95). In summary,
the presence of pentagonally-shaped cells in chick em-
bryo tissue culture monolayers is an evident fact that
mediates close packing in the overall organization of in
vitro cellular monolayers.
3.9. More Evidence for Five-Side Cells in the
Formation of Pentagonal Colonies
The proposition forwarded here is that five-sided
colonies arise as the result of close-packing of five-sided
cells, an example of self-similarity of recursive struc-
tures. Therefore, the burden of proof is evidence that
cells that form pentagonal colonies are built from five-
sided cells. There are two experimental situations that
reveal the presence of five sided cells under conditions
favoring epidermal keratinocyte proliferation. The first
involves the enzymatic dissociation of rapidly dividing
cultures of keratinocytes by incubation with trypsin and
EDTA. As the cells retract from each other and prior to
complete rounding and detachment from the underlying
plastic substrate individual cells are observed showing
their connections to surrounding cells. One readily ob-
serves thread-like connections emanating from each of
five vertices of each retracting cell. Each cell appears to
have a head attachment, a left and a right side attach-
ment, and a broad tail attachment (data not shown).
3.10. Model Pentagonal Colony Constructed
from Pentagonal-Shaped Cells
Figure 14 shows a model colony composed of the
close-packing of >90% five-sided cells (a). Note: com-
plete space filling required several even-sided cells
(shaded). The model was subjected to Markham Rota-
tion analysis using the center of gravity as the center of
rotation. It shows five-fold reflective rotational symme-
try (pentagonal, N = 5) enhancement of the model col-
ony and of its individual cell shapes when the angle of
rotation was 72˚ (b). By contrast, no image enhancement
was discernible when reflective rotation occurred for a
60˚ angle of rotation (c, N = 6).
Table 1. Summary: frequency of polygonally-shaped cells in various in vitro cellular monolayers and in basal layer cells of human epidermis.
Cell Types Pentagons (%) Hexagons (%) Heptagons (%) Quadragons (%) Edge Effects@/(Clustering)
NHK 73 9 0 18 –/(–)
MDCK 79 7 0 14 –/(–)
CELEC 32 50 14 4 –/(–)
CEREC 40 34 20 6 –/(–)
CECH 35 31 15 17 –/(–)
NHEBC 56 39 0 5 +/(–)
Mean ± SD 52.5 ± 8.4 28.3 ± 15.6 8.2 ± 8.4 10.7 ± 5.8 P:H = 1.9
NHK, Normal human keratinocyte colony; MDCK, Mouse distal kidney cells, CELEC, Chick embryo epithelial cells; CEREC, Chick embryo retinal pigment
cells; CECH, Chick embryo chondrocytes; NHK, Normal Human epidermal basal layer Keraatinocyte cells. @Nearest neighbor cell contact statistical analysis
(-), not significant (P < 0.95).

J. J. Wille / Natural Science 3 (2011) 866-883 876
3.11. Rotational Analysis of Confluent NHK
Cultures
We know that a field of regular pentagonally-shaped
tiles does not cover the entire two-dimension space. Simi-
larly, when a two-dimensional field of pentagonally-
shaped NHK colonies merge together in the process of
forming a confluent culture they presumably fail to
completely fill the entire space and leave holes. That this
does not happen is shown in Figures 15 and 16, which
present photographs shows what does happen. Not un-
expectedly, as the culture approaches confluency five-
sided colonies disappear to be replaced by a patchwork
of seamless rivers of randomly organized cells. But are
they?
Figure 14. Rotational symmetry of a 46-cell
model close-packed isomorphic pentagonal
colony. (a) N = 1-fold (pentagons are shaded);
(b) N = 5-fold and (c) N = 6-fold.
Figure 15. Rotational symmetry of confluent
NHK cultured in SFM (0.1 mMCa++, d7).
Entire colony stains blue with Mallory’s
trichrome. (a) N = 1-fold; (b) N = 3-fold; (c)
N = 4-fold; and (d) N = 5-fold.
Figure 16. Rotational symmetry of confluent
NHK colony cultured in SFM (2 mMCa++,
d7). Entre colony stains blue with Mallory’s
Trichrome stain. (a) N = 1-fold; (b) N = 3-fold;
N = 4-fold and N = 5-fold.
A secondary passage NHK culture (NF171P2, GF+,
0.1) was plated at 500 cells per dish and fed low calcium
(0.1 mM Ca++) SFM for seven days. The cells were
fixed and stained with Mallory’s Trichrome stain. Figure
15(a) is a photograph showing a confluent area which
stains entirely blue as expected if all the keratinocytes
are undifferentiated in rapidly growing culture. Figures
15(b)-(d) show the results of Markham rotational analy-
ses when an arbitrary center of gravity is picked as the
center of rotation. Enhancement of individual cell pro-
files was more evenly distributed and stronger when the
reflective angle of rotation was 120˚ (Figure 15(b), N =
3). A cruciform enhancement of cell profiles images was
seen when the reflective angle of rotation was 90˚ (Fig-
ure 15(c), N = 4). By contrast, a peripheral ring of cell
profile enhancements was observed when the reflective
angle of rotation was 72˚ (Figure 9(d), N = 5). There
was no obvious enhancement of cell profile images
when the reflective angle was 60˚ (data not shown). In
summary, Figure 9 suggests that 3-, 4-, and 5-fold re-
flective symmetries are present and compatible as the
predominant packing numbers in confluent cultures
grown in low calcium (0.1 mM) SFM.
A secondary passage NHK culture (NF171P2, GF+, 2)
was plated at 500 cells per dish and fed high calcium (2
mMCa++) SFM for seven days. The cells were fixed and
stained with Mallory’s Trichrome stain. Figure 16(a) is a
photograph showing a confluent cell culture area, which
stains entirely blue as expected if all the cells are undif-
ferentiated in a rapidly dividing culture. Figures 16(b)-
(d) show the results of Markham Rotational analyses
when an arbitrary center of gravity is picked as the cen-

J. J. Wille / Natural Science 3 (2011) 866-883
877
877
ter of rotation. Enhancement of individual cell profiles
was more evenly and stronger when the reflective angle
of rotation was either 120˚ or 90˚, Figure 16(b), N = 3
and Figure 16(c), N = 4, respectively. By contrast, a
peripheral ring of cell profile enhancements observed
when the reflective angle of rotation was 72˚ (Figure
10(d), N = 5). No evident cell profile enhancement was
seen when the reflective angle of rotation was 60˚ (data
not shown). In summary, Figure 16 suggests that 3-, 4-,
and 5-fold reflective symmetries are present and com-
patible as the predominant packing numbers in confluent
cultures grown in high calcium (2 mM) SFM.
3.12. Validation of 5,6-Fold Mixed
Symmetries
Figure 17 presents a model of five-six rotational sym-
metry encountered in a “Chinese Window” [22]. Although,
the loci of the pentagons and hexagons are highly regu-
lar in the identity image (Figure 17(a)), Markham rota-
tional analysis around the center of gravity produces
strong enhancement of individual pentagons shapes and
hexagon shapes exactly as they occur in the identity im-
age only when the reflective angle of rotation is either
72˚ (Figure 17(c), N = 5) for pentagons, and 60˚ (Figure
17(d), N = 6) for hexagons. Neither pentagons nor hexa-
gons are enhanced when the reflective angle of rotation
is 90˚ (Figure 17(b), N = 4).
Figure 17. Validation of Markham-Rotational Symmetry Test.
Chinese Window with 5-fold and 6-fold rotational symmetry.
From Elementary Particles. C. N. Yang. Princeton Unversity
Press, p. 32 (1961).
3.13. Rotational Symmetry in
Pseudocellular Aggregates
Microscopic examination of histological specimens of
dead but once living material has turned up curious ex-
amples of highly ordered arrays of close-packed po-
lygonal “cellular units”. These “curiousities” were stud-
ied in the late 19th and early 20th century and reviewed in
detail elsewhere [7]. Whether these artifacts represent
the remnants of a living organization or are examples of
crystallization upon a living pre-pattern remains un-
solved.
Case 1: The Molluscan Shell. In the early stages of
calcification sections of the molluscan shell as shown in
Figure 18 [7] (see page 655) display a network of poly-
gonally-shaped “cells”. It isn’t known if these are truly
cells or artifacts of calcification. Here we assume that
they are the products of living cells that serve, perhaps,
as a pre-pattern for the later stages of calcification. We
examined the frequency of the three polygons: hexagons
(0.657), pentagons (0.286), and quadragons (0.057).
Statistical calculation showed a significant deviation of
the frequency from chance if it is assumed that all the
polygons were hexagonal (P < 0.001) or even if the fre-
quencies of hexagons and pentagon are assumed to be
equal (P < 0.01). Nearest neighbor analysis of cell con-
tacts among the three polygon types and 6 expected fre-
quency classes of contacts showed there was no signifi-
cant deviation from chance expectations given their ob-
served relative frequencies. In this respect, molluscan
shell “cell aggregates” share the same statistical behav-
ior as all of the above living tissues analysed. Table 2
summarizes the data on polygon frequencies in cellular
aggregates for all living systems. The table also shows
that the average frequencies for pentagons (52.5), hexa-
gons (28.3), heptagons (8.2) and quadragons (10.7). The
ratio of average percent of pentagons to average percent
of hexagons shown at the bottom of the table was 1.9,
i.e., approximately two to one.
(a) (b)
Figure 18. Molluscan shell “cellular aggregates” (a) early
stage; (b) later crystalization stage. Photograph after Capenter
(Figure 296 in Growth and Form, Thompson, 1943).

J. J. Wille / Natural Science 3 (2011) 866-883 878
Table 2. Frequency of polygonally-shaped unit-cells in model cell aggregates.
Artificial Cells Pentagons (%) Hexagons (%) Heptagons (%) Quadragons (%) Edge@ Effects/(Clustering)
Clay spheres 55.9 40.7 0 3.4 +/(+)
Gel-cells 10.2 73.5 16.3 0 +/(+)
Calcospherite 49.3 50.7 0 0 –/(+)
Mean ± SD 38.5 ± 20.2 55 ± 13.7 5.4 ± 7.7 1.1 ± 1.6 P:H = 0.7
@, Nearest neighbor statistical cell contact statistical analysis (+), significant (P < 0.001).
3.14. Model Artificial Cellular Aggregates
It was of interest to examine three definitely non-liv-
ing cellular aggregates for evidence of the number and
arrangement of pentagonal and other polygonal shaped
“cells”. Four different cases are examined below:
Case 1: Leduc’s Gelatin Ferrocyanide Diffusion Reac-
tion Pattern. Figure 19 presents a photograph of an arti-
ficial cellular tissue formed by the diffusion in gelatin of
drops of a solution of potassium ferrocyanide after Le-
duc’s diffusion experiments [7] (see Figure 181, page
501). Here, we have enumerated the 49 interior polygons
as 36 hexagons (green), 8 heptagons (blue) and 5 penta-
gons (clear) with frequencies of 0.735, 0.163, and 0.102,
respectively. Further statistical calculations showed sig-
nificant deviation from chance on the assumption that all
of the interior polygons are hexagons (P < 0.01) or if of
the observed polygon types are equally probable (P <
0.001). Nearest neighbor analysis of the 6 classes of ex-
pected cell contacts generating 120 observed cell con-
tacts among the three different polygon types (see lines
connecting cells) revealed a statistically random out-
come based on the observed polygon frequencies. By
contrast, a highly significant deviation from chance ex-
pectations occurred when the frequency of the three
polygon types were made equal (P < 0.001). Inspection
of the locations of the pentagons and heptagons relative
to the hexagons indicates that the former lie at the pe-
riphery and fall into the phenomenon of “edge effects”
when conditions are not perfectly symmetrical for the
production of regular hexagons by mutual interaction of
polygons.
Case 2. “Crowded” Clay Spheres. Figures 20(a)-(d)
is a photograph reproduced here after Honda [7]. It
models the situation where deformable clay spheres are
first organized into closely packed configuration (a), and
later when crowded together (c) they form a regular
hexagonal array. By contrast, when the clay spheres are
randomly arrayed prior to close packed configuration (b),
and then crowded they form a cellular aggregate with an
irregular hexagonal array (d). We enumerated the 59
interior polygon types as 24 hexagons (clear), 33 penta-
gons (green) and 2 quadragons (yellow) with frequencies
of 0.41, 0.56, and 0.03, respectively. Statistical calcula-
tions performed using these frequencies showed a highly
Figure 19. Physico-chemically-generated “Leduc figure” re-
sembling cellular organization of living tissues (photograph
after Leduc Figure 181 in Growth and Form, Thompson, 1943).
Hexagonally-shaped “cells” are colored green, pentagonally-
shaped cells are not colored, and cell with greater than 6 sides
are colored blue. Cell associations with surrounding cells are
shown as lines across cell boundaries.
Figure 20. Deformable clay spheres (photograph after Honda,
1983). (a) uniformly close-packed spheres prior to compres-
sion; (c) “a” after compression; (b) randomly arranged spheres
prior to compression; (d) “b” after compression. A cluster of
hexagon are not colored; cluster of pentagons are colored green;
polygons with have more than 6 sides are colored yellow.

J. J. Wille / Natural Science 3 (2011) 866-883
879
879
significant deviation from chance expectation on the
assumption that all of the 59 polygons are hexagons (P <
0.001). Likewise, the statistical expectation that all of
the polygons in the polygonal array are pentagons was
significantly different from that observed (P < 0. 001) or
even if it is assumed that the frequencies of the three
polygon types were set equal (P < 0.01). Nearest
neighbor statistical analysis of the 121 cell contacts be-
tween the 6 predicted frequency classes of cell contacts
among the three polygon types showed a significant de-
viation from chance based on the observed frequencies
of each polygon type (P < 0.01). A highly significant
deviation from chance expectations was obtained by
assuming that the 116 cell contacts were restricted to
equal frequencies of pentagons and hexagons (P < 0.001).
Although, the topological location of pentagons and
hexagons are clearly separated and clustered by polygon
type, the separation appears to be a mutual peripheral
edge effect with no central preference of hexagons.
Case 3: Calcospherite: A Diffusion-Crystalizaton Pat-
tern. Figure 21 is a photograph reproduced from Thomp-
son [7] (see page 655) of stages in the formation of a
calcospherite that are formed by bringing a soluble salt
of lime into solution with a colloid medium (albumin)
and then to precipitate it out in the form of a carbonate.
After several days the calcium carbonate is deposited in
the form of rounded concretions, which have tendency to
aggreegte in layers and then assume an often regular
hexagonal polygonal outline. This purely physical phe-
nomenon generates a cellular tissue that resembles a
living tissue. Here we enumerate the 67 interior polygo-
nal outlines (Figure 21, right), that consist of 34 hexa-
gons, and 33 pentagons with frequencies of 0.508 and
0.493, respectively. Statistical calculations of the ex-
pected outcome if it is assumed that all of the polygons
are either all hexagons or all pentagons yielded highly
significant deviations from chance based on the ob-
served frequencies of each polygon type (P < 0.001). If
one assume that hexagons and pentagons are equally
probable, then there is no statistical deviation from
chance expectations relative to their observed frequen-
cies (P < 0.3), indicating that sphetites pack in equal
proportions of pentagons and hexagons. Nearest neigh-
bor statistical analysis of the 115 cell contacts between
the 3 expected frequency classes and two polugon types
revealed a highly significant deviation from chance ex-
pectations based on the observed frequencies of penta-
gons and hexagons (P < 0.001). A highly significant de-
viation from chance was also the case for cell contacts
between the 3 expected frequency classes when it is as-
sumed that hexagons and pentagons are equally probable
(P < 0.001). No preferred location of the two types of
polygons could be discerned, but there is a tendency for
pentagons to be clustered.
Table 2 summarizes the results presented on the fre-
quency of the different polygonally-shaped cell outlines
obtained from analyzing three artificial or model cell
aggregates. The average percents of pentagons, hexa-
gons, heptagons, and quadragons were 38.5, 55, 5.4 and
1.1, respectively. The table also shows that all three arti-
ficial systems show clustering of similar polygon types
and two demonstrated clear edge effects. In addition, we
calculated the ratio of the average percent pentagons to
the average percent hexagons (P:H = 0.7). This indicates
that hexagon packing is preferred over pentagonal pack-
ing for artificial cell aggregates.
Case 4: Autotactic Swimming Patterns in Motile Cul-
tures of Tetrahymena [23]. Figure 22 presents the pho-
tograph of the kinetics of Benard-type-cell swimming
pattern development. The 90 second time photograph
displays a stable pattern and was used for further analy-
sis. For our purpose here, we performed an analysis of
the frequency of individual foci (cell aggregates) having
five, six or more nearest neighbors in the regular array of
close packed foci. Foci located around the perimeter or
not in focus were eliminated from the analysis. This
leaves foci that are centered in the second and third
Figure 21. Physically-generated CALCOSPHERITES (PHO-
TOGRAPH AFTER HARting. Figure 295 cited in Growth and
Form, Thompson, 1943). Concretions of calcium carbonate
deposited in egg white.
Figure 22. Kinetics of development of Benard-cell foci in
swimming patterns of cultures of the ciliated protozoan,
Tetrahymena pyriformis W (after Wille and Ehret, 1968).

J. J. Wille / Natural Science 3 (2011) 866-883 880
horizontal rows of the interior for analysis. The foci can
be connected by diagonal lines that form a typical dou-
ble- pitched pattern akin to the Fibonnaci sequence seen
Sunflower seed crowns. Here we enumerate the 22 inte-
rior polygonal outlines (Figure 23), that consist of 12
hexagons, and 10 pentagons with frequencies of 0.54
and 0.46, respectively. Statistical calculations of the ex-
pected outcome if it is assumed that all of the polygons
are either all hexagons or all pentagons yielded highly
significant deviations from chance based on the ob-
served frequencies of each polygon type (P < 0.001). If
one assume that hexagons and pentagons are equally pro-
bable, then there is no statistical deviation from chance
expectations relative to their observed frequencies (P <
0.3), indicating that foci pack in equal proportions of
pentagons and hexagons. Nearest neighbor statistical
analysis of the 44 foci contacts between the 3 expected
frequency classes and two polygon types revealed a sig-
nificant deviation from chance expectations based on the
observed frequencies of pentagons and hexagons. (P <
0.01). A preferred location of the two types of polygons
could be discerned, and there is a tendency for pentagons
to be clustered.
Figure 23 present a histogram plot of the frequency of
foci having five (5), six (6) or seven (7) nearest neighbors
as a function of their position from left (L) to right (R) in
the second(grey bar) and third (white bar) horizontal
rows. A preferred location of the two types of polygons
could be discerned, and there is a tendency for pentagons
to be clustered centrally. Further inspection of the histo-
gram shows that foci located between the 4th and 7th po-
sition have 75% (6/8) of foci with five nearest neighbors,
and only 25% (2/8) foci with six nearest neighbors. By
contrast, 80% (8/10) foci located nearer the margins
(edges) of the dish at left positions (1-3), and right posi-
tions (8 and 9) have six neighbors, 10% (1/10) with
Figure 23. Histogram plot of nearest neighbor analysis of
Benard-cell foci seen in Figure 22. Data for row 2, red bar;
data for row 3, clear bar.
seven nearest neighbors, and 10% (1/10) with five near-
est neighbors. These results are unexpected on the notion
that edges propagate odd numbers of nearest neighbors
in close packed arrays. Here, dynamic stability of Benard-
cells yields a predominance of odd numbers, i.e. 5, of
nearest neighbors in the interior of the regularly ordered
autotactic cell aggregation pattern. A possible explana-
tion lies in the initiation or “seeding” effect of foci for-
mation at the dish edges as seen at the 30 second and 60
second time points in the kinetics of pattern formation
(Figure 22). This suggests that the formation of Benard-
cells in the interior are less stable and dependent on the
seeding effect at the edges. This idea also confers a new
insight into the possibility that pentagonal symmetry
patterns on a cellular level of organization reflects a
condition of dynamic instability relative to hexagonal
packing arrangement.
4. DISCUSSION
“It is in the likeness of this self-developing series that
the faculty of propagation is, in my opinion formed.”
Johannes Kepler as quoted by Mario Livio [24].
D’Arcy Thompson [7] recognized the significance of
symmetry in the shapes of animal bodies ranging from
lowly foaminferan amoeboid shells to highly developed
molluscan shells, and called attention to the possibility
that artificial cellular aggregates can assume hexagonal
and pentagonal packing patterns similar to those ob-
served in histological preparations of animal cells. To
quote him, “Broadly speaking, nevertheless, it is evident
that massed-cells in many forms of tissues tend towards
polyhedral forms that approximate to those demanded by
the theory, as was earlier pointed out by several authors
quoted by Thompson [7] (who cites Berthold, ’86; Er-
reera, ’86, ’87, and Chabray, ’87), and the conclusion is
irresistible that this grouping of cells conforms to the
same general physical laws as that of non-living bodies”.
The truth of this statement remains unanswered to this
day. It is not surprising then to find that proliferating
colonies of human basal epidermal keratinocytes form
congeries of cells that also assume a pentagonal shape,
which persists through many cellular generations. Our
approach has been to validate this finding first in cul-
tured epidermal keratinocytes and then to generalize it as
a universal phenomenon not strictly limited to human skin
but to a variety of vertebrate tissues. For human kerati-
nocyte colonies we accomplished this by establishing the
order of rotational symmetry using the Markham “twist”
technique. For other cell types we applied the technique
of statistical evaluation for the frequency of appearance
and nearest neighbor frequency analysis. This demon-
strated a nonrandom occurrence of pentagonal shaped
cells in colonies, and a lack of clustering and edge ef-

J. J. Wille / Natural Science 3 (2011) 866-883
881
881
fects (except for chick embryo cells) in the distribution
of cell shapes within colonies. We also performed ex-
periments on partially disaggregated colonies and noted
the presence of five-sided cells and their orientation and
connections to neighboring five-sided cells. We believe
these studies support an intrinsic and pervasive role of
pentagonal symmetry in the organization of epithelial
cells and their cellular tissues. These observations also
raise an important question of what underlies the forma-
tion and persistence of pentagonal colony symmetry
under the changing dynamics of cell growth and division.
This situation is highly unusual assuming hexagonal
packing to be the rule for close packing of similar sized
cells [8]. Strict adherence to pentagonal symmetry is lost
upon coalescence of individual colonies in confluent
monolayer cultures and instead, a “Chinese Window”
mosaic” composed of four- five- and six-sided rotation-
ally enhanced images appear. Photographic and statisti-
cal analyses showed that the frequency of pentagons and
hexagons in cellular monolayers is not due to chance. A
highly significant deviation from random associations
was found if the frequencies of the three different po-
lygonal classes were made equal or if only five- or six-
sided cell contacts were allowed as expected. We also
examined the frequency of “nearest neighbors” in cellu-
lar monolayers of each of the six different living systems
which displayed all permutations of cell-cell contacts
between four- five- six- and seven-sided cells. The sta-
tistical analyses demonstrated a random association of
cell contacts between the different polygonal classes. Si-
milar analyses were performed on three different model
artificial cellular tissues that have a remarkable resem-
blance to living cellular tissues: one formed by crowding
deformable clay spheres into a close packed array from
an initially random configuration, another artificial cel-
lular tissue formed by diffusing gelatin with a solution of
ferrocyanide and so-called calcosperites. In all three
cases statistical analysis of nearest neighbor association
of cell contacts between four- five- and six-sided clay
spheres and the five- six- and seven-sided calcosperites
and gelatin unit cells based on their relative frequencies
demonstrated highly significant deviation from random
associations. Other deviations from random associations
were found in the topological locations of pentagons and
hexagon favoring “edge effects” for the boundary posi-
tioning of pentagons and heptagons relative to centrally
located hexagons. By contrast, edge effects do not ac-
count for the location of pentagons found in living cel-
lular tissues. The significance of these observations is
not yet apparent, but evidence obtained by observing
retraction of cell-cell contacts during wound healing and
cell dissociation indicates that individual keratinocyte
cells are, in fact, are five-sided. Further, we speculate
that keratinocytes display bilateral symmetry rotated
around a perpendicular axis, dividing left and right sides,
that runs from one vertex (head-end) and bisects the op-
posite base side (tail-end), thus, creating a five-sided
figure. It is well known that regular pentagons cannot
tile a plane as do triangles and other regular even-sided
polygons. But a plane can be completely tiled using each
of two irregular shaped pentagons or with a pair of
shapes [25] a second tiliing pattern that employs planar
quasiperiodic coverage is Gummelt’s decorated deca-
gons [26], that Hark back to Kepler’s decagons [24]. The
appearance of coalesced keratinocyte and chick embryo
confluent monlayer cultures seem to obey this principle.
There we observe a “Chinese Window” configuration
having two or more polygonally-shaped cells existing
side-by-side. The subject of close packing of hard
spheres with five-fold symmetry has been approached
and a solution given in the plane by constructing over-
lapping layers of concentric pentagons with alternating
odd number of spheres in the first layer and an even
number of spheres in the second plane such that an infi-
nite space filling structure or tessellation can be con-
structed [27]. Space filling decagon-sided polygons were
proposed and seen in crystallographic analysis of metal
alloys as examples of planar quasicrystals composed of
overlapping rhomboids [28,29]. Of course cells are not
hard spheres and the adhesive forces applied to them by
their attachments and detachments to one another and to
the substrate have been the topics of many previous
studies [30-32]. This is not the situation when examining
frequencies of cell shapes and distribution of cell shapes
within colonies for artificial cell aggregates. What then
is the significance of pentagonal versus other polygonal
cell-cell packing patterns for the dynamics of cell growth
and differentiation? Before answering that we make here
a connection between pentagonal symmetry witnessed in
cell colonies and the Golden Ratio, which embodies the
well-known number PHI(φ), the ratio of two numbers of
the Fibonacci sequence. As a historical note, Johannes
Kepler, the 16th C mathematician, is quoted by Mario
Livio [24] as saying, “It is in the likeness of this, self-
developing series (referring to the recursive properties of
the Fibonacci sequence) that the faculty of propagation
is, in my opinion formed”. This insight may underlie the
growth pattern of propagating colonies of basal kereati-
nocyte which resembles the generative or equiangular
spiral, itself generated by continued cell division of unit
shaped cells, and resulting in a space-filling two-dimen-
sional tiling pattern that conserves non-overlapping and
meets the rules of minimal energy configurations [7].
Further studies are needed to examine the dynamics of
cell growth and differentiation as they affect the cellular
arrangement of close packed arrays of cells in tissues.

J. J. Wille / Natural Science 3 (2011) 866-883 882
Earlier theoretical modeling [33] of the development of
pentagonal and radial symmetry, primarily in early em-
bryology of Echinoderms, employed a two-variable re-
action-diffusion mathematical scheme much like the
original Turing model with special initial conditions and
fixed diuffsuion coefficients for the diffusible chemical
entities [34]. This model was confirmed by numerical
calculations and waveform iterations that predicted the
formation of a pentagonal “seed” within the embryo that
lays the basis for perpetuation of overall radial and pen-
tagonal symmetry of the adult. Much still needs to be
done to understand the underlying molecular basis of
these models. In this regard, the past decade has wit-
nessed advances underlying symmetry breaking, which
involve directional assembly of cytoskeletal polymers,
molecular motors that drive cellular motion, and the
regulatory action of GTPases, kinases and proteases that
provide feedeback mechanisms for epithelial cell polar-
ity [35].
REFERENCES
[1] Weyl, H. (1952) Symmetry. Princeton University Press,
Princeton.
[2] Goddard, T., Huang, C. and Ferrin, T. (2004) New ap-
proaches for visualizing virus capsids. Poster for NCRR
Principle Investigator Meeting, 1-7. www.cgl.ucsf.edu.
[3] Hickman, C.P.Sr., Hickman, C.P.Jr. and Hickman, F.M.
(1974) Integrated principles of zoology. 5th Edition, The
CV Mosby Company, St. Louis.
[4] Romer, A.S. (1974) The vertebrate body: A shorter ver-
sion of the second edition. W.B. Saunders Company,
Philadelphia.
[5] Breder, C.M.Jr. (1955) Observations on the occurrence
and attributes of pentagonal symmetry. Bulletin of
American Museum of Natural History, 106, 173-220.
[6] Frankel, J. (1984) Pattern formation in ciliated protozoa.
In: Malacinski, G.M. and Bryant, S.V., Eds., Pattern
Formation: A Primer in Developmental Biology, Mac-
millan Publishing Company, New York, 163-196.
[7] Thompson, D.W. (1943) On growth and form. New Edi-
tion, Cambridge University Press, The Macmillan Com-
pany, Cambridge.
[8] Honda H. (1983) Geometrical models for cells and tis-
sues. International Review of Cytology, 83, 191-246.
doi:10.1016/S0074-7696(08)62339-6
[9] Ehret, C.F. (1967) Paratene theory of the shapes of cells.
Journal of Theoretical Biology, 15, 263-272.
doi:10.1016/0022-5193(67)90207-X
[10] Othmer. H.G. and Scriven, L.E. (1971) Instability and
dynamic pattern in cellular networks. Journal of Theo-
retical Biology, 32, 507-537.
doi:10.1016/0022-5193(71)90154-8
[11] Chen, C.S. Mrksich, M., Huang, S., Whitesides. G.M.
and Ingebar, D.E. (1997) Geometric control of cell life
and death. Science , 276, 1425-1428.
doi:10.1126/science.276.5317.1425
PMid:9162012
[12] Li, R. and Bowerman, B. (2010) Symmetry breaking in
biology. In: Symmetry Breaking in Biology. Cold Spring
Harbor Perspect Biology, Cold Spring Harbor Labora-
tory Press, Cold Spring Harbor, 2, 1-6.
[13] Aoyama, Y. and Kitajima, Y. (1999) Pemphigus vul-
garis-IgG causes a rapid depletion of desmoglein 3 (Dsg
3) from the Triton-X-100 soluble pools, leading to the
formation of Dsg 3-depleted desmosomes in a human
squamous carcinoma cell line, DJM-1 cells. Journal of
Investigative Dermatology, 112, 67-71.
doi:10.1046/j.1523-1747.1999.00463.x
PMid:9886266
[14] Penas, P.F., Gomez, M., Buezo, G.F., Rios, L., Yanez-Mo,
M., Cabanas, C., Sanchez-Madrid, F. and Garcia-Diez, A.
(1998) Differentiation expression of activation epitopes
of beta-1 integrins in psoriasis and normal skin. Journal
of Investigative Dermatology, 111, 19-24.
doi:10.1046/j.1523-1747.1998.00232.x
PMid:9665381
[15] Inamatsu, M., Matsizaki, T. Iwanari, H. and Yoshizatu, K.
(1998) Establishment of rat dermal papilla cell lines that
sustain the potency to induce hair follicles from afollicu-
lar skin. Journal of Investigative Dermatology, 111,
767-775. doi:10.1046/j.1523-1747.1998.00382.x
PMid:9804336
[16] Tateno, C. and Yoshizato, K. (1999) Growth potential
and differentiation capacity of adult rat hepatocytes in
vitro. Wound Repair and Regeneration, 7, 36-44.
doi:10.1046/j.1524-475X.1999.00036.x
PMid:10231504
[17] Rambo, C.C. and Szego, C.M. (1983) Estrogen action at
endometrial membranes: Alterations in luminal surface
detectable within seconds. Journal of Cell Biology, 97,
679-685. doi:10.1083/jcb.97.3.679
PMid:6885915
[18] Markham, R., Frey, S. and Hills, G.J. (1963) A photo-
graphic image enhancement technique applied viral str-
uctures. Virology, 20, 88.
doi:10.1016/0042-6822(63)90143-0
[19] Ayoub, P. and Sklaar, G. (1983) A Modification of the
Mallory connective tissue stain as a stain for keratin.
Oral Surgery, 16, 80-581.
[20] Wille, J., Pittelkow, M.R., Shipley, G.D. and Scott, R.E.
(1984) Integrated control of growth and differentiation of
normal human prokeratinocytes cultured in serum-free
medium: Clonal analyses, growth kinetics and cell cycle
studies. Journal of Cellular Physiology, 121, 31-44.
doi:10.1002/jcp.1041210106
PMid:6207187
[21] Aroeti, B. (2007) Published image. Chroma Technology
Corp, Institute for Life Science, Hebrew University, Je-
rusalem.
[22] Yang, C.N. (1962) Elementary particles. Princeton Uni-
versity Press, Princeton, 51.
[23] Wille, J.J. and Ehret, C.F. (1968) Circadian rhythm of
pattern formation in populations of free-swimming or-
ganism, Terahymena. Journal of Protozoology, 15, 789-
797.
[24] Livio, M. (2002) The golden ratio. Broadway Book, New
York, 110.
[25] Penrose, R. (1999) The emperor’s new mind. Oxford
University Press, Oxford, 172-173.

J. J. Wille / Natural Science 3 (2011) 866-883
883
883
[26] Urban, K.W. (1998) From tilings to coverings. Nature,
396, 14-15. doi:10.1038/23806
[27] Bagley, A. (1965) A dense packing of hard spheres with
five-fold symmetry. Nature, 208, 674-675.
doi:10.1038/208674a0
[28] Penrose, R. (1974) The role of aesthetics in pure and
applird mathematical research. Bulletin of the Institute of
Mathematics and Its Applications, 10, 266-271.
[29] Steinhardt, P.J., Jeong, H.-C., Saitoh, K., Tanaka, E., Abe,
E. and Tsai, A.P. (1998) Experimental verification of the
quasi-unit cell model of quasicrystal structure. Nature,
396, 55-57. doi:10.1038/23902
[30] Steinberg, M. (1963) Reconstruction of tissues by disso-
ciated cells. Some morphogenetic tissue movements and
the sorting out of embryonic cells may have a common
explanation. Science, 141, 401-408.
doi:10.1126/science.141.3579.401
PMid:13983728
[31] Beysens, D.A., Forgacs, G. and Glazier, J.A. (2000) Cell
sorting is analogous to phase ordering in fluids. Pro-
ceedings of the National Academy of Sciences of the
United States of America, 97, 9467-9471.
doi:10.1073/pnas.97.17.9467
[32] Forgacs, G. and Foty, R.A. (2004) Biological implica-
tions of tissue viscoelasticity. In: Deutsch, A., Folke, M.,
Howard, J. and Zimmermann, W., Eds., Function and
Regulation of Cellular Systems: Experiments and Models.
Biskhauser, Basel, 269-277.
doi:10.1007/978-3-0348-7895-1_27
[33] Arago’n, J.L., Torres, M., Gil, D., Barrio, R.A. and P.K.
Maini, P.K. (2000) Turing patterns with pentagonal
symmetry. Physical Review E, 65, 051913, 1-9.
[34] Turing, A.M. (1952) The chemical basis of morphogene-
sis. Philosophical Transactions of the Royal Society B:
Biological Sciences, 237, 37-72.
doi:10.1098/rstb.1952.0012
[35] Li, R. and Bowerman, B. (2010) Symmetry breaking in
biology. Cold Spring Harbor Laboratory Perspectives in
Biology, 2a003475, 1-18.