Journal of Quantum Informatio n Science, 2011, 1, 1-6
doi:10.4236/jqis.2011.11001 Published Online June 2011 (http://www.SciRP.org/journal/jqis)
Incomparability through Superposition of Many
States under LOCC
Amit Bhar
Department of Mat hematics, Jogesh Chandra Chaudhuri College,
Kolkata, India
Received April 1, 2011; revised May 21, 2011; accept ed J un e 1, 2011
Abstract
In this paper we investigate the global property of the states constructed through superposition of many states
by using the concept of incomparability under LOCC as the inherent property of the states. In our work we
are able to form a bridge between comparable and incomparable classes of states through linear
superposition of a states and the concept of strong incomparability criterian under LOCC. PACS number(s):
03.67.Hk, 03.65.Ud, 03.65.Ta, 03.67.–a, 89.70+c.
Keywords: LOCC, Entanglement, Incomparability, Superposition
1. Introduction
Quantum information, quantum entanglement and quantum
computation become the most attracting and useful
branches of quantum mechanics. Specially quantum
entanglement, has been regarded as the fundamental
resource was firstly introduced by Einstein, Podolsky
and Rosen (EPR) [1] and by dingeroSch  [2]. In [1] EPR
raised a question wheather quantum mechanics is local
and complete theory or not. The most significant progress
towards this resolution of EPR problem was made by
Bell [3] through famous Bell’s inequality and the latter
feature of quantum mechanics called usually a non-local
theory. Quantum entanglement mainly reveals some
non-local behaviors of quantum mechanics and performs
many informative tasks like Teleportation, Dense Coding,
Cloning and many others [4-6]. Such type of informative
tasks are possible if and only if quantum entanglement
exists positively. So characterization and quantification
of entanglement [7,8] are both not only necessary
important but also a serious task to the scientists in the
field of quantum information and computation.
For a few decades many researchers tried to observe
the underline physics of quantum entanglement [9,10] and
suggested many algorithms and concepts to prove some
new results for both characterizing and quantification of
quantum entanglement [11,12]. Recently, Lindu, Popescu
and Smolin [13] have raised the following problem: given
a bipartite quantum state  and a certain decom-
position of it as a superposition of two other states.
In =

  what is the relation between
the entanglement of

and those of the two states in
the superposition? They also considered the following
examples to illustrate the above problem.
11
=00 11
22
  and
11
=22





where 11 =00 11
22

. The first example very
clearly explains that

is a maximally entangled state
but each state is fully separable [14,15]. That is,
superposition of fully separable states form a maximally
entangled state ,and the second example provides the most
opposite information of the first i.e.

 is separable
but each state is maximally entangled. So just observing
the above facts it is quite understood that the
superposition of states will give some new physics and
concepts in the area of detection and characterization of
quantum entanglement.
The aim of this paper is to use the notion of
incomparability [16-18] under LOCC which is a branch
of memorization criteria to discuss some interesting
situations in which the state property incomparability
under LOCC is remained same or not to the state generated
through the superposition of many states.

A. BHAR
2
This paper is organized as follows: first, in section II,
we give the idea of incomparability and some fundamental
results and concepts of this topic. Section III is devoted
to discuss the main results. The paper is ended in section
IV with a brief conclusion of our new achievement.
2. Notion of Incomparability
For achieving our goal of our work it is now quite
essential to define the condition for a pair of states to be
incomparable with each other and the notion of
incomparability of a pair of bipartite pure states is a
consequence of Nielsen’s [5,19] famous and fundamental
majorization criterion. To illustrate the notion suppose we
consider the conversion of the pure bipartite state

to  shared between two parties, say, Alice and Bob
by deterministic LOCC with the consideration that the
pair

, is in their Schmidt bases

,
AB
ii
with decreasing order of Schmidt coefficients:
=1
=d
iAB
iii
 , =1 =d
iAB
iii
 , where
0
1 ii


and 0,
1 ii


for 1,,1,2,=

di  and
i
d
i
i
d
i

 1=1= =1= . The Schmidt vectors corresponding
to the states | and | are

d




,,, 21 

and

d




,,, 21 
. Now the Nielsen’s criterion
 is possible with certainty under LOCC if
and only if 

is majorized by ,


denoted by



 and described as,
dk
i
k
i
i
k
i
,1,2,=
1=1=
   (1)
The vital physical phenomenon that the non-increasement
of entanglement by LOCC is observed as a consequence
of the notion: If  is possible under LOCC
with certainty, then
 
EE [where



E
denote the von-Neumann entropy of the reduced density
operator of any subsystem and known as the entropy of
entanglement]. Now in case of failure of the above
criterion (1), it is usually denoted by 
. Also it
may happen that  under LOCC. And if it
happens that both 
 and   then
we denote it as 
 and describe 

as a pair of incomparable states. One of the peculiar
features of such incomparable pairs is that we are unable
to say which state has a greater amount of entanglement
content than that of the other. For 22 systems there
are no pair of incomparable pure entangled states as
described above. Now we want to mention explicitly the
criterion of incomparability for a pair of pure entangled
states , of nm system where


min,= 3mn .
Suppose the Schmidt vectors corresponding to the two
states are

321,,aaa and

321 ,, bbb respectively,
where , 0>>> , 0>>>321321bbbaaa 1=
321aaa


321
=bbb


. Then it follows from Nielsen’s criterion
that ,

 are incomparable if and only if, either of
the pair of relations.
113 3
11 3 3
>& >
>&>
abab
baba
(2)
will hold.
A most powerful usefulness of incomparability is that
if a pair of states is incomparable then we can not
compare the amount of entanglement of the pair.
3. Main Results
Now we arrive at that stage where we can discuss some
newly interesting basic consequences of superposition
through the concept of incomparability under LOCC. Now
for our discussion we take two parties say A and B. Now
=ψ

  (3)
where 22
=1   and
=ψ



  
(4)
where 1=
22



 be their explicit forms. Now we
write the explicit forms of ψ, ψ,

and


in
the following
2
=0
2
=0
2
=0
2
=0
ψ=,
=,
ψ=
=
i
i
j
j
i
i
j
i
aii
bjj
ii and
jj









(5)
Now we discuss the problem that we have already
raised in this paper in the following cases through imposing
restrictions on ,,,,






and also iiii ba


,,, for all
i = 0,1,2.
CASE: I
For two states

and 

of two parties A and B we
have imposed the following restriction that the states
ψ, ψ

and

and


are mutually incomparable
to each other under LOCC. So the fundamental question
is wheather this incomparability under LOCC of states
becomes the global phenomenon of  and


i.e.
clearly to say that

and   are incomparable
under LOCC to each other or not and we illustrate all the
results through the following tabular form:
CASE: II
Two states

and


of two parties A and B with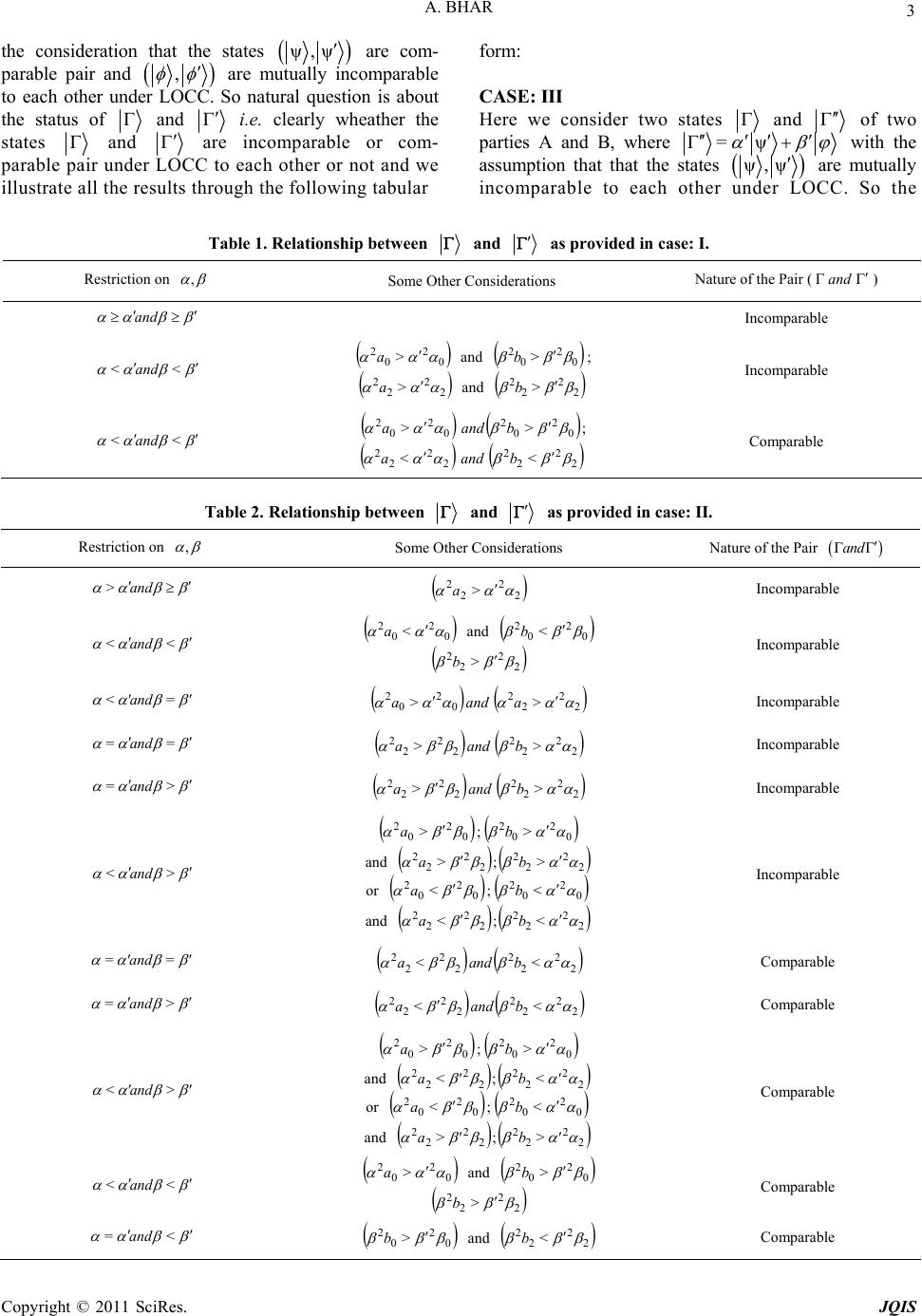

A. BHAR
3
the consideration that the states

ψ,ψ are com-
parable pair and

,


 are mutually incomparable
to each other under LOCC. So natural question is about
the status of  and 
 i.e. clearly wheather the
states  and 
 are incomparable or com-
parable pair under LOCC to each other or not and we
illustrate all the results through the following tabular
form:
CASE: III
Here we consider two states  and


 of two
parties A and B, where =ψ


 
 with the
assumption that that the states

ψ,ψ are mutually
incomparable to each other under LOCC. So the
Table 1. Relationship between

and


as provided in case: I.
Restriction on


, Some Other Considerations Nature of the Pair (


and )




   and Incomparable




 << and


0
2
0
2>


a and


0
2
0
2>


b;


2
2
2
2>

 a and


2
2
2
2>


b
Incomparable




 << and




0
2
0
2
0
2
0
2>>

 banda ;




2
2
2
2
2
2
2
2<<

 banda
Comparable
Table 2. Relationship between

and


as provided in case: II.
Restriction on


, Some Other Considerations Nature of the Pair

 and







   and >


2
2
2
2>


a Incomparable




 << and


0
2
0
2<


a and


0
2
0
2<


b


2
2
2
2>

 b
Incomparable




 =< and




2
2
2
2
0
2
0
2>>

 aanda Incomparable




 == and




2
2
2
2
2
2
2
2>>
 banda Incomparable




 >= and




2
2
2
2
2
2
2
2>>
 banda  Incomparable




 >< and


0
2
0
2>


a;


0
2
0
2>


b
and




2
2
2
2
2
2
2
2>;>

ba
or


0
2
0
2<


a;


0
2
0
2<


b
and




2
2
2
2
2
2
2
2<;<

ba
Incomparable




== and




2
2
2
2
2
2
2
2<<
 banda Comparable




>= and




2
2
2
2
2
2
2
2<<
 banda  Comparable




 >< and


0
2
0
2>


a;


0
2
0
2>


b
and




2
2
2
2
2
2
2
2<;<

ba
or


0
2
0
2<


a;


0
2
0
2<


b
and




2
2
2
2
2
2
2
2>;>

 ba
Comparable




 << and


0
2
0
2>


a and


0
2
0
2>


b


2
2
2
2>


b
Comparable




 <=and


0
2
0
2>


b and


2
2
2
2<

 b Comparable

A. BHAR
4
Table 3. Relationship betwee n

and



as provided in case: III.
Restriction on


, Some Other Considerations Nature of the Pair

and









and Incomparable




 << and


0
2
0
2<

 a and


2
2
2
2<


a Incomparable




=< and








2
2
2
2
0
2
0
2<><>

 oraandora Incomparable




 <= and


0
2
0
2>ba

;


0
2
0
2>
 b
and




2
2
2
2
2
2
2
2>;>
 bba 
or


0
2
0
2<ba

;


0
2
0
2<
 b
and




2
2
2
2
2
2
2
2<;<
 bba 
Incomparable




<= and


0
2
0
2>ba

;


0
2
0
2>
 b
and




2
2
2
2
2
2
2
2<;<
 bba 
or


0
2
0
2<ba

;


0
2
0
2<
 b
and




2222
2222
>;>abb

 
Comparable




 =< and








2
2
2
2
0
2
0
2<>><

 oraandora Comparable
Table 4. Relationship betwee n

and



as provided in case: IV.
Restriction on


, Some Other Considerations Nature of The Pair

and









and >


2
2
2
2>


a Incomparable







and
<


0
2
0
2<


a Incomparable




 <=and


0
2
0
2>ba

;


0
2
0
2>
 b
and




2
2
2
2
2
2
2
2>;>
 bba
or




0
2
2
2
2
2
2
2>;>
 bba
and


0
2
0
2<ba

;


2
2
0
2<
 b
Incomparable




 <=and


2
2
2
2
2
2
2
2>>
 bandba  Incomparable




>= and


2
2
2
2
2
2
2
2>>
 bandba  Comparable




<= and


0
2
0
2>ba

;


0
2
0
2>
 b
and




2
2
2
2
2
2
2
2<;<
 bba
or


0
2
0
2<ba

;


0
2
0
2<
 b
and




2
2
2
2
2
2
2
2>;>
 bba 
Comparable




== and Comparable




=> and


2
2
2
2<


a Comparable






and
<


0
2
0
2>


a Comparable

A. BHAR
5
Table 5. Relationship between

and


as provided in case: V.
Restriction on


, Some Other Considerations Nature of the Pair 
and







 >> and


2
2
2
2>


a and


2
2
2
2>


b Incomparable




 << and


0
2
0
2<


a and


0
2
0
2<


b Incomparable




 == and Comparable




 >> and


2
2
2
2<


a and


2
2
2
2<


b Comparable




 >= and


2
2
2
2<
  b Comparable




 => and


2
2
2
2<


a Comparable




 << and


0
2
0
2>
  a and


0
2
0
2>
  b Comparable




 <= and


0
2
0
2>


b Comparable




 =< and


0
2
0
2>

 a Comparable
fundamental question is weather this incomparability
under LOCC of states becomes the global phenomenon
of  and 
 i.e. clearly to say that wheather

and 
 are incomparable under LOCC to each other
or not and we illustrate all the results through the
following tabular form:
CASE: IV
For two states  and   of two parties A and B
with the restriction that the states

ψ,ψ

are
comparable to each other under LOCC and our
investigation of the status of the pair  and



leads some interesting consequences that have been
provided through the following tabular form:
CASE: V
Here the two states  and 
 of two parties A and
B with the basic considerations that the states ψ,ψ

and

and

 are comparable to each other under
LOCC. So the fundamental question is wheather the
notion of incomparability under LOCC of states can play
the crucial role to change the status of  and


.
Through observations some peculiar facts have been
revealed and we illustrate all the results through the
following tabular form:
4. Conclusion
Through the long path of observation and investigation
the most interesting physical fact that superposition of
many states which forms a bridge between the two classes
of states i.e. comparable and incomparable has been
vividly revealed and some simple algebra and intuitive
ideas become helpful to come to arrive the serious
conclusion that the extension of dimension of


ψ,ψ

and


,



will give exactly the same results if we
are thinking of strong incomparability instead of general
sense of incomparability of states.The result, that
constructing of locally comparable states through the
superposition of locally incomparable states may be
helpful for revealing some new physics of detection of
entanglement. The concept, that converting incom-
parability of states to comparability under LOCC,
directly may considered as an information processing
task and detecting entanglement of the states which are
used for constructing a new state.
5. References
[1] A. Einstein, B. Podolsky and N. Rosen, “Can a Quantum
-Mechanical Description of Physical Reality be
Considered Completely?,” Physical Review A, Vol. 47,
1935, pp. 777-780. doi:10.1103/PhysRev.47.777
[2] E. Schrödinger, “Die Gegenwartige Situation in Der
Qunteunme Chnik,”
N
aturwissenschaften
 , Vol. 23, 1935,
p. 807.
[3] J. S. Bell, “On the Einstein Podolsky Rosen Paradox,”
Physics, Vol. 1, No. 3, 1964, pp. 195-200.
[4] W. H. Zurek, “Quantum Cloning: Schrodinger’s Sheep,”
Nature, Vol. 404, 2000, pp. 130-131.
doi:10.1038 /3 500 468 4
[5] M. A. Nielsen, “Condition for a Class of Entanglement
Transformations,” Physical Review Letter, Vol. 83, No. 2,
1999, pp. 436-139. doi:10.1103/PhysRevLett.83.436
[6] D. Bouwmeester, A. Ekert and A. Zeilinger, “The Physics

A. BHAR
6
of Quantum Teleportation and Quantum Computation,”
Springer, New York, 2000.
[7] M. Horodecki, R. Horodecki, A. S. De and U. Sen, “No
Deleting and No Cloning Principles as Consequences of
Conservation of Quantum Information,” Quantum Phys-
ics, 2003. http://arxiv.org/abs/quant-ph/0306044v1
[8] S. J. Van Enk, “Relation between Cloning and the
Universal not Derived Form Conservation Laws,”
Physical Review Letter, Vol. 95, No. 1, 2005, Article ID
010502. doi:10.1103/PhysRevLett.95.010502
[9] D. P. DiVincenzo, A. Ekert, C. A. Fuchs, et al., “Optimal
Universal and State Dependent Quantum Cloning,”
Physical Review A, Vol. 57, No. 4, 1998, pp. 2368-2378.
doi:10.1103/PhysRevA.57.2368
[10] D. Dieks, “Communication by EPR Devices,” Physics
Letter A, Vol. 92, No. 6, 1982, pp. 271-272.
doi:10.1016/0375-9601(82)90084-6
[11] A. K. Pati and S. L. Braunstein, “Quantum Deleting and
Signalling,” Physics Letter A, Vol. 315, No. 3-4, 2003, pp.
208-212. doi:10.1016/S0375-9601(03)01047-8
[12] A. K. Pati, “Probabilistic Exact Cloning and Probabilistic
No-signalling,” Physics Letter A, Vol. 270, 2000, pp.
103-1407. doi:10.1016/S0375-9601(00)00281-4
[13] N. Linden, S. Popescu and J. Smolin, “Entanglement of
Superposition,” Physical Review Letter, Vol. 97, No. 10,
2000, Article ID 100502.
[14] H. P. Yuen, “Amplification of Quantum States and
Noiseless Photon Amplifiers,” Physics Letter A, Vol. 113,
No. 8, 1986, pp. 405-407.
doi:10.1016/0375-9601(86)90660-2
[15] N. Gisin and S. Popescu, “Spin Flips and Quantum
Information Foe Antiparallel Spins,” Physcal Review
Letter, Vol. 83, No. 2, 1999, pp. 432-435.
doi:10.1103/PhysRevLett.83.432
[16] I. Chattopadhyay, S. K. Choudhary, G. Kar, et al., “No
Fliping as a Consequence of No-signalling and
Nonincresement of Entanglement under LOCC,” Physics
Letters A, Vol. 351, No. 6, 2005, pp. 384-387.
[17] I. Chattopadhyay and D. Sarkar, “Entanglement of For-
mation in Non-monotonic with Concurrence: A Simple
Proof,” Quantum Information and Computation, Vol. 5,
No. 3, 2005, pp. 247-257.
[18] I. Chattopadhyay and D. Sarkar, “Impossible of Exact
Ipping of Three Arbitrary Quantum States via
Incomparability,” Physical Review A, Vol. 73, No. 4,
2006, Article ID 044303.
[19] M. A. Nielsen and I. L. Chuang, “Quantum Computation
and Quantum Information,” Cambridge University Press,
Cambridge, 2000.