Paper Menu >>
Journal Menu >>
 Chinese Studies 2013. Vol.2, No.4, 197-203 Published Online November 2013 in SciRes (http://www.scirp.org/journal/chnstd) http://dx.doi.org/10.4236/chnstd.2013.24032 Open Access 197 Yintelligence™: The Mapping of the Pre-Heaven or FuXi Hexagrams to the Post-Heaven or King Wen Hexagrams Gabriel Felley University of Applied Sciences and Arts (UNW), Institute for Information Sys tems, Olten, Switzerland Email: gabriel.felley@fhnw.ch Received October 6th, 2013; revised November 11th, 2013; accepted November 20th, 2013 Copyright © 2013 Gabriel Felley. This is an open access article distributed under the Creative Commons Attri- bution License, which permits unrestricted use, distribution, and reproduction in any medium, provided the original work is properly cited. The Yijing 易經 belongs to the famous group of the five classics. In the Ming and Qing dynasties, the Four Books and Five Classics四書五經 were the subject of mandatory study by those Confucian scholars who wished to become government officials. The Yijing (Faure & Javary, 2002) consists of 64 figures called hexagrams 卦 formed by six Yin (broken) or Yang (unbroken) lines and commentaries on each of these hexagrams and their lines. The whole set of the hexagrams and their interdependencies make a dynamic model for the understanding or the anticipating of the different steps change goes through. The Yijing does not predict the future but gives a snap-shot of the actual situation and the holistic potentialities which may be deployed or not in order to better adapt to the on-going change. The different stages of a change are explained by the Yin-Yang polarity and the never-ending transformation of old-Yin 老陰 in young-Yang 少陽, old-Yang老陽, young-Yin少陰 and old-Yin again. The 64 hexagrams are built by pairing the 8 basic trigrams obtained by adding to each bigram a supplementary Yin or Yang line. Figure 1 depicts a 4-regions space (East/young Yang, South /old Yang, West/young Yin, North/old Yin) and a cyclic movement. A forthcoming article will explain the relation between this space organization and the whole set of 64 hexagrams as a network. The cyclic change is of major interest. Purpose: The 64 hexa- grams of the Yijing are organized in 16 “first degree” nuclear families (Javary, 1997), which may be con- solidated in 4 “second degree” nuclear families. There are another 16 families organizing the 64 hexa- grams in a different structure. In the literature they are called the pre-heaven先天 hexagrams (Schlum- berger, 1987), and in th is paper they are referred to as the FuXi伏羲 hexagrams. They form 16 cyclic fami- lies, whose structure will be analyzed in this paper. This cyclicity induces a “predecessor-successor” rela- tionship between the hexagrams belonging to the same FuXi family: H1H2H3H4H1. While the 4 members of each nuclear family are centered on a “master” hexagram that corresponds to a common inner lines’ structure, each FuXi family is cyclically structured and we call the hexagrams belonging to the same family FuXi related. Each Wen hexagram corresponds exactly to a FuXi hexagram. This corre- spondence reflects the respective trigrams’ arrangements of King Wen and FuXi. The trigrams of the FuXi hexagram and those of the corresponding Wen hexagram occupy the same locations in the FuXi and the King Wen arrangements displayed in the Figure 2. This paper explains the relation between the Wen hexagrams and their corresponding FuXi hexagrams based on structural and semantic properties. Sum- mary: It has been demonstrated, that the pre-heaven and post-heaven hexagrams are pairwise related through specific relations defined by the opposition of the post-heaven hexagrams and 2 members of par- ticular nuclear families which have opposite envelopes. Basically, the links between pre-heaven or FuXi hexagrams and post-heaven or Wen hexagrams are based on the hexagrams’ structure, especially on the opposition of 2 hexagrams. Two FuXi related hexagrams are connected to each other by a “predeces- sor-successor” like relation, the pre-heaven or FuXi hexagram somehow gives the necessary prerequisite which the post-heaven hexagram must own. In a pair of opposite hexagrams, the one possesses the com- plementary dimensions which are absent in the other. All these links may be represented using the tri- grams deployed adequately on two circles, one with the pre-heaven trigrams, the FuXi arrangement, and the second with the post-heaven trigram or King Wen arrangement. Two corresponding trigrams are iden- tically positioned in the two circular arrangements. The logical and sophisticated system applied to link the Wen to the FuXi hexagrams again reveals how subtly the whole system of 64 hexagrams has been conceptualized and evidences a new and original insight into the structure of the Yijing. Keywords: Yijing; I Ching; Yintelligence; Book of Changes; Hexagram; King Wen; Fu Xi Introduction The Two Trigram’s Arrangements form FuXi and King Wen There are traditionally two basic organizations of the 8 tri- grams, the FuXi 伏羲 and King Wen 文王 arrangements (Figure 2). Each of these illustrates a specific dynamic and the interdependencies between the trigrams. The implicate arrangement shows a perfect symmetry of the trigrams, which are diametrically opposed. Considering the 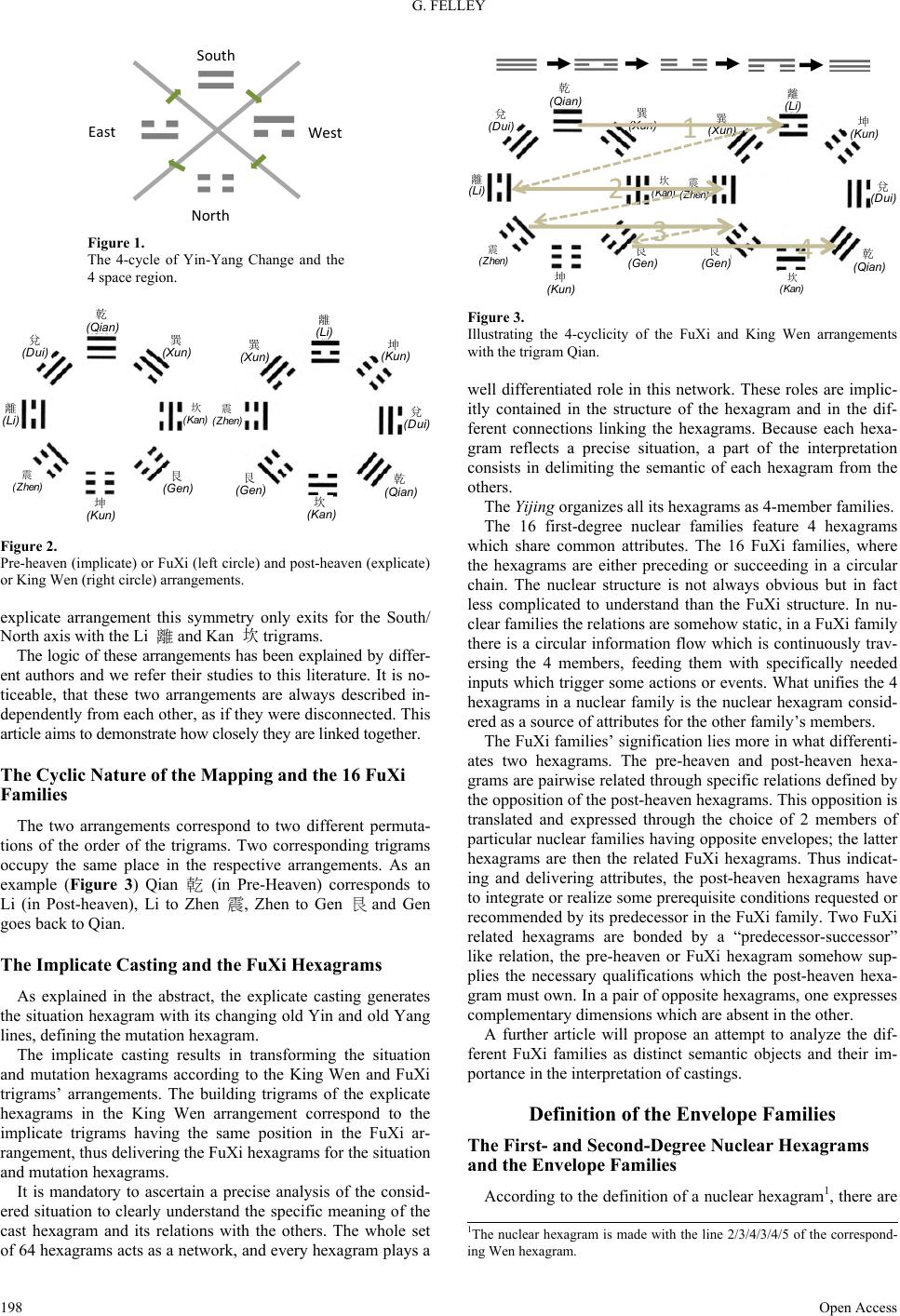 G. FELLEY South North West East Figure 1. The 4-cycle of Yin-Yang Change and the 4 space region. 乾 (Qian) 兌 (Dui) 離 (Li) 震 (Z hen) 坤 (Kun) 艮 (Gen) 坎 (Kan) 巽 (Xun) 巽 (Xun) 坤 (Kun) 兌 (Dui) 乾 (Qian) 坎 (Kan) 艮 (Gen) 震 (Zhen) 離 (Li) Figure 2. Pre-heaven (implicate) or FuXi (left circle) and post-heaven (explicate) or King Wen (right circle) arrangements. explicate arrangement this symmetry only exits for the South/ North axis with the Li 離and Kan 坎trigrams. The logic of these arrangement s has been explained by differ- ent authors and we refer their studies to this literature. It is no- ticeable, that these two arrangements are always described in- dependently from each other, as if they were disconnected. This article aims to demonstrate how closely they are linked together. The Cyclic Nature of the Mapping and the 16 FuXi Families The two arrangements correspond to two different permuta- tions of the order of the trigrams. Two corresponding trigrams occupy the same place in the respective arrangements. As an example (Figure 3) Qian 乾 (in Pre-Heaven) corresponds to Li (in Post-heaven), Li to Zhen 震, Zhen to Gen 艮and Gen goes back to Qian. The Implicate Casting and the FuXi Hexagrams As explained in the abstract, the explicate casting generates the situation hexagram with its changing old Yin and old Yang lines, defining the mutation hexagram. The implicate casting results in transforming the situation and mutation hexagrams according to the King Wen and FuXi trigrams’ arrangements. The building trigrams of the explicate hexagrams in the King Wen arrangement correspond to the implicate trigrams having the same position in the FuXi ar- rangement, thus delivering the FuXi hexagrams for the situation and mutation hexagrams. It is mandatory to ascertain a precise analysis of the consid- ered situation to clearly understand the specific meaning of the cast hexagram and its relations with the others. The whole set of 64 hexagrams acts as a network, and every hexagram plays a 2 34 1 乾 (Qian) 兌 (Dui) 艮 (Gen) 離 (Li) 坤 (Kun) 坎 (Kan) 巽 (Xun) 震 (Zhen) 巽 (Xun) 離 (Li) 坤 (Kun) 兌 (Dui) 乾 (Qian) 坎 (Kan) 艮 (Gen) 震 (Zhen) Figure 3. Illustrating the 4-cyclicity of the FuXi and King Wen arrangements with the trigram Qian. well differentiated role in this network. These roles are implic- itly contained in the structure of the hexagram and in the dif- ferent connections linking the hexagrams. Because each hexa- gram reflects a precise situation, a part of the interpretation consists in delimiting the semantic of each hexagram from the others. The Yijing organizes all its hexagrams as 4-member families. The 16 first-degree nuclear families feature 4 hexagrams which share common attributes. The 16 FuXi families, where the hexagrams are either preceding or succeeding in a circular chain. The nuclear structure is not always obvious but in fact less complicated to understand than the FuXi structure. In nu- clear families the relations are somehow static, in a FuXi family there is a circular information flow which is continuously trav- ersing the 4 members, feeding them with specifically needed inputs which trigger some actions or events. What unifies the 4 hexagrams in a nuclear family is the nuclear hexagram consid- ered as a source of attributes for the other family’s members. The FuXi familie s’ signification lies more in what differenti- ates two hexagrams. The pre-heaven and post-heaven hexa- grams are pairwise related through specific relations defined by the opposition of the post-heaven hexagrams. This opposition is translated and expressed through the choice of 2 members of particular nuclear families having opposite envelopes; the latter hexagrams are then the related FuXi hexagrams. Thus indicat- ing and delivering attributes, the post-heaven hexagrams have to integrate or realize some prerequisite conditions requested or recommended by its predecessor in the FuXi fa mily. Two FuXi related hexagrams are bonded by a “predecessor-successor” like relation, the pre-heaven or FuXi hexagram somehow sup- plies the necessary qualifications which the post-heaven hexa- gram must own. In a pair of opposite hexagrams, one expresses complementary dimensions which are absent in the other. A further article will propose an attempt to analyze the dif- ferent FuXi families as distinct semantic objects and their im- portance in the interpretation of castings. Definition of the Envelope Families The First- and Second-Degree Nuclear Hexagrams and the Envelope Families According to the definition of a nuclear hexagram1, there are 1The nuclear hexagram is made with the line 2/3/4/3/4/5 of the correspond- ing Wen hexagram. Open Access 198 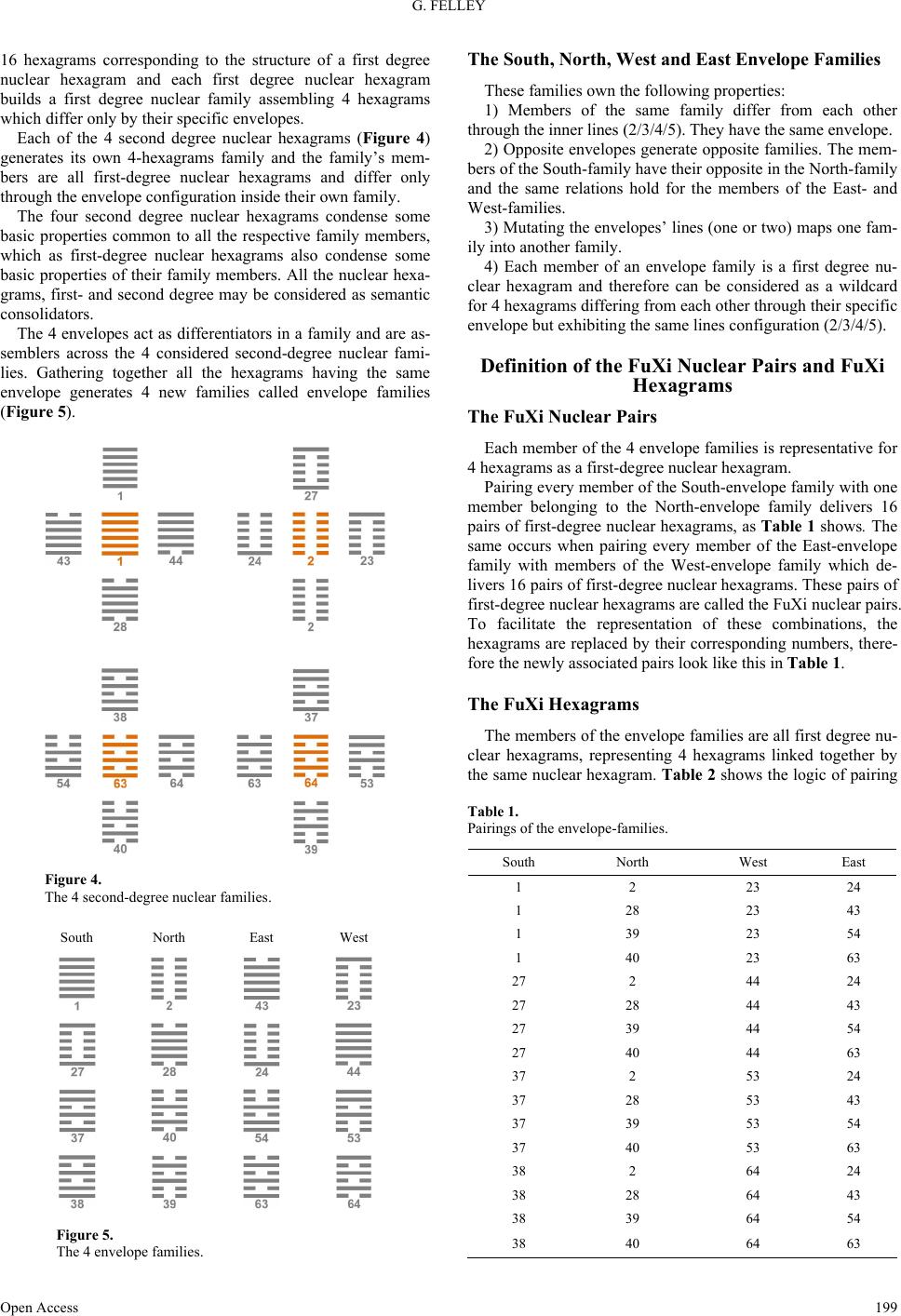 G. FELLEY 16 hexagrams corresponding to the structure of a first degree nuclear hexagram and each first degree nuclear hexagram builds a first degree nuclear family assembling 4 hexagrams which differ only by their specific envelopes. Each of the 4 second degree nuclear hexagrams (Figure 4) generates its own 4-hexagrams family and the family’s mem- bers are all first-degree nuclear hexagrams and differ only through the envelope configurati on inside their own family. The four second degree nuclear hexagrams condense some basic properties common to all the respective family members, which as first-degree nuclear hexagrams also condense some basic properties of their family members. All the nuclear hexa- grams, first- and second degree may be considered as semantic consolidators. The 4 envelopes act as differentiators in a family and are as- semblers across the 4 considered second-degree nuclear fami- lies. Gathering together all the hexagrams having the same envelope generates 4 new families called envelope families (Figure 5). Figure 4. The 4 second-degree nuclear families. South North East West Figure 5. The 4 envelope families. The South, North, West and East Envelope Families These families own the following properties: 1) Members of the same family differ from each other through the inner lines (2/3/4/5). They have the same envelope. 2) Opposite envelopes generate opposite families. The mem- bers of the South-family have their opposite in the North-family and the same relations hold for the members of the East- and West-families. 3) Mutating the envelopes’ lines (one or two) maps one fam- ily into another family. 4) Each member of an envelope family is a first degree nu- clear hexagram and therefore can be considered as a wildcard for 4 hexagrams differing from each other through their specific envelope but exhibiting the same lines configuration (2/3/4/5). Definition of the FuXi Nuclear Pairs and FuXi Hexagrams The FuXi Nuclear Pairs Each member of the 4 envelope families is representative for 4 hexagrams as a first-degree nuclear hexagram. Pairing every member of the South-envelope family with one member belonging to the North-envelope family delivers 16 pairs of first-degree nuclear hexagrams, as Table 1 shows. The same occurs when pairing every member of the East-envelope family with members of the West-envelope family which de- livers 16 pairs of first-degree nuclear hexagrams. These pairs of first-degree nuclear hexagrams are called the FuXi nuclear pairs. To facilitate the representation of these combinations, the hexagrams are replaced by their corresponding numbers, there- fore the newly associated pairs look like this in Table 1. The FuXi Hexagrams The members of the envelope families are all first degree nu- clear hexagrams, representing 4 hexagrams linked together by the same nuclear hexagram. Table 2 shows the logic of pairing Table 1. Pairings of the envelope-families. South North West East 1 2 23 24 1 28 23 43 1 39 23 54 1 40 23 63 27 2 44 24 27 28 44 43 27 39 44 54 27 40 44 63 37 2 53 24 37 28 53 43 37 39 53 54 37 40 53 63 38 2 64 24 38 28 64 43 38 39 64 54 38 40 64 63 Open Access 199 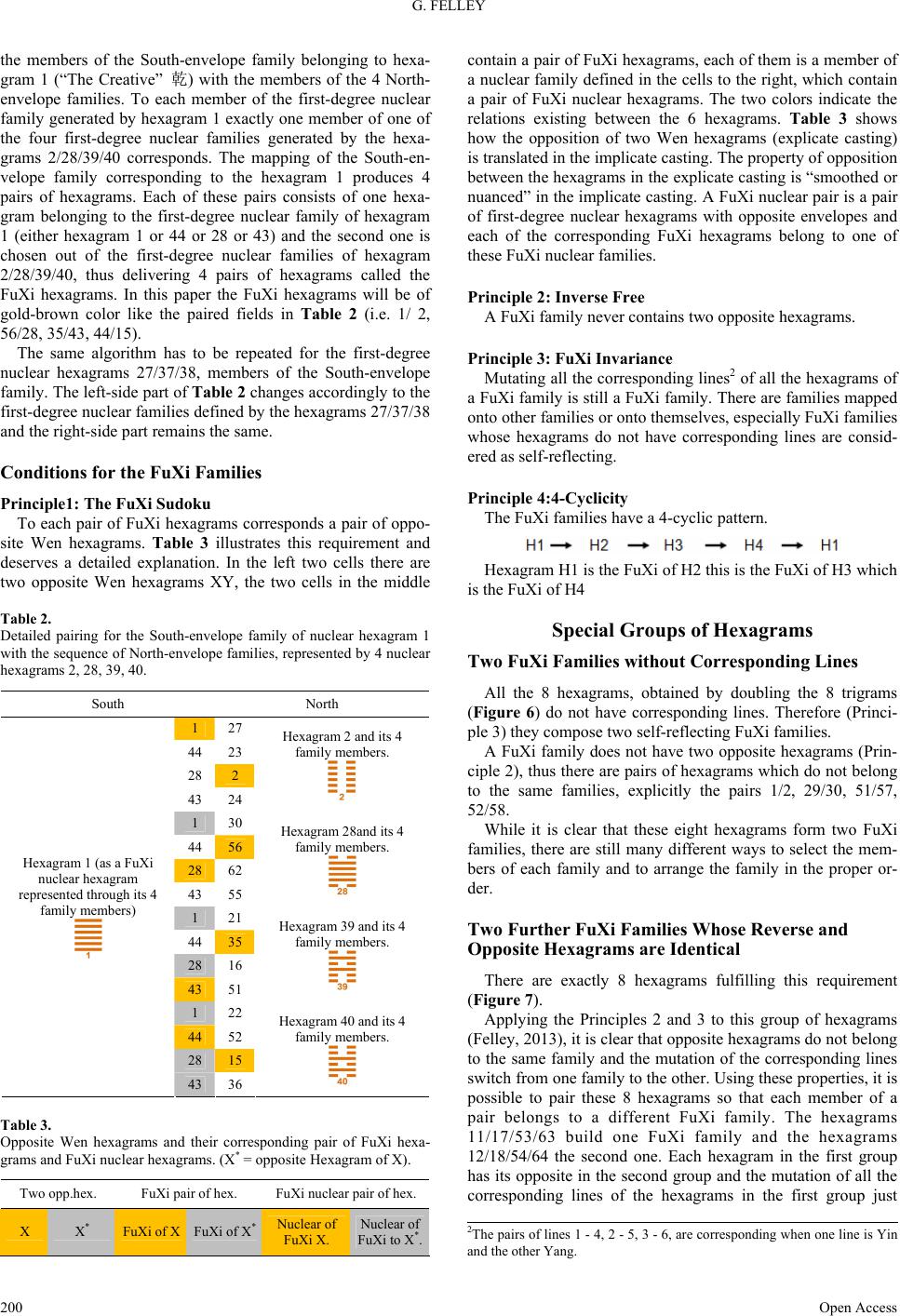 G. FELLEY the members of the South-envelope family belonging to hexa- gram 1 (“The Creative” 乾) with the members of the 4 North- envelope families. To each member of the first-degree nuclear family generate d by hexagram 1 exactly one member of one of the four first-degree nuclear families generated by the hexa- grams 2/28/39/40 corresponds. The mapping of the South-en- velope family corresponding to the hexagram 1 produces 4 pairs of hexagrams. Each of these pairs consists of one hexa- gram belonging to the first-degree nuclear family of hexagram 1 (either hexagram 1 or 44 or 28 or 43) and the second one is chosen out of the first-degree nuclear families of hexagram 2/28/39/40, thus delivering 4 pairs of hexagrams called the FuXi hexagrams. In this paper the FuXi hexagrams will be of gold-brown color like the paired fields in Table 2 (i.e. 1/ 2, 56/28, 35/43, 44/15). The same algorithm has to be repeated for the first-degree nuclear hexagrams 27/37/38, members of the South-envelope family. Th e left-side part of Table 2 changes accordingly to the first-degree nuclear families defined by the hexagrams 27/37/38 and the right-side part remains the same. Conditions for the FuXi Families Principle1: The F uX i Sudoku To each pair of FuXi hexagrams corresponds a pair of oppo- site Wen hexagrams. Table 3 illustrates this requirement and deserves a detailed explanation. In the left two cells there are two opposite Wen hexagrams XY, the two cells in the middle Table 2. Detailed pairing for the South-envelope family of nuclear hexagram 1 with the sequence of North-envelope families, represented by 4 nuclear hexagrams 2, 28, 39, 40. South North 1 27 44 23 28 2 43 24 Hexagram 2 and its 4 family members. 1 30 44 56 28 62 43 55 Hexagram 28and its 4 family members. 1 21 44 35 28 16 43 51 Hexagram 39 and its 4 family members. 1 22 44 52 28 15 Hexagram 1 (as a FuXi nuclear hexagram represented t hrough its 4 family members) 43 36 Hexagram 40 and its 4 family members. Table 3. Opposite Wen hexagrams and their corresponding pair of FuXi hexa- grams and FuXi nuclear he x ag rams. (X* = opposite Hexagram of X). Two opp.hex. FuXi pair of hex. FuXi nuclear pair of hex. X X* FuXi of X FuXi of X* Nuclear of FuXi X. Nuclear of FuXi to X*. contain a pair of FuXi hexagrams, each of them is a member of a nuclear family defined in the cells to the right, which contain a pair of FuXi nuclear hexagrams. The two colors indicate the relations existing between the 6 hexagrams. Table 3 shows how the opposition of two Wen hexagrams (explicate casting) is translated in the implicate casting. The property of opposition between the hexagrams in the explicate casting is “smoothed or nuanced” in the implicate casting. A FuXi nuclear pair is a pair of first-degree nuclear hexagrams with opposite envelopes and each of the corresponding FuXi hexagrams belong to one of these FuXi nuclear families. Principle 2: Inverse Free A FuXi family never contains two opposite hexagrams. Principle 3: FuXi Invariance Mutating all the corresponding lines2 of all the hexagrams of a FuXi family is still a FuXi family. There are families mapped onto other families or onto themselves, especially FuXi families whose hexagrams do not have corresponding lines are consid- ered as self-reflecting. Principle 4:4-Cyclicity The FuXi families have a 4-cyclic pattern. Hexagram H1 is the FuXi of H2 this is the FuXi of H3 which is the FuXi of H4 Special Groups of Hexagrams Two FuXi Families without Corresponding Lines All the 8 hexagrams, obtained by doubling the 8 trigrams (Figure 6) do not have corresponding lines. Therefore (Princi- ple 3) they compose two self-reflecting FuXi families. A FuXi family does not have two opposite hexagrams (Prin- ciple 2), thus there are pairs of hexagrams which do not belong to the same families, explicitly the pairs 1/2, 29/30, 51/57, 52/58. While it is clear that these eight hexagrams form two FuXi families, there are still many different ways to select the mem- bers of each family and to arrange the family in the proper or- der. Two Further FuXi Families Whose Reverse a nd Opposite Hexagrams are Identical There are exactly 8 hexagrams fulfilling this requirement (Figure 7). Applying the Principles 2 and 3 to this group of hexagrams (Felley, 2013), it is clear that opposite hexagrams do not belong to the same family and the mutation of the corresponding lines switch from one family to the other. Using these properties, it is possible to pair these 8 hexagrams so that each member of a pair belongs to a different FuXi family. The hexagrams 11/17/53/63 build one FuXi family and the hexagrams 12/18/54/64 the second one. Each hexagram in the first group has its opposite in the second group and the mutation of all the corresponding lines of the hexagrams in the first group just 2The pairs of lines 1 - 4, 2 - 5, 3 - 6, are corresponding when one line is Yin and the other Yang. Open Access 200 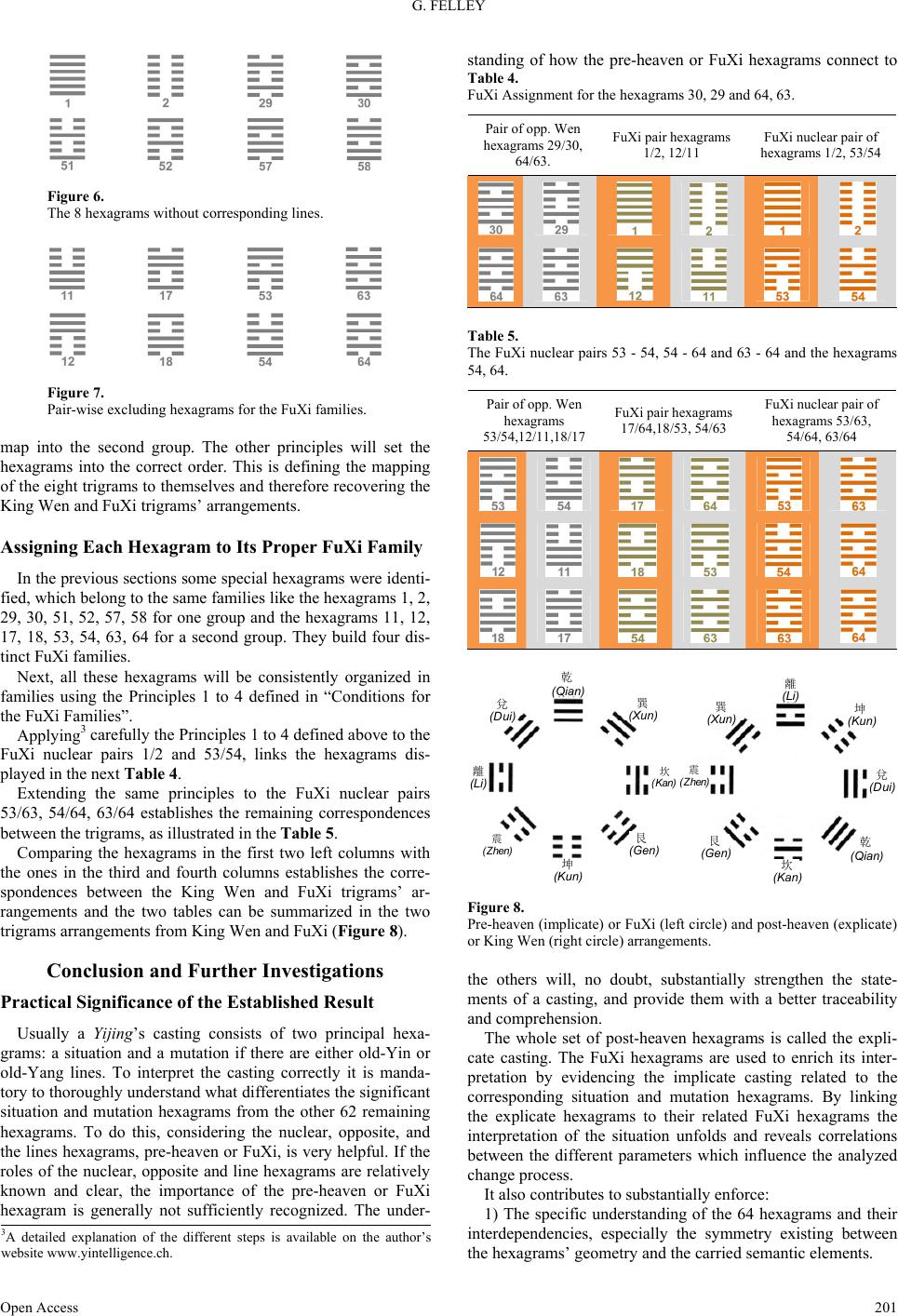 G. FELLEY Figure 6. The 8 hexagrams without corresponding lines. Figure 7. Pair-wise excluding hexagr ams for the FuXi families. map into the second group. The other principles will set the hexagrams into the correct order. This is defining the mapping of the eight trigrams to themselves and therefore recovering the King Wen and FuXi trigrams’ arrangements. Assigning Each Hexagram to Its Proper FuXi Family In the previous sections some special hexagrams were identi- fied, which belong to the same families like the hexagrams 1, 2, 29, 30, 51, 52, 57, 58 for one group and the hexagrams 11, 12, 17, 18, 53, 54, 63, 64 for a second group. They build four dis- tinct FuXi fami lies. Next, all these hexagrams will be consistently organized in families using the Principles 1 to 4 defined in “Conditions for the FuXi Fami lies”. Applying3 carefully the Principles 1 to 4 defined above to the FuXi nuclear pairs 1/2 and 53/54, links the hexagrams dis- played in the next Table 4. Extending the same principles to the FuXi nuclear pairs 53/63, 54/64, 63/64 establishes the remaining correspondences between the trigrams, as illustrated in the Table 5. Comparing the hexagrams in the first two left columns with the ones in the third and fourth columns establishes the corre- spondences between the King Wen and FuXi trigrams’ ar- rangements and the two tables can be summarized in the two trigrams arrangements from King Wen and FuXi (Figure 8). Conclusion and Further Investigations Practical Significance of the Established Result Usually a Yijing’s casting consists of two principal hexa- grams: a situation and a mutation if there are either old-Yin or old-Yang lines. To interpret the casting correctly it is manda- tory to thoroughly understand what differentiates the significant situation and mutation hexagrams from the other 62 remaining hexagrams. To do this, considering the nuclear, opposite, and the lines hexagrams, pre-heaven or FuXi, is very helpful. If the roles of the nuclear, opposite and line hexagrams are relatively known and clear, the importance of the pre-heaven or FuXi hexagram is generally not sufficiently recognized. The under- standing of how the pre-heaven or FuXi hexagrams connect to Table 4. FuXi Assignment for the hexagrams 30, 29 and 64, 63. Pair of opp. Wen hexagrams 29/30, 64/63. FuXi pair hexagrams 1/2, 12/11 FuXi nuclear pair of hexagrams 1/2, 53/54 Table 5. The FuXi nuclear pairs 53 - 54, 54 - 64 and 63 - 64 and the hexagrams 54, 64. Pair of opp. Wen hexagrams 53/54,12/11,18/17 FuXi pair hexagrams 17/64,18/ 53, 54/63 FuXi nuclear pair of hexagrams 53/63, 54/64, 63/ 64 乾 (Qian) 兌 (Dui) 離 (Li) 震 (Z hen) 坤 (Kun) 艮 (Gen) 坎 (Kan) 巽 (Xun) 巽 (Xun) 坤 (Kun) 兌 (Dui) 乾 (Qian) 坎 (Kan) 艮 (Gen) 震 (Z hen) 離 (Li) Figure 8. Pre-heaven (implicate) or FuXi (left circle) and post-heaven (explicate) or King Wen (right circle) arrangements. the others will, no doubt, substantially strengthen the state- ments of a casting, and provide them with a better traceability and comprehension. The whole set of post-heaven hexagrams is called the expli- cate casting. The FuXi hexagrams are used to enrich its inter- pretation by evidencing the implicate casting related to the corresponding situation and mutation hexagrams. By linking the explicate hexagrams to their related FuXi hexagrams the interpretation of the situation unfolds and reveals correlations between the different parameters which influence the analyzed change process. It also contributes to substantially enforce: 1) The specific understanding of the 64 hexagrams and their interdependencies, especially the symmetry existing between the hexagrams’ geometry and the carried semantic elements. 3A detailed explanation of the different steps is available on the author’s website www.yintelligence.ch. Open Access 201  G. FELLEY 2) The integration of the FuXi hexagrams in a casting’s in- terpretation considerably helps to demonstrate the relevance and consistency of the casting by revealing hidden connections between the selected hexagrams. 3) The perception of the Yijing internal logic as a 4-valued logical system and therefore the support of a thin granular con- ceptual framework for decision making. 4) The assumption that the trigrams play a significant role in organizing the hexagrams especially to describe the mapping of the pre-heaven to the post-heaven hexagrams. The Four-Valued Logic under Pinning the Yijing Among the 64 hexagrams there are some couples with a strong polarity, for example hexagrams 1, 2, 11, 12, 31, 32, 41, 42, 63, 64 (Figure 9). These sequences may be considered as a line of growing com- plexity in the hexagrams’ specific logic. Starting with hexagra ms 1 and 2, equipped with a uniform, erratic Yang and Yin logic, this sequence goes through different states to end with hexa- grams 63 and 64 both displaying a sophisticated intricacy. The process is launched with the re-arrangement of the tri- grams Qian and Kun坤, creating six corresponding lines, which generate the two-cyclic dynamic of hexagrams 11/12 (Figure 10). This dynamic culminates in the delicate interplay of the hexagrams 63 and 64, the last closes the Book of Changes ig- niting a new organization. This entanglement of the hexagrams is deployed by means of mutating the corresponding lines of the pair of hexagrams 11/12. Mutating the lines 1 and 4 produces hexagrams 32/42, mutating the lines 3 and 6 produces hexagrams 41/31 and mu- tating the inner lines 2 and 5 results in the last two hexagrams 63 and 64. While the hexagrams 31, 32, 41, 42 are related to each other being either reverse or opposite and the hexagrams 11 and 12 are reverse and opposite4, the hexagrams 63/64 are the only two consolidating all these attributes with the fact that they are the reciprocal first-degree nuclear hexagrams and iden- tical to their second-degree nuclear hexagrams, the last property being also shared by the hexagrams 1 and 2. Thus the four hexagrams 1, 2, 63, 64 play an outstanding role in the Yijing’s inherent logical structure. This is based on a four-valued logic defined by the very deep characteristics of each of these four special hexagrams. These characteristics will be analyzed more in detail in a forthcoming article at a later date. Figure 9. A special sequence of pairs of hexagrams. Hex 11 Hex 12 Hex 31 Hex 32 Hex 41 Hex 42 H ex 63 Hex 64 Figure 10. Cyclic dynamic of hexagrams 11/12, 31/32, 41/42, 63/ 64. Principle of Inheritance or Seeding Within a given FuXi family, the cyclic chain of the four hexagrams defines a predecessor and a successor for each hexa- gram, thus creating a kind of “inheritance” of hexagram spe- cific attributes. The impact of these influences deserves more attention and will be analyzed in a forthcoming article. As an example, hexagrams 3 and 50 are considered in more detail (Table 6). These two hexagrams have a special meaning, because they also divide the Book of Changes in two parts. All the hexagrams between these two also have their opposite be- tween them and consequently all hexagrams between the hexa- grams 51 and 64 have their opposite in that range5. The opposition of the Wen hexagrams 3 and 50 corresponds to the opposition of the FuXi nuclear hexagrams 40 and 37. Noticeable is the fact that the two FuXi hexagrams 36 and 10 are almost opposite each other. Only the line 1 does not fulfill the right conditions. The hexagrams 3 and 50 both describe situations of qualita- tively different renewals, the hexagrams 36 and 10 explain qualitatively different behavioral attitudes rooted in the oppo- site nuclear hexagrams 40 and 37, most suitable to achieve the respective goals of the hexagrams 3 and 50. To investigate all these interdependencies between the hexa- grams belonging to the same FuXi family and their assigned FuXi nuclear pairs will be the central theme of forthcoming publications. This example aims to demonstrate the possible benefits derived from this consideration of the generation proc- ess for the FuXi hexagrams. The FuXi Hexagrams as Marks for the Book of Changes It is usual to consider the hexagrams 1 to 30 as the first part of the Book of Changes and the remaining hexagrams 31 to 64 as its second part. Each part contains 18 different hexagram figures, some of them can be reversed; some cannot. The hexagrams 1 and 2 are respectively the FuXi hexagrams of the hexagrams “Fire” 離 and “Abysmal” 坎6, and they close the first part of the Book of changes. Further, as illustrated by Table 7, hexagram 3, “The diffi- culty of the Beginning” 屯, is the FuXi of hexagram 31, “Influ- ence” 咸, the hexagram initiating the second part of the Book of Changes. Table 6. Hexagrams 3 and 50 with their FuXi hexagrams. Pair of opp. Wen hexagrams 3/50. FuXi pair he xagrams 36/10 FuXi nuclear pair of hexagrams 40/37 5This fact explains the positions of the hexagrams, “Difficulty in the Begin- ning” and “The Caldron”, in the Book of Change. Hexagram “Difficulty in the Begin ning ” has to be pl aced in the right begin ning af ter hex agrams “ The Creative” and “The Receptive” therefore it becomes the number 3. Hexa- gram “The Caldron” as the opposite of hexagram 3 has to be placed at the end of the first part, thus receiving the number 50. This immediately fixes the position of the hexagrams 4 and 49 as the respective reverses of hexa- grams 3 an d 50. 6As it can be easily checked using the two trigrams arrangements. 4Like the hexagrams 17/18 and 53/54. All of them resulting from mutating the lines 3 and 4 or 1 and 6 from hexagrams 11/12. Open Access 202 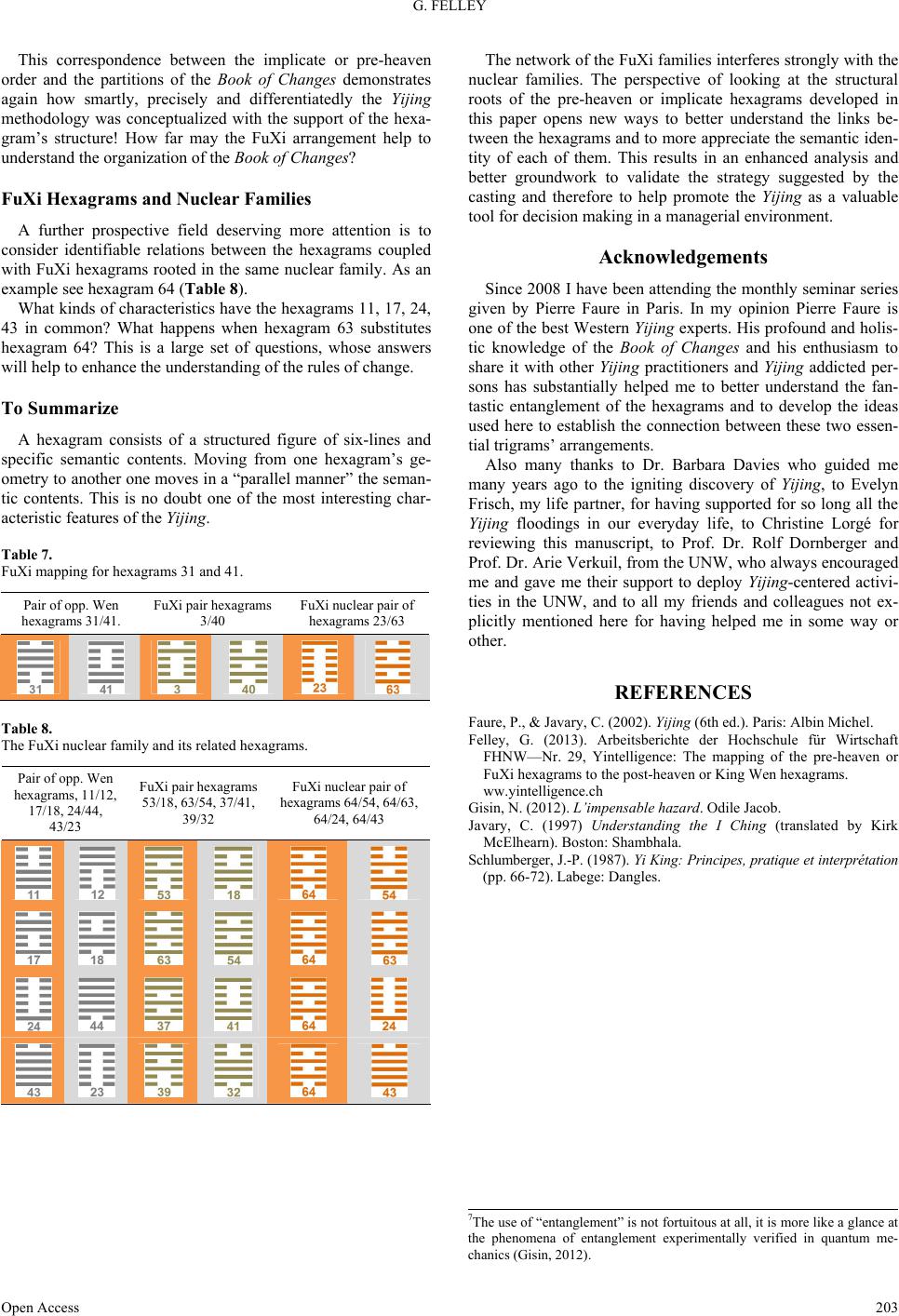 G. FELLEY Open Access 203 This correspondence between the implicate or pre-heaven order and the partitions of the Book of Changes demonstrates again how smartly, precisely and differentiatedly the Yijing methodology was conceptualized with the support of the hexa- gram’s structure! How far may the FuXi arrangement help to understand the organization of the Book of Changes? The network of the FuXi families interferes strongly with the nuclear families. The perspective of looking at the structural roots of the pre-heaven or implicate hexagrams developed in this paper opens new ways to better understand the links be- tween the hexagrams and to more appreciate the semantic iden- tity of each of them. This results in an enhanced analysis and better groundwork to validate the strategy suggested by the casting and therefore to help promote the Yijing as a valuable tool for decision making in a managerial environment. FuXi Hexagrams and Nuclear Families A further prospective field deserving more attention is to consider identifiable relations between the hexagrams coupled with FuXi hexagrams rooted in the same nuclear family. As an example see hexagram 64 (Table 8). Acknowledgements Since 2008 I have been attending the monthly seminar series given by Pierre Faure in Paris. In my opinion Pierre Faure is one of the best Western Yijing experts. His profound and holis- tic knowledge of the Book of Changes and his enthusiasm to share it with other Yijing practitioners and Yijing addicted per- sons has substantially helped me to better understand the fan- tastic entanglement of the hexagrams and to develop the ideas used here to establish the connection between these two essen- tial trigrams’ arrangements. What kinds of characteristics have the hexagrams 11, 17, 24, 43 in common? What happens when hexagram 63 substitutes hexagram 64? This is a large set of questions, whose answers will help to enhance the understanding of the rules of change. To Summarize A hexagram consists of a structured figure of six-lines and specific semantic contents. Moving from one hexagram’s ge- ometry to another one moves in a “parallel manner” the seman- tic contents. This is no doubt one of the most interesting char- acteristic features of the Yijing. Also many thanks to Dr. Barbara Davies who guided me many years ago to the igniting discovery of Yijing, to Evelyn Frisch, my life partner, for having supported for so long all the Yijing floodings in our everyday life, to Christine Lorgé for reviewing this manuscript, to Prof. Dr. Rolf Dornberger and Prof. Dr. Arie Verkuil, from the UNW, who always encouraged me and gave me their support to deploy Yijing-centered activi- ties in the UNW, and to all my friends and colleagues not ex- plicitly mentioned here for having helped me in some way or other. Table 7. FuXi mapping for hexagrams 31 and 41. Pair of opp. Wen hexagrams 31/41. FuXi pair hexagra ms 3/40 FuXi nuclear pair of hexagrams 23/63 REFERENCES Faure, P., & Javary, C. (2002). Yijing (6th ed.). Paris: Albin Michel. Table 8. Felley, G. (2013). Arbeitsberichte der Hochschule für Wirtschaft FHNW—Nr. 29, Yintelligence: The mapping of the pre-heaven or FuXi hexagrams to the post-heaven or King Wen hexagrams. ww.yintelligence.ch The FuXi nuclear family and its related hexagrams. Pair of opp. Wen hexagrams, 11/12, 17/18, 24/ 44, 43/23 FuXi pair hexagrams 53/18, 63/54, 37/41, 39/32 FuXi nuclear pair of hexagrams 64/54, 64/6 3 , 64/24, 64/ 43 Gisin, N. (2012). L’impensable hazard. Odile Jacob. Javary, C. (1997) Understanding the I Ching (translated by Kirk McElhearn). Boston: Shambhala. Schlumberger, J.-P. (1987). Yi King: Prin cipes, pratique et in terprétation (pp. 66-72). Labe ge : D a n gl e s . 7The use of “entanglement” is not fortuitous at all, it is more like a glance at the phenomena of entanglement experimentally verified in quantum me- chanics (Gisin, 2012). |

